2194:
1566:
2189:{\displaystyle {\begin{aligned}m_{1}&={\dfrac {1+5}{2}}=3&&\Rightarrow \;m_{1}^{2}=9\leq 19&&\Rightarrow \;I_{2}=\\m_{2}&={\dfrac {3+5}{2}}=4&&\Rightarrow \;m_{2}^{2}=16\leq 19&&\Rightarrow \;I_{3}=\\m_{3}&={\dfrac {4+5}{2}}=4.5&&\Rightarrow \;m_{3}^{2}=20.25>19&&\Rightarrow \;I_{4}=\\m_{4}&={\dfrac {4+4.5}{2}}=4.25&&\Rightarrow \;m_{4}^{2}=18.0625\leq 19&&\Rightarrow \;I_{5}=\\m_{5}&={\dfrac {4.25+4.5}{2}}=4.375&&\Rightarrow \;m_{5}^{2}=19.140625>19&&\Rightarrow \;I_{5}=\\&\vdots &&\end{aligned}}}
17:
2767:
5898:
1064:
1559:
must certainly found within this interval. Thus, using this interval, one can continue to the next step of the algorithm by calculating the midpoint of the interval, determining whether the square of the midpoint is greater than or less than 19, and setting the boundaries of the next interval
5728:
7374:
3572:
7769:
886:
3879:
2509:
3495:
3578:
Put into words, property 1 means, that the intervals are nested according to their index. The second property formalizes the notion, that interval sizes get arbitrarily small; meaning, that for an arbitrary constant
5011:
2939:
2308:
This procedure can be repeated as many times as needed to attain the desired level of precision. Theoretically, by repeating the steps indefinitely, one can arrive at the true value of this square root.
5893:{\displaystyle I_{n+1}:=\left\{{\begin{matrix}\left&&{\text{if}}\;m_{n}\;{\text{is an upper bound of}}\;A\\\left&&{\text{if}}\;m_{n}\;{\text{is not an upper bound}}\end{matrix}}\right.}
4019:
8036:
7966:
7137:
1571:
4401:
5720:
8097:
7442:
7214:
4288:
824:
2253:
6772:
6421:
5215:
3936:
3780:
3422:
3252:
2417:
2777:
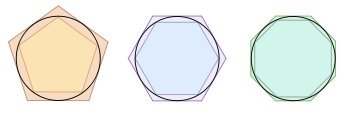
As shown in the image, lower and upper bounds for the circumference of a circle can be obtained with inscribed and circumscribed regular polygons. When examining a circle with diameter
6889:
4912:
758:
7163:
are convergent (and that all convergent sequences are Cauchy sequences) can be proven. This in turn allows for a proof of the completeness property above, showing their equivalence.
3712:
536:
1218:
6997:
5167:
5130:
5093:
4696:
7274:
6525:
4457:
3374:
1488:
346:
Historically - long before anyone defined nested intervals in a textbook - people implicitly constructed such nestings for concrete calculation purposes. For example, the ancient
6131:
7909:
7496:
7086:
7025:
6284:
5658:
5428:
5352:
4174:
4146:
3603:
2973:
446:
341:
295:
6558:
7881:
7657:
6176:
2589:
111:
3501:
6718:
6353:
3643:
3137:
3058:. In contrast to mathematically infinite sequences, an applied computational algorithm terminates at some point, when the desired zero has been found or sufficiently well
2301:
2277:
2220:
1557:
1442:
198:
4087:
2746:
2691:
2343:
1338:
1306:
1279:
1142:
1118:
7397:
7237:
7058:
6480:
6256:
4308:
4235:
2667:
637:
6951:
6447:
5498:
4803:
4655:
3114:
and other sciences. The axiomatic description of nested intervals (or an equivalent axiom) has become an important foundation for the modern understanding of calculus.
7564:
7530:
1372:
5065:
4742:
4516:
4045:
3669:
1533:
1255:
1175:
148:
7795:
7594:
7468:
6831:
6079:
6020:
5994:
5630:
5524:
5274:
4768:
4483:
3311:
3177:
2634:
2369:
1406:
682:
581:
500:
7621:
7266:
6916:
6585:
6223:
5551:
5459:
5380:
5304:
4603:
3807:
2718:
1094:
878:
851:
418:
249:
175:
60:
3020:
2993:
2828:, whose side lengths (and therefore circumference) can be directly calculated from the circle diameter. Furthermore, a way to compute the side length of a regular
2815:
384:
7841:
2849:
7815:
6688:
6668:
6648:
6628:
6605:
6373:
6324:
6304:
6196:
5968:
5948:
5924:
5571:
5400:
5324:
5034:
4953:
4933:
4870:
4850:
4825:
4716:
4623:
4576:
4556:
4536:
4214:
4194:
3956:
3623:
3157:
2889:
2869:
2795:
2371:
even faster. The modern description using nested intervals is similar to the algorithm above, but instead of using a sequence of midpoints, one uses a sequence
601:
218:
1059:{\displaystyle I_{n+1}:=\left\{{\begin{matrix}\left&&{\text{if}}\;\;m_{n}^{2}\leq x\\\left&&{\text{if}}\;\;m_{n}^{2}>x\end{matrix}}\right.}
7669:
3815:
2425:
6423:
is a sequence of nested intervals, the interval lengths get arbitrarily small; in particular, there exists an interval with a length smaller than
3434:
2303:
to be estimated with a greater precision, either by increasing the lower bounds of the interval or decreasing the upper bounds of the interval.
4958:
4245:
This axiom is fundamental in the sense that a sequence of nested intervals does not necessarily contain a rational number - meaning that
3030:
Early uses of sequences of nested intervals (or can be described as such with modern mathematics), can be found in the predecessors of
8305:
468:
as methods for specific calculations. Some variations and modern interpretations of these ancient techniques will be introduced here:
3106:
from the late 1600s has posed a huge challenge for mathematicians trying to prove their methods rigorously; despite their success in
8282:
8253:
8232:
8211:
2894:
2318:
351:
4176:. This contradicts property 2 from the definition of nested intervals; therefore, the intersection can contain at most one number
8153:
7156:
4322:
2998:
Around the year 1600 CE, Archimedes' method was still the gold standard for calculating Pi and was used by Dutch mathematician
8185:
3967:
8300:
7974:
7914:
2891:-gon). By successively doubling the number of edges until reaching 96-sided polygons, Archimedes reached an interval with
2222:
is able to be constricted so that the values that remain within the interval are closer and closer to the actual value of
7091:
4346:
4325:. This means that one of the four has to be introduced axiomatically, while the other three can be successively proven.
5663:
464:
As stated in the introduction, historic users of mathematics discovered the nesting of intervals and closely related
8041:
7402:
7174:
4248:
767:
113:
as an index. In order for a sequence of intervals to be considered nested intervals, two conditions have to be met:
5138:
2225:
6729:
6378:
5172:
3893:
3737:
3379:
3209:
3099:
2374:
390:
6836:
5903:
Note that this interval sequence is well defined and obviously a sequence of nested intervals by construction.
448:). In modern mathematics, nested intervals are used as a construction method for the real numbers (in order to
4875:
1177:, and if the midpoint is larger, one can set it as the upper bound of the next interval. This guarantees that
690:
7369:{\displaystyle I_{n}=\left(0,{\frac {1}{n}}\right)=\left\{x\in \mathbb {R} :0<x<{\frac {1}{n}}\right\}}
6720:. In effect the two are actually equivalent, meaning that either of the two can be introduced axiomatically.
3674:
505:
7240:
3091:
3051:
1180:
6956:
5144:
5107:
5070:
4673:
180:
The length of the intervals get arbitrarily small (meaning the length falls below every possible threshold
6485:
4406:
3782:
is a sequence of nested intervals, there always exists a real number, that is contained in every interval
3316:
1447:
1409:
63:
33:
6698:
As was seen, the existence of suprema and infima of bounded sets is a consequence of the completeness of
8136:
6084:
3079:
3071:
3035:
449:
393:
over all the natural numbers, or, put differently, the set of numbers, that are found in every
Interval
7886:
7473:
7271:
The possibility of an empty intersection can be illustrated by looking at a sequence of open intervals
7063:
7002:
6261:
5635:
5405:
5329:
4151:
4092:
3582:
2944:
1257:
get halved in every step of the recursion. Therefore, it is possible to get lower and upper bounds for
423:
300:
254:
6530:
3964:
This statement can easily be verified by contradiction. Assume that there exist two different numbers
3567:{\displaystyle \quad \forall \varepsilon >0\;\exists N\in \mathbb {N} :\;\;|I_{N}|<\varepsilon }
8263:
Königsberger, Konrad (2003), "2.3 Die Vollständigkeit von R (the completeness of the real numbers)",
7846:
7567:
4314:
3184:
7626:
7216:
over all the naturals (i.e. the set of all points common to each interval) is that it is either the
6136:
2518:
72:
8100:
3726:. In this case a sequence of nested intervals refers to a sequence that only satisfies property 1.
3087:
3055:
2721:
453:
6701:
6332:
3628:
3120:
2282:
2258:
2201:
1538:
1423:
183:
4050:
2999:
2727:
2672:
2324:
1311:
1287:
1260:
1123:
1099:
7382:
7222:
7030:
6452:
6228:
4293:
4219:
3645:. It is also worth noting that property 1 immediately implies that every interval with an index
2749:
2639:
609:
16:
6921:
6426:
5464:
4773:
4628:
8278:
8249:
8228:
8207:
8181:
7535:
7501:
7171:
Without any specifying what is meant by interval, all that can be said about the intersection
3722:
Note that some authors refer to such interval-sequences, satisfying both properties above, as
1343:
8243:
8222:
8201:
5041:
4721:
4488:
4024:
3648:
1493:
1420:
To demonstrate this algorithm, here is an example of how it can be used to find the value of
1223:
1147:
120:
8270:
7774:
7573:
7447:
6777:
6025:
5999:
5973:
5576:
5503:
5220:
4747:
4462:
3257:
3162:
3047:
3043:
3022:, which took him decades. Soon after, more powerful methods for the computation were found.
2594:
2348:
1377:
642:
541:
479:
8264:
7599:
7245:
6894:
6563:
6201:
5529:
5437:
5358:
5282:
4581:
3785:
2696:
1072:
856:
829:
396:
227:
153:
38:
7663:
7160:
4318:
3083:
3046:, sequences of nested intervals is used in algorithms for numerical computation. I.e. the
3005:
2978:
2800:
369:
7820:
2831:
7800:
7764:{\displaystyle I_{n}=\left=\left\{x\in \mathbb {R} :0\leq x\leq {\frac {1}{n}}\right\}}
6673:
6653:
6633:
6613:
6590:
6358:
6309:
6289:
6181:
5953:
5933:
5909:
5556:
5385:
5309:
5019:
4938:
4918:
4855:
4835:
4810:
4701:
4608:
4561:
4541:
4521:
4199:
4179:
3941:
3608:
3142:
2874:
2854:
2780:
586:
362:, in order to get a lower and upper bound for the circles circumference - which is the
203:
67:
4518:. Comparing to the section above, one achieves a sequence of nested intervals for the
8294:
8108:
8104:
3180:
3059:
2773:
can be estimated by computing the perimeters of circumscribed and inscribed polygons.
2255:. That is to say, each successive change in the bounds of the interval within which
1144:. If the midpoint is smaller, one can set it as the lower bound of the next interval
604:
8132:
8115:
there must be something between them. This shows that the intersection of (even an
3095:
3874:{\displaystyle \exists x\in \mathbb {R} :\;x\in \bigcap _{n\in \mathbb {N} }I_{n}}
2748:(as does of course the lower interval bound). This algorithm is a special case of
2504:{\displaystyle c_{n+1}:={\frac {1}{2}}\cdot \left(c_{n}+{\frac {x}{c_{n}}}\right)}
8128:
8116:
7666:. If one changes the situation above by looking at closed intervals of the type
7152:
3111:
3075:
25:
8274:
2821:
355:
2321:
uses an even more efficient algorithm that yields accurate approximations of
8148:
8112:
7217:
761:
465:
347:
4089:
Since both numbers have to be contained in every interval, it follows that
2766:
358:
constructed sequences of polygons, that inscribed and circumscribed a unit
8227:, Dover Books on Mathematics, Courier Dover Publications, pp. 21–22,
3490:{\displaystyle \quad \forall n\in \mathbb {N} :\;\;I_{n+1}\subseteq I_{n}}
7148:
3103:
3039:
3031:
2198:
Each time a new midpoint is calculated, the range of possible values for
8242:
Sohrab, Houshang H. (2003), "Theorem 2.1.5 (Nested
Intervals Theorem)",
8131:
in the plane must have a common intersection. This result was shown by
5096:, that is bounded from below, as the greatest lower bound of that set.
3107:
3074:, nested intervals provide one method of axiomatically introducing the
2825:
5926:
be the number in every interval (whose existence is guaranteed by the
6225:. But this is a contradiction to property 1 of the supremum (meaning
1120:
in order to determine whether the midpoint is smaller or larger than
359:
5006:{\displaystyle \forall \sigma <s:\;\exists x\in A:\;x>\sigma }
8221:
Shilov, Georgi E. (2012), "1.8 The
Principle of Nested Intervals",
1281:
with arbitrarily good precision (given enough computational time).
1220:. With this construction the intervals are nested and their length
7971:
One can also consider the complement of each interval, written as
2765:
6607:, contradicting property 2 of all sequences of nested intervals.
5434:
The construction follows a recursion by starting with any number
117:
Every interval in the sequence is contained in the previous one (
5132:
has a supremum (infimum), if it is bounded from above (below).
2975:
is still often used as a rough, but pragmatic approximation of
2797:, the circumference is (by definition of Pi) the circle number
639:, one can get an even better candidate for the first interval:
8119:
number of) nested, closed, and bounded intervals is nonempty.
2934:{\displaystyle {\tfrac {223}{71}}<\pi <{\tfrac {22}{7}}}
6022:. Furthermore, this would imply the existence of an interval
6918:
is an upper bound. This implies, that the least upper bound
4196:. The completeness axiom guarantees that such a real number
5887:
2761:
1053:
363:
5169:
that has an upper bound. One can now construct a sequence
32:
can be intuitively understood as an ordered collection of
8200:
Fridy, J. A. (2000), "3.3 The Nested
Intervals Theorem",
7797:
one still can always find intervals not containing said
1490:, the first interval for the algorithm can be defined as
1412:
after the desired level of precision has been acquired.
1069:
To put this into words, one can compare the midpoint of
8103:, the complement of the intersection is a union of two
7444:. This result comes from the fact that, for any number
7159:
using nested intervals. In a follow-up, the fact, that
4343:, one can prove that in the real numbers, the equation
5756:
2920:
2899:
914:
389:
The central question to be posed is the nature of the
8044:
7977:
7917:
7889:
7849:
7823:
7803:
7777:
7672:
7629:
7602:
7576:
7538:
7504:
7476:
7450:
7405:
7385:
7277:
7248:
7225:
7177:
7094:
7066:
7033:
7005:
6959:
6924:
6897:
6839:
6780:
6732:
6704:
6676:
6656:
6650:
and that a lower upper bound cannot exist. Therefore
6636:
6616:
6593:
6566:
6533:
6488:
6455:
6429:
6381:
6361:
6335:
6312:
6292:
6264:
6231:
6204:
6184:
6139:
6087:
6028:
6002:
5976:
5956:
5936:
5912:
5731:
5666:
5638:
5579:
5559:
5532:
5506:
5467:
5440:
5408:
5388:
5361:
5332:
5312:
5285:
5223:
5175:
5147:
5110:
5073:
5044:
5022:
4961:
4941:
4921:
4878:
4858:
4838:
4813:
4776:
4750:
4724:
4704:
4676:
4631:
4611:
4584:
4564:
4544:
4524:
4491:
4465:
4409:
4349:
4296:
4251:
4222:
4202:
4182:
4154:
4095:
4053:
4027:
4014:{\displaystyle x,y\in \cap _{n\in \mathbb {N} }I_{n}}
3970:
3944:
3938:
of nested intervals contains exactly one real number
3896:
3818:
3788:
3740:
3677:
3651:
3631:
3611:
3585:
3504:
3437:
3382:
3376:
denotes the length of such an interval. One can call
3319:
3260:
3212:
3165:
3145:
3123:
3008:
2981:
2947:
2897:
2877:
2857:
2834:
2803:
2783:
2730:
2699:
2675:
2642:
2597:
2521:
2428:
2377:
2351:
2327:
2285:
2261:
2228:
2204:
2072:
1952:
1832:
1712:
1592:
1569:
1541:
1496:
1450:
1426:
1380:
1346:
1314:
1290:
1263:
1226:
1183:
1150:
1126:
1102:
1075:
889:
859:
832:
770:
693:
645:
612:
589:
544:
508:
482:
426:
399:
372:
303:
257:
230:
206:
186:
156:
123:
75:
41:
8127:
In two dimensions there is a similar result: nested
8031:{\displaystyle (-\infty ,a_{n})\cup (b_{n},\infty )}
7961:{\displaystyle \cap _{n\in \mathbb {N} }I_{n}=\{0\}}
7570:
of the real numbers. Therefore, no matter how small
2871:-gon can be found, starting at the regular hexagon (
7132:{\displaystyle s\in \cap _{n\in \mathbb {N} }I_{n}}
3086:, being a necessity for discussing the concepts of
2669:, will provide accurate upper and lower bounds for
8091:
8030:
7960:
7903:
7875:
7835:
7809:
7789:
7763:
7651:
7615:
7588:
7558:
7524:
7490:
7462:
7436:
7391:
7368:
7260:
7231:
7208:
7131:
7080:
7052:
7019:
6991:
6945:
6910:
6883:
6825:
6766:
6712:
6682:
6662:
6642:
6622:
6599:
6579:
6552:
6519:
6474:
6441:
6415:
6367:
6347:
6318:
6298:
6278:
6250:
6217:
6190:
6170:
6125:
6073:
6014:
5988:
5962:
5942:
5918:
5892:
5714:
5652:
5624:
5565:
5545:
5518:
5492:
5453:
5422:
5394:
5374:
5346:
5318:
5298:
5268:
5209:
5161:
5124:
5087:
5059:
5028:
5005:
4947:
4927:
4906:
4864:
4844:
4819:
4797:
4762:
4736:
4710:
4690:
4649:
4617:
4597:
4570:
4550:
4530:
4510:
4477:
4451:
4396:{\displaystyle x=y^{j},\;j\in \mathbb {N} ,x>0}
4395:
4302:
4282:
4229:
4208:
4188:
4168:
4140:
4081:
4039:
4013:
3950:
3930:
3873:
3801:
3774:
3706:
3663:
3637:
3625:) with a length strictly smaller than that number
3617:
3597:
3566:
3489:
3416:
3368:
3305:
3246:
3171:
3151:
3131:
3014:
2987:
2967:
2933:
2883:
2863:
2843:
2809:
2789:
2740:
2712:
2685:
2661:
2628:
2583:
2503:
2411:
2363:
2337:
2295:
2271:
2247:
2214:
2188:
1551:
1527:
1482:
1436:
1400:
1366:
1332:
1300:
1273:
1249:
1212:
1169:
1136:
1112:
1088:
1058:
872:
845:
818:
752:
676:
631:
595:
575:
530:
494:
440:
412:
378:
335:
289:
243:
212:
192:
169:
142:
105:
54:
4661:Existence of infimum and supremum in bounded Sets
3809:. In formal notation this axiom guarantees, that
2515:This results in a sequence of intervals given by
7659:implying that the intersection has to be empty.
6931:
6833:be a sequence of nested intervals. Then the set
5045:
4783:
4340:
476:When trying to find the square root of a number
5715:{\displaystyle m_{n}:={\frac {a_{n}+b_{n}}{2}}}
4698:has an upper bound, i.e. there exists a number
4625:-th interval is lower or equal or greater than
4459:. This means there exists a unique real number
3254:be a sequence of closed intervals of the type
224:In other words, the left bound of the interval
8269:, Springer-Lehrbuch, Springer, p. 10-15,
8135:to classify the singular behaviour of certain
8092:{\displaystyle (-\infty ,0)\cup (1/n,\infty )}
7771:, one can see this very clearly. Now for each
7437:{\displaystyle \cap _{n\in \mathbb {N} }I_{n}}
7209:{\displaystyle \cap _{n\in \mathbb {N} }I_{n}}
4283:{\displaystyle \cap _{n\in \mathbb {N} }I_{n}}
819:{\displaystyle m_{n}={\frac {a_{n}+b_{n}}{2}}}
603:has to be found. If one knows the next higher
8203:Introductory Analysis: The Theory of Calculus
6329:Assume that there exists a lower upper bound
2248:{\displaystyle {\sqrt {19}}=4.35889894\dots }
8:
7955:
7949:
7255:
7249:
6878:
6846:
6560:. Following the rules of this construction,
3605:one can always find an interval (with index
6767:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
6416:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
5210:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
3931:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
3775:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
3417:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
3247:{\displaystyle (I_{n})_{n\in \mathbb {N} }}
2412:{\displaystyle (c_{n})_{n\in \mathbb {N} }}
1374:, and the algorithm can be used by setting
20:4 members of a sequence of nested intervals
5927:
5877:
5866:
5817:
5811:
5800:
5036:can exist. Analogously one can define the
4993:
4977:
4894:
4369:
4223:
3836:
3537:
3536:
3518:
3457:
3456:
2141:
2105:
2021:
1985:
1901:
1865:
1781:
1745:
1661:
1625:
1560:accordingly before repeating the process:
1027:
1026:
959:
958:
297:), and the right bound can only decrease (
8072:
8043:
8013:
7994:
7976:
7940:
7930:
7929:
7922:
7916:
7897:
7896:
7888:
7865:
7848:
7822:
7802:
7776:
7746:
7727:
7726:
7697:
7677:
7671:
7640:
7628:
7607:
7601:
7575:
7542:
7537:
7514:
7503:
7484:
7483:
7475:
7449:
7428:
7418:
7417:
7410:
7404:
7384:
7351:
7332:
7331:
7302:
7282:
7276:
7247:
7224:
7200:
7190:
7189:
7182:
7176:
7123:
7113:
7112:
7105:
7093:
7074:
7073:
7065:
7044:
7032:
7013:
7012:
7004:
6983:
6964:
6958:
6923:
6902:
6896:
6884:{\displaystyle A:=\{a_{1},a_{2},\dots \}}
6866:
6853:
6838:
6814:
6801:
6785:
6779:
6758:
6757:
6750:
6740:
6731:
6706:
6705:
6703:
6675:
6655:
6635:
6615:
6592:
6571:
6565:
6538:
6532:
6499:
6487:
6466:
6454:
6428:
6407:
6406:
6399:
6389:
6380:
6360:
6334:
6311:
6291:
6272:
6271:
6263:
6236:
6230:
6209:
6203:
6183:
6144:
6138:
6105:
6092:
6086:
6062:
6049:
6033:
6027:
6001:
5975:
5955:
5935:
5911:
5878:
5871:
5861:
5847:
5834:
5812:
5805:
5795:
5781:
5768:
5755:
5736:
5730:
5700:
5687:
5680:
5671:
5665:
5646:
5645:
5637:
5613:
5600:
5584:
5578:
5558:
5537:
5531:
5505:
5472:
5466:
5445:
5439:
5416:
5415:
5407:
5387:
5366:
5360:
5340:
5339:
5331:
5311:
5290:
5284:
5276:, that has the following two properties:
5257:
5244:
5228:
5222:
5201:
5200:
5193:
5183:
5174:
5155:
5154:
5146:
5118:
5117:
5109:
5081:
5080:
5072:
5043:
5021:
4960:
4940:
4920:
4877:
4857:
4837:
4812:
4775:
4749:
4723:
4703:
4684:
4683:
4675:
4641:
4636:
4630:
4610:
4589:
4583:
4563:
4543:
4523:
4502:
4490:
4464:
4439:
4435:
4421:
4416:
4408:
4377:
4376:
4360:
4348:
4295:
4274:
4264:
4263:
4256:
4250:
4221:
4201:
4181:
4162:
4161:
4153:
4133:
4119:
4111:
4105:
4096:
4094:
4068:
4054:
4052:
4026:
4005:
3995:
3994:
3987:
3969:
3943:
3922:
3921:
3914:
3904:
3895:
3865:
3855:
3854:
3847:
3829:
3828:
3817:
3793:
3787:
3766:
3765:
3758:
3748:
3739:
3693:
3687:
3678:
3676:
3650:
3630:
3610:
3584:
3553:
3547:
3538:
3529:
3528:
3503:
3481:
3462:
3449:
3448:
3436:
3408:
3407:
3400:
3390:
3381:
3360:
3347:
3335:
3329:
3320:
3318:
3294:
3281:
3265:
3259:
3238:
3237:
3230:
3220:
3211:
3164:
3144:
3125:
3124:
3122:
3007:
2980:
2951:
2946:
2919:
2898:
2896:
2876:
2856:
2833:
2802:
2782:
2731:
2729:
2704:
2698:
2676:
2674:
2647:
2641:
2602:
2596:
2570:
2555:
2546:
2526:
2520:
2488:
2479:
2470:
2448:
2433:
2427:
2403:
2402:
2395:
2385:
2376:
2350:
2328:
2326:
2286:
2284:
2262:
2260:
2229:
2227:
2205:
2203:
2146:
2115:
2110:
2071:
2058:
2026:
1995:
1990:
1951:
1938:
1906:
1875:
1870:
1831:
1818:
1786:
1755:
1750:
1711:
1698:
1666:
1635:
1630:
1591:
1578:
1570:
1568:
1542:
1540:
1501:
1495:
1474:
1455:
1449:
1427:
1425:
1390:
1379:
1350:
1345:
1313:
1291:
1289:
1264:
1262:
1242:
1236:
1227:
1225:
1198:
1184:
1182:
1155:
1149:
1127:
1125:
1103:
1101:
1080:
1074:
1037:
1032:
1021:
1007:
994:
969:
964:
953:
939:
926:
913:
894:
888:
864:
858:
837:
831:
804:
791:
784:
775:
769:
746:
745:
727:
714:
698:
692:
650:
644:
617:
611:
588:
549:
543:
515:
507:
481:
434:
433:
425:
404:
398:
371:
327:
308:
302:
281:
262:
256:
235:
229:
205:
185:
161:
155:
128:
122:
74:
46:
40:
4907:{\displaystyle \forall x\in A:\;x\leq s}
3002:, to compute more than thirty digits of
764:by looking at the sequence of midpoints
753:{\displaystyle I_{n}=,n\in \mathbb {N} }
15:
8168:
7911:. One can conclude that, in this case,
7239:, a point on the number line (called a
3707:{\displaystyle |I_{n}|<\varepsilon }
531:{\displaystyle 1\leq {\sqrt {x}}\leq x}
3183:, meaning the axioms of order and the
1213:{\displaystyle {\sqrt {x}}\in I_{n+1}}
7167:Further discussion of related aspects
6992:{\displaystyle a_{n}\leq s\leq b_{n}}
6610:In two steps, it has been shown that
5162:{\displaystyle A\subset \mathbb {R} }
5125:{\displaystyle A\subset \mathbb {R} }
5088:{\displaystyle B\subset \mathbb {R} }
4691:{\displaystyle A\subset \mathbb {R} }
4578:, by looking at whether the midpoint
4315:existence of the infimum and supremum
7:
8266:Analysis 1, 6. Auflage (6th edition)
8224:Elementary Real and Complex Analysis
6520:{\displaystyle s-a_{n}<s-\sigma }
4452:{\displaystyle y={\sqrt{x}}=x^{1/j}}
4310:, if only considering the rationals.
3369:{\displaystyle |I_{n}|:=b_{n}-a_{n}}
3066:The construction of the real numbers
1483:{\displaystyle 1^{2}<19<5^{2}}
6891:is bounded from above, where every
6587:would have to be an upper bound of
5461:, that is not an upper bound (e.g.
2762:Pi § Polygon approximation era
354:of numbers. In contrast, the famed
8083:
8051:
8022:
7984:
7386:
7226:
6126:{\displaystyle b_{m}-a_{m}<x-s}
5970:, otherwise there exists a number
4978:
4962:
4879:
4317:(proof below), the convergence of
4297:
3890:The intersection of each sequence
3819:
3519:
3506:
3439:
3104:differential and integral calculus
14:
8038:- which, in our last example, is
7904:{\displaystyle n\in \mathbb {N} }
7491:{\displaystyle n\in \mathbb {N} }
7081:{\displaystyle n\in \mathbb {N} }
7020:{\displaystyle n\in \mathbb {N} }
6279:{\displaystyle m\in \mathbb {N} }
5653:{\displaystyle n\in \mathbb {N} }
5423:{\displaystyle n\in \mathbb {N} }
5347:{\displaystyle n\in \mathbb {N} }
4169:{\displaystyle n\in \mathbb {N} }
4141:{\displaystyle |I_{n}|\geq |x-y|}
3598:{\displaystyle \varepsilon >0}
2968:{\displaystyle 22/7\approx 3.143}
2755:
538:, which gives the first interval
441:{\displaystyle n\in \mathbb {N} }
352:method for computing square roots
336:{\displaystyle b_{n+1}\leq b_{n}}
290:{\displaystyle a_{n+1}\geq a_{n}}
7596:, one can always find intervals
7153:accumulation points of sequences
6553:{\displaystyle a_{n}>\sigma }
4330:Direct consequences of the axiom
3117:In the context of this article,
3050:can be used for calculating the
7876:{\displaystyle 0\leq x\leq 1/n}
7662:The situation is different for
4313:The axiom is equivalent to the
4047:it follows that they differ by
3505:
3438:
8206:, Academic Press, p. 29,
8086:
8066:
8060:
8045:
8025:
8006:
8000:
7978:
7652:{\displaystyle x\notin I_{n},}
7399:results from the intersection
6940:
6934:
6820:
6794:
6747:
6733:
6396:
6382:
6171:{\displaystyle b_{m}-s<x-s}
6068:
6042:
5619:
5593:
5263:
5237:
5190:
5176:
5054:
5048:
4792:
4786:
4339:By generalizing the algorithm
4134:
4120:
4112:
4097:
4069:
4055:
3911:
3897:
3755:
3741:
3694:
3679:
3554:
3539:
3397:
3383:
3336:
3321:
3300:
3274:
3227:
3213:
2756:Archimedes' circle measurement
2623:
2611:
2584:{\displaystyle I_{n+1}:=\left}
2392:
2378:
2167:
2155:
2138:
2102:
2047:
2035:
2018:
1982:
1927:
1915:
1898:
1862:
1807:
1795:
1778:
1742:
1687:
1675:
1658:
1622:
1522:
1510:
1243:
1228:
853:is already known (starting at
733:
707:
671:
659:
570:
558:
106:{\displaystyle n=1,2,3,\dots }
1:
8176:Königsberger, Konrad (2004).
8154:Cantor's intersection theorem
6306:is in fact an upper bound of
5660:one can compute the midpoint
5526:and an arbitrary upper bound
2693:very fast. In practice, only
2279:must lie allows the value of
7379:In this case, the empty set
7147:After formally defining the
6713:{\displaystyle \mathbb {R} }
6348:{\displaystyle \sigma <s}
4935:is the least upper bound of
4341:shown above for square roots
3638:{\displaystyle \varepsilon }
3426:sequence of nested intervals
3132:{\displaystyle \mathbb {R} }
2720:has to be considered, which
2296:{\displaystyle {\sqrt {19}}}
2272:{\displaystyle {\sqrt {19}}}
2215:{\displaystyle {\sqrt {19}}}
1552:{\displaystyle {\sqrt {19}}}
1437:{\displaystyle {\sqrt {19}}}
193:{\displaystyle \varepsilon }
30:sequence of nested intervals
7623:in the sequence, such that
7470:there exists some value of
7157:Bolzano–Weierstrass theorem
5382:is never an upper bound of
4323:Bolzano–Weierstrass theorem
4082:{\displaystyle |x-y|>0.}
2741:{\displaystyle {\sqrt {x}}}
2686:{\displaystyle {\sqrt {x}}}
2338:{\displaystyle {\sqrt {x}}}
1333:{\displaystyle 0<y<1}
1301:{\displaystyle {\sqrt {y}}}
1274:{\displaystyle {\sqrt {x}}}
1137:{\displaystyle {\sqrt {x}}}
1113:{\displaystyle {\sqrt {x}}}
472:Computation of square roots
8322:
7392:{\displaystyle \emptyset }
7232:{\displaystyle \emptyset }
7053:{\displaystyle s\in I_{n}}
6475:{\displaystyle s\in I_{n}}
6251:{\displaystyle b_{m}<s}
5139:Without loss of generality
4770:, one can call the number
4303:{\displaystyle \emptyset }
4230:{\displaystyle \;\square }
3724:shrinking nested intervals
2759:
2662:{\displaystyle k^{2}>x}
632:{\displaystyle k^{2}>x}
502:, one can be certain that
8306:Theorems in real analysis
8275:10.1007/978-3-642-18490-1
7155:, one can also prove the
6946:{\displaystyle s=\sup(A)}
6442:{\displaystyle s-\sigma }
6198:also being an element of
5493:{\displaystyle a_{1}=c-1}
4798:{\displaystyle s=\sup(A)}
4650:{\displaystyle m_{n}^{k}}
4403:can always be solved for
3100:Gottfried Wilhelm Leibniz
8248:, Springer, p. 45,
8180:. Springer. p. 11.
7559:{\displaystyle 1/n<x}
7525:{\displaystyle n>1/x}
7149:convergence of sequences
3671:must also have a length
1367:{\displaystyle 1/y>1}
7566:. This is given by the
5060:{\displaystyle \inf(B)}
4737:{\displaystyle x\leq b}
4511:{\displaystyle x=y^{k}}
4040:{\displaystyle x\neq y}
3664:{\displaystyle n\geq N}
2851:-gon from the previous
1528:{\displaystyle I_{1}:=}
1250:{\displaystyle |I_{n}|}
1170:{\displaystyle I_{n+1}}
143:{\displaystyle I_{n+1}}
8137:differential equations
8093:
8032:
7962:
7905:
7877:
7837:
7811:
7791:
7790:{\displaystyle x>0}
7765:
7653:
7617:
7590:
7589:{\displaystyle x>0}
7560:
7526:
7492:
7464:
7463:{\displaystyle x>0}
7438:
7393:
7370:
7262:
7233:
7210:
7133:
7082:
7054:
7021:
6993:
6947:
6912:
6885:
6827:
6826:{\displaystyle I_{n}=}
6768:
6714:
6684:
6664:
6644:
6624:
6601:
6581:
6554:
6521:
6476:
6443:
6417:
6369:
6349:
6320:
6300:
6280:
6252:
6219:
6192:
6172:
6127:
6075:
6074:{\displaystyle I_{m}=}
6016:
6015:{\displaystyle x>s}
5990:
5989:{\displaystyle x\in A}
5964:
5944:
5920:
5894:
5716:
5654:
5626:
5625:{\displaystyle I_{n}=}
5567:
5547:
5520:
5519:{\displaystyle c\in A}
5494:
5455:
5424:
5396:
5376:
5348:
5320:
5300:
5270:
5269:{\displaystyle I_{n}=}
5211:
5163:
5141:one can look at a set
5126:
5089:
5061:
5030:
5007:
4949:
4929:
4908:
4866:
4846:
4821:
4799:
4764:
4763:{\displaystyle x\in A}
4738:
4712:
4692:
4651:
4619:
4599:
4572:
4552:
4532:
4512:
4479:
4478:{\displaystyle y>0}
4453:
4397:
4304:
4284:
4231:
4210:
4190:
4170:
4142:
4083:
4041:
4015:
3952:
3932:
3875:
3803:
3776:
3708:
3665:
3639:
3619:
3599:
3568:
3491:
3418:
3370:
3307:
3306:{\displaystyle I_{n}=}
3248:
3173:
3172:{\displaystyle \cdot }
3153:
3133:
3016:
2989:
2969:
2935:
2885:
2865:
2845:
2822:Archimedes of Syracuse
2811:
2791:
2774:
2742:
2714:
2687:
2663:
2630:
2629:{\displaystyle I_{1}=}
2585:
2505:
2413:
2365:
2364:{\displaystyle x>0}
2339:
2297:
2273:
2249:
2216:
2190:
1553:
1529:
1484:
1438:
1402:
1401:{\displaystyle x:=1/y}
1368:
1334:
1302:
1275:
1251:
1214:
1171:
1138:
1114:
1090:
1060:
874:
847:
820:
754:
678:
677:{\displaystyle I_{1}=}
633:
597:
577:
576:{\displaystyle I_{1}=}
532:
496:
495:{\displaystyle x>1}
456:of rational numbers).
442:
414:
380:
337:
291:
245:
214:
200:after a certain index
194:
171:
150:is always a subset of
144:
107:
56:
21:
8094:
8033:
7963:
7906:
7878:
7838:
7812:
7792:
7766:
7654:
7618:
7616:{\displaystyle I_{n}}
7591:
7561:
7527:
7493:
7465:
7439:
7394:
7371:
7268:), or some interval.
7263:
7261:{\displaystyle \{x\}}
7234:
7211:
7134:
7083:
7055:
7022:
6994:
6948:
6913:
6911:{\displaystyle b_{n}}
6886:
6828:
6769:
6715:
6685:
6665:
6645:
6630:is an upper bound of
6625:
6602:
6582:
6580:{\displaystyle a_{n}}
6555:
6522:
6477:
6444:
6418:
6370:
6350:
6321:
6301:
6281:
6253:
6220:
6218:{\displaystyle I_{m}}
6193:
6173:
6128:
6076:
6017:
5991:
5965:
5950:is an upper bound of
5945:
5921:
5895:
5880:is not an upper bound
5717:
5655:
5627:
5568:
5548:
5546:{\displaystyle b_{1}}
5521:
5495:
5456:
5454:{\displaystyle a_{1}}
5425:
5397:
5377:
5375:{\displaystyle a_{n}}
5349:
5321:
5306:is an upper bound of
5301:
5299:{\displaystyle b_{n}}
5271:
5212:
5164:
5127:
5090:
5062:
5031:
5016:Only one such number
5008:
4950:
4930:
4909:
4867:
4852:is an upper bound of
4847:
4822:
4800:
4765:
4739:
4713:
4693:
4652:
4620:
4600:
4598:{\displaystyle m_{n}}
4573:
4553:
4533:
4513:
4480:
4454:
4398:
4305:
4285:
4232:
4211:
4191:
4171:
4143:
4084:
4042:
4016:
3953:
3933:
3876:
3804:
3802:{\displaystyle I_{n}}
3777:
3730:Axiom of completeness
3709:
3666:
3640:
3620:
3600:
3569:
3492:
3419:
3371:
3308:
3249:
3174:
3154:
3134:
3072:mathematical analysis
3026:Other implementations
3017:
2990:
2970:
2936:
2886:
2866:
2846:
2824:started with regular
2812:
2792:
2769:
2760:Further information:
2743:
2715:
2713:{\displaystyle c_{n}}
2688:
2664:
2631:
2586:
2506:
2414:
2366:
2340:
2298:
2274:
2250:
2217:
2191:
1554:
1530:
1485:
1439:
1403:
1369:
1335:
1303:
1284:One can also compute
1276:
1252:
1215:
1172:
1139:
1115:
1091:
1089:{\displaystyle I_{n}}
1061:
875:
873:{\displaystyle I_{1}}
848:
846:{\displaystyle I_{n}}
826:. Given the interval
821:
755:
679:
634:
598:
578:
533:
497:
443:
415:
413:{\displaystyle I_{n}}
381:
338:
292:
246:
244:{\displaystyle I_{n}}
215:
195:
172:
170:{\displaystyle I_{n}}
145:
108:
57:
55:{\displaystyle I_{n}}
19:
8301:Sets of real numbers
8042:
7975:
7915:
7887:
7847:
7821:
7801:
7775:
7670:
7627:
7600:
7574:
7568:Archimedean property
7536:
7502:
7474:
7448:
7403:
7383:
7275:
7246:
7223:
7175:
7143:Further consequences
7092:
7064:
7031:
7003:
6957:
6922:
6895:
6837:
6778:
6730:
6702:
6674:
6654:
6634:
6614:
6591:
6564:
6531:
6486:
6453:
6427:
6379:
6359:
6333:
6310:
6290:
6262:
6229:
6202:
6182:
6137:
6085:
6026:
6000:
5974:
5954:
5934:
5910:
5814:is an upper bound of
5729:
5664:
5636:
5577:
5557:
5530:
5504:
5465:
5438:
5406:
5386:
5359:
5330:
5310:
5283:
5221:
5217:of nested intervals
5173:
5145:
5108:
5071:
5042:
5020:
4959:
4939:
4919:
4876:
4856:
4836:
4811:
4774:
4748:
4722:
4702:
4674:
4629:
4609:
4582:
4562:
4542:
4522:
4489:
4463:
4407:
4347:
4294:
4249:
4220:
4200:
4180:
4152:
4093:
4051:
4025:
3968:
3942:
3894:
3816:
3786:
3738:
3675:
3649:
3629:
3609:
3583:
3502:
3435:
3380:
3317:
3258:
3210:
3185:Archimedean property
3163:
3143:
3139:in conjunction with
3121:
3056:continuous functions
3015:{\displaystyle \pi }
3006:
2988:{\displaystyle \pi }
2979:
2945:
2895:
2875:
2855:
2832:
2810:{\displaystyle \pi }
2801:
2781:
2728:
2697:
2673:
2640:
2595:
2519:
2426:
2375:
2349:
2325:
2283:
2259:
2226:
2202:
1567:
1539:
1494:
1448:
1424:
1408:and calculating the
1378:
1344:
1312:
1288:
1261:
1224:
1181:
1148:
1124:
1100:
1073:
887:
857:
830:
768:
691:
687:The other intervals
643:
610:
587:
542:
506:
480:
424:
397:
379:{\displaystyle \pi }
370:
301:
255:
228:
204:
184:
154:
121:
73:
39:
8245:Basic Real Analysis
7883:holds true for any
7836:{\displaystyle x=0}
6670:is the supremum of
4646:
2120:
2000:
1880:
1760:
1640:
1042:
974:
760:can now be defined
460:Historic motivation
251:can only increase (
8105:disjoint open sets
8089:
8028:
7958:
7901:
7873:
7833:
7807:
7787:
7761:
7649:
7613:
7586:
7556:
7522:
7488:
7460:
7434:
7389:
7366:
7258:
7229:
7206:
7129:
7078:
7050:
7017:
6989:
6943:
6908:
6881:
6823:
6764:
6710:
6680:
6660:
6640:
6620:
6597:
6577:
6550:
6517:
6472:
6439:
6413:
6365:
6345:
6316:
6296:
6276:
6248:
6215:
6188:
6168:
6123:
6071:
6012:
5986:
5960:
5940:
5916:
5890:
5885:
5712:
5650:
5622:
5563:
5543:
5516:
5490:
5451:
5420:
5392:
5372:
5344:
5316:
5296:
5266:
5207:
5159:
5122:
5085:
5057:
5026:
5003:
4945:
4925:
4904:
4862:
4842:
4817:
4795:
4760:
4734:
4708:
4688:
4647:
4632:
4615:
4595:
4568:
4548:
4528:
4508:
4475:
4449:
4393:
4335:Existence of roots
4300:
4280:
4227:
4206:
4186:
4166:
4138:
4079:
4037:
4011:
3948:
3928:
3871:
3860:
3799:
3772:
3704:
3661:
3635:
3615:
3595:
3564:
3487:
3414:
3366:
3303:
3244:
3179:is an Archimedean
3169:
3149:
3129:
3012:
3000:Ludolph van Ceulen
2985:
2965:
2941:. The upper bound
2931:
2929:
2908:
2881:
2861:
2844:{\displaystyle 2n}
2841:
2807:
2787:
2775:
2738:
2710:
2683:
2659:
2626:
2581:
2501:
2409:
2361:
2335:
2293:
2269:
2245:
2212:
2186:
2184:
2106:
2089:
1986:
1969:
1866:
1849:
1746:
1729:
1626:
1609:
1549:
1525:
1480:
1434:
1398:
1364:
1330:
1298:
1271:
1247:
1210:
1167:
1134:
1110:
1086:
1056:
1051:
1028:
960:
880:), one can define
870:
843:
816:
750:
674:
629:
593:
573:
528:
492:
438:
410:
376:
333:
287:
241:
210:
190:
167:
140:
103:
52:
22:
8123:Higher dimensions
7810:{\displaystyle x}
7754:
7705:
7359:
7310:
6683:{\displaystyle A}
6663:{\displaystyle s}
6643:{\displaystyle A}
6623:{\displaystyle s}
6600:{\displaystyle A}
6368:{\displaystyle A}
6319:{\displaystyle A}
6299:{\displaystyle s}
6191:{\displaystyle s}
5963:{\displaystyle A}
5943:{\displaystyle s}
5919:{\displaystyle s}
5881:
5864:
5815:
5798:
5710:
5566:{\displaystyle A}
5395:{\displaystyle A}
5319:{\displaystyle A}
5029:{\displaystyle s}
4948:{\displaystyle A}
4928:{\displaystyle s}
4865:{\displaystyle A}
4845:{\displaystyle s}
4820:{\displaystyle A}
4711:{\displaystyle b}
4618:{\displaystyle n}
4571:{\displaystyle y}
4551:{\displaystyle x}
4531:{\displaystyle k}
4426:
4209:{\displaystyle x}
4189:{\displaystyle x}
3951:{\displaystyle x}
3843:
3618:{\displaystyle N}
3152:{\displaystyle +}
3092:differentiability
2928:
2907:
2884:{\displaystyle 6}
2864:{\displaystyle n}
2790:{\displaystyle 1}
2736:
2681:
2561:
2494:
2456:
2333:
2319:Babylonian method
2291:
2267:
2234:
2210:
2088:
1968:
1848:
1728:
1608:
1547:
1444:. Note that since
1432:
1296:
1269:
1189:
1132:
1108:
1024:
956:
814:
596:{\displaystyle x}
520:
213:{\displaystyle N}
8313:
8287:
8258:
8237:
8216:
8192:
8191:
8173:
8101:De Morgan's laws
8098:
8096:
8095:
8090:
8076:
8037:
8035:
8034:
8029:
8018:
8017:
7999:
7998:
7967:
7965:
7964:
7959:
7945:
7944:
7935:
7934:
7933:
7910:
7908:
7907:
7902:
7900:
7882:
7880:
7879:
7874:
7869:
7842:
7840:
7839:
7834:
7816:
7814:
7813:
7808:
7796:
7794:
7793:
7788:
7770:
7768:
7767:
7762:
7760:
7756:
7755:
7747:
7730:
7711:
7707:
7706:
7698:
7682:
7681:
7664:closed intervals
7658:
7656:
7655:
7650:
7645:
7644:
7622:
7620:
7619:
7614:
7612:
7611:
7595:
7593:
7592:
7587:
7565:
7563:
7562:
7557:
7546:
7531:
7529:
7528:
7523:
7518:
7497:
7495:
7494:
7489:
7487:
7469:
7467:
7466:
7461:
7443:
7441:
7440:
7435:
7433:
7432:
7423:
7422:
7421:
7398:
7396:
7395:
7390:
7375:
7373:
7372:
7367:
7365:
7361:
7360:
7352:
7335:
7316:
7312:
7311:
7303:
7287:
7286:
7267:
7265:
7264:
7259:
7238:
7236:
7235:
7230:
7215:
7213:
7212:
7207:
7205:
7204:
7195:
7194:
7193:
7161:Cauchy sequences
7138:
7136:
7135:
7130:
7128:
7127:
7118:
7117:
7116:
7087:
7085:
7084:
7079:
7077:
7059:
7057:
7056:
7051:
7049:
7048:
7026:
7024:
7023:
7018:
7016:
6998:
6996:
6995:
6990:
6988:
6987:
6969:
6968:
6952:
6950:
6949:
6944:
6917:
6915:
6914:
6909:
6907:
6906:
6890:
6888:
6887:
6882:
6871:
6870:
6858:
6857:
6832:
6830:
6829:
6824:
6819:
6818:
6806:
6805:
6790:
6789:
6773:
6771:
6770:
6765:
6763:
6762:
6761:
6745:
6744:
6719:
6717:
6716:
6711:
6709:
6689:
6687:
6686:
6681:
6669:
6667:
6666:
6661:
6649:
6647:
6646:
6641:
6629:
6627:
6626:
6621:
6606:
6604:
6603:
6598:
6586:
6584:
6583:
6578:
6576:
6575:
6559:
6557:
6556:
6551:
6543:
6542:
6526:
6524:
6523:
6518:
6504:
6503:
6481:
6479:
6478:
6473:
6471:
6470:
6448:
6446:
6445:
6440:
6422:
6420:
6419:
6414:
6412:
6411:
6410:
6394:
6393:
6374:
6372:
6371:
6366:
6354:
6352:
6351:
6346:
6325:
6323:
6322:
6317:
6305:
6303:
6302:
6297:
6285:
6283:
6282:
6277:
6275:
6257:
6255:
6254:
6249:
6241:
6240:
6224:
6222:
6221:
6216:
6214:
6213:
6197:
6195:
6194:
6189:
6178:follows, due to
6177:
6175:
6174:
6169:
6149:
6148:
6132:
6130:
6129:
6124:
6110:
6109:
6097:
6096:
6080:
6078:
6077:
6072:
6067:
6066:
6054:
6053:
6038:
6037:
6021:
6019:
6018:
6013:
5995:
5993:
5992:
5987:
5969:
5967:
5966:
5961:
5949:
5947:
5946:
5941:
5925:
5923:
5922:
5917:
5899:
5897:
5896:
5891:
5889:
5886:
5882:
5879:
5876:
5875:
5865:
5862:
5859:
5857:
5853:
5852:
5851:
5839:
5838:
5816:
5813:
5810:
5809:
5799:
5796:
5793:
5791:
5787:
5786:
5785:
5773:
5772:
5747:
5746:
5721:
5719:
5718:
5713:
5711:
5706:
5705:
5704:
5692:
5691:
5681:
5676:
5675:
5659:
5657:
5656:
5651:
5649:
5631:
5629:
5628:
5623:
5618:
5617:
5605:
5604:
5589:
5588:
5572:
5570:
5569:
5564:
5552:
5550:
5549:
5544:
5542:
5541:
5525:
5523:
5522:
5517:
5499:
5497:
5496:
5491:
5477:
5476:
5460:
5458:
5457:
5452:
5450:
5449:
5429:
5427:
5426:
5421:
5419:
5401:
5399:
5398:
5393:
5381:
5379:
5378:
5373:
5371:
5370:
5353:
5351:
5350:
5345:
5343:
5325:
5323:
5322:
5317:
5305:
5303:
5302:
5297:
5295:
5294:
5275:
5273:
5272:
5267:
5262:
5261:
5249:
5248:
5233:
5232:
5216:
5214:
5213:
5208:
5206:
5205:
5204:
5188:
5187:
5168:
5166:
5165:
5160:
5158:
5131:
5129:
5128:
5123:
5121:
5094:
5092:
5091:
5086:
5084:
5066:
5064:
5063:
5058:
5035:
5033:
5032:
5027:
5012:
5010:
5009:
5004:
4954:
4952:
4951:
4946:
4934:
4932:
4931:
4926:
4913:
4911:
4910:
4905:
4871:
4869:
4868:
4863:
4851:
4849:
4848:
4843:
4826:
4824:
4823:
4818:
4804:
4802:
4801:
4796:
4769:
4767:
4766:
4761:
4743:
4741:
4740:
4735:
4717:
4715:
4714:
4709:
4697:
4695:
4694:
4689:
4687:
4656:
4654:
4653:
4648:
4645:
4640:
4624:
4622:
4621:
4616:
4604:
4602:
4601:
4596:
4594:
4593:
4577:
4575:
4574:
4569:
4557:
4555:
4554:
4549:
4537:
4535:
4534:
4529:
4517:
4515:
4514:
4509:
4507:
4506:
4484:
4482:
4481:
4476:
4458:
4456:
4455:
4450:
4448:
4447:
4443:
4427:
4425:
4417:
4402:
4400:
4399:
4394:
4380:
4365:
4364:
4319:Cauchy sequences
4309:
4307:
4306:
4301:
4289:
4287:
4286:
4281:
4279:
4278:
4269:
4268:
4267:
4236:
4234:
4233:
4228:
4215:
4213:
4212:
4207:
4195:
4193:
4192:
4187:
4175:
4173:
4172:
4167:
4165:
4147:
4145:
4144:
4139:
4137:
4123:
4115:
4110:
4109:
4100:
4088:
4086:
4085:
4080:
4072:
4058:
4046:
4044:
4043:
4038:
4020:
4018:
4017:
4012:
4010:
4009:
4000:
3999:
3998:
3957:
3955:
3954:
3949:
3937:
3935:
3934:
3929:
3927:
3926:
3925:
3909:
3908:
3880:
3878:
3877:
3872:
3870:
3869:
3859:
3858:
3832:
3808:
3806:
3805:
3800:
3798:
3797:
3781:
3779:
3778:
3773:
3771:
3770:
3769:
3753:
3752:
3713:
3711:
3710:
3705:
3697:
3692:
3691:
3682:
3670:
3668:
3667:
3662:
3644:
3642:
3641:
3636:
3624:
3622:
3621:
3616:
3604:
3602:
3601:
3596:
3573:
3571:
3570:
3565:
3557:
3552:
3551:
3542:
3532:
3496:
3494:
3493:
3488:
3486:
3485:
3473:
3472:
3452:
3423:
3421:
3420:
3415:
3413:
3412:
3411:
3395:
3394:
3375:
3373:
3372:
3367:
3365:
3364:
3352:
3351:
3339:
3334:
3333:
3324:
3312:
3310:
3309:
3304:
3299:
3298:
3286:
3285:
3270:
3269:
3253:
3251:
3250:
3245:
3243:
3242:
3241:
3225:
3224:
3203:
3202:
3198:
3178:
3176:
3175:
3170:
3158:
3156:
3155:
3150:
3138:
3136:
3135:
3130:
3128:
3102:'s discovery of
3094:. Historically,
3084:rational numbers
3048:Bisection method
3044:computer science
3021:
3019:
3018:
3013:
2994:
2992:
2991:
2986:
2974:
2972:
2971:
2966:
2955:
2940:
2938:
2937:
2932:
2930:
2921:
2909:
2900:
2890:
2888:
2887:
2882:
2870:
2868:
2867:
2862:
2850:
2848:
2847:
2842:
2816:
2814:
2813:
2808:
2796:
2794:
2793:
2788:
2772:
2747:
2745:
2744:
2739:
2737:
2732:
2719:
2717:
2716:
2711:
2709:
2708:
2692:
2690:
2689:
2684:
2682:
2677:
2668:
2666:
2665:
2660:
2652:
2651:
2635:
2633:
2632:
2627:
2607:
2606:
2590:
2588:
2587:
2582:
2580:
2576:
2575:
2574:
2562:
2560:
2559:
2547:
2537:
2536:
2510:
2508:
2507:
2502:
2500:
2496:
2495:
2493:
2492:
2480:
2475:
2474:
2457:
2449:
2444:
2443:
2418:
2416:
2415:
2410:
2408:
2407:
2406:
2390:
2389:
2370:
2368:
2367:
2362:
2344:
2342:
2341:
2336:
2334:
2329:
2302:
2300:
2299:
2294:
2292:
2287:
2278:
2276:
2275:
2270:
2268:
2263:
2254:
2252:
2251:
2246:
2235:
2230:
2221:
2219:
2218:
2213:
2211:
2206:
2195:
2193:
2192:
2187:
2185:
2182:
2181:
2173:
2151:
2150:
2134:
2119:
2114:
2098:
2090:
2084:
2073:
2063:
2062:
2031:
2030:
2014:
1999:
1994:
1978:
1970:
1964:
1953:
1943:
1942:
1911:
1910:
1894:
1879:
1874:
1858:
1850:
1844:
1833:
1823:
1822:
1791:
1790:
1774:
1759:
1754:
1738:
1730:
1724:
1713:
1703:
1702:
1671:
1670:
1654:
1639:
1634:
1618:
1610:
1604:
1593:
1583:
1582:
1558:
1556:
1555:
1550:
1548:
1543:
1534:
1532:
1531:
1526:
1506:
1505:
1489:
1487:
1486:
1481:
1479:
1478:
1460:
1459:
1443:
1441:
1440:
1435:
1433:
1428:
1407:
1405:
1404:
1399:
1394:
1373:
1371:
1370:
1365:
1354:
1339:
1337:
1336:
1331:
1307:
1305:
1304:
1299:
1297:
1292:
1280:
1278:
1277:
1272:
1270:
1265:
1256:
1254:
1253:
1248:
1246:
1241:
1240:
1231:
1219:
1217:
1216:
1211:
1209:
1208:
1190:
1185:
1176:
1174:
1173:
1168:
1166:
1165:
1143:
1141:
1140:
1135:
1133:
1128:
1119:
1117:
1116:
1111:
1109:
1104:
1095:
1093:
1092:
1087:
1085:
1084:
1065:
1063:
1062:
1057:
1055:
1052:
1041:
1036:
1025:
1022:
1019:
1017:
1013:
1012:
1011:
999:
998:
973:
968:
957:
954:
951:
949:
945:
944:
943:
931:
930:
905:
904:
879:
877:
876:
871:
869:
868:
852:
850:
849:
844:
842:
841:
825:
823:
822:
817:
815:
810:
809:
808:
796:
795:
785:
780:
779:
759:
757:
756:
751:
749:
732:
731:
719:
718:
703:
702:
683:
681:
680:
675:
655:
654:
638:
636:
635:
630:
622:
621:
602:
600:
599:
594:
582:
580:
579:
574:
554:
553:
537:
535:
534:
529:
521:
516:
501:
499:
498:
493:
447:
445:
444:
439:
437:
419:
417:
416:
411:
409:
408:
385:
383:
382:
377:
364:circle number Pi
342:
340:
339:
334:
332:
331:
319:
318:
296:
294:
293:
288:
286:
285:
273:
272:
250:
248:
247:
242:
240:
239:
219:
217:
216:
211:
199:
197:
196:
191:
176:
174:
173:
168:
166:
165:
149:
147:
146:
141:
139:
138:
112:
110:
109:
104:
64:real number line
61:
59:
58:
53:
51:
50:
8321:
8320:
8316:
8315:
8314:
8312:
8311:
8310:
8291:
8290:
8285:
8262:
8256:
8241:
8235:
8220:
8214:
8199:
8196:
8195:
8188:
8175:
8174:
8170:
8165:
8159:
8145:
8125:
8040:
8039:
8009:
7990:
7973:
7972:
7936:
7918:
7913:
7912:
7885:
7884:
7845:
7844:
7843:, the property
7819:
7818:
7799:
7798:
7773:
7772:
7719:
7715:
7690:
7686:
7673:
7668:
7667:
7636:
7625:
7624:
7603:
7598:
7597:
7572:
7571:
7534:
7533:
7500:
7499:
7472:
7471:
7446:
7445:
7424:
7406:
7401:
7400:
7381:
7380:
7324:
7320:
7295:
7291:
7278:
7273:
7272:
7244:
7243:
7221:
7220:
7196:
7178:
7173:
7172:
7169:
7145:
7119:
7101:
7090:
7089:
7088:, respectively
7062:
7061:
7040:
7029:
7028:
7001:
7000:
6979:
6960:
6955:
6954:
6920:
6919:
6898:
6893:
6892:
6862:
6849:
6835:
6834:
6810:
6797:
6781:
6776:
6775:
6746:
6736:
6728:
6727:
6700:
6699:
6696:
6690:by definition.
6672:
6671:
6652:
6651:
6632:
6631:
6612:
6611:
6589:
6588:
6567:
6562:
6561:
6534:
6529:
6528:
6495:
6484:
6483:
6462:
6451:
6450:
6425:
6424:
6395:
6385:
6377:
6376:
6357:
6356:
6331:
6330:
6308:
6307:
6288:
6287:
6260:
6259:
6232:
6227:
6226:
6205:
6200:
6199:
6180:
6179:
6140:
6135:
6134:
6101:
6088:
6083:
6082:
6058:
6045:
6029:
6024:
6023:
5998:
5997:
5972:
5971:
5952:
5951:
5932:
5931:
5908:
5907:
5884:
5883:
5867:
5858:
5843:
5830:
5829:
5825:
5822:
5821:
5801:
5792:
5777:
5764:
5763:
5759:
5751:
5732:
5727:
5726:
5696:
5683:
5682:
5667:
5662:
5661:
5634:
5633:
5609:
5596:
5580:
5575:
5574:
5555:
5554:
5533:
5528:
5527:
5502:
5501:
5468:
5463:
5462:
5441:
5436:
5435:
5404:
5403:
5384:
5383:
5362:
5357:
5356:
5328:
5327:
5308:
5307:
5286:
5281:
5280:
5253:
5240:
5224:
5219:
5218:
5189:
5179:
5171:
5170:
5143:
5142:
5106:
5105:
5102:
5069:
5068:
5040:
5039:
5018:
5017:
4957:
4956:
4937:
4936:
4917:
4916:
4874:
4873:
4854:
4853:
4834:
4833:
4809:
4808:
4772:
4771:
4746:
4745:
4720:
4719:
4700:
4699:
4672:
4671:
4668:
4663:
4627:
4626:
4607:
4606:
4585:
4580:
4579:
4560:
4559:
4540:
4539:
4520:
4519:
4498:
4487:
4486:
4461:
4460:
4431:
4405:
4404:
4356:
4345:
4344:
4337:
4332:
4292:
4291:
4270:
4252:
4247:
4246:
4242:
4218:
4217:
4198:
4197:
4178:
4177:
4150:
4149:
4101:
4091:
4090:
4049:
4048:
4023:
4022:
4001:
3983:
3966:
3965:
3940:
3939:
3910:
3900:
3892:
3891:
3888:
3861:
3814:
3813:
3789:
3784:
3783:
3754:
3744:
3736:
3735:
3732:
3720:
3683:
3673:
3672:
3647:
3646:
3627:
3626:
3607:
3606:
3581:
3580:
3543:
3500:
3499:
3477:
3458:
3433:
3432:
3396:
3386:
3378:
3377:
3356:
3343:
3325:
3315:
3314:
3290:
3277:
3261:
3256:
3255:
3226:
3216:
3208:
3207:
3204:
3200:
3196:
3194:
3193:
3161:
3160:
3141:
3140:
3119:
3118:
3068:
3036:differentiation
3028:
3004:
3003:
2977:
2976:
2943:
2942:
2893:
2892:
2873:
2872:
2853:
2852:
2830:
2829:
2820:Around 250 BCE
2799:
2798:
2779:
2778:
2770:
2764:
2758:
2750:Newton's method
2726:
2725:
2700:
2695:
2694:
2671:
2670:
2643:
2638:
2637:
2598:
2593:
2592:
2566:
2551:
2545:
2541:
2522:
2517:
2516:
2484:
2466:
2465:
2461:
2429:
2424:
2423:
2391:
2381:
2373:
2372:
2347:
2346:
2323:
2322:
2315:
2281:
2280:
2257:
2256:
2224:
2223:
2200:
2199:
2183:
2180:
2171:
2170:
2142:
2133:
2097:
2074:
2064:
2054:
2051:
2050:
2022:
2013:
1977:
1954:
1944:
1934:
1931:
1930:
1902:
1893:
1857:
1834:
1824:
1814:
1811:
1810:
1782:
1773:
1737:
1714:
1704:
1694:
1691:
1690:
1662:
1653:
1617:
1594:
1584:
1574:
1565:
1564:
1537:
1536:
1497:
1492:
1491:
1470:
1451:
1446:
1445:
1422:
1421:
1418:
1376:
1375:
1342:
1341:
1340:. In this case
1310:
1309:
1286:
1285:
1259:
1258:
1232:
1222:
1221:
1194:
1179:
1178:
1151:
1146:
1145:
1122:
1121:
1098:
1097:
1076:
1071:
1070:
1050:
1049:
1018:
1003:
990:
989:
985:
982:
981:
950:
935:
922:
921:
917:
909:
890:
885:
884:
860:
855:
854:
833:
828:
827:
800:
787:
786:
771:
766:
765:
723:
710:
694:
689:
688:
646:
641:
640:
613:
608:
607:
585:
584:
545:
540:
539:
504:
503:
478:
477:
474:
462:
422:
421:
420:(thus, for all
400:
395:
394:
368:
367:
323:
304:
299:
298:
277:
258:
253:
252:
231:
226:
225:
202:
201:
182:
181:
157:
152:
151:
124:
119:
118:
71:
70:
68:natural numbers
42:
37:
36:
12:
11:
5:
8319:
8317:
8309:
8308:
8303:
8293:
8292:
8289:
8288:
8283:
8260:
8254:
8239:
8233:
8218:
8212:
8194:
8193:
8186:
8167:
8166:
8164:
8161:
8157:
8156:
8151:
8144:
8141:
8124:
8121:
8088:
8085:
8082:
8079:
8075:
8071:
8068:
8065:
8062:
8059:
8056:
8053:
8050:
8047:
8027:
8024:
8021:
8016:
8012:
8008:
8005:
8002:
7997:
7993:
7989:
7986:
7983:
7980:
7957:
7954:
7951:
7948:
7943:
7939:
7932:
7928:
7925:
7921:
7899:
7895:
7892:
7872:
7868:
7864:
7861:
7858:
7855:
7852:
7832:
7829:
7826:
7806:
7786:
7783:
7780:
7759:
7753:
7750:
7745:
7742:
7739:
7736:
7733:
7729:
7725:
7722:
7718:
7714:
7710:
7704:
7701:
7696:
7693:
7689:
7685:
7680:
7676:
7648:
7643:
7639:
7635:
7632:
7610:
7606:
7585:
7582:
7579:
7555:
7552:
7549:
7545:
7541:
7521:
7517:
7513:
7510:
7507:
7486:
7482:
7479:
7459:
7456:
7453:
7431:
7427:
7420:
7416:
7413:
7409:
7388:
7364:
7358:
7355:
7350:
7347:
7344:
7341:
7338:
7334:
7330:
7327:
7323:
7319:
7315:
7309:
7306:
7301:
7298:
7294:
7290:
7285:
7281:
7257:
7254:
7251:
7228:
7203:
7199:
7192:
7188:
7185:
7181:
7168:
7165:
7144:
7141:
7126:
7122:
7115:
7111:
7108:
7104:
7100:
7097:
7076:
7072:
7069:
7047:
7043:
7039:
7036:
7015:
7011:
7008:
6986:
6982:
6978:
6975:
6972:
6967:
6963:
6942:
6939:
6936:
6933:
6930:
6927:
6905:
6901:
6880:
6877:
6874:
6869:
6865:
6861:
6856:
6852:
6848:
6845:
6842:
6822:
6817:
6813:
6809:
6804:
6800:
6796:
6793:
6788:
6784:
6760:
6756:
6753:
6749:
6743:
6739:
6735:
6708:
6695:
6692:
6679:
6659:
6639:
6619:
6596:
6574:
6570:
6549:
6546:
6541:
6537:
6527:and therefore
6516:
6513:
6510:
6507:
6502:
6498:
6494:
6491:
6469:
6465:
6461:
6458:
6438:
6435:
6432:
6409:
6405:
6402:
6398:
6392:
6388:
6384:
6364:
6344:
6341:
6338:
6315:
6295:
6274:
6270:
6267:
6247:
6244:
6239:
6235:
6212:
6208:
6187:
6167:
6164:
6161:
6158:
6155:
6152:
6147:
6143:
6122:
6119:
6116:
6113:
6108:
6104:
6100:
6095:
6091:
6070:
6065:
6061:
6057:
6052:
6048:
6044:
6041:
6036:
6032:
6011:
6008:
6005:
5985:
5982:
5979:
5959:
5939:
5915:
5901:
5900:
5888:
5874:
5870:
5860:
5856:
5850:
5846:
5842:
5837:
5833:
5828:
5824:
5823:
5820:
5808:
5804:
5794:
5790:
5784:
5780:
5776:
5771:
5767:
5762:
5758:
5757:
5754:
5750:
5745:
5742:
5739:
5735:
5709:
5703:
5699:
5695:
5690:
5686:
5679:
5674:
5670:
5648:
5644:
5641:
5621:
5616:
5612:
5608:
5603:
5599:
5595:
5592:
5587:
5583:
5562:
5540:
5536:
5515:
5512:
5509:
5489:
5486:
5483:
5480:
5475:
5471:
5448:
5444:
5432:
5431:
5418:
5414:
5411:
5391:
5369:
5365:
5354:
5342:
5338:
5335:
5315:
5293:
5289:
5265:
5260:
5256:
5252:
5247:
5243:
5239:
5236:
5231:
5227:
5203:
5199:
5196:
5192:
5186:
5182:
5178:
5157:
5153:
5150:
5120:
5116:
5113:
5101:
5098:
5083:
5079:
5076:
5056:
5053:
5050:
5047:
5025:
5014:
5013:
5002:
4999:
4996:
4992:
4989:
4986:
4983:
4980:
4976:
4973:
4970:
4967:
4964:
4944:
4924:
4914:
4903:
4900:
4897:
4893:
4890:
4887:
4884:
4881:
4861:
4841:
4816:
4794:
4791:
4788:
4785:
4782:
4779:
4759:
4756:
4753:
4733:
4730:
4727:
4707:
4686:
4682:
4679:
4667:
4664:
4662:
4659:
4644:
4639:
4635:
4614:
4592:
4588:
4567:
4547:
4527:
4505:
4501:
4497:
4494:
4474:
4471:
4468:
4446:
4442:
4438:
4434:
4430:
4424:
4420:
4415:
4412:
4392:
4389:
4386:
4383:
4379:
4375:
4372:
4368:
4363:
4359:
4355:
4352:
4336:
4333:
4331:
4328:
4327:
4326:
4311:
4299:
4277:
4273:
4266:
4262:
4259:
4255:
4241:
4238:
4226:
4205:
4185:
4164:
4160:
4157:
4136:
4132:
4129:
4126:
4122:
4118:
4114:
4108:
4104:
4099:
4078:
4075:
4071:
4067:
4064:
4061:
4057:
4036:
4033:
4030:
4008:
4004:
3997:
3993:
3990:
3986:
3982:
3979:
3976:
3973:
3947:
3924:
3920:
3917:
3913:
3907:
3903:
3899:
3887:
3884:
3883:
3882:
3868:
3864:
3857:
3853:
3850:
3846:
3842:
3839:
3835:
3831:
3827:
3824:
3821:
3796:
3792:
3768:
3764:
3761:
3757:
3751:
3747:
3743:
3731:
3728:
3719:
3716:
3703:
3700:
3696:
3690:
3686:
3681:
3660:
3657:
3654:
3634:
3614:
3594:
3591:
3588:
3576:
3575:
3563:
3560:
3556:
3550:
3546:
3541:
3535:
3531:
3527:
3524:
3521:
3517:
3514:
3511:
3508:
3497:
3484:
3480:
3476:
3471:
3468:
3465:
3461:
3455:
3451:
3447:
3444:
3441:
3410:
3406:
3403:
3399:
3393:
3389:
3385:
3363:
3359:
3355:
3350:
3346:
3342:
3338:
3332:
3328:
3323:
3302:
3297:
3293:
3289:
3284:
3280:
3276:
3273:
3268:
3264:
3240:
3236:
3233:
3229:
3223:
3219:
3215:
3192:
3189:
3168:
3148:
3127:
3067:
3064:
3027:
3024:
3011:
2984:
2964:
2961:
2958:
2954:
2950:
2927:
2924:
2918:
2915:
2912:
2906:
2903:
2880:
2860:
2840:
2837:
2806:
2786:
2757:
2754:
2735:
2707:
2703:
2680:
2658:
2655:
2650:
2646:
2625:
2622:
2619:
2616:
2613:
2610:
2605:
2601:
2579:
2573:
2569:
2565:
2558:
2554:
2550:
2544:
2540:
2535:
2532:
2529:
2525:
2513:
2512:
2499:
2491:
2487:
2483:
2478:
2473:
2469:
2464:
2460:
2455:
2452:
2447:
2442:
2439:
2436:
2432:
2405:
2401:
2398:
2394:
2388:
2384:
2380:
2360:
2357:
2354:
2332:
2314:
2311:
2310:
2309:
2306:
2304:
2290:
2266:
2244:
2241:
2238:
2233:
2209:
2196:
2179:
2176:
2174:
2172:
2169:
2166:
2163:
2160:
2157:
2154:
2149:
2145:
2140:
2137:
2135:
2132:
2129:
2126:
2123:
2118:
2113:
2109:
2104:
2101:
2099:
2096:
2093:
2087:
2083:
2080:
2077:
2070:
2067:
2065:
2061:
2057:
2053:
2052:
2049:
2046:
2043:
2040:
2037:
2034:
2029:
2025:
2020:
2017:
2015:
2012:
2009:
2006:
2003:
1998:
1993:
1989:
1984:
1981:
1979:
1976:
1973:
1967:
1963:
1960:
1957:
1950:
1947:
1945:
1941:
1937:
1933:
1932:
1929:
1926:
1923:
1920:
1917:
1914:
1909:
1905:
1900:
1897:
1895:
1892:
1889:
1886:
1883:
1878:
1873:
1869:
1864:
1861:
1859:
1856:
1853:
1847:
1843:
1840:
1837:
1830:
1827:
1825:
1821:
1817:
1813:
1812:
1809:
1806:
1803:
1800:
1797:
1794:
1789:
1785:
1780:
1777:
1775:
1772:
1769:
1766:
1763:
1758:
1753:
1749:
1744:
1741:
1739:
1736:
1733:
1727:
1723:
1720:
1717:
1710:
1707:
1705:
1701:
1697:
1693:
1692:
1689:
1686:
1683:
1680:
1677:
1674:
1669:
1665:
1660:
1657:
1655:
1652:
1649:
1646:
1643:
1638:
1633:
1629:
1624:
1621:
1619:
1616:
1613:
1607:
1603:
1600:
1597:
1590:
1587:
1585:
1581:
1577:
1573:
1572:
1546:
1524:
1521:
1518:
1515:
1512:
1509:
1504:
1500:
1477:
1473:
1469:
1466:
1463:
1458:
1454:
1431:
1417:
1414:
1397:
1393:
1389:
1386:
1383:
1363:
1360:
1357:
1353:
1349:
1329:
1326:
1323:
1320:
1317:
1295:
1268:
1245:
1239:
1235:
1230:
1207:
1204:
1201:
1197:
1193:
1188:
1164:
1161:
1158:
1154:
1131:
1107:
1083:
1079:
1067:
1066:
1054:
1048:
1045:
1040:
1035:
1031:
1020:
1016:
1010:
1006:
1002:
997:
993:
988:
984:
983:
980:
977:
972:
967:
963:
952:
948:
942:
938:
934:
929:
925:
920:
916:
915:
912:
908:
903:
900:
897:
893:
867:
863:
840:
836:
813:
807:
803:
799:
794:
790:
783:
778:
774:
748:
744:
741:
738:
735:
730:
726:
722:
717:
713:
709:
706:
701:
697:
673:
670:
667:
664:
661:
658:
653:
649:
628:
625:
620:
616:
605:perfect square
592:
572:
569:
566:
563:
560:
557:
552:
548:
527:
524:
519:
514:
511:
491:
488:
485:
473:
470:
461:
458:
436:
432:
429:
407:
403:
375:
330:
326:
322:
317:
314:
311:
307:
284:
280:
276:
271:
268:
265:
261:
238:
234:
222:
221:
209:
189:
178:
164:
160:
137:
134:
131:
127:
102:
99:
96:
93:
90:
87:
84:
81:
78:
49:
45:
13:
10:
9:
6:
4:
3:
2:
8318:
8307:
8304:
8302:
8299:
8298:
8296:
8286:
8284:9783642184901
8280:
8276:
8272:
8268:
8267:
8261:
8257:
8255:9780817642112
8251:
8247:
8246:
8240:
8236:
8234:9780486135007
8230:
8226:
8225:
8219:
8215:
8213:9780122676550
8209:
8205:
8204:
8198:
8197:
8189:
8183:
8179:
8172:
8169:
8162:
8160:
8155:
8152:
8150:
8147:
8146:
8142:
8140:
8138:
8134:
8130:
8122:
8120:
8118:
8114:
8110:
8109:connectedness
8106:
8102:
8080:
8077:
8073:
8069:
8063:
8057:
8054:
8048:
8019:
8014:
8010:
8003:
7995:
7991:
7987:
7981:
7969:
7952:
7946:
7941:
7937:
7926:
7923:
7919:
7893:
7890:
7870:
7866:
7862:
7859:
7856:
7853:
7850:
7830:
7827:
7824:
7804:
7784:
7781:
7778:
7757:
7751:
7748:
7743:
7740:
7737:
7734:
7731:
7723:
7720:
7716:
7712:
7708:
7702:
7699:
7694:
7691:
7687:
7683:
7678:
7674:
7665:
7660:
7646:
7641:
7637:
7633:
7630:
7608:
7604:
7583:
7580:
7577:
7569:
7553:
7550:
7547:
7543:
7539:
7532:), such that
7519:
7515:
7511:
7508:
7505:
7480:
7477:
7457:
7454:
7451:
7429:
7425:
7414:
7411:
7407:
7377:
7362:
7356:
7353:
7348:
7345:
7342:
7339:
7336:
7328:
7325:
7321:
7317:
7313:
7307:
7304:
7299:
7296:
7292:
7288:
7283:
7279:
7269:
7252:
7242:
7219:
7201:
7197:
7186:
7183:
7179:
7166:
7164:
7162:
7158:
7154:
7150:
7142:
7140:
7124:
7120:
7109:
7106:
7102:
7098:
7095:
7070:
7067:
7045:
7041:
7037:
7034:
7009:
7006:
6984:
6980:
6976:
6973:
6970:
6965:
6961:
6937:
6928:
6925:
6903:
6899:
6875:
6872:
6867:
6863:
6859:
6854:
6850:
6843:
6840:
6815:
6811:
6807:
6802:
6798:
6791:
6786:
6782:
6754:
6751:
6741:
6737:
6725:
6721:
6693:
6691:
6677:
6657:
6637:
6617:
6608:
6594:
6572:
6568:
6547:
6544:
6539:
6535:
6514:
6511:
6508:
6505:
6500:
6496:
6492:
6489:
6467:
6463:
6459:
6456:
6436:
6433:
6430:
6403:
6400:
6390:
6386:
6362:
6342:
6339:
6336:
6327:
6313:
6293:
6286:). Therefore
6268:
6265:
6245:
6242:
6237:
6233:
6210:
6206:
6185:
6165:
6162:
6159:
6156:
6153:
6150:
6145:
6141:
6133:, from which
6120:
6117:
6114:
6111:
6106:
6102:
6098:
6093:
6089:
6063:
6059:
6055:
6050:
6046:
6039:
6034:
6030:
6009:
6006:
6003:
5983:
5980:
5977:
5957:
5937:
5929:
5913:
5904:
5872:
5868:
5854:
5848:
5844:
5840:
5835:
5831:
5826:
5818:
5806:
5802:
5788:
5782:
5778:
5774:
5769:
5765:
5760:
5752:
5748:
5743:
5740:
5737:
5733:
5725:
5724:
5723:
5707:
5701:
5697:
5693:
5688:
5684:
5677:
5672:
5668:
5642:
5639:
5614:
5610:
5606:
5601:
5597:
5590:
5585:
5581:
5560:
5538:
5534:
5513:
5510:
5507:
5487:
5484:
5481:
5478:
5473:
5469:
5446:
5442:
5412:
5409:
5389:
5367:
5363:
5355:
5336:
5333:
5313:
5291:
5287:
5279:
5278:
5277:
5258:
5254:
5250:
5245:
5241:
5234:
5229:
5225:
5197:
5194:
5184:
5180:
5151:
5148:
5140:
5137:
5133:
5114:
5111:
5099:
5097:
5095:
5077:
5074:
5051:
5023:
5000:
4997:
4994:
4990:
4987:
4984:
4981:
4974:
4971:
4968:
4965:
4942:
4922:
4915:
4901:
4898:
4895:
4891:
4888:
4885:
4882:
4859:
4839:
4831:
4830:
4829:
4827:
4814:
4789:
4780:
4777:
4757:
4754:
4751:
4731:
4728:
4725:
4705:
4680:
4677:
4665:
4660:
4658:
4642:
4637:
4633:
4612:
4590:
4586:
4565:
4545:
4525:
4503:
4499:
4495:
4492:
4472:
4469:
4466:
4444:
4440:
4436:
4432:
4428:
4422:
4418:
4413:
4410:
4390:
4387:
4384:
4381:
4373:
4370:
4366:
4361:
4357:
4353:
4350:
4342:
4334:
4329:
4324:
4320:
4316:
4312:
4275:
4271:
4260:
4257:
4253:
4244:
4243:
4239:
4237:
4224:
4203:
4183:
4158:
4155:
4130:
4127:
4124:
4116:
4106:
4102:
4076:
4073:
4065:
4062:
4059:
4034:
4031:
4028:
4006:
4002:
3991:
3988:
3984:
3980:
3977:
3974:
3971:
3963:
3959:
3945:
3918:
3915:
3905:
3901:
3885:
3866:
3862:
3851:
3848:
3844:
3840:
3837:
3833:
3825:
3822:
3812:
3811:
3810:
3794:
3790:
3762:
3759:
3749:
3745:
3729:
3727:
3725:
3717:
3715:
3701:
3698:
3688:
3684:
3658:
3655:
3652:
3632:
3612:
3592:
3589:
3586:
3561:
3558:
3548:
3544:
3533:
3525:
3522:
3515:
3512:
3509:
3498:
3482:
3478:
3474:
3469:
3466:
3463:
3459:
3453:
3445:
3442:
3431:
3430:
3429:
3427:
3404:
3401:
3391:
3387:
3361:
3357:
3353:
3348:
3344:
3340:
3330:
3326:
3295:
3291:
3287:
3282:
3278:
3271:
3266:
3262:
3234:
3231:
3221:
3217:
3199:
3190:
3188:
3186:
3182:
3181:ordered field
3166:
3146:
3115:
3113:
3109:
3105:
3101:
3097:
3093:
3089:
3085:
3081:
3077:
3073:
3065:
3063:
3061:
3057:
3053:
3049:
3045:
3041:
3037:
3033:
3025:
3023:
3009:
3001:
2996:
2982:
2962:
2959:
2956:
2952:
2948:
2925:
2922:
2916:
2913:
2910:
2904:
2901:
2878:
2858:
2838:
2835:
2827:
2823:
2818:
2804:
2784:
2768:
2763:
2753:
2751:
2733:
2723:
2705:
2701:
2678:
2656:
2653:
2648:
2644:
2620:
2617:
2614:
2608:
2603:
2599:
2577:
2571:
2567:
2563:
2556:
2552:
2548:
2542:
2538:
2533:
2530:
2527:
2523:
2497:
2489:
2485:
2481:
2476:
2471:
2467:
2462:
2458:
2453:
2450:
2445:
2440:
2437:
2434:
2430:
2422:
2421:
2420:
2399:
2396:
2386:
2382:
2358:
2355:
2352:
2330:
2320:
2313:Herons method
2312:
2307:
2305:
2288:
2264:
2242:
2239:
2236:
2231:
2207:
2197:
2177:
2175:
2164:
2161:
2158:
2152:
2147:
2143:
2136:
2130:
2127:
2124:
2121:
2116:
2111:
2107:
2100:
2094:
2091:
2085:
2081:
2078:
2075:
2068:
2066:
2059:
2055:
2044:
2041:
2038:
2032:
2027:
2023:
2016:
2010:
2007:
2004:
2001:
1996:
1991:
1987:
1980:
1974:
1971:
1965:
1961:
1958:
1955:
1948:
1946:
1939:
1935:
1924:
1921:
1918:
1912:
1907:
1903:
1896:
1890:
1887:
1884:
1881:
1876:
1871:
1867:
1860:
1854:
1851:
1845:
1841:
1838:
1835:
1828:
1826:
1819:
1815:
1804:
1801:
1798:
1792:
1787:
1783:
1776:
1770:
1767:
1764:
1761:
1756:
1751:
1747:
1740:
1734:
1731:
1725:
1721:
1718:
1715:
1708:
1706:
1699:
1695:
1684:
1681:
1678:
1672:
1667:
1663:
1656:
1650:
1647:
1644:
1641:
1636:
1631:
1627:
1620:
1614:
1611:
1605:
1601:
1598:
1595:
1588:
1586:
1579:
1575:
1563:
1562:
1561:
1544:
1519:
1516:
1513:
1507:
1502:
1498:
1475:
1471:
1467:
1464:
1461:
1456:
1452:
1429:
1415:
1413:
1411:
1395:
1391:
1387:
1384:
1381:
1361:
1358:
1355:
1351:
1347:
1327:
1324:
1321:
1318:
1315:
1293:
1282:
1266:
1237:
1233:
1205:
1202:
1199:
1195:
1191:
1186:
1162:
1159:
1156:
1152:
1129:
1105:
1081:
1077:
1046:
1043:
1038:
1033:
1029:
1014:
1008:
1004:
1000:
995:
991:
986:
978:
975:
970:
965:
961:
946:
940:
936:
932:
927:
923:
918:
910:
906:
901:
898:
895:
891:
883:
882:
881:
865:
861:
838:
834:
811:
805:
801:
797:
792:
788:
781:
776:
772:
763:
742:
739:
736:
728:
724:
720:
715:
711:
704:
699:
695:
685:
668:
665:
662:
656:
651:
647:
626:
623:
618:
614:
606:
590:
567:
564:
561:
555:
550:
546:
525:
522:
517:
512:
509:
489:
486:
483:
471:
469:
467:
459:
457:
455:
451:
430:
427:
405:
401:
392:
387:
373:
365:
361:
357:
353:
350:discovered a
349:
344:
328:
324:
320:
315:
312:
309:
305:
282:
278:
274:
269:
266:
263:
259:
236:
232:
207:
187:
179:
162:
158:
135:
132:
129:
125:
116:
115:
114:
100:
97:
94:
91:
88:
85:
82:
79:
76:
69:
65:
47:
43:
35:
31:
27:
18:
8265:
8244:
8223:
8202:
8177:
8171:
8158:
8133:Hermann Weyl
8129:closed disks
8126:
7970:
7661:
7498:(namely any
7378:
7270:
7170:
7146:
7027:. Therefore
6723:
6722:
6697:
6609:
6328:
5996:, such that
5905:
5902:
5433:
5135:
5134:
5103:
5037:
5015:
4807:supremum of
4806:
4718:, such that
4669:
4538:-th root of
4485:, such that
4338:
4290:could yield
3961:
3960:
3889:
3733:
3723:
3721:
3577:
3425:
3205:
3116:
3096:Isaac Newton
3076:real numbers
3069:
3060:approximated
3029:
2997:
2819:
2776:
2514:
2316:
1419:
1283:
1068:
686:
475:
463:
391:intersection
388:
345:
223:
29:
23:
8117:uncountable
6449:. But from
5722:and define
5067:) of a set
4832:the number
3112:engineering
3040:integration
762:recursively
583:, in which
348:Babylonians
26:mathematics
8295:Categories
8187:354040371X
8178:Analysis 1
8163:References
7817:, but for
4955:, meaning
4872:, meaning
4666:Definition
3191:Definition
3088:continuity
3080:completion
2240:4.35889894
1410:reciprocal
466:algorithms
356:Archimedes
8149:Bisection
8113:real line
8107:. By the
8084:∞
8064:∪
8052:∞
8049:−
8023:∞
8004:∪
7985:∞
7982:−
7927:∈
7920:∩
7894:∈
7860:≤
7854:≤
7744:≤
7738:≤
7724:∈
7634:∉
7481:∈
7415:∈
7408:∩
7387:∅
7329:∈
7241:singleton
7227:∅
7218:empty set
7187:∈
7180:∩
7110:∈
7103:∩
7099:∈
7071:∈
7038:∈
7010:∈
6977:≤
6971:≤
6953:fulfills
6876:…
6755:∈
6548:σ
6515:σ
6512:−
6493:−
6482:one gets
6460:∈
6437:σ
6434:−
6404:∈
6337:σ
6269:∈
6163:−
6151:−
6118:−
6099:−
5981:∈
5643:∈
5632:for some
5573:). Given
5511:∈
5485:−
5413:∈
5337:∈
5198:∈
5152:⊂
5115:⊂
5104:Each set
5078:⊂
5038:infimum (
5001:σ
4985:∈
4979:∃
4966:σ
4963:∀
4899:≤
4886:∈
4880:∀
4755:∈
4729:≤
4681:⊂
4558:, namely
4374:∈
4298:∅
4261:∈
4254:∩
4225:◻
4159:∈
4128:−
4117:≥
4063:−
4032:≠
3992:∈
3985:∩
3981:∈
3919:∈
3852:∈
3845:⋂
3841:∈
3826:∈
3820:∃
3763:∈
3702:ε
3656:≥
3633:ε
3587:ε
3562:ε
3526:∈
3520:∃
3510:ε
3507:∀
3475:⊆
3446:∈
3440:∀
3405:∈
3354:−
3235:∈
3167:⋅
3010:π
2983:π
2960:≈
2914:π
2805:π
2722:converges
2459:⋅
2419:given by
2400:∈
2243:…
2178:⋮
2139:⇒
2125:19.140625
2103:⇒
2019:⇒
2008:≤
1983:⇒
1899:⇒
1863:⇒
1779:⇒
1768:≤
1743:⇒
1659:⇒
1648:≤
1623:⇒
1192:∈
976:≤
743:∈
523:≤
513:≤
431:∈
374:π
321:≤
275:≥
188:ε
101:…
34:intervals
8143:See also
7060:for all
6999:for all
6375:. Since
6258:for all
5906:Now let
5500:, where
5402:for any
5326:for all
4744:for all
4321:and the
4216:exists.
4148:for all
3313:, where
3032:calculus
2826:hexagons
2636:, where
1535:, since
450:complete
8111:of the
5100:Theorem
4605:of the
4021:. From
3886:Theorem
3108:physics
3098:'s and
3082:of the
3078:as the
2345:for an
2005:18.0625
1416:Example
1308:, when
62:on the
8281:
8252:
8231:
8210:
8184:
6724:Proof:
6694:Remark
5136:Proof:
4828:, if
3962:Proof:
3718:Remark
3195:": -->
3187:hold.
3042:). In
360:circle
8099:. By
6774:with
6081:with
5928:axiom
4240:Notes
3428:, if
3052:roots
2963:3.143
2165:4.375
2095:4.375
1885:20.25
454:field
66:with
8279:ISBN
8250:ISBN
8229:ISBN
8208:ISBN
8182:ISBN
7782:>
7581:>
7551:<
7509:>
7455:>
7349:<
7343:<
7151:and
6726:Let
6545:>
6506:<
6340:<
6243:<
6157:<
6112:<
6007:>
4998:>
4969:<
4805:the
4470:>
4388:>
4074:>
3699:<
3590:>
3559:<
3513:>
3206:Let
3197:edit
3159:and
3090:and
3038:and
2917:<
2911:<
2654:>
2591:and
2356:>
2317:The
2159:4.25
2128:>
2076:4.25
2039:4.25
1975:4.25
1888:>
1468:<
1462:<
1359:>
1325:<
1319:<
1044:>
624:>
487:>
452:the
28:, a
8271:doi
6932:sup
6355:of
5930:).
5553:of
5046:inf
4784:sup
4670:If
3734:If
3070:In
3054:of
2902:223
2817:.
2724:to
2082:4.5
2045:4.5
1962:4.5
1925:4.5
1855:4.5
1096:to
386:).
343:).
24:In
8297::
8277:,
8139:.
7968:.
7376:.
7139:.
6844::=
6326:.
5863:if
5797:if
5749::=
5678::=
4657:.
4077:0.
3958:.
3714:.
3424:a
3341::=
3110:,
3062:.
2995:.
2949:22
2923:22
2905:71
2752:.
2539::=
2446::=
2289:19
2265:19
2232:19
2208:19
2131:19
2011:19
1891:19
1771:19
1765:16
1651:19
1545:19
1508::=
1465:19
1430:19
1385::=
1023:if
955:if
907::=
684:.
220:).
177:).
8273::
8259:.
8238:.
8217:.
8190:.
8087:)
8081:,
8078:n
8074:/
8070:1
8067:(
8061:)
8058:0
8055:,
8046:(
8026:)
8020:,
8015:n
8011:b
8007:(
8001:)
7996:n
7992:a
7988:,
7979:(
7956:}
7953:0
7950:{
7947:=
7942:n
7938:I
7931:N
7924:n
7898:N
7891:n
7871:n
7867:/
7863:1
7857:x
7851:0
7831:0
7828:=
7825:x
7805:x
7785:0
7779:x
7758:}
7752:n
7749:1
7741:x
7735:0
7732::
7728:R
7721:x
7717:{
7713:=
7709:]
7703:n
7700:1
7695:,
7692:0
7688:[
7684:=
7679:n
7675:I
7647:,
7642:n
7638:I
7631:x
7609:n
7605:I
7584:0
7578:x
7554:x
7548:n
7544:/
7540:1
7520:x
7516:/
7512:1
7506:n
7485:N
7478:n
7458:0
7452:x
7430:n
7426:I
7419:N
7412:n
7363:}
7357:n
7354:1
7346:x
7340:0
7337::
7333:R
7326:x
7322:{
7318:=
7314:)
7308:n
7305:1
7300:,
7297:0
7293:(
7289:=
7284:n
7280:I
7256:}
7253:x
7250:{
7202:n
7198:I
7191:N
7184:n
7125:n
7121:I
7114:N
7107:n
7096:s
7075:N
7068:n
7046:n
7042:I
7035:s
7014:N
7007:n
6985:n
6981:b
6974:s
6966:n
6962:a
6941:)
6938:A
6935:(
6929:=
6926:s
6904:n
6900:b
6879:}
6873:,
6868:2
6864:a
6860:,
6855:1
6851:a
6847:{
6841:A
6821:]
6816:n
6812:b
6808:,
6803:n
6799:a
6795:[
6792:=
6787:n
6783:I
6759:N
6752:n
6748:)
6742:n
6738:I
6734:(
6707:R
6678:A
6658:s
6638:A
6618:s
6595:A
6573:n
6569:a
6540:n
6536:a
6509:s
6501:n
6497:a
6490:s
6468:n
6464:I
6457:s
6431:s
6408:N
6401:n
6397:)
6391:n
6387:I
6383:(
6363:A
6343:s
6314:A
6294:s
6273:N
6266:m
6246:s
6238:m
6234:b
6211:m
6207:I
6186:s
6166:s
6160:x
6154:s
6146:m
6142:b
6121:s
6115:x
6107:m
6103:a
6094:m
6090:b
6069:]
6064:m
6060:b
6056:,
6051:m
6047:a
6043:[
6040:=
6035:m
6031:I
6010:s
6004:x
5984:A
5978:x
5958:A
5938:s
5914:s
5873:n
5869:m
5855:]
5849:n
5845:b
5841:,
5836:n
5832:m
5827:[
5819:A
5807:n
5803:m
5789:]
5783:n
5779:m
5775:,
5770:n
5766:a
5761:[
5753:{
5744:1
5741:+
5738:n
5734:I
5708:2
5702:n
5698:b
5694:+
5689:n
5685:a
5673:n
5669:m
5647:N
5640:n
5620:]
5615:n
5611:b
5607:,
5602:n
5598:a
5594:[
5591:=
5586:n
5582:I
5561:A
5539:1
5535:b
5514:A
5508:c
5488:1
5482:c
5479:=
5474:1
5470:a
5447:1
5443:a
5430:.
5417:N
5410:n
5390:A
5368:n
5364:a
5341:N
5334:n
5314:A
5292:n
5288:b
5264:]
5259:n
5255:b
5251:,
5246:n
5242:a
5238:[
5235:=
5230:n
5226:I
5202:N
5195:n
5191:)
5185:n
5181:I
5177:(
5156:R
5149:A
5119:R
5112:A
5082:R
5075:B
5055:)
5052:B
5049:(
5024:s
4995:x
4991::
4988:A
4982:x
4975::
4972:s
4943:A
4923:s
4902:s
4896:x
4892::
4889:A
4883:x
4860:A
4840:s
4815:A
4793:)
4790:A
4787:(
4781:=
4778:s
4758:A
4752:x
4732:b
4726:x
4706:b
4685:R
4678:A
4643:k
4638:n
4634:m
4613:n
4591:n
4587:m
4566:y
4546:x
4526:k
4504:k
4500:y
4496:=
4493:x
4473:0
4467:y
4445:j
4441:/
4437:1
4433:x
4429:=
4423:j
4419:x
4414:=
4411:y
4391:0
4385:x
4382:,
4378:N
4371:j
4367:,
4362:j
4358:y
4354:=
4351:x
4276:n
4272:I
4265:N
4258:n
4204:x
4184:x
4163:N
4156:n
4135:|
4131:y
4125:x
4121:|
4113:|
4107:n
4103:I
4098:|
4070:|
4066:y
4060:x
4056:|
4035:y
4029:x
4007:n
4003:I
3996:N
3989:n
3978:y
3975:,
3972:x
3946:x
3923:N
3916:n
3912:)
3906:n
3902:I
3898:(
3881:.
3867:n
3863:I
3856:N
3849:n
3838:x
3834::
3830:R
3823:x
3795:n
3791:I
3767:N
3760:n
3756:)
3750:n
3746:I
3742:(
3695:|
3689:n
3685:I
3680:|
3659:N
3653:n
3613:N
3593:0
3574:.
3555:|
3549:N
3545:I
3540:|
3534::
3530:N
3523:N
3516:0
3483:n
3479:I
3470:1
3467:+
3464:n
3460:I
3454::
3450:N
3443:n
3409:N
3402:n
3398:)
3392:n
3388:I
3384:(
3362:n
3358:a
3349:n
3345:b
3337:|
3331:n
3327:I
3322:|
3301:]
3296:n
3292:b
3288:,
3283:n
3279:a
3275:[
3272:=
3267:n
3263:I
3239:N
3232:n
3228:)
3222:n
3218:I
3214:(
3201:]
3147:+
3126:R
3034:(
2957:7
2953:/
2926:7
2879:6
2859:n
2839:n
2836:2
2785:1
2771:π
2734:x
2706:n
2702:c
2679:x
2657:x
2649:2
2645:k
2624:]
2621:k
2618:,
2615:0
2612:[
2609:=
2604:1
2600:I
2578:]
2572:n
2568:c
2564:,
2557:n
2553:c
2549:x
2543:[
2534:1
2531:+
2528:n
2524:I
2511:.
2498:)
2490:n
2486:c
2482:x
2477:+
2472:n
2468:c
2463:(
2454:2
2451:1
2441:1
2438:+
2435:n
2431:c
2404:N
2397:n
2393:)
2387:n
2383:c
2379:(
2359:0
2353:x
2331:x
2237:=
2168:]
2162:,
2156:[
2153:=
2148:5
2144:I
2122:=
2117:2
2112:5
2108:m
2092:=
2086:2
2079:+
2069:=
2060:5
2056:m
2048:]
2042:,
2036:[
2033:=
2028:5
2024:I
2002:=
1997:2
1992:4
1988:m
1972:=
1966:2
1959:+
1956:4
1949:=
1940:4
1936:m
1928:]
1922:,
1919:4
1916:[
1913:=
1908:4
1904:I
1882:=
1877:2
1872:3
1868:m
1852:=
1846:2
1842:5
1839:+
1836:4
1829:=
1820:3
1816:m
1808:]
1805:5
1802:,
1799:4
1796:[
1793:=
1788:3
1784:I
1762:=
1757:2
1752:2
1748:m
1735:4
1732:=
1726:2
1722:5
1719:+
1716:3
1709:=
1700:2
1696:m
1688:]
1685:5
1682:,
1679:3
1676:[
1673:=
1668:2
1664:I
1645:9
1642:=
1637:2
1632:1
1628:m
1615:3
1612:=
1606:2
1602:5
1599:+
1596:1
1589:=
1580:1
1576:m
1523:]
1520:5
1517:,
1514:1
1511:[
1503:1
1499:I
1476:2
1472:5
1457:2
1453:1
1396:y
1392:/
1388:1
1382:x
1362:1
1356:y
1352:/
1348:1
1328:1
1322:y
1316:0
1294:y
1267:x
1244:|
1238:n
1234:I
1229:|
1206:1
1203:+
1200:n
1196:I
1187:x
1163:1
1160:+
1157:n
1153:I
1130:x
1106:x
1082:n
1078:I
1047:x
1039:2
1034:n
1030:m
1015:]
1009:n
1005:m
1001:,
996:n
992:a
987:[
979:x
971:2
966:n
962:m
947:]
941:n
937:b
933:,
928:n
924:m
919:[
911:{
902:1
899:+
896:n
892:I
866:1
862:I
839:n
835:I
812:2
806:n
802:b
798:+
793:n
789:a
782:=
777:n
773:m
747:N
740:n
737:,
734:]
729:n
725:b
721:,
716:n
712:a
708:[
705:=
700:n
696:I
672:]
669:k
666:,
663:1
660:[
657:=
652:1
648:I
627:x
619:2
615:k
591:x
571:]
568:x
565:,
562:1
559:[
556:=
551:1
547:I
526:x
518:x
510:1
490:1
484:x
435:N
428:n
406:n
402:I
366:(
329:n
325:b
316:1
313:+
310:n
306:b
283:n
279:a
270:1
267:+
264:n
260:a
237:n
233:I
208:N
163:n
159:I
136:1
133:+
130:n
126:I
98:,
95:3
92:,
89:2
86:,
83:1
80:=
77:n
48:n
44:I
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.