4255:
558:
3706:, and the outcome of a race that may contain ties (including all the horses, not just the first three finishers) may be described using a weak ordering. For this reason, the ordered Bell numbers count the possible number of outcomes of a horse race. In contrast, when items are ordered or ranked in a way that does not allow ties (such as occurs with the ordering of cards in a deck of cards, or batting orders among
8753:
4168:. For a restricted class of parking functions, in which each car parks either on its preferred spot or on the next spot, the number of parking functions is given by the ordered Bell numbers. Each restricted parking function corresponds to a weak ordering in which the cars that get their preferred spot are ordered by these spots, and each remaining car is tied with the car in its preferred spot. The
22:
1155:
3776:, cross-trained workers are allocated to groups of workers at different stages of a production line. The number of alternative assignments for a given number of workers, taking into account the choices of how many stages to use and how to assign workers to each stage, is an ordered Bell number. As another example, in the computer simulation of
4790:
are constructed by ranking certain constraints, and (in a phenomenon called factorial typology) the number of different grammars that can be formed in this way is limited to the number of permutations of the constraints. A paper reviewed by
Ellison and Klein suggested an extension of this linguistic
4102:
parking spots. Each car has a preferred parking spot, given by its value in the sequence. When a car arrives on the street, it parks in its preferred spot, or, if that is full, in the next available spot. A sequence of preferences forms a parking function if and only if each car can find a parking
561:
13 plane trees with ordered leaves and equal-length root-leaf paths, with the gaps between adjacent leaves labeled by the height above the leaves of the nearest common ancestor. These labels induce a weak ordering on the gaps, showing that the trees of this type are counted by the ordered Bell
1656:
2105:
Here, the left hand side is just the definition of the exponential generating function and the right hand side is the function obtained from this summation. The form of this function corresponds to the fact that the ordered Bell numbers are the numbers in the first column of the
3767:
with a numeric keypad, in which several keys may be pressed simultaneously and a combination consists of a sequence of keypresses that includes each key exactly once. As they show, the number of different combinations in such a system is given by the ordered Bell numbers. In
5207:
term from the sum (because only nonempty sequences are considered), and adding one separately from the sum (to make the result exceed, rather than equalling, the sum). These differences have offsetting effects, and the resulting weights are the ordered Bell numbers.
1858:
131:
on equivalent forms of multiple integrals. Because weak orderings have many names, ordered Bell numbers may also be called by those names, for instance as the numbers of preferential arrangements or the numbers of asymmetric generalized weak orders.
2363:
4791:
model in which ties between constraints are allowed, so that the ranking of constraints becomes a weak order rather than a total order. As they point out, the much larger magnitude of the ordered Bell numbers, relative to the corresponding
3843:
prime factors, an ordered multiplicative partition can be described by a weak ordering on its prime factors, describing which prime appears in which term of the partition. Thus, the number of ordered multiplicative partitions is given by
1044:
3974:
ordered multiplicative partitions. Numbers that are neither squarefree nor prime powers have a number of ordered multiplicative partitions that (as a function of the number of prime factors) is between these two extreme cases.
1469:
2103:
4524:
arguments to the relation and false for others. He defines the "complexity" of a relation to mean the number of other relations one can derive from the given one by permuting and repeating its arguments. For instance, for
191:
of this relation partition the elements of the ordering into subsets of mutually tied elements, and these equivalence classes can then be linearly ordered by the weak ordering. Thus, a weak ordering can be described as an
2830:
3216:
choices of the weak ordering on the rest of the elements. Multiplying these two factors, and then summing over the choices of how many elements to include in the first set, gives the number of weak orderings,
752:
Because weak orderings can be described as total orderings on the subsets of a partition, one can count weak orderings by counting total orderings and partitions, and combining the results appropriately. The
5141:
3069:
1698:
1743:
2483:
2532:
3585:
3514:
6059:
5958:
3444:
2232:
3374:
932:
792:
4954:, the problem of assigning weights to sequences of words with the property that the weight of any sequence exceeds the sum of weights of all its subsequences can be solved by using weight
4250:
1123:
2890:
2922:
1325:, the convex hull of points whose coordinates are permutations of (1,2,3,4), in the three-dimensional subspace of points whose coordinate sum is 10. This polyhedron has one volume (
1994:
and working with the function that results from summing this series can provide useful information about the sequence. The fast growth of the ordered Bell numbers causes their
6855:
3179:
4878:
4849:
964:
4489:
4166:
2152:
2623:
2005:
5411:
4431:
4392:
3972:
2959:
517:
5176:
4770:
4732:
4694:
4659:
4624:
3279:
3214:
2714:
1149:
4458:
4314:
4283:
2556:
2383:
1980:
1885:
5205:
5030:
4981:
4351:
3871:
3244:
2743:
2652:
400:
298:
4549:
3138:
2856:
2413:
1453:
1427:
1401:
1375:
1349:
1295:
1237:
644:
598:
547:
371:
3754:
955:
857:
total orderings of its subsets. Therefore, the ordered Bell numbers can be counted by summing over the possible numbers of subsets in a partition (the parameter
855:
6099:
6079:
5998:
5978:
5001:
4930:
4906:
4589:
4569:
4522:
4121:
4100:
4080:
4060:
4040:
4020:
4000:
3939:
3915:
3895:
3841:
3728:
3675:
3655:
3635:
3611:
3303:
3112:
3092:
2676:
2580:
2220:
2196:
2172:
1953:
1929:
1905:
1738:
1718:
1315:
1269:
1211:
1191:
895:
875:
832:
812:
692:
664:
618:
484:
440:
318:
269:
86:
6682:; Meyles et al credit the connection between parking functions and ordered Bell numbers to a 2021 bachelor's thesis by Kimberly P. Hadaway of Williams College
3694:
faces, Cayley trees, Cayley permutations, and equivalent formulae in Fubini's theorem. Weak orderings in turn have many other applications. For instance, in
2748:
4803:
If a fair coin (with equal probability of heads or tails) is flipped repeatedly until the first time the result is heads, the number of tails follows a
6012:
5384:
4320:. The faces of the complex intersect the sphere in 24 triangles, 36 arcs, and 14 vertices; one more face, at the center of the sphere, is not visible.
736:
412:
6848:
5038:
4394:. Here, a Coxeter group can be thought of as a finite system of reflection symmetries, closed under repeated reflections, whose mirrors partition a
704:. These numbers were called Fubini numbers by Louis Comtet, because they count the different ways to rearrange the ordering of sums or integrals in
694:
positive integers that include at least one copy of each positive integer between one and the maximum value in the sequence) "Cayley permutations".
6740:
Proceedings of the 18th
International Conference on Formal Power Series and Algebraic Combinatorics (FPSAC / SFCA), San Diego, California, 2006
6629:
5750:
418:
When the elements to be ordered are unlabeled (only the number of elements in each tied set matters, not their identities) what remains is a
1651:{\displaystyle a(n)=\sum _{k=0}^{n}\sum _{j=0}^{k}(-1)^{k-j}{\binom {k}{j}}j^{n}={\frac {1}{2}}\sum _{m=0}^{\infty }{\frac {m^{n}}{2^{m}}}.}
6764:
6166:
4491:
angles. The complex formed by these three lines has 13 faces: the origin, six rays from the origin, and six regions between pairs of rays.
2418:
600:
totally ordered leaves. In the trees considered by Cayley, each root-to-leaf path has the same length, and the number of nodes at distance
1462:, the formula for the ordered Bell numbers may be expanded out into a double summation. The ordered Bell numbers may also be given by an
7655:
6841:
754:
8782:
7650:
6499:
3281:(there is one weak ordering on zero items). Based on this recurrence, these numbers can be shown to obey certain periodic patterns in
1429:). The total number of these faces is 1 + 14 + 36 + 24 = 75, an ordered Bell number, corresponding to the summation formula above for
7665:
7645:
5250:. Because of this application, de Koninck calls these numbers "horse numbers", but this name does not appear to be in widespread use.
3593:. Peter Bala has conjectured that this sequence is eventually periodic (after a finite number of terms) modulo each positive integer
6507:
6377:
6352:
5626:
5319:
5244:
4172:, counted by the factorials, are parking functions for which each car parks on its preferred spot. This application also provides a
1982:
weak orderings, distinguished from each other by the subset of the consecutive increasing pairs that are tied in the weak ordering.
4183:
8358:
7938:
6692:
2978:
1666:
5874:
5711:
2222:
starts with the numbers in the same row of Pascal's triangle, and then continues with an infinite repeating sequence of zeros.
1999:
739:(OEIS). This became one of the first successful uses of the OEIS to discover equivalences between different counting problems.
6587:
Zhu, Yi; Filipov, Evgueni T. (October 2019), "An efficient numerical approach for simulating contact in origami assemblages",
7660:
6301:
5836:
5654:
8444:
674:
call the trees of this type "Cayley trees", and they call the sequences that may be used to label their gaps (sequences of
6202:
6161:
3703:
2488:
7760:
3803:. For instance, 30 has 13 multiplicative partitions, as a product of one divisor (30 itself), two divisors (for instance
3521:
8110:
7429:
7222:
4815:
3450:
1995:
724:, and the weak orderings that are counted by the ordered Bell numbers may be interpreted as a partition together with a
8145:
8115:
7790:
7780:
3114:
items that go into the first equivalence class of the ordering, together with a smaller weak ordering on the remaining
8286:
7700:
7434:
7414:
6021:
5920:
3918:
3380:
419:
7976:
3614:
2535:
8140:
3310:
900:
760:
8777:
8235:
7858:
7615:
7424:
7406:
7300:
7290:
7280:
8120:
3759:
Problems in many areas can be formulated using weak orderings, with solutions counted using ordered Bell numbers.
8363:
7908:
7529:
7315:
7310:
7305:
7295:
7272:
6697:
6257:
5178:. This recurrence differs from the one given earlier for the ordered Bell numbers, in two respects: omitting the
3897:, an ordered multiplicative partition is a product of powers of the same prime number, with exponents summing to
49:
6817:
Proceedings of the 4th IEEE International
Conference on Data Mining (ICDM 2004), 1–4 November 2004, Brighton, UK
1853:{\displaystyle a(n)=\sum _{k=0}^{n-1}2^{k}\left\langle {\begin{matrix}n\\k\end{matrix}}\right\rangle =A_{n}(2),}
7348:
6456:
3792:
1053:
148:
7605:
1169:
An alternative interpretation of the terms of this sum is that they count the features of each dimension in a
2861:
8474:
8439:
8225:
8135:
8009:
7984:
7893:
7883:
7495:
7477:
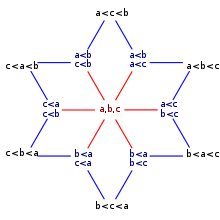
7397:
5688:
4940:
2895:
1911:. One way to explain this summation formula involves a mapping from weak orderings on the numbers from 1 to
104:
4912:. Truncating this series to a bounded number of terms and then applying the result for unbounded values of
2562:. That is, the ratio between the ordered Bell numbers and their approximation tends to one in the limit as
700:
traces the problem of counting weak orderings, which has the same sequence as its solution, to the work of
8734:
8004:
7878:
7509:
7285:
7065:
6992:
6531:
5605:
4804:
4177:
2559:
1046:
By general results on summations involving
Stirling numbers, it follows that the ordered Bell numbers are
667:
2358:{\displaystyle a(n)={\frac {n!}{2}}\sum _{k=-\infty }^{\infty }(\log 2+2\pi ik)^{-(n+1)},\qquad n\geq 1.}
7989:
7843:
7770:
6925:
6299:
Barthélémy, J.-P. (1980), "An asymptotic equivalent for the number of total preorders on a finite set",
5230:
176:
8698:
8338:
171:. This possibility describes various real-world scenarios, including certain sporting contests such as
3143:
2534:. Thus, the ordered Bell numbers are larger than the factorials by an exponential factor. Here, as in
466:} discussed above corresponds in this way to the composition 2 + 1 + 3. The number of compositions of
8631:
8525:
8489:
8230:
7953:
7933:
7750:
7419:
7207:
7179:
6118:
5536:
5455:
5268:
4881:
4854:
4825:
4808:
2655:
2199:
1459:
1322:
1159:
184:
136:
1935:, obtained by sorting each tied set into numerical order. Under this mapping, each permutation with
8353:
8217:
8212:
8180:
7943:
7918:
7913:
7888:
7818:
7814:
7745:
7467:
7263:
7232:
6425:
5803:
5033:
4936:
4885:
4173:
2970:
2389:. This leads to an approximation for the ordered Bell numbers, obtained by using only the term for
2226:
705:
571:
140:
128:
8752:
4254:
731:
The equivalence between counting Cayley trees and counting weak orderings was observed in 1970 by
8756:
8510:
8505:
8419:
8393:
8291:
8270:
8042:
7923:
7873:
7795:
7765:
7705:
7472:
7452:
7383:
7096:
6773:
6743:
6714:
6672:
6540:
6394:
6282:
6235:
6219:
6135:
5883:
5579:
5563:
5545:
5495:
5430:
5336:
5285:
4467:
4126:
3282:
2112:
721:
116:
7640:
5600:
6732:
2593:
8650:
8595:
8449:
8424:
8398:
8175:
7853:
7848:
7775:
7755:
7740:
7462:
7444:
7363:
7353:
7338:
7116:
7101:
6624:
6503:
6493:
6348:
5633:
5622:
5263:
5240:
4779:
4772:
obtained by repeating an argument. (Repeating the other argument produces the same relation.)
4697:
4498:
3982:, in mathematics, is a finite sequence of positive integers with the property that, for every
2583:
2386:
193:
188:
65:
4401:
4364:
3944:
2931:
489:
8686:
8479:
8065:
8037:
8027:
8019:
7903:
7868:
7863:
7830:
7524:
7487:
7378:
7373:
7368:
7358:
7330:
7217:
7169:
7164:
7121:
7060:
6820:
6783:
6706:
6664:
6638:
6604:
6596:
6570:
6465:
6386:
6340:
6310:
6266:
6211:
6175:
6127:
5893:
5845:
5759:
5663:
5649:
5614:
5555:
5534:(2010), "The hypercube of resistors, asymptotic expansions, and preferential arrangements",
5487:
5420:
5328:
5277:
5234:
5146:
4787:
4740:
4702:
4664:
4629:
4594:
3979:
3796:
3764:
3249:
3184:
2684:
1128:
717:
108:
6795:
6650:
6479:
6438:
6406:
6362:
6322:
6278:
6231:
6187:
6147:
5905:
5859:
5816:
5771:
5675:
5575:
5507:
5442:
5348:
5297:
4436:
4292:
4261:
2541:
2368:
2229:
of this generating function, the ordered Bell numbers can be expressed by the infinite sum
1990:
As with many other integer sequences, reinterpreting the sequence as the coefficients of a
1958:
1863:
834:
nonempty subsets. A weak ordering may be obtained from such a partition by choosing one of
557:
8662:
8551:
8484:
8410:
8333:
8307:
8125:
7838:
7695:
7630:
7600:
7590:
7585:
7251:
7159:
7106:
6950:
6890:
6791:
6646:
6475:
6434:
6402:
6358:
6318:
6274:
6227:
6183:
6143:
5901:
5855:
5812:
5767:
5671:
5571:
5503:
5438:
5344:
5293:
5181:
5006:
4957:
4909:
4461:
4395:
4354:
4327:
3847:
3220:
2719:
2628:
2175:
2107:
1908:
1661:
1463:
1318:
1240:
376:
274:
180:
144:
6525:
Ellison, T. Mark; Klein, Ewan (2001), "Review: The Best of All
Possible Words (review of
4528:
3117:
2835:
2392:
1432:
1406:
1380:
1354:
1328:
1274:
1216:
623:
577:
526:
350:
6223:
5567:
3736:
937:
837:
8667:
8535:
8520:
8384:
8348:
8323:
8199:
8170:
8155:
8032:
7928:
7898:
7625:
7580:
7457:
7055:
7050:
7045:
7017:
7002:
6915:
6900:
6878:
6865:
6609:
6084:
6064:
5983:
5963:
5531:
5473:
4986:
4951:
4944:
4915:
4891:
4735:
4574:
4554:
4507:
4106:
4085:
4065:
4045:
4025:
4005:
3985:
3924:
3900:
3880:
3826:
3781:
3713:
3660:
3640:
3620:
3596:
3288:
3097:
3077:
2661:
2587:
2565:
2205:
2181:
2157:
1938:
1914:
1890:
1723:
1703:
1300:
1254:
1196:
1176:
880:
860:
817:
797:
677:
649:
603:
469:
425:
303:
254:
71:
5719:(revised and enlarged ed.), D. Reidel Publishing Co., p. 228, archived from
5425:
8771:
8590:
8574:
8515:
8469:
8165:
8150:
8060:
7785:
7343:
7212:
7174:
7131:
7012:
6997:
6987:
6945:
6935:
6910:
6314:
6286:
6239:
5850:
5748:
Knopfmacher, A.; Mays, M. E. (2005), "A survey of factorization counting functions",
5667:
5596:
4358:
3788:
3773:
3691:
1170:
1163:
1039:{\displaystyle a(n)=\sum _{k=0}^{n}k!\left\{{\begin{matrix}n\\k\end{matrix}}\right\}}
156:
100:
61:
45:
6454:; Maïga, Hamadoun (2017), "Some new identities and congruences for Fubini numbers",
6116:
Velleman, Daniel J.; Call, Gregory S. (1995), "Permutations and combination locks",
5720:
5583:
8626:
8615:
8530:
8368:
8343:
8260:
8160:
8130:
8105:
8089:
7994:
7961:
7710:
7684:
7595:
7534:
7111:
7007:
6940:
6920:
6895:
6589:
Proceedings of the Royal
Society A: Mathematical, Physical and Engineering Sciences
6451:
6420:
5478:
4819:
4317:
3816:
3699:
3695:
1991:
732:
709:
124:
6810:"Spam filtering using a Markov random field model with variable weighting schemas"
5872:
Liu, Lily L.; Wang, Yi (2007), "On the log-convexity of combinatorial sequences",
3780:, the ordered Bell numbers give the number of orderings in which the creases of a
2098:{\displaystyle \sum _{n=0}^{\infty }a(n){\frac {x^{n}}{n!}}={\frac {1}{2-e^{x}}}.}
6809:
5618:
2969:
As well as the formulae above, the ordered Bell numbers may be calculated by the
8585:
8460:
8265:
7729:
7620:
7575:
7570:
7320:
7227:
7126:
6955:
6930:
6905:
6164:; Woan, Wen Jin; Woodson, Leon C. (1992), "How to guess a generating function",
6002:
5374:
4783:
4286:
4169:
3874:
3590:
2924:. This sequence of approximations, and this example from it, were calculated by
1932:
1248:
1244:
725:
713:
520:
197:
120:
112:
6667:; Jordaan, Richter; Kirby, Gordon Rojas; Sehayek, Sam; Spingarn, Ethan (2023),
4103:
spot on or after its preferred spot. The number of parking functions of length
2928:, using a general method for solving equations numerically (here, the equation
344:, or with both tied. The figure shows the 13 weak orderings on three elements.
8722:
8703:
7999:
7610:
6642:
6574:
6470:
6344:
6018:, which gives the row-by-row ordering of an infinite triangle of numbers with
5897:
5791:
5787:
5763:
4285:
cuts space into 24 triangular cones, shown here by their intersections with a
3812:
3690:
As has already been mentioned, the ordered Bell numbers count weak orderings,
1047:
172:
168:
152:
93:
89:
6833:
6215:
5559:
3756:, which is significantly smaller than the corresponding ordered Bell number.
1660:
Another summation formula expresses the ordered Bell numbers in terms of the
442:
as an ordered sum of positive integers. For instance, the ordered partition {
405:
1, 1, 3, 13, 75, 541, 4683, 47293, 545835, 7087261, 102247563, ... (sequence
8328:
8255:
8247:
8052:
7966:
7084:
6824:
5406:
4947:
WQSym whose dimensions in each grade are given by the ordered Bell numbers.
4792:
3731:
2925:
958:
666:
pairs of adjacent leaves, that may be weakly ordered by the height of their
620:
from the root must be strictly smaller than the number of nodes at distance
21:
6600:
6529:, Archangeli, Diana & Langendoen, D. Terence, eds., Blackwell, 1997)",
1154:
320:
elements. For instance, there are three weak orderings on the two elements
119:; the ordered Bell numbers count partitions that have been equipped with a
6270:
8429:
6255:
Bailey, Ralph W. (1998), "The number of weak orderings of a finite set",
3707:
123:. Their alternative name, the Fubini numbers, comes from a connection to
88:
elements. Weak orderings arrange their elements into a sequence allowing
3589:
Many more modular identities are known, including identities modulo any
8434:
8093:
6718:
6544:
6398:
6139:
5499:
5434:
5340:
5289:
3800:
3777:
1162:, with its vertices labeled by their four-dimensional coordinates as a
6808:
Chhabra, Shalendra; Yerazunis, William S.; Siefkes, Christian (2004),
6787:
6748:
5888:
3799:
is a representation of the number as a product of one or more of its
3784:
can be folded, allowing sets of creases to be folded simultaneously.
2825:{\displaystyle \lim _{n\to \infty }{\frac {n\,a(n-1)}{a(n)}}=\log 2.}
6710:
6390:
6179:
6131:
5491:
5462:, Graduate Texts in Mathematics, vol. 152, Springer, p. 18
5332:
5281:
4504:, mathematical statements that might be true of some choices of the
6677:
3819:; 30 is squarefree, but 20 is not, because its prime factorization
200:
on the sets of the partition. For instance, the ordered partition {
6778:
6375:
Kauffman, Dolores H. (1963), "Note on preferential arrangements",
5834:
Sprugnoli, Renzo (1994), "Riordan arrays and combinatorial sums",
5550:
4253:
1153:
20:
5713:
Advanced
Combinatorics: The Art of Finite and Infinite Expansions
3074:
The intuitive meaning of this formula is that a weak ordering on
25:
The 13 possible strict weak orderings on a set of three elements
6561:
system balancing: Definition, formulation, and exact solution",
6492:
Harris, John; Hirst, Jeffry L.; Mossinghoff, Michael J. (2008),
4795:, allows this theory to generate a much richer set of grammars.
1458:
By expanding each
Stirling number in this formula into a sum of
135:
These numbers may be computed via a summation formula involving
8720:
8684:
8648:
8612:
8572:
8197:
8086:
7812:
7727:
7682:
7559:
7249:
7196:
7148:
7082:
7034:
6972:
6876:
6837:
3094:
items may be broken down into a choice of some nonempty set of
167:
Weak orderings arrange their elements into a sequence allowing
5136:{\displaystyle W(n)=1+\sum _{k=1}^{n-1}{\binom {n}{k}}W(n-1),}
4818:
of the ordered Bell numbers fails to converge, it describes a
3702:
have eliminated most but not all ties, called in this context
5476:(1956), "Semiorders and a theory of utility discrimination",
99:
The ordered Bell numbers were studied in the 19th century by
519:. This is because a composition is determined by its set of
6006:
5378:
1321:. For instance, the three-dimensional permutohedron is the
407:
4002:
up to the sequence length, the sequence contains at least
3064:{\displaystyle a(n)=\sum _{i=1}^{n}{\binom {n}{i}}a(n-i).}
1693:{\displaystyle \langle {\scriptstyle {n \atop k}}\rangle }
224:} describes an ordered partition on six elements in which
6557:
Yu, Yang; Wang, Junwei; Ma, Ke; Sun, Wei (August 2018), "
232:
are tied and both less than the other four elements, and
5409:(1952), "On the factorization of squarefree integers",
4932:
approximates the resistance to arbitrarily high order.
646:, until reaching the leaves. In such a tree, there are
175:. A weak ordering can be formalized axiomatically by a
6762:
Féray, Valentin (2015), "Cyclic inclusion-exclusion",
6035:
5934:
5695:, Deighton: Bell and Co., Proposition XXII, p. 93
4859:
4830:
3148:
2499:
1804:
1674:
1015:
908:
768:
6669:
Unit-interval parking functions and the permutohedron
6200:
Lewis, Barry (2010), "Revisiting the Pascal matrix",
6087:
6067:
6024:
5986:
5966:
5923:
5184:
5149:
5041:
5009:
4989:
4960:
4918:
4894:
4857:
4828:
4743:
4705:
4667:
4632:
4597:
4577:
4557:
4531:
4510:
4470:
4439:
4404:
4398:
into the cells of the
Coxeter complex. For instance,
4367:
4330:
4295:
4264:
4186:
4129:
4109:
4088:
4068:
4048:
4028:
4008:
3988:
3947:
3927:
3903:
3883:
3850:
3829:
3739:
3716:
3663:
3643:
3623:
3599:
3524:
3453:
3383:
3313:
3291:
3252:
3223:
3187:
3146:
3120:
3100:
3080:
2981:
2934:
2898:
2864:
2838:
2751:
2722:
2687:
2664:
2631:
2596:
2568:
2544:
2491:
2478:{\displaystyle a(n)\sim {\frac {1}{2}}{n!}\,c^{n+1},}
2421:
2395:
2371:
2235:
2208:
2184:
2160:
2115:
2008:
1961:
1941:
1917:
1893:
1866:
1746:
1726:
1706:
1669:
1472:
1435:
1409:
1383:
1357:
1331:
1303:
1277:
1257:
1219:
1199:
1179:
1131:
1056:
967:
940:
903:
883:
863:
840:
820:
800:
763:
680:
652:
626:
606:
580:
529:
492:
472:
428:
379:
353:
306:
277:
257:
143:. They also count combinatorial objects that have a
74:
2527:{\displaystyle c={\tfrac {1}{\log 2}}\approx 1.4427}
1740:
pairs of consecutive items are in increasing order:
1271:. These vectors are defined in a space of dimension
523:, which may be any subset of the integers from 1 to
8544:
8498:
8458:
8409:
8383:
8316:
8300:
8279:
8246:
8211:
8051:
8018:
7975:
7952:
7829:
7517:
7508:
7486:
7443:
7405:
7396:
7329:
7271:
7262:
6423:(1988), "Periodicity of a combinatorial sequence",
5601:"On the analytical forms called trees, second part"
4811:of this distribution are the ordered Bell numbers.
3580:{\displaystyle a(n+500)\equiv a(n){\pmod {10000}}.}
6093:
6073:
6053:
5992:
5972:
5952:
5317:Gross, O. A. (1962), "Preferential arrangements",
5199:
5170:
5135:
5024:
4995:
4975:
4924:
4900:
4872:
4843:
4764:
4726:
4688:
4653:
4618:
4583:
4563:
4543:
4516:
4483:
4452:
4425:
4386:
4345:
4308:
4277:
4244:
4160:
4115:
4094:
4074:
4054:
4034:
4014:
3994:
3966:
3933:
3909:
3889:
3865:
3835:
3748:
3722:
3669:
3649:
3629:
3605:
3579:
3509:{\displaystyle a(n+100)\equiv a(n){\pmod {1000}},}
3508:
3438:
3368:
3297:
3273:
3238:
3208:
3173:
3132:
3106:
3086:
3063:
2953:
2916:
2884:
2850:
2824:
2737:
2708:
2670:
2646:
2617:
2574:
2550:
2526:
2477:
2407:
2377:
2357:
2214:
2190:
2166:
2146:
2097:
1974:
1947:
1923:
1899:
1879:
1852:
1732:
1712:
1692:
1650:
1447:
1421:
1395:
1369:
1343:
1309:
1289:
1263:
1231:
1205:
1185:
1143:
1117:
1038:
949:
926:
889:
869:
849:
826:
806:
786:
686:
658:
638:
612:
592:
541:
511:
478:
434:
422:or ordered integer partition, a representation of
394:
365:
312:
292:
263:
80:
5106:
5093:
4939:, an analogous construction to the (commutative)
4062:, describes the following process: a sequence of
3823:repeats the prime 2. For squarefree numbers with
3034:
3021:
1571:
1558:
300:, gives the number of distinct weak orderings on
6054:{\displaystyle k!\{{\scriptstyle {n \atop k}}\}}
5953:{\displaystyle k!\{{\scriptstyle {n \atop k}}\}}
5412:Proceedings of the American Mathematical Society
4661:derived relations. These are the given relation
3439:{\displaystyle a(n+20)\equiv a(n){\pmod {100}},}
2753:
2415:in this sum and discarding the remaining terms:
1297:, but they and their convex hull all lie in an
566:The ordered Bell numbers appear in the work of
6625:"A survey of factorization counting functions"
4464:across three lines that meet at the origin at
4180:on the ordered Bell numbers of a simple form,
3369:{\displaystyle a(n+4)\equiv a(n){\pmod {10}},}
2002:is used. For the ordered Bell numbers, it is:
927:{\displaystyle \{{\scriptstyle {n \atop k}}\}}
787:{\displaystyle \{{\scriptstyle {n \atop k}}\}}
6849:
6733:"Polynomial realizations of some trialgebras"
3164:
3151:
8:
6048:
6031:
5947:
5930:
5829:
5827:
5825:
5239:, American Mathematical Society, p. 4,
4775:
4734:obtained by swapping the arguments, and the
4245:{\displaystyle n!\leq a(n)\leq (n+1)^{n-1}.}
3760:
1687:
1670:
921:
904:
781:
764:
6819:, IEEE Computer Society, pp. 347–350,
6731:Novelli, J.-C.; Thibon, J.-Y. (June 2006),
5258:
5256:
1247:of points whose coordinate vectors are the
1213:th term counting the features of dimension
671:
155:number or the faces of all dimensions of a
147:to the weak orderings, such as the ordered
8717:
8681:
8645:
8609:
8569:
8243:
8208:
8194:
8083:
7826:
7809:
7724:
7679:
7556:
7514:
7402:
7268:
7259:
7246:
7193:
7150:Possessing a specific set of other numbers
7145:
7079:
7031:
6969:
6873:
6856:
6842:
6834:
670:; this weak ordering determines the tree.
92:, such as might arise as the outcome of a
6777:
6747:
6676:
6608:
6469:
6339:, Springer-Verlag, New York, p. 42,
6250:
6248:
6086:
6066:
6036:
6034:
6023:
6013:On-Line Encyclopedia of Integer Sequences
5985:
5965:
5935:
5933:
5922:
5887:
5849:
5698:
5549:
5424:
5385:On-Line Encyclopedia of Integer Sequences
5183:
5148:
5105:
5092:
5090:
5078:
5067:
5040:
5008:
4988:
4959:
4917:
4893:
4858:
4856:
4829:
4827:
4742:
4704:
4666:
4631:
4596:
4576:
4556:
4530:
4509:
4497:uses the ordered Bell numbers to analyze
4475:
4469:
4444:
4438:
4403:
4372:
4366:
4329:
4300:
4294:
4269:
4263:
4227:
4185:
4146:
4128:
4108:
4087:
4067:
4047:
4027:
4007:
3987:
3952:
3946:
3926:
3917:, and this ordered sum of exponents is a
3902:
3882:
3849:
3828:
3738:
3715:
3662:
3642:
3622:
3598:
3558:
3523:
3487:
3452:
3417:
3382:
3347:
3312:
3290:
3251:
3222:
3186:
3163:
3150:
3147:
3145:
3119:
3099:
3079:
3033:
3020:
3018:
3012:
3001:
2980:
2939:
2933:
2897:
2868:
2863:
2837:
2774:
2768:
2756:
2750:
2721:
2686:
2663:
2630:
2595:
2582:grows arbitrarily large. As expressed in
2567:
2543:
2498:
2490:
2460:
2455:
2447:
2437:
2420:
2394:
2370:
2321:
2284:
2270:
2251:
2234:
2207:
2183:
2159:
2135:
2114:
2083:
2067:
2048:
2042:
2024:
2013:
2007:
1966:
1960:
1940:
1916:
1892:
1871:
1865:
1832:
1803:
1793:
1777:
1766:
1745:
1725:
1705:
1675:
1673:
1668:
1637:
1627:
1621:
1615:
1604:
1590:
1581:
1570:
1557:
1555:
1543:
1524:
1513:
1503:
1492:
1471:
1434:
1408:
1382:
1356:
1330:
1302:
1276:
1256:
1218:
1198:
1178:
1130:
1118:{\displaystyle a(n-1)a(n+1)\geq a(n)^{2}}
1109:
1055:
1014:
998:
987:
966:
939:
909:
907:
902:
882:
862:
839:
819:
799:
769:
767:
762:
737:On-Line Encyclopedia of Integer Sequences
701:
697:
679:
651:
625:
605:
579:
528:
497:
491:
471:
427:
378:
352:
305:
276:
256:
73:
1955:consecutive increasing pairs comes from
1050:, meaning that they obey the inequality
556:
6502:(2nd ed.), Springer, p. 132,
6111:
6109:
6107:
5782:
5780:
5743:
5741:
5739:
5401:
5399:
5397:
5395:
5312:
5310:
5308:
5306:
5225:
5223:
5221:
5217:
2885:{\displaystyle 375/541\approx 0.693161}
897:, multiplying the number of partitions
6695:(1956), "Two measures of complexity",
6630:International Journal of Number Theory
6563:Computers & Industrial Engineering
5751:International Journal of Number Theory
5369:
5367:
5365:
5363:
5361:
5359:
5357:
4494:
3710:players), the number of orderings for
2917:{\displaystyle \log 2\approx 0.693145}
567:
248:, which are all tied with each other.
6623:Knopfmacher, A.; Mays, M. E. (2005),
6520:
6518:
5526:
5524:
5522:
5520:
5518:
5516:
4042:. A sequence of this type, of length
3772:, a Japanese technique for balancing
3246:. As a base case for the recurrence,
1986:Generating function and approximation
271:th ordered Bell number, denoted here
7:
6765:SIAM Journal on Discrete Mathematics
6167:SIAM Journal on Discrete Mathematics
196:, a partition of its elements and a
5796:candidates when ties are permitted"
4460:, the system of reflections of the
3566:
3495:
3425:
3355:
755:Stirling numbers of the second kind
6500:Undergraduate Texts in Mathematics
6037:
5936:
5097:
4353:counts the number of faces in the
3155:
3025:
2965:Recurrence and modular periodicity
2763:
2285:
2280:
2025:
1700:, which count the permutations of
1676:
1616:
1562:
910:
770:
14:
6378:The American Mathematical Monthly
5426:10.1090/S0002-9939-1952-0050620-1
5320:The American Mathematical Monthly
3815:when it is a product of distinct
2681:Comparing the approximations for
934:by the number of total orderings
570:, who used them to count certain
8751:
8359:Perfect digit-to-digit invariant
6000:-dimensional associahedron, see
5635:Collected Works of Arthur Cayley
3941:. Thus, in this case, there are
3174:{\displaystyle {\tbinom {n}{i}}}
2000:exponential generating function
5875:Advances in Applied Mathematics
5652:(1984), "Cayley permutations",
4873:{\displaystyle {\tfrac {2}{n}}}
4844:{\displaystyle {\tfrac {1}{n}}}
4786:. In this theory, grammars for
4626:. By Kemeny's analysis, it has
3559:
3488:
3418:
3348:
2345:
708:, which in turn is named after
6527:Optimality Theory: An Overview
6495:Combinatorics and Graph Theory
5194:
5188:
5159:
5153:
5127:
5115:
5051:
5045:
5019:
5013:
4970:
4964:
4759:
4753:
4721:
4715:
4683:
4677:
4642:
4636:
4613:
4607:
4551:, a relation on two arguments
4414:
4408:
4340:
4334:
4224:
4211:
4205:
4199:
4143:
4130:
4082:cars arrives on a street with
3860:
3854:
3570:
3560:
3555:
3549:
3540:
3528:
3499:
3489:
3484:
3478:
3469:
3457:
3429:
3419:
3414:
3408:
3399:
3387:
3359:
3349:
3344:
3338:
3329:
3317:
3262:
3256:
3233:
3227:
3203:
3191:
3181:choices of the first set, and
3055:
3043:
2991:
2985:
2804:
2798:
2790:
2778:
2760:
2732:
2726:
2703:
2691:
2641:
2635:
2612:
2606:
2431:
2425:
2337:
2325:
2318:
2290:
2245:
2239:
2198:is an infinite matrix form of
2132:
2116:
2039:
2033:
1844:
1838:
1756:
1750:
1540:
1530:
1482:
1476:
1106:
1099:
1090:
1078:
1072:
1060:
977:
971:
728:on the sets in the partition.
389:
383:
287:
281:
1:
7198:Expressible via specific sums
6337:Ramanujan's notebooks. Part I
6203:American Mathematical Monthly
3657:that are relatively prime to
3637:, the number of residues mod
3613:, with a period that divides
1351:), 14 two-dimensional faces (
794:, count the partitions of an
735:, using an early form of the
6315:10.1016/0012-365X(80)90159-4
5851:10.1016/0012-365X(92)00570-H
5792:"The number of orderings of
5668:10.1016/0012-365X(84)90136-5
5619:10.1017/CBO9780511703706.026
4816:ordinary generating function
1996:ordinary generating function
8287:Multiplicative digital root
5266:(1982), "Races with ties",
4888:of opposite vertices of an
4484:{\displaystyle 60^{\circ }}
4289:. The reflection planes of
4161:{\displaystyle (n+1)^{n-1}}
3873:. On the other hand, for a
2147:{\displaystyle (2I-P)^{-1}}
373:, the ordered Bell numbers
328:: they can be ordered with
8799:
6003:Sloane, N. J. A.
5917:For the interpretation of
5375:Sloane, N. J. A.
4776:Ellison & Klein (2001)
3761:Velleman & Call (1995)
8783:Enumerative combinatorics
8747:
8730:
8716:
8694:
8680:
8658:
8644:
8622:
8608:
8581:
8568:
8364:Perfect digital invariant
8207:
8193:
8101:
8082:
7939:Superior highly composite
7825:
7808:
7736:
7723:
7691:
7678:
7566:
7555:
7258:
7245:
7203:
7192:
7155:
7144:
7092:
7078:
7041:
7030:
6983:
6968:
6886:
6872:
6698:The Journal of Philosophy
6643:10.1142/S1793042105000315
6575:10.1016/j.cie.2018.05.048
6471:10.1016/j.jnt.2016.09.032
6345:10.1007/978-1-4612-1088-7
6335:Berndt, Bruce C. (1985),
6258:Social Choice and Welfare
5980:-dimensional faces of an
5898:10.1016/j.aam.2006.11.002
5764:10.1142/S1793042105000315
5236:Those Fascinating Numbers
3686:Combinatorial enumeration
3285:: for sufficiently large
2618:{\displaystyle 1\pm o(1)}
1251:of the numbers from 1 to
877:) and, for each value of
672:Mor & Fraenkel (1984)
149:multiplicative partitions
50:enumerative combinatorics
7977:Euler's totient function
7761:Euler–Jacobi pseudoprime
7036:Other polynomial numbers
6457:Journal of Number Theory
6224:10.4169/000298910x474989
6216:10.4169/000298910X474989
5568:10.4169/002557010X529752
5560:10.4169/002557010X529752
4941:quasisymmetric functions
4324:The ordered Bell number
4258:The Coxeter complex for
4022:values that are at most
3793:multiplicative partition
3615:Euler's totient function
2858:gives the approximation
2536:Stirling's approximation
1998:to diverge; instead the
163:Definitions and examples
145:bijective correspondence
16:Number of weak orderings
7791:Somer–Lucas pseudoprime
7781:Lucas–Carmichael number
7616:Lazy caterer's sequence
6825:10.1109/ICDM.2004.10031
6101:th row of the triangle.
6007:"Sequence A019538"
5379:"Sequence A000670"
4851:and then multiplied by
4778:apply these numbers to
4426:{\displaystyle a(3)=13}
4387:{\displaystyle A_{n-1}}
3967:{\displaystyle 2^{n-1}}
3811:, etc.). An integer is
2954:{\displaystyle e^{x}=2}
1239:. A permutohedron is a
512:{\displaystyle 2^{n-1}}
107:. They are named after
105:William Allen Whitworth
7666:Wedderburn–Etherington
7066:Lucky numbers of Euler
6663:Meyles, Lucas Chaves;
6601:10.1098/rspa.2019.0366
6532:Journal of Linguistics
6095:
6075:
6055:
5994:
5974:
5954:
5710:Comtet, Louis (1974),
5606:Philosophical Magazine
5201:
5172:
5171:{\displaystyle W(1)=0}
5137:
5089:
5026:
4997:
4977:
4926:
4902:
4874:
4845:
4805:geometric distribution
4766:
4765:{\displaystyle x=f(x)}
4728:
4727:{\displaystyle x=f(y)}
4690:
4689:{\displaystyle y=f(x)}
4655:
4654:{\displaystyle a(n)=3}
4620:
4619:{\displaystyle y=f(x)}
4585:
4565:
4545:
4518:
4485:
4454:
4427:
4388:
4347:
4321:
4310:
4279:
4246:
4178:upper and lower bounds
4162:
4117:
4096:
4076:
4056:
4036:
4016:
3996:
3968:
3935:
3911:
3891:
3867:
3837:
3807:), or three divisors (
3750:
3724:
3671:
3651:
3631:
3607:
3581:
3510:
3440:
3370:
3299:
3275:
3274:{\displaystyle a(0)=1}
3240:
3210:
3209:{\displaystyle a(n-i)}
3175:
3134:
3108:
3088:
3065:
3017:
2955:
2918:
2886:
2852:
2826:
2739:
2710:
2709:{\displaystyle a(n-1)}
2672:
2648:
2619:
2576:
2560:asymptotic equivalence
2552:
2538:to the factorial, the
2528:
2479:
2409:
2379:
2359:
2289:
2216:
2192:
2168:
2148:
2099:
2029:
1976:
1949:
1925:
1901:
1881:
1854:
1788:
1734:
1714:
1694:
1652:
1620:
1529:
1508:
1449:
1423:
1397:
1371:
1345:
1311:
1291:
1265:
1233:
1207:
1187:
1166:
1145:
1144:{\displaystyle n>0}
1119:
1040:
1003:
951:
928:
891:
871:
851:
828:
808:
788:
688:
668:lowest common ancestor
660:
640:
614:
594:
563:
543:
513:
480:
436:
396:
367:
314:
294:
265:
111:, who wrote about the
82:
41:
7954:Prime omega functions
7771:Frobenius pseudoprime
7561:Combinatorial numbers
7430:Centered dodecahedral
7223:Primary pseudoperfect
6271:10.1007/s003550050123
6096:
6076:
6056:
5995:
5975:
5955:
5460:Lectures on Polytopes
5202:
5173:
5138:
5063:
5032:is obtained from the
5027:
4998:
4978:
4927:
4903:
4875:
4846:
4767:
4729:
4691:
4656:
4621:
4586:
4566:
4546:
4519:
4486:
4455:
4453:{\displaystyle A_{2}}
4428:
4389:
4348:
4311:
4309:{\displaystyle A_{3}}
4280:
4278:{\displaystyle A_{3}}
4257:
4247:
4163:
4118:
4097:
4077:
4057:
4037:
4017:
3997:
3969:
3936:
3912:
3892:
3868:
3838:
3751:
3725:
3672:
3652:
3632:
3608:
3582:
3511:
3441:
3371:
3300:
3276:
3241:
3211:
3176:
3135:
3109:
3089:
3066:
2997:
2956:
2919:
2887:
2853:
2827:
2740:
2711:
2673:
2649:
2620:
2577:
2553:
2551:{\displaystyle \sim }
2529:
2480:
2410:
2380:
2378:{\displaystyle \log }
2360:
2266:
2217:
2193:
2169:
2149:
2100:
2009:
1977:
1975:{\displaystyle 2^{k}}
1950:
1926:
1902:
1882:
1880:{\displaystyle A_{n}}
1855:
1762:
1735:
1715:
1695:
1653:
1600:
1509:
1488:
1460:binomial coefficients
1450:
1424:
1398:
1372:
1346:
1312:
1292:
1266:
1234:
1208:
1188:
1157:
1146:
1120:
1041:
983:
952:
929:
892:
872:
852:
829:
809:
789:
689:
661:
641:
615:
595:
560:
544:
514:
481:
437:
397:
368:
315:
295:
266:
177:partially ordered set
137:binomial coefficients
83:
24:
8413:-composition related
8213:Arithmetic functions
7815:Arithmetic functions
7751:Elliptic pseudoprime
7435:Centered icosahedral
7415:Centered tetrahedral
6742:, pp. 243–254,
6302:Discrete Mathematics
6119:Mathematics Magazine
6085:
6065:
6022:
5984:
5964:
5921:
5837:Discrete Mathematics
5655:Discrete Mathematics
5537:Mathematics Magazine
5269:Mathematics Magazine
5200:{\displaystyle W(0)}
5182:
5147:
5039:
5025:{\displaystyle W(n)}
5007:
4987:
4976:{\displaystyle W(n)}
4958:
4937:noncommutative rings
4916:
4892:
4882:asymptotic expansion
4855:
4826:
4741:
4703:
4665:
4630:
4595:
4591:might take the form
4575:
4555:
4529:
4508:
4468:
4437:
4402:
4365:
4346:{\displaystyle a(n)}
4328:
4293:
4262:
4184:
4127:
4107:
4086:
4066:
4046:
4026:
4006:
3986:
3945:
3925:
3901:
3881:
3866:{\displaystyle a(n)}
3848:
3827:
3737:
3714:
3661:
3641:
3621:
3597:
3522:
3451:
3381:
3311:
3289:
3250:
3239:{\displaystyle a(n)}
3221:
3185:
3144:
3118:
3098:
3078:
2979:
2932:
2896:
2862:
2836:
2832:For example, taking
2749:
2738:{\displaystyle a(n)}
2720:
2685:
2662:
2656:decays exponentially
2647:{\displaystyle o(1)}
2629:
2594:
2566:
2542:
2489:
2419:
2393:
2369:
2233:
2206:
2182:
2158:
2113:
2006:
1959:
1939:
1915:
1891:
1864:
1744:
1724:
1704:
1667:
1470:
1433:
1407:
1403:), and 24 vertices (
1381:
1355:
1329:
1323:truncated octahedron
1301:
1275:
1255:
1217:
1197:
1177:
1160:truncated octahedron
1158:A three-dimensional
1129:
1054:
965:
938:
901:
881:
861:
838:
818:
798:
761:
678:
650:
624:
604:
578:
527:
490:
470:
426:
395:{\displaystyle a(n)}
377:
351:
304:
293:{\displaystyle a(n)}
275:
255:
185:equivalence relation
72:
54:ordered Bell numbers
8339:Kaprekar's constant
7859:Colossally abundant
7746:Catalan pseudoprime
7646:Schröder–Hipparchus
7425:Centered octahedral
7301:Centered heptagonal
7291:Centered pentagonal
7281:Centered triangular
6881:and related numbers
6426:Fibonacci Quarterly
5804:Fibonacci Quarterly
5532:Pippenger, Nicholas
5034:recurrence equation
4886:resistance distance
4822:that (evaluated at
4544:{\displaystyle n=2}
4174:combinatorial proof
3821:2 · 2 · 5
3809:3 · 5 · 2
3133:{\displaystyle n-i}
2971:recurrence relation
2851:{\displaystyle n=5}
2408:{\displaystyle k=0}
2227:contour integration
1909:Eulerian polynomial
1448:{\displaystyle n=3}
1422:{\displaystyle k=3}
1396:{\displaystyle k=2}
1370:{\displaystyle k=1}
1344:{\displaystyle k=0}
1290:{\displaystyle n+1}
1232:{\displaystyle n-k}
722:partitions of a set
639:{\displaystyle i+1}
593:{\displaystyle n+1}
542:{\displaystyle n-1}
366:{\displaystyle n=0}
189:equivalence classes
141:recurrence relation
117:partitions of a set
8757:Mathematics portal
8699:Aronson's sequence
8445:Smarandache–Wellin
8202:-dependent numbers
7909:Primitive abundant
7796:Strong pseudoprime
7786:Perrin pseudoprime
7766:Fermat pseudoprime
7706:Wolstenholme prime
7530:Squared triangular
7316:Centered decagonal
7311:Centered nonagonal
7306:Centered octagonal
7296:Centered hexagonal
6595:(2230): 20190366,
6091:
6071:
6051:
6046:
5990:
5970:
5950:
5945:
5456:Ziegler, Günter M.
5264:Mendelson, Elliott
5197:
5168:
5133:
5022:
4993:
4983:for a sequence of
4973:
4935:In the algebra of
4922:
4898:
4870:
4868:
4841:
4839:
4762:
4724:
4686:
4651:
4616:
4581:
4561:
4541:
4514:
4481:
4450:
4423:
4384:
4357:associated with a
4343:
4322:
4316:cut the sphere in
4306:
4275:
4242:
4158:
4113:
4092:
4072:
4052:
4032:
4012:
3992:
3964:
3931:
3907:
3887:
3863:
3833:
3749:{\displaystyle n!}
3746:
3720:
3667:
3647:
3627:
3603:
3577:
3506:
3436:
3366:
3295:
3283:modular arithmetic
3271:
3236:
3206:
3171:
3169:
3130:
3104:
3084:
3061:
2951:
2914:
2882:
2848:
2822:
2767:
2735:
2706:
2668:
2644:
2615:
2572:
2548:
2524:
2516:
2475:
2405:
2375:
2355:
2212:
2188:
2164:
2144:
2095:
1972:
1945:
1921:
1897:
1877:
1850:
1819:
1730:
1710:
1690:
1685:
1648:
1445:
1419:
1393:
1367:
1341:
1307:
1287:
1261:
1229:
1203:
1183:
1167:
1141:
1115:
1036:
1030:
950:{\displaystyle k!}
947:
924:
919:
887:
867:
850:{\displaystyle k!}
847:
824:
814:-element set into
804:
784:
779:
684:
656:
636:
610:
590:
564:
539:
509:
476:
432:
392:
363:
310:
290:
261:
115:, which count the
78:
42:
8778:Integer sequences
8765:
8764:
8743:
8742:
8712:
8711:
8676:
8675:
8640:
8639:
8604:
8603:
8564:
8563:
8560:
8559:
8379:
8378:
8189:
8188:
8078:
8077:
8074:
8073:
8020:Aliquot sequences
7831:Divisor functions
7804:
7803:
7776:Lucas pseudoprime
7756:Euler pseudoprime
7741:Carmichael number
7719:
7718:
7674:
7673:
7551:
7550:
7547:
7546:
7543:
7542:
7504:
7503:
7392:
7391:
7349:Square triangular
7241:
7240:
7188:
7187:
7140:
7139:
7074:
7073:
7026:
7025:
6964:
6963:
6788:10.1137/140991364
6665:Harris, Pamela E.
6162:Shapiro, Louis W.
6094:{\displaystyle n}
6081:th number on the
6074:{\displaystyle k}
6044:
6016:, OEIS Foundation
5993:{\displaystyle n}
5973:{\displaystyle k}
5960:as the number of
5943:
5693:Choice and Chance
5388:, OEIS Foundation
5231:de Koninck, J. M.
5104:
4996:{\displaystyle n}
4925:{\displaystyle n}
4901:{\displaystyle n}
4867:
4838:
4788:natural languages
4780:optimality theory
4698:converse relation
4584:{\displaystyle y}
4564:{\displaystyle x}
4517:{\displaystyle n}
4116:{\displaystyle n}
4095:{\displaystyle n}
4075:{\displaystyle n}
4055:{\displaystyle n}
4035:{\displaystyle i}
4015:{\displaystyle i}
3995:{\displaystyle i}
3934:{\displaystyle n}
3910:{\displaystyle n}
3890:{\displaystyle n}
3836:{\displaystyle n}
3765:combination locks
3723:{\displaystyle n}
3670:{\displaystyle k}
3650:{\displaystyle k}
3630:{\displaystyle k}
3606:{\displaystyle k}
3298:{\displaystyle n}
3162:
3140:items. There are
3107:{\displaystyle i}
3087:{\displaystyle n}
3032:
2808:
2752:
2671:{\displaystyle n}
2584:little o notation
2575:{\displaystyle n}
2515:
2445:
2387:natural logarithm
2264:
2215:{\displaystyle P}
2200:Pascal's triangle
2191:{\displaystyle P}
2167:{\displaystyle I}
2090:
2062:
1948:{\displaystyle k}
1924:{\displaystyle n}
1900:{\displaystyle n}
1733:{\displaystyle k}
1713:{\displaystyle n}
1683:
1643:
1598:
1569:
1310:{\displaystyle n}
1264:{\displaystyle n}
1206:{\displaystyle k}
1186:{\displaystyle n}
917:
890:{\displaystyle k}
870:{\displaystyle k}
827:{\displaystyle k}
807:{\displaystyle n}
777:
687:{\displaystyle n}
659:{\displaystyle n}
613:{\displaystyle i}
479:{\displaystyle n}
435:{\displaystyle n}
313:{\displaystyle n}
264:{\displaystyle n}
194:ordered partition
81:{\displaystyle n}
8790:
8755:
8718:
8687:Natural language
8682:
8646:
8614:Generated via a
8610:
8570:
8475:Digit-reassembly
8440:Self-descriptive
8244:
8209:
8195:
8146:Lucas–Carmichael
8136:Harmonic divisor
8084:
8010:Sparsely totient
7985:Highly cototient
7894:Multiply perfect
7884:Highly composite
7827:
7810:
7725:
7680:
7661:Telephone number
7557:
7515:
7496:Square pyramidal
7478:Stella octangula
7403:
7269:
7260:
7252:Figurate numbers
7247:
7194:
7146:
7080:
7032:
6970:
6874:
6858:
6851:
6844:
6835:
6828:
6827:
6814:
6805:
6799:
6798:
6781:
6772:(4): 2284–2311,
6759:
6753:
6752:
6751:
6737:
6728:
6722:
6721:
6689:
6683:
6681:
6680:
6660:
6654:
6653:
6620:
6614:
6613:
6612:
6584:
6578:
6577:
6554:
6548:
6547:
6522:
6513:
6512:
6489:
6483:
6482:
6473:
6448:
6442:
6441:
6417:
6411:
6409:
6372:
6366:
6365:
6332:
6326:
6325:
6296:
6290:
6289:
6252:
6243:
6242:
6197:
6191:
6190:
6157:
6151:
6150:
6113:
6102:
6100:
6098:
6097:
6092:
6080:
6078:
6077:
6072:
6060:
6058:
6057:
6052:
6047:
6045:
6017:
5999:
5997:
5996:
5991:
5979:
5977:
5976:
5971:
5959:
5957:
5956:
5951:
5946:
5944:
5915:
5909:
5908:
5891:
5869:
5863:
5862:
5853:
5844:(1–3): 267–290,
5831:
5820:
5819:
5800:
5795:
5784:
5775:
5774:
5745:
5734:
5733:
5732:
5731:
5725:
5718:
5707:
5701:
5699:Pippenger (2010)
5696:
5689:Whitworth, W. A.
5685:
5679:
5678:
5645:
5639:
5631:
5613:(121): 374–378,
5593:
5587:
5586:
5553:
5528:
5511:
5510:
5470:
5464:
5463:
5452:
5446:
5445:
5428:
5403:
5390:
5389:
5371:
5352:
5351:
5314:
5301:
5300:
5260:
5251:
5249:
5227:
5206:
5204:
5203:
5198:
5177:
5175:
5174:
5169:
5142:
5140:
5139:
5134:
5111:
5110:
5109:
5096:
5088:
5077:
5031:
5029:
5028:
5023:
5002:
5000:
4999:
4994:
4982:
4980:
4979:
4974:
4931:
4929:
4928:
4923:
4907:
4905:
4904:
4899:
4879:
4877:
4876:
4871:
4869:
4860:
4850:
4848:
4847:
4842:
4840:
4831:
4771:
4769:
4768:
4763:
4733:
4731:
4730:
4725:
4695:
4693:
4692:
4687:
4660:
4658:
4657:
4652:
4625:
4623:
4622:
4617:
4590:
4588:
4587:
4582:
4570:
4568:
4567:
4562:
4550:
4548:
4547:
4542:
4523:
4521:
4520:
4515:
4501:
4490:
4488:
4487:
4482:
4480:
4479:
4459:
4457:
4456:
4451:
4449:
4448:
4432:
4430:
4429:
4424:
4393:
4391:
4390:
4385:
4383:
4382:
4352:
4350:
4349:
4344:
4315:
4313:
4312:
4307:
4305:
4304:
4284:
4282:
4281:
4276:
4274:
4273:
4251:
4249:
4248:
4243:
4238:
4237:
4167:
4165:
4164:
4159:
4157:
4156:
4122:
4120:
4119:
4114:
4101:
4099:
4098:
4093:
4081:
4079:
4078:
4073:
4061:
4059:
4058:
4053:
4041:
4039:
4038:
4033:
4021:
4019:
4018:
4013:
4001:
3999:
3998:
3993:
3980:parking function
3973:
3971:
3970:
3965:
3963:
3962:
3940:
3938:
3937:
3932:
3916:
3914:
3913:
3908:
3896:
3894:
3893:
3888:
3872:
3870:
3869:
3864:
3842:
3840:
3839:
3834:
3822:
3810:
3806:
3797:positive integer
3755:
3753:
3752:
3747:
3732:factorial number
3729:
3727:
3726:
3721:
3676:
3674:
3673:
3668:
3656:
3654:
3653:
3648:
3636:
3634:
3633:
3628:
3612:
3610:
3609:
3604:
3586:
3584:
3583:
3578:
3573:
3515:
3513:
3512:
3507:
3502:
3445:
3443:
3442:
3437:
3432:
3375:
3373:
3372:
3367:
3362:
3304:
3302:
3301:
3296:
3280:
3278:
3277:
3272:
3245:
3243:
3242:
3237:
3215:
3213:
3212:
3207:
3180:
3178:
3177:
3172:
3170:
3168:
3167:
3154:
3139:
3137:
3136:
3131:
3113:
3111:
3110:
3105:
3093:
3091:
3090:
3085:
3070:
3068:
3067:
3062:
3039:
3038:
3037:
3024:
3016:
3011:
2960:
2958:
2957:
2952:
2944:
2943:
2923:
2921:
2920:
2915:
2891:
2889:
2888:
2883:
2872:
2857:
2855:
2854:
2849:
2831:
2829:
2828:
2823:
2809:
2807:
2793:
2769:
2766:
2744:
2742:
2741:
2736:
2715:
2713:
2712:
2707:
2677:
2675:
2674:
2669:
2653:
2651:
2650:
2645:
2624:
2622:
2621:
2616:
2581:
2579:
2578:
2573:
2557:
2555:
2554:
2549:
2533:
2531:
2530:
2525:
2517:
2514:
2500:
2484:
2482:
2481:
2476:
2471:
2470:
2454:
2446:
2438:
2414:
2412:
2411:
2406:
2384:
2382:
2381:
2376:
2364:
2362:
2361:
2356:
2341:
2340:
2288:
2283:
2265:
2260:
2252:
2221:
2219:
2218:
2213:
2197:
2195:
2194:
2189:
2173:
2171:
2170:
2165:
2153:
2151:
2150:
2145:
2143:
2142:
2104:
2102:
2101:
2096:
2091:
2089:
2088:
2087:
2068:
2063:
2061:
2053:
2052:
2043:
2028:
2023:
1981:
1979:
1978:
1973:
1971:
1970:
1954:
1952:
1951:
1946:
1930:
1928:
1927:
1922:
1906:
1904:
1903:
1898:
1886:
1884:
1883:
1878:
1876:
1875:
1859:
1857:
1856:
1851:
1837:
1836:
1824:
1820:
1798:
1797:
1787:
1776:
1739:
1737:
1736:
1731:
1719:
1717:
1716:
1711:
1699:
1697:
1696:
1691:
1686:
1684:
1662:Eulerian numbers
1657:
1655:
1654:
1649:
1644:
1642:
1641:
1632:
1631:
1622:
1619:
1614:
1599:
1591:
1586:
1585:
1576:
1575:
1574:
1561:
1554:
1553:
1528:
1523:
1507:
1502:
1454:
1452:
1451:
1446:
1428:
1426:
1425:
1420:
1402:
1400:
1399:
1394:
1376:
1374:
1373:
1368:
1350:
1348:
1347:
1342:
1316:
1314:
1313:
1308:
1296:
1294:
1293:
1288:
1270:
1268:
1267:
1262:
1238:
1236:
1235:
1230:
1212:
1210:
1209:
1204:
1192:
1190:
1189:
1184:
1150:
1148:
1147:
1142:
1124:
1122:
1121:
1116:
1114:
1113:
1045:
1043:
1042:
1037:
1035:
1031:
1002:
997:
957:. That is, as a
956:
954:
953:
948:
933:
931:
930:
925:
920:
918:
896:
894:
893:
888:
876:
874:
873:
868:
856:
854:
853:
848:
833:
831:
830:
825:
813:
811:
810:
805:
793:
791:
790:
785:
780:
778:
718:Eric Temple Bell
706:Fubini's theorem
702:Whitworth (1886)
698:Pippenger (2010)
693:
691:
690:
685:
665:
663:
662:
657:
645:
643:
642:
637:
619:
617:
616:
611:
599:
597:
596:
591:
548:
546:
545:
540:
518:
516:
515:
510:
508:
507:
485:
483:
482:
477:
441:
439:
438:
433:
410:
401:
399:
398:
393:
372:
370:
369:
364:
319:
317:
316:
311:
299:
297:
296:
291:
270:
268:
267:
262:
139:, or by using a
129:Fubini's theorem
109:Eric Temple Bell
87:
85:
84:
79:
39:
8798:
8797:
8793:
8792:
8791:
8789:
8788:
8787:
8768:
8767:
8766:
8761:
8739:
8735:Strobogrammatic
8726:
8708:
8690:
8672:
8654:
8636:
8618:
8600:
8577:
8556:
8540:
8499:Divisor-related
8494:
8454:
8405:
8375:
8312:
8296:
8275:
8242:
8215:
8203:
8185:
8097:
8096:related numbers
8070:
8047:
8014:
8005:Perfect totient
7971:
7948:
7879:Highly abundant
7821:
7800:
7732:
7715:
7687:
7670:
7656:Stirling second
7562:
7539:
7500:
7482:
7439:
7388:
7325:
7286:Centered square
7254:
7237:
7199:
7184:
7151:
7136:
7088:
7087:defined numbers
7070:
7037:
7022:
6993:Double Mersenne
6979:
6960:
6882:
6868:
6866:natural numbers
6862:
6832:
6831:
6812:
6807:
6806:
6802:
6761:
6760:
6756:
6735:
6730:
6729:
6725:
6711:10.2307/2022697
6705:(24): 722–733,
6693:Kemeny, John G.
6691:
6690:
6686:
6662:
6661:
6657:
6622:
6621:
6617:
6586:
6585:
6581:
6556:
6555:
6551:
6524:
6523:
6516:
6510:
6491:
6490:
6486:
6450:
6449:
6445:
6419:
6418:
6414:
6391:10.2307/2312790
6374:
6373:
6369:
6355:
6334:
6333:
6329:
6298:
6297:
6293:
6254:
6253:
6246:
6199:
6198:
6194:
6180:10.1137/0405040
6159:
6158:
6154:
6132:10.2307/2690567
6115:
6114:
6105:
6083:
6082:
6063:
6062:
6020:
6019:
6001:
5982:
5981:
5962:
5961:
5919:
5918:
5916:
5912:
5871:
5870:
5866:
5833:
5832:
5823:
5798:
5793:
5786:
5785:
5778:
5747:
5746:
5737:
5729:
5727:
5723:
5716:
5709:
5708:
5704:
5687:
5686:
5682:
5650:Fraenkel, A. S.
5647:
5646:
5642:
5629:
5595:
5594:
5590:
5530:
5529:
5514:
5492:10.2307/1905751
5474:Luce, R. Duncan
5472:
5471:
5467:
5454:
5453:
5449:
5405:
5404:
5393:
5373:
5372:
5355:
5333:10.2307/2312725
5316:
5315:
5304:
5282:10.2307/2690085
5262:
5261:
5254:
5247:
5229:
5228:
5219:
5214:
5180:
5179:
5145:
5144:
5143:with base case
5091:
5037:
5036:
5005:
5004:
4985:
4984:
4956:
4955:
4914:
4913:
4910:hypercube graph
4890:
4889:
4853:
4852:
4824:
4823:
4801:
4739:
4738:
4701:
4700:
4663:
4662:
4628:
4627:
4593:
4592:
4573:
4572:
4553:
4552:
4527:
4526:
4506:
4505:
4499:
4471:
4466:
4465:
4462:Euclidean plane
4440:
4435:
4434:
4433:corresponds to
4400:
4399:
4396:Euclidean space
4368:
4363:
4362:
4355:Coxeter complex
4326:
4325:
4296:
4291:
4290:
4265:
4260:
4259:
4223:
4182:
4181:
4142:
4125:
4124:
4105:
4104:
4084:
4083:
4064:
4063:
4044:
4043:
4024:
4023:
4004:
4003:
3984:
3983:
3948:
3943:
3942:
3923:
3922:
3899:
3898:
3879:
3878:
3846:
3845:
3825:
3824:
3820:
3808:
3804:
3735:
3734:
3712:
3711:
3688:
3683:
3659:
3658:
3639:
3638:
3619:
3618:
3595:
3594:
3587:
3520:
3519:
3517:
3449:
3448:
3446:
3379:
3378:
3376:
3309:
3308:
3287:
3286:
3248:
3247:
3219:
3218:
3183:
3182:
3149:
3142:
3141:
3116:
3115:
3096:
3095:
3076:
3075:
3019:
2977:
2976:
2967:
2935:
2930:
2929:
2894:
2893:
2860:
2859:
2834:
2833:
2794:
2770:
2747:
2746:
2718:
2717:
2683:
2682:
2660:
2659:
2627:
2626:
2592:
2591:
2564:
2563:
2540:
2539:
2504:
2487:
2486:
2456:
2417:
2416:
2391:
2390:
2385:stands for the
2367:
2366:
2317:
2253:
2231:
2230:
2204:
2203:
2180:
2179:
2176:identity matrix
2156:
2155:
2131:
2111:
2110:
2108:infinite matrix
2079:
2072:
2054:
2044:
2004:
2003:
1988:
1962:
1957:
1956:
1937:
1936:
1913:
1912:
1889:
1888:
1867:
1862:
1861:
1828:
1818:
1817:
1811:
1810:
1799:
1789:
1742:
1741:
1722:
1721:
1720:items in which
1702:
1701:
1665:
1664:
1633:
1623:
1577:
1556:
1539:
1468:
1467:
1464:infinite series
1431:
1430:
1405:
1404:
1379:
1378:
1353:
1352:
1327:
1326:
1319:affine subspace
1299:
1298:
1273:
1272:
1253:
1252:
1241:convex polytope
1215:
1214:
1195:
1194:
1175:
1174:
1127:
1126:
1105:
1052:
1051:
1029:
1028:
1022:
1021:
1010:
963:
962:
936:
935:
899:
898:
879:
878:
859:
858:
836:
835:
816:
815:
796:
795:
759:
758:
750:
745:
676:
675:
648:
647:
622:
621:
602:
601:
576:
575:
555:
525:
524:
493:
488:
487:
468:
467:
424:
423:
416:
406:
375:
374:
349:
348:
302:
301:
273:
272:
253:
252:
181:incomparability
165:
70:
69:
26:
17:
12:
11:
5:
8796:
8794:
8786:
8785:
8780:
8770:
8769:
8763:
8762:
8760:
8759:
8748:
8745:
8744:
8741:
8740:
8738:
8737:
8731:
8728:
8727:
8721:
8714:
8713:
8710:
8709:
8707:
8706:
8701:
8695:
8692:
8691:
8685:
8678:
8677:
8674:
8673:
8671:
8670:
8668:Sorting number
8665:
8663:Pancake number
8659:
8656:
8655:
8649:
8642:
8641:
8638:
8637:
8635:
8634:
8629:
8623:
8620:
8619:
8613:
8606:
8605:
8602:
8601:
8599:
8598:
8593:
8588:
8582:
8579:
8578:
8575:Binary numbers
8573:
8566:
8565:
8562:
8561:
8558:
8557:
8555:
8554:
8548:
8546:
8542:
8541:
8539:
8538:
8533:
8528:
8523:
8518:
8513:
8508:
8502:
8500:
8496:
8495:
8493:
8492:
8487:
8482:
8477:
8472:
8466:
8464:
8456:
8455:
8453:
8452:
8447:
8442:
8437:
8432:
8427:
8422:
8416:
8414:
8407:
8406:
8404:
8403:
8402:
8401:
8390:
8388:
8385:P-adic numbers
8381:
8380:
8377:
8376:
8374:
8373:
8372:
8371:
8361:
8356:
8351:
8346:
8341:
8336:
8331:
8326:
8320:
8318:
8314:
8313:
8311:
8310:
8304:
8302:
8301:Coding-related
8298:
8297:
8295:
8294:
8289:
8283:
8281:
8277:
8276:
8274:
8273:
8268:
8263:
8258:
8252:
8250:
8241:
8240:
8239:
8238:
8236:Multiplicative
8233:
8222:
8220:
8205:
8204:
8200:Numeral system
8198:
8191:
8190:
8187:
8186:
8184:
8183:
8178:
8173:
8168:
8163:
8158:
8153:
8148:
8143:
8138:
8133:
8128:
8123:
8118:
8113:
8108:
8102:
8099:
8098:
8087:
8080:
8079:
8076:
8075:
8072:
8071:
8069:
8068:
8063:
8057:
8055:
8049:
8048:
8046:
8045:
8040:
8035:
8030:
8024:
8022:
8016:
8015:
8013:
8012:
8007:
8002:
7997:
7992:
7990:Highly totient
7987:
7981:
7979:
7973:
7972:
7970:
7969:
7964:
7958:
7956:
7950:
7949:
7947:
7946:
7941:
7936:
7931:
7926:
7921:
7916:
7911:
7906:
7901:
7896:
7891:
7886:
7881:
7876:
7871:
7866:
7861:
7856:
7851:
7846:
7844:Almost perfect
7841:
7835:
7833:
7823:
7822:
7813:
7806:
7805:
7802:
7801:
7799:
7798:
7793:
7788:
7783:
7778:
7773:
7768:
7763:
7758:
7753:
7748:
7743:
7737:
7734:
7733:
7728:
7721:
7720:
7717:
7716:
7714:
7713:
7708:
7703:
7698:
7692:
7689:
7688:
7683:
7676:
7675:
7672:
7671:
7669:
7668:
7663:
7658:
7653:
7651:Stirling first
7648:
7643:
7638:
7633:
7628:
7623:
7618:
7613:
7608:
7603:
7598:
7593:
7588:
7583:
7578:
7573:
7567:
7564:
7563:
7560:
7553:
7552:
7549:
7548:
7545:
7544:
7541:
7540:
7538:
7537:
7532:
7527:
7521:
7519:
7512:
7506:
7505:
7502:
7501:
7499:
7498:
7492:
7490:
7484:
7483:
7481:
7480:
7475:
7470:
7465:
7460:
7455:
7449:
7447:
7441:
7440:
7438:
7437:
7432:
7427:
7422:
7417:
7411:
7409:
7400:
7394:
7393:
7390:
7389:
7387:
7386:
7381:
7376:
7371:
7366:
7361:
7356:
7351:
7346:
7341:
7335:
7333:
7327:
7326:
7324:
7323:
7318:
7313:
7308:
7303:
7298:
7293:
7288:
7283:
7277:
7275:
7266:
7256:
7255:
7250:
7243:
7242:
7239:
7238:
7236:
7235:
7230:
7225:
7220:
7215:
7210:
7204:
7201:
7200:
7197:
7190:
7189:
7186:
7185:
7183:
7182:
7177:
7172:
7167:
7162:
7156:
7153:
7152:
7149:
7142:
7141:
7138:
7137:
7135:
7134:
7129:
7124:
7119:
7114:
7109:
7104:
7099:
7093:
7090:
7089:
7083:
7076:
7075:
7072:
7071:
7069:
7068:
7063:
7058:
7053:
7048:
7042:
7039:
7038:
7035:
7028:
7027:
7024:
7023:
7021:
7020:
7015:
7010:
7005:
7000:
6995:
6990:
6984:
6981:
6980:
6973:
6966:
6965:
6962:
6961:
6959:
6958:
6953:
6948:
6943:
6938:
6933:
6928:
6923:
6918:
6913:
6908:
6903:
6898:
6893:
6887:
6884:
6883:
6877:
6870:
6869:
6863:
6861:
6860:
6853:
6846:
6838:
6830:
6829:
6800:
6754:
6723:
6684:
6655:
6637:(4): 563–581,
6615:
6579:
6549:
6539:(1): 127–143,
6514:
6508:
6484:
6443:
6412:
6367:
6353:
6327:
6309:(3): 311–313,
6291:
6265:(4): 559–562,
6244:
6192:
6174:(4): 497–499,
6160:Getu, Seyoum;
6152:
6126:(4): 243–253,
6103:
6090:
6070:
6050:
6043:
6040:
6033:
6030:
6027:
5989:
5969:
5949:
5942:
5939:
5932:
5929:
5926:
5910:
5882:(4): 453–476,
5864:
5821:
5776:
5758:(4): 563–581,
5735:
5702:
5697:, as cited by
5680:
5662:(1): 101–112,
5640:
5627:
5588:
5544:(5): 331–346,
5512:
5465:
5447:
5419:(5): 701–705,
5391:
5353:
5302:
5276:(3): 170–175,
5252:
5245:
5216:
5215:
5213:
5210:
5196:
5193:
5190:
5187:
5167:
5164:
5161:
5158:
5155:
5152:
5132:
5129:
5126:
5123:
5120:
5117:
5114:
5108:
5103:
5100:
5095:
5087:
5084:
5081:
5076:
5073:
5070:
5066:
5062:
5059:
5056:
5053:
5050:
5047:
5044:
5021:
5018:
5015:
5012:
4992:
4972:
4969:
4966:
4963:
4952:spam filtering
4945:graded algebra
4921:
4897:
4880:) provides an
4866:
4863:
4837:
4834:
4800:
4797:
4761:
4758:
4755:
4752:
4749:
4746:
4736:unary relation
4723:
4720:
4717:
4714:
4711:
4708:
4685:
4682:
4679:
4676:
4673:
4670:
4650:
4647:
4644:
4641:
4638:
4635:
4615:
4612:
4609:
4606:
4603:
4600:
4580:
4560:
4540:
4537:
4534:
4513:
4502:-ary relations
4478:
4474:
4447:
4443:
4422:
4419:
4416:
4413:
4410:
4407:
4381:
4378:
4375:
4371:
4342:
4339:
4336:
4333:
4303:
4299:
4272:
4268:
4241:
4236:
4233:
4230:
4226:
4222:
4219:
4216:
4213:
4210:
4207:
4204:
4201:
4198:
4195:
4192:
4189:
4155:
4152:
4149:
4145:
4141:
4138:
4135:
4132:
4112:
4091:
4071:
4051:
4031:
4011:
3991:
3961:
3958:
3955:
3951:
3930:
3906:
3886:
3877:with exponent
3862:
3859:
3856:
3853:
3832:
3782:crease pattern
3774:assembly lines
3745:
3742:
3719:
3700:photo finishes
3687:
3684:
3682:
3679:
3666:
3646:
3626:
3602:
3576:
3572:
3569:
3565:
3562:
3557:
3554:
3551:
3548:
3545:
3542:
3539:
3536:
3533:
3530:
3527:
3518:
3505:
3501:
3498:
3494:
3491:
3486:
3483:
3480:
3477:
3474:
3471:
3468:
3465:
3462:
3459:
3456:
3447:
3435:
3431:
3428:
3424:
3421:
3416:
3413:
3410:
3407:
3404:
3401:
3398:
3395:
3392:
3389:
3386:
3377:
3365:
3361:
3358:
3354:
3351:
3346:
3343:
3340:
3337:
3334:
3331:
3328:
3325:
3322:
3319:
3316:
3307:
3294:
3270:
3267:
3264:
3261:
3258:
3255:
3235:
3232:
3229:
3226:
3205:
3202:
3199:
3196:
3193:
3190:
3166:
3161:
3158:
3153:
3129:
3126:
3123:
3103:
3083:
3072:
3071:
3060:
3057:
3054:
3051:
3048:
3045:
3042:
3036:
3031:
3028:
3023:
3015:
3010:
3007:
3004:
3000:
2996:
2993:
2990:
2987:
2984:
2966:
2963:
2950:
2947:
2942:
2938:
2913:
2910:
2907:
2904:
2901:
2881:
2878:
2875:
2871:
2867:
2847:
2844:
2841:
2821:
2818:
2815:
2812:
2806:
2803:
2800:
2797:
2792:
2789:
2786:
2783:
2780:
2777:
2773:
2765:
2762:
2759:
2755:
2734:
2731:
2728:
2725:
2705:
2702:
2699:
2696:
2693:
2690:
2667:
2643:
2640:
2637:
2634:
2614:
2611:
2608:
2605:
2602:
2599:
2588:relative error
2571:
2547:
2523:
2520:
2513:
2510:
2507:
2503:
2497:
2494:
2474:
2469:
2466:
2463:
2459:
2453:
2450:
2444:
2441:
2436:
2433:
2430:
2427:
2424:
2404:
2401:
2398:
2374:
2354:
2351:
2348:
2344:
2339:
2336:
2333:
2330:
2327:
2324:
2320:
2316:
2313:
2310:
2307:
2304:
2301:
2298:
2295:
2292:
2287:
2282:
2279:
2276:
2273:
2269:
2263:
2259:
2256:
2250:
2247:
2244:
2241:
2238:
2211:
2202:. Each row of
2187:
2163:
2141:
2138:
2134:
2130:
2127:
2124:
2121:
2118:
2094:
2086:
2082:
2078:
2075:
2071:
2066:
2060:
2057:
2051:
2047:
2041:
2038:
2035:
2032:
2027:
2022:
2019:
2016:
2012:
1987:
1984:
1969:
1965:
1944:
1920:
1896:
1874:
1870:
1849:
1846:
1843:
1840:
1835:
1831:
1827:
1823:
1816:
1813:
1812:
1809:
1806:
1805:
1802:
1796:
1792:
1786:
1783:
1780:
1775:
1772:
1769:
1765:
1761:
1758:
1755:
1752:
1749:
1729:
1709:
1689:
1682:
1679:
1672:
1647:
1640:
1636:
1630:
1626:
1618:
1613:
1610:
1607:
1603:
1597:
1594:
1589:
1584:
1580:
1573:
1568:
1565:
1560:
1552:
1549:
1546:
1542:
1538:
1535:
1532:
1527:
1522:
1519:
1516:
1512:
1506:
1501:
1498:
1495:
1491:
1487:
1484:
1481:
1478:
1475:
1444:
1441:
1438:
1418:
1415:
1412:
1392:
1389:
1386:
1366:
1363:
1360:
1340:
1337:
1334:
1306:
1286:
1283:
1280:
1260:
1228:
1225:
1222:
1202:
1182:
1140:
1137:
1134:
1112:
1108:
1104:
1101:
1098:
1095:
1092:
1089:
1086:
1083:
1080:
1077:
1074:
1071:
1068:
1065:
1062:
1059:
1034:
1027:
1024:
1023:
1020:
1017:
1016:
1013:
1009:
1006:
1001:
996:
993:
990:
986:
982:
979:
976:
973:
970:
946:
943:
923:
916:
913:
906:
886:
866:
846:
843:
823:
803:
783:
776:
773:
766:
749:
746:
744:
741:
716:, named after
683:
655:
635:
632:
629:
609:
589:
586:
583:
554:
551:
538:
535:
532:
506:
503:
500:
496:
475:
431:
404:
391:
388:
385:
382:
362:
359:
356:
347:Starting from
309:
289:
286:
283:
280:
260:
164:
161:
77:
62:weak orderings
58:Fubini numbers
15:
13:
10:
9:
6:
4:
3:
2:
8795:
8784:
8781:
8779:
8776:
8775:
8773:
8758:
8754:
8750:
8749:
8746:
8736:
8733:
8732:
8729:
8724:
8719:
8715:
8705:
8702:
8700:
8697:
8696:
8693:
8688:
8683:
8679:
8669:
8666:
8664:
8661:
8660:
8657:
8652:
8647:
8643:
8633:
8630:
8628:
8625:
8624:
8621:
8617:
8611:
8607:
8597:
8594:
8592:
8589:
8587:
8584:
8583:
8580:
8576:
8571:
8567:
8553:
8550:
8549:
8547:
8543:
8537:
8534:
8532:
8529:
8527:
8526:Polydivisible
8524:
8522:
8519:
8517:
8514:
8512:
8509:
8507:
8504:
8503:
8501:
8497:
8491:
8488:
8486:
8483:
8481:
8478:
8476:
8473:
8471:
8468:
8467:
8465:
8462:
8457:
8451:
8448:
8446:
8443:
8441:
8438:
8436:
8433:
8431:
8428:
8426:
8423:
8421:
8418:
8417:
8415:
8412:
8408:
8400:
8397:
8396:
8395:
8392:
8391:
8389:
8386:
8382:
8370:
8367:
8366:
8365:
8362:
8360:
8357:
8355:
8352:
8350:
8347:
8345:
8342:
8340:
8337:
8335:
8332:
8330:
8327:
8325:
8322:
8321:
8319:
8315:
8309:
8306:
8305:
8303:
8299:
8293:
8290:
8288:
8285:
8284:
8282:
8280:Digit product
8278:
8272:
8269:
8267:
8264:
8262:
8259:
8257:
8254:
8253:
8251:
8249:
8245:
8237:
8234:
8232:
8229:
8228:
8227:
8224:
8223:
8221:
8219:
8214:
8210:
8206:
8201:
8196:
8192:
8182:
8179:
8177:
8174:
8172:
8169:
8167:
8164:
8162:
8159:
8157:
8154:
8152:
8149:
8147:
8144:
8142:
8139:
8137:
8134:
8132:
8129:
8127:
8124:
8122:
8119:
8117:
8116:Erdős–Nicolas
8114:
8112:
8109:
8107:
8104:
8103:
8100:
8095:
8091:
8085:
8081:
8067:
8064:
8062:
8059:
8058:
8056:
8054:
8050:
8044:
8041:
8039:
8036:
8034:
8031:
8029:
8026:
8025:
8023:
8021:
8017:
8011:
8008:
8006:
8003:
8001:
7998:
7996:
7993:
7991:
7988:
7986:
7983:
7982:
7980:
7978:
7974:
7968:
7965:
7963:
7960:
7959:
7957:
7955:
7951:
7945:
7942:
7940:
7937:
7935:
7934:Superabundant
7932:
7930:
7927:
7925:
7922:
7920:
7917:
7915:
7912:
7910:
7907:
7905:
7902:
7900:
7897:
7895:
7892:
7890:
7887:
7885:
7882:
7880:
7877:
7875:
7872:
7870:
7867:
7865:
7862:
7860:
7857:
7855:
7852:
7850:
7847:
7845:
7842:
7840:
7837:
7836:
7834:
7832:
7828:
7824:
7820:
7816:
7811:
7807:
7797:
7794:
7792:
7789:
7787:
7784:
7782:
7779:
7777:
7774:
7772:
7769:
7767:
7764:
7762:
7759:
7757:
7754:
7752:
7749:
7747:
7744:
7742:
7739:
7738:
7735:
7731:
7726:
7722:
7712:
7709:
7707:
7704:
7702:
7699:
7697:
7694:
7693:
7690:
7686:
7681:
7677:
7667:
7664:
7662:
7659:
7657:
7654:
7652:
7649:
7647:
7644:
7642:
7639:
7637:
7634:
7632:
7629:
7627:
7624:
7622:
7619:
7617:
7614:
7612:
7609:
7607:
7604:
7602:
7599:
7597:
7594:
7592:
7589:
7587:
7584:
7582:
7579:
7577:
7574:
7572:
7569:
7568:
7565:
7558:
7554:
7536:
7533:
7531:
7528:
7526:
7523:
7522:
7520:
7516:
7513:
7511:
7510:4-dimensional
7507:
7497:
7494:
7493:
7491:
7489:
7485:
7479:
7476:
7474:
7471:
7469:
7466:
7464:
7461:
7459:
7456:
7454:
7451:
7450:
7448:
7446:
7442:
7436:
7433:
7431:
7428:
7426:
7423:
7421:
7420:Centered cube
7418:
7416:
7413:
7412:
7410:
7408:
7404:
7401:
7399:
7398:3-dimensional
7395:
7385:
7382:
7380:
7377:
7375:
7372:
7370:
7367:
7365:
7362:
7360:
7357:
7355:
7352:
7350:
7347:
7345:
7342:
7340:
7337:
7336:
7334:
7332:
7328:
7322:
7319:
7317:
7314:
7312:
7309:
7307:
7304:
7302:
7299:
7297:
7294:
7292:
7289:
7287:
7284:
7282:
7279:
7278:
7276:
7274:
7270:
7267:
7265:
7264:2-dimensional
7261:
7257:
7253:
7248:
7244:
7234:
7231:
7229:
7226:
7224:
7221:
7219:
7216:
7214:
7211:
7209:
7208:Nonhypotenuse
7206:
7205:
7202:
7195:
7191:
7181:
7178:
7176:
7173:
7171:
7168:
7166:
7163:
7161:
7158:
7157:
7154:
7147:
7143:
7133:
7130:
7128:
7125:
7123:
7120:
7118:
7115:
7113:
7110:
7108:
7105:
7103:
7100:
7098:
7095:
7094:
7091:
7086:
7081:
7077:
7067:
7064:
7062:
7059:
7057:
7054:
7052:
7049:
7047:
7044:
7043:
7040:
7033:
7029:
7019:
7016:
7014:
7011:
7009:
7006:
7004:
7001:
6999:
6996:
6994:
6991:
6989:
6986:
6985:
6982:
6977:
6971:
6967:
6957:
6954:
6952:
6949:
6947:
6946:Perfect power
6944:
6942:
6939:
6937:
6936:Seventh power
6934:
6932:
6929:
6927:
6924:
6922:
6919:
6917:
6914:
6912:
6909:
6907:
6904:
6902:
6899:
6897:
6894:
6892:
6889:
6888:
6885:
6880:
6875:
6871:
6867:
6859:
6854:
6852:
6847:
6845:
6840:
6839:
6836:
6826:
6822:
6818:
6811:
6804:
6801:
6797:
6793:
6789:
6785:
6780:
6775:
6771:
6767:
6766:
6758:
6755:
6750:
6745:
6741:
6734:
6727:
6724:
6720:
6716:
6712:
6708:
6704:
6700:
6699:
6694:
6688:
6685:
6679:
6674:
6670:
6666:
6659:
6656:
6652:
6648:
6644:
6640:
6636:
6632:
6631:
6626:
6619:
6616:
6611:
6606:
6602:
6598:
6594:
6590:
6583:
6580:
6576:
6572:
6568:
6564:
6560:
6553:
6550:
6546:
6542:
6538:
6534:
6533:
6528:
6521:
6519:
6515:
6511:
6509:9780387797106
6505:
6501:
6497:
6496:
6488:
6485:
6481:
6477:
6472:
6467:
6463:
6459:
6458:
6453:
6452:Diagana, Toka
6447:
6444:
6440:
6436:
6432:
6428:
6427:
6422:
6421:Poonen, Bjorn
6416:
6413:
6408:
6404:
6400:
6396:
6392:
6388:
6384:
6380:
6379:
6371:
6368:
6364:
6360:
6356:
6354:0-387-96110-0
6350:
6346:
6342:
6338:
6331:
6328:
6324:
6320:
6316:
6312:
6308:
6304:
6303:
6295:
6292:
6288:
6284:
6280:
6276:
6272:
6268:
6264:
6260:
6259:
6251:
6249:
6245:
6241:
6237:
6233:
6229:
6225:
6221:
6217:
6213:
6209:
6205:
6204:
6196:
6193:
6189:
6185:
6181:
6177:
6173:
6169:
6168:
6163:
6156:
6153:
6149:
6145:
6141:
6137:
6133:
6129:
6125:
6121:
6120:
6112:
6110:
6108:
6104:
6088:
6068:
6041:
6038:
6028:
6025:
6015:
6014:
6008:
6004:
5987:
5967:
5940:
5937:
5927:
5924:
5914:
5911:
5907:
5903:
5899:
5895:
5890:
5885:
5881:
5877:
5876:
5868:
5865:
5861:
5857:
5852:
5847:
5843:
5839:
5838:
5830:
5828:
5826:
5822:
5818:
5814:
5810:
5806:
5805:
5797:
5789:
5783:
5781:
5777:
5773:
5769:
5765:
5761:
5757:
5753:
5752:
5744:
5742:
5740:
5736:
5726:on 2014-07-04
5722:
5715:
5714:
5706:
5703:
5700:
5694:
5690:
5684:
5681:
5677:
5673:
5669:
5665:
5661:
5657:
5656:
5651:
5644:
5641:
5638:
5636:
5630:
5628:9781108004961
5624:
5620:
5616:
5612:
5609:, Series IV,
5608:
5607:
5602:
5598:
5592:
5589:
5585:
5581:
5577:
5573:
5569:
5565:
5561:
5557:
5552:
5547:
5543:
5539:
5538:
5533:
5527:
5525:
5523:
5521:
5519:
5517:
5513:
5509:
5505:
5501:
5497:
5493:
5489:
5485:
5481:
5480:
5475:
5469:
5466:
5461:
5457:
5451:
5448:
5444:
5440:
5436:
5432:
5427:
5422:
5418:
5414:
5413:
5408:
5402:
5400:
5398:
5396:
5392:
5387:
5386:
5380:
5376:
5370:
5368:
5366:
5364:
5362:
5360:
5358:
5354:
5350:
5346:
5342:
5338:
5334:
5330:
5326:
5322:
5321:
5313:
5311:
5309:
5307:
5303:
5299:
5295:
5291:
5287:
5283:
5279:
5275:
5271:
5270:
5265:
5259:
5257:
5253:
5248:
5246:9780821886311
5242:
5238:
5237:
5232:
5226:
5224:
5222:
5218:
5211:
5209:
5191:
5185:
5165:
5162:
5156:
5150:
5130:
5124:
5121:
5118:
5112:
5101:
5098:
5085:
5082:
5079:
5074:
5071:
5068:
5064:
5060:
5057:
5054:
5048:
5042:
5035:
5016:
5010:
5003:words, where
4990:
4967:
4961:
4953:
4948:
4946:
4942:
4938:
4933:
4919:
4911:
4908:-dimensional
4895:
4887:
4883:
4864:
4861:
4835:
4832:
4821:
4817:
4814:Although the
4812:
4810:
4806:
4798:
4796:
4794:
4789:
4785:
4781:
4777:
4773:
4756:
4750:
4747:
4744:
4737:
4718:
4712:
4709:
4706:
4699:
4680:
4674:
4671:
4668:
4648:
4645:
4639:
4633:
4610:
4604:
4601:
4598:
4578:
4558:
4538:
4535:
4532:
4511:
4503:
4496:
4495:Kemeny (1956)
4492:
4476:
4472:
4463:
4445:
4441:
4420:
4417:
4411:
4405:
4397:
4379:
4376:
4373:
4369:
4360:
4359:Coxeter group
4356:
4337:
4331:
4319:
4318:great circles
4301:
4297:
4288:
4270:
4266:
4256:
4252:
4239:
4234:
4231:
4228:
4220:
4217:
4214:
4208:
4202:
4196:
4193:
4190:
4187:
4179:
4175:
4171:
4153:
4150:
4147:
4139:
4136:
4133:
4110:
4089:
4069:
4049:
4029:
4009:
3989:
3981:
3976:
3959:
3956:
3953:
3949:
3928:
3920:
3904:
3884:
3876:
3857:
3851:
3830:
3818:
3817:prime numbers
3814:
3802:
3798:
3794:
3791:, an ordered
3790:
3789:number theory
3785:
3783:
3779:
3775:
3771:
3766:
3762:
3757:
3743:
3740:
3733:
3717:
3709:
3705:
3701:
3697:
3693:
3692:permutohedron
3685:
3680:
3678:
3664:
3644:
3624:
3616:
3600:
3592:
3574:
3567:
3563:
3552:
3546:
3543:
3537:
3534:
3531:
3525:
3503:
3496:
3492:
3481:
3475:
3472:
3466:
3463:
3460:
3454:
3433:
3426:
3422:
3411:
3405:
3402:
3396:
3393:
3390:
3384:
3363:
3356:
3352:
3341:
3335:
3332:
3326:
3323:
3320:
3314:
3306:
3292:
3284:
3268:
3265:
3259:
3253:
3230:
3224:
3200:
3197:
3194:
3188:
3159:
3156:
3127:
3124:
3121:
3101:
3081:
3058:
3052:
3049:
3046:
3040:
3029:
3026:
3013:
3008:
3005:
3002:
2998:
2994:
2988:
2982:
2975:
2974:
2973:
2972:
2964:
2962:
2948:
2945:
2940:
2936:
2927:
2911:
2908:
2905:
2902:
2899:
2879:
2876:
2873:
2869:
2865:
2845:
2842:
2839:
2819:
2816:
2813:
2810:
2801:
2795:
2787:
2784:
2781:
2775:
2771:
2757:
2729:
2723:
2700:
2697:
2694:
2688:
2679:
2665:
2657:
2638:
2632:
2609:
2603:
2600:
2597:
2589:
2585:
2569:
2561:
2545:
2537:
2521:
2518:
2511:
2508:
2505:
2501:
2495:
2492:
2472:
2467:
2464:
2461:
2457:
2451:
2448:
2442:
2439:
2434:
2428:
2422:
2402:
2399:
2396:
2388:
2372:
2352:
2349:
2346:
2342:
2334:
2331:
2328:
2322:
2314:
2311:
2308:
2305:
2302:
2299:
2296:
2293:
2277:
2274:
2271:
2267:
2261:
2257:
2254:
2248:
2242:
2236:
2228:
2223:
2209:
2201:
2185:
2177:
2161:
2139:
2136:
2128:
2125:
2122:
2119:
2109:
2092:
2084:
2080:
2076:
2073:
2069:
2064:
2058:
2055:
2049:
2045:
2036:
2030:
2020:
2017:
2014:
2010:
2001:
1997:
1993:
1985:
1983:
1967:
1963:
1942:
1934:
1918:
1910:
1894:
1872:
1868:
1847:
1841:
1833:
1829:
1825:
1821:
1814:
1807:
1800:
1794:
1790:
1784:
1781:
1778:
1773:
1770:
1767:
1763:
1759:
1753:
1747:
1727:
1707:
1680:
1677:
1663:
1658:
1645:
1638:
1634:
1628:
1624:
1611:
1608:
1605:
1601:
1595:
1592:
1587:
1582:
1578:
1566:
1563:
1550:
1547:
1544:
1536:
1533:
1525:
1520:
1517:
1514:
1510:
1504:
1499:
1496:
1493:
1489:
1485:
1479:
1473:
1465:
1461:
1456:
1442:
1439:
1436:
1416:
1413:
1410:
1390:
1387:
1384:
1377:), 36 edges (
1364:
1361:
1358:
1338:
1335:
1332:
1324:
1320:
1317:-dimensional
1304:
1284:
1281:
1278:
1258:
1250:
1246:
1242:
1226:
1223:
1220:
1200:
1180:
1173:of dimension
1172:
1171:permutohedron
1165:
1164:permutohedron
1161:
1156:
1152:
1138:
1135:
1132:
1110:
1102:
1096:
1093:
1087:
1084:
1081:
1075:
1069:
1066:
1063:
1057:
1049:
1032:
1025:
1018:
1011:
1007:
1004:
999:
994:
991:
988:
984:
980:
974:
968:
960:
944:
941:
914:
911:
884:
864:
844:
841:
821:
801:
774:
771:
756:
747:
742:
740:
738:
734:
729:
727:
723:
719:
715:
711:
707:
703:
699:
695:
681:
673:
669:
653:
633:
630:
627:
607:
587:
584:
581:
573:
569:
568:Cayley (1859)
559:
552:
550:
536:
533:
530:
522:
504:
501:
498:
494:
473:
465:
461:
457:
453:
449:
445:
429:
421:
414:
409:
403:
386:
380:
360:
357:
354:
345:
343:
339:
335:
331:
327:
323:
307:
284:
278:
258:
249:
247:
243:
239:
236:is less than
235:
231:
227:
223:
219:
215:
211:
207:
203:
199:
195:
190:
186:
182:
178:
174:
170:
162:
160:
158:
157:permutohedron
154:
150:
146:
142:
138:
133:
130:
126:
122:
118:
114:
110:
106:
102:
101:Arthur Cayley
97:
95:
91:
75:
67:
63:
59:
55:
51:
47:
46:number theory
38:
34:
30:
23:
19:
8490:Transposable
8354:Narcissistic
8261:Digital root
8181:Super-Poulet
8141:Jordan–Pólya
8090:prime factor
7995:Noncototient
7962:Almost prime
7944:Superperfect
7919:Refactorable
7914:Quasiperfect
7889:Hyperperfect
7730:Pseudoprimes
7701:Wall–Sun–Sun
7636:Ordered Bell
7635:
7606:Fuss–Catalan
7518:non-centered
7468:Dodecahedral
7445:non-centered
7331:non-centered
7233:Wolstenholme
6978:× 2 ± 1
6975:
6974:Of the form
6941:Eighth power
6921:Fourth power
6816:
6803:
6769:
6763:
6757:
6749:math/0605061
6739:
6726:
6702:
6696:
6687:
6668:
6658:
6634:
6628:
6618:
6592:
6588:
6582:
6566:
6562:
6558:
6552:
6536:
6530:
6526:
6494:
6487:
6461:
6455:
6446:
6433:(1): 70–76,
6430:
6424:
6415:
6382:
6376:
6370:
6336:
6330:
6306:
6300:
6294:
6262:
6256:
6210:(1): 50–66,
6207:
6201:
6195:
6171:
6165:
6155:
6123:
6117:
6010:
5913:
5889:math/0602672
5879:
5873:
5867:
5841:
5835:
5808:
5802:
5755:
5749:
5728:, retrieved
5721:the original
5712:
5705:
5692:
5683:
5659:
5653:
5643:
5634:
5610:
5604:
5591:
5541:
5535:
5483:
5479:Econometrica
5477:
5468:
5459:
5450:
5416:
5410:
5382:
5324:
5318:
5273:
5267:
5235:
4949:
4934:
4820:power series
4813:
4802:
4774:
4493:
4323:
4170:permutations
3977:
3786:
3769:
3758:
3696:horse racing
3689:
3681:Applications
3588:
3073:
2968:
2680:
2224:
1992:power series
1989:
1933:permutations
1659:
1457:
1249:permutations
1168:
751:
733:Donald Knuth
730:
720:, count the
714:Bell numbers
710:Guido Fubini
696:
565:
521:partial sums
463:
459:
455:
451:
447:
443:
417:
346:
341:
337:
333:
329:
325:
321:
250:
245:
241:
237:
233:
229:
225:
221:
217:
213:
209:
205:
201:
166:
134:
125:Guido Fubini
113:Bell numbers
98:
57:
53:
43:
36:
32:
28:
18:
8511:Extravagant
8506:Equidigital
8461:permutation
8420:Palindromic
8394:Automorphic
8292:Sum-product
8271:Sum-product
8226:Persistence
8121:Erdős–Woods
8043:Untouchable
7924:Semiperfect
7874:Hemiperfect
7535:Tesseractic
7473:Icosahedral
7453:Tetrahedral
7384:Dodecagonal
7085:Recursively
6956:Prime power
6931:Sixth power
6926:Fifth power
6906:Power of 10
6864:Classes of
6569:: 318–325,
6464:: 547–569,
5788:Good, I. J.
5486:: 178–191,
4943:produces a
4784:linguistics
4287:unit sphere
4123:is exactly
3919:composition
3875:prime power
3730:items is a
3591:prime power
2745:shows that
2654:error term
2225:Based on a
1245:convex hull
1193:, with the
757:, denoted
726:total order
572:plane trees
486:is exactly
420:composition
198:total order
173:horse races
121:total order
8772:Categories
8723:Graphemics
8596:Pernicious
8450:Undulating
8425:Pandigital
8399:Trimorphic
8000:Nontotient
7849:Arithmetic
7463:Octahedral
7364:Heptagonal
7354:Pentagonal
7339:Triangular
7180:Sierpiński
7102:Jacobsthal
6901:Power of 3
6896:Power of 2
6678:2305.15554
5730:2013-03-12
5597:Cayley, A.
5407:Sklar, Abe
5327:(1): 4–8,
5212:References
4793:factorials
3813:squarefree
3805:6 · 5
3704:dead heats
2625:, and the
2558:indicates
1048:log-convex
179:for which
153:squarefree
94:horse race
60:count the
8480:Parasitic
8329:Factorion
8256:Digit sum
8248:Digit sum
8066:Fortunate
8053:Primorial
7967:Semiprime
7904:Practical
7869:Descartes
7864:Deficient
7854:Betrothed
7696:Wieferich
7525:Pentatope
7488:pyramidal
7379:Decagonal
7374:Nonagonal
7369:Octagonal
7359:Hexagonal
7218:Practical
7165:Congruent
7097:Fibonacci
7061:Loeschian
6779:1410.1772
6385:(1): 62,
6287:120845059
6240:207520945
5811:: 11–18,
5648:Mor, M.;
5551:0904.1757
5122:−
5083:−
5065:∑
4477:∘
4377:−
4232:−
4209:≤
4194:≤
4151:−
3957:−
3763:consider
3544:≡
3473:≡
3403:≡
3333:≡
3198:−
3125:−
3050:−
2999:∑
2926:Ramanujan
2909:≈
2903:
2877:≈
2817:
2785:−
2764:∞
2761:→
2698:−
2601:±
2546:∼
2519:≈
2509:
2435:∼
2350:≥
2323:−
2309:π
2297:
2286:∞
2281:∞
2278:−
2268:∑
2137:−
2126:−
2077:−
2026:∞
2011:∑
1782:−
1764:∑
1688:⟩
1671:⟨
1617:∞
1602:∑
1548:−
1534:−
1511:∑
1490:∑
1224:−
1094:≥
1067:−
985:∑
961:formula:
959:summation
748:Summation
534:−
502:−
8552:Friedman
8485:Primeval
8430:Repdigit
8387:-related
8334:Kaprekar
8308:Meertens
8231:Additive
8218:dynamics
8126:Friendly
8038:Sociable
8028:Amicable
7839:Abundant
7819:dynamics
7641:Schröder
7631:Narayana
7601:Eulerian
7591:Delannoy
7586:Dedekind
7407:centered
7273:centered
7160:Amenable
7117:Narayana
7107:Leonardo
7003:Mersenne
6951:Powerful
6891:Achilles
5790:(1975),
5691:(1886),
5637:, p. 113
5599:(1859),
5584:17260512
5458:(1995),
5233:(2009),
4884:for the
4361:of type
3801:divisors
3708:baseball
2912:0.693145
2880:0.693161
1822:⟩
1801:⟨
1125:for all
743:Formulas
562:numbers.
8725:related
8689:related
8653:related
8651:Sorting
8536:Vampire
8521:Harshad
8463:related
8435:Repunit
8349:Lychrel
8324:Dudeney
8176:Størmer
8171:Sphenic
8156:Regular
8094:divisor
8033:Perfect
7929:Sublime
7899:Perfect
7626:Motzkin
7581:Catalan
7122:Padovan
7056:Leyland
7051:Idoneal
7046:Hilbert
7018:Woodall
6796:3427040
6719:2022697
6651:2196796
6610:6834023
6545:4176645
6480:3581932
6439:0931425
6407:0144827
6399:2312790
6363:0781125
6323:0560774
6279:1647055
6232:2599467
6188:1186818
6148:1363707
6140:2690567
6061:as the
6005:(ed.),
5906:2356431
5860:1297386
5817:0376367
5772:2196796
5676:0732206
5576:2762645
5508:0078632
5500:1905751
5443:0050620
5435:2032169
5377:(ed.),
5349:0130837
5341:2312725
5298:0653432
5290:2690085
4809:moments
3778:origami
2678:grows.
2174:is the
2154:. Here
1887:is the
553:History
411:in the
408:A000670
340:before
336:, with
332:before
8591:Odious
8516:Frugal
8470:Cyclic
8459:Digit-
8166:Smooth
8151:Pronic
8111:Cyclic
8088:Other
8061:Euclid
7711:Wilson
7685:Primes
7344:Square
7213:Polite
7175:Riesel
7170:Knödel
7132:Perrin
7013:Thabit
6998:Fermat
6988:Cullen
6911:Square
6879:Powers
6794:
6717:
6649:
6607:
6543:
6506:
6478:
6437:
6405:
6397:
6361:
6351:
6321:
6285:
6277:
6238:
6230:
6222:
6186:
6146:
6138:
5904:
5858:
5815:
5770:
5674:
5625:
5582:
5574:
5566:
5506:
5498:
5441:
5433:
5347:
5339:
5296:
5288:
5243:
4807:. The
4696:, the
2586:, the
2522:1.4427
2485:where
2365:Here,
1860:where
1243:, the
712:. The
244:, and
187:. The
183:is an
52:, the
8632:Prime
8627:Lucky
8616:sieve
8545:Other
8531:Smith
8411:Digit
8369:Happy
8344:Keith
8317:Other
8161:Rough
8131:Giuga
7596:Euler
7458:Cubic
7112:Lucas
7008:Proth
6813:(PDF)
6774:arXiv
6744:arXiv
6736:(PDF)
6715:JSTOR
6673:arXiv
6541:JSTOR
6395:JSTOR
6283:S2CID
6236:S2CID
6220:JSTOR
6136:JSTOR
5884:arXiv
5799:(PDF)
5724:(PDF)
5717:(PDF)
5632:, in
5580:S2CID
5564:JSTOR
5546:arXiv
5496:JSTOR
5431:JSTOR
5337:JSTOR
5286:JSTOR
4799:Other
3795:of a
3568:10000
574:with
151:of a
64:on a
8586:Evil
8266:Self
8216:and
8106:Blum
7817:and
7621:Lobb
7576:Cake
7571:Bell
7321:Star
7228:Ulam
7127:Pell
6916:Cube
6559:Seru
6504:ISBN
6349:ISBN
6011:The
5623:ISBN
5383:The
5241:ISBN
4571:and
4176:for
3770:seru
3497:1000
2716:and
2590:is
2178:and
1136:>
413:OEIS
402:are
324:and
251:The
228:and
169:ties
127:and
103:and
90:ties
48:and
8704:Ban
8092:or
7611:Lah
6821:doi
6784:doi
6707:doi
6639:doi
6605:PMC
6597:doi
6593:475
6571:doi
6567:122
6466:doi
6462:173
6387:doi
6341:doi
6311:doi
6267:doi
6212:doi
6208:117
6176:doi
6128:doi
5894:doi
5846:doi
5842:132
5760:doi
5664:doi
5615:doi
5556:doi
5488:doi
5421:doi
5329:doi
5278:doi
4950:In
4782:in
3921:of
3787:In
3617:of
3564:mod
3538:500
3516:and
3493:mod
3467:100
3427:100
3423:mod
3353:mod
2961:).
2900:log
2892:to
2874:541
2866:375
2814:log
2754:lim
2658:as
2506:log
2373:log
2294:log
1931:to
1907:th
454:},{
450:},{
212:},{
208:},{
68:of
66:set
56:or
44:In
8774::
6815:,
6792:MR
6790:,
6782:,
6770:29
6768:,
6738:,
6713:,
6703:52
6701:,
6671:,
6647:MR
6645:,
6633:,
6627:,
6603:,
6591:,
6565:,
6537:37
6535:,
6517:^
6498:,
6476:MR
6474:,
6460:,
6435:MR
6431:26
6429:,
6403:MR
6401:,
6393:,
6383:70
6381:,
6359:MR
6357:,
6347:,
6319:MR
6317:,
6307:29
6305:,
6281:,
6275:MR
6273:,
6263:15
6261:,
6247:^
6234:,
6228:MR
6226:,
6218:,
6206:,
6184:MR
6182:,
6170:,
6144:MR
6142:,
6134:,
6124:68
6122:,
6106:^
6009:,
5902:MR
5900:,
5892:,
5880:39
5878:,
5856:MR
5854:,
5840:,
5824:^
5813:MR
5809:13
5807:,
5801:,
5779:^
5768:MR
5766:,
5754:,
5738:^
5672:MR
5670:,
5660:48
5658:,
5621:,
5611:18
5603:,
5578:,
5572:MR
5570:,
5562:,
5554:,
5542:83
5540:,
5515:^
5504:MR
5502:,
5494:,
5484:24
5482:,
5439:MR
5437:,
5429:,
5415:,
5394:^
5381:,
5356:^
5345:MR
5343:,
5335:,
5325:69
5323:,
5305:^
5294:MR
5292:,
5284:,
5274:55
5272:,
5255:^
5220:^
4473:60
4421:13
3978:A
3698:,
3677:.
3397:20
3357:10
3305:,
2820:2.
2353:1.
1466::
1455:.
1151:.
549:.
415:).
240:,
159:.
96:.
35:,
31:,
6976:a
6857:e
6850:t
6843:v
6823::
6786::
6776::
6746::
6709::
6675::
6641::
6635:1
6599::
6573::
6468::
6410:.
6389::
6343::
6313::
6269::
6214::
6178::
6172:5
6130::
6089:n
6069:k
6049:}
6042:k
6039:n
6032:{
6029:!
6026:k
5988:n
5968:k
5948:}
5941:k
5938:n
5931:{
5928:!
5925:k
5896::
5886::
5848::
5794:n
5762::
5756:1
5666::
5617::
5558::
5548::
5490::
5423::
5417:3
5331::
5280::
5195:)
5192:0
5189:(
5186:W
5166:0
5163:=
5160:)
5157:1
5154:(
5151:W
5131:,
5128:)
5125:1
5119:n
5116:(
5113:W
5107:)
5102:k
5099:n
5094:(
5086:1
5080:n
5075:1
5072:=
5069:k
5061:+
5058:1
5055:=
5052:)
5049:n
5046:(
5043:W
5020:)
5017:n
5014:(
5011:W
4991:n
4971:)
4968:n
4965:(
4962:W
4920:n
4896:n
4865:n
4862:2
4836:n
4833:1
4760:)
4757:x
4754:(
4751:f
4748:=
4745:x
4722:)
4719:y
4716:(
4713:f
4710:=
4707:x
4684:)
4681:x
4678:(
4675:f
4672:=
4669:y
4649:3
4646:=
4643:)
4640:n
4637:(
4634:a
4614:)
4611:x
4608:(
4605:f
4602:=
4599:y
4579:y
4559:x
4539:2
4536:=
4533:n
4512:n
4500:n
4446:2
4442:A
4418:=
4415:)
4412:3
4409:(
4406:a
4380:1
4374:n
4370:A
4341:)
4338:n
4335:(
4332:a
4302:3
4298:A
4271:3
4267:A
4240:.
4235:1
4229:n
4225:)
4221:1
4218:+
4215:n
4212:(
4206:)
4203:n
4200:(
4197:a
4191:!
4188:n
4154:1
4148:n
4144:)
4140:1
4137:+
4134:n
4131:(
4111:n
4090:n
4070:n
4050:n
4030:i
4010:i
3990:i
3960:1
3954:n
3950:2
3929:n
3905:n
3885:n
3861:)
3858:n
3855:(
3852:a
3831:n
3744:!
3741:n
3718:n
3665:k
3645:k
3625:k
3601:k
3575:.
3571:)
3561:(
3556:)
3553:n
3550:(
3547:a
3541:)
3535:+
3532:n
3529:(
3526:a
3504:,
3500:)
3490:(
3485:)
3482:n
3479:(
3476:a
3470:)
3464:+
3461:n
3458:(
3455:a
3434:,
3430:)
3420:(
3415:)
3412:n
3409:(
3406:a
3400:)
3394:+
3391:n
3388:(
3385:a
3364:,
3360:)
3350:(
3345:)
3342:n
3339:(
3336:a
3330:)
3327:4
3324:+
3321:n
3318:(
3315:a
3293:n
3269:1
3266:=
3263:)
3260:0
3257:(
3254:a
3234:)
3231:n
3228:(
3225:a
3204:)
3201:i
3195:n
3192:(
3189:a
3165:)
3160:i
3157:n
3152:(
3128:i
3122:n
3102:i
3082:n
3059:.
3056:)
3053:i
3047:n
3044:(
3041:a
3035:)
3030:i
3027:n
3022:(
3014:n
3009:1
3006:=
3003:i
2995:=
2992:)
2989:n
2986:(
2983:a
2949:2
2946:=
2941:x
2937:e
2906:2
2870:/
2846:5
2843:=
2840:n
2811:=
2805:)
2802:n
2799:(
2796:a
2791:)
2788:1
2782:n
2779:(
2776:a
2772:n
2758:n
2733:)
2730:n
2727:(
2724:a
2704:)
2701:1
2695:n
2692:(
2689:a
2666:n
2642:)
2639:1
2636:(
2633:o
2613:)
2610:1
2607:(
2604:o
2598:1
2570:n
2512:2
2502:1
2496:=
2493:c
2473:,
2468:1
2465:+
2462:n
2458:c
2452:!
2449:n
2443:2
2440:1
2432:)
2429:n
2426:(
2423:a
2403:0
2400:=
2397:k
2347:n
2343:,
2338:)
2335:1
2332:+
2329:n
2326:(
2319:)
2315:k
2312:i
2306:2
2303:+
2300:2
2291:(
2275:=
2272:k
2262:2
2258:!
2255:n
2249:=
2246:)
2243:n
2240:(
2237:a
2210:P
2186:P
2162:I
2140:1
2133:)
2129:P
2123:I
2120:2
2117:(
2093:.
2085:x
2081:e
2074:2
2070:1
2065:=
2059:!
2056:n
2050:n
2046:x
2040:)
2037:n
2034:(
2031:a
2021:0
2018:=
2015:n
1968:k
1964:2
1943:k
1919:n
1895:n
1873:n
1869:A
1848:,
1845:)
1842:2
1839:(
1834:n
1830:A
1826:=
1815:k
1808:n
1795:k
1791:2
1785:1
1779:n
1774:0
1771:=
1768:k
1760:=
1757:)
1754:n
1751:(
1748:a
1728:k
1708:n
1681:k
1678:n
1646:.
1639:m
1635:2
1629:n
1625:m
1612:0
1609:=
1606:m
1596:2
1593:1
1588:=
1583:n
1579:j
1572:)
1567:j
1564:k
1559:(
1551:j
1545:k
1541:)
1537:1
1531:(
1526:k
1521:0
1518:=
1515:j
1505:n
1500:0
1497:=
1494:k
1486:=
1483:)
1480:n
1477:(
1474:a
1443:3
1440:=
1437:n
1417:3
1414:=
1411:k
1391:2
1388:=
1385:k
1365:1
1362:=
1359:k
1339:0
1336:=
1333:k
1305:n
1285:1
1282:+
1279:n
1259:n
1227:k
1221:n
1201:k
1181:n
1139:0
1133:n
1111:2
1107:)
1103:n
1100:(
1097:a
1091:)
1088:1
1085:+
1082:n
1079:(
1076:a
1073:)
1070:1
1064:n
1061:(
1058:a
1033:}
1026:k
1019:n
1012:{
1008:!
1005:k
1000:n
995:0
992:=
989:k
981:=
978:)
975:n
972:(
969:a
945:!
942:k
922:}
915:k
912:n
905:{
885:k
865:k
845:!
842:k
822:k
802:n
782:}
775:k
772:n
765:{
682:n
654:n
634:1
631:+
628:i
608:i
588:1
585:+
582:n
537:1
531:n
505:1
499:n
495:2
474:n
464:f
462:,
460:e
458:,
456:d
452:c
448:b
446:,
444:a
430:n
390:)
387:n
384:(
381:a
361:0
358:=
355:n
342:a
338:b
334:b
330:a
326:b
322:a
308:n
288:)
285:n
282:(
279:a
259:n
246:f
242:e
238:d
234:c
230:b
226:a
222:f
220:,
218:e
216:,
214:d
210:c
206:b
204:,
202:a
76:n
40:}
37:c
33:b
29:a
27:{
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.