354:
57:
1683:
maximum principle says that the maximum of the function is to be found on the boundary, but may re-occur in the interior as well. Other, even weaker maximum principles exist which merely bound a function in terms of its maximum on the boundary.
379:
The step-wise continuation technique may, however, come up against difficulties. These may have an essentially topological nature, leading to inconsistencies (defining more than one value). They may alternatively have to do with the presence of
2207:
2343:
1060:
1930:
828:
174:
133:
1861:
1042:
587:
1460:
1139:
625:
995:
953:
1300:
548:
1789:
1384:
1240:
1178:
890:
723:
1438:
1411:
1354:
1327:
1267:
1205:
1117:
1090:
917:
855:
777:
249:(i.e., not just the magnitude of the angle). Conformal maps preserve both angles and the shapes of infinitesimally small figures, but not necessarily their size or
243:
212:
689:
669:
645:
528:
1059:
449:
may be defined between them. Riemann surfaces are nowadays considered the natural setting for studying the global behavior of these functions, especially
1664:
739:. It is a remarkable fact, fundamental to the theory of univalent functions, that univalence is essentially preserved under uniform convergence.
1660:
388:
is rather different, since singularities then cannot be isolated points, and its investigation was a major reason for the development of
2111:
1679:
maximum principle says that if a function achieves its maximum in the interior of the domain, the function is uniformly a constant. The
2433:
2384:
2344:
Berichte über die
Verhandlungen der Königlich Sächsischen Gesellschaft der Wissenschaften zu Leipzig. Mathematisch-Physische Classe
1672:
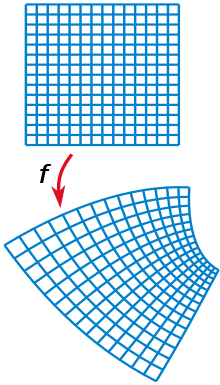
1656:
2093:
with vertices at the branch and ramification points, respectively, and use these to compute the Euler characteristics. Then
1048:
2452:
1503:, which it helps to prove. It is however one of the simplest results capturing the rigidity of holomorphic functions.
372:. Analytic continuation often succeeds in defining further values of a function, for example in a new region where an
319:
473:
Topics in this area include "Maximum principle; Schwarz's lemma, Lindelöf principle, analogues and generalizations".
1723:
782:
381:
1876:
283:, is a homeomorphism between plane domains which to first order takes small circles to small ellipses of bounded
385:
303:
246:
138:
1948:
105:
1693:
1500:
1481:
1142:
31:
262:
77:
1956:
1489:
450:
400:
Topics in this area include
Riemann surfaces for algebraic functions and zeros for algebraic functions.
353:
2014: > 1. An equivalent way of thinking about this is that there exists a small neighborhood
1711:
1539:
488:
446:
365:
85:
1828:
1000:
560:
2338:
1799:
648:
89:
1443:
1122:
595:
2048:
1727:
1715:
504:
482:
458:
2105:
different from zero, but fewer than expected vertices. Therefore, we find a "corrected" formula
1730:, in this case. It is a prototype result for many others, and is often applied in the theory of
958:
2429:
2395:
2380:
2310:
1979:
1652:
1646:
922:
462:
369:
27:
1959:. What the Riemann–Hurwitz formula does is to add in a correction to allow for ramification (
1272:
533:
2410:
2352:
2326:
2318:
2300:
2291:
1764:
1703:
1485:
1359:
1210:
1148:
860:
698:
423:
419:
389:
268:
1416:
1389:
1332:
1305:
1245:
1183:
1095:
1068:
895:
833:
755:
221:
190:
2356:
2330:
2322:
1975:
1735:
1731:
1555:
551:
409:
373:
1532:
674:
654:
630:
513:
430:: locally near every point they look like patches of the complex plane, but the global
2446:
1707:
1471:
1386:, and consequently the composition, is analytic, we then have a conformal mapping of
496:
427:
342:
307:
93:
68:
maps pairs of lines intersecting at 90° to pairs of curves still intersecting at 90°.
51:
2286:
1819:
1807:
857:
having at least two boundary points. Then there exists a unique analytic function
42:
The following are some of the most important topics in geometric function theory:
492:
454:
1747:
726:
692:
2314:
1528:
1499:
to itself. The lemma is less celebrated than stronger theorems, such as the
1496:
376:
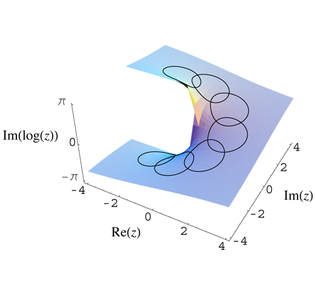
representation in terms of which it is initially defined becomes divergent.
250:
1440:, proving "any two simply connected regions different from the whole plane
56:
2415:
1493:
431:
311:
23:
2341:(1928), "Über einige Extremalprobleme der konformen Abbildung. I, II.",
2264:
MSC classification for 30CXX, Geometric
Function Theory, retrieved from
2305:
1668:
1051:
demonstrates the existence of a mapping function, it does not actually
284:
2265:
435:
330:
at every point maps circles to ellipses with eccentricity bounded by
16:
Study of space and shapes locally given by a convergent power series
2202:{\displaystyle \chi (S')=N\cdot \chi (S)-\sum _{P\in S'}(e_{P}-1)}
439:
352:
215:
81:
55:
1675:
is to be found on the boundary of that domain. Specifically, the
426:. Riemann surfaces can be thought of as deformed versions of the
1955:, as we are entitled to do since the Euler characteristic is a
2403:
International
Journal of Mathematics and Mathematical Sciences
60:
A rectangular grid (top) and its image under a conformal map
2377:
Geometric
Function Theory: Explorations in Complex Analysis
396:
Geometric properties of polynomials and algebraic functions
357:
Analytic continuation of natural logarithm (imaginary part)
2223:= 1, so this is quite safe). This formula is known as the
434:
can be quite different. For example, they can look like a
2426:
Conformal
Invariants: Topics in Geometric Function Theory
2114:
1879:
1831:
1767:
1446:
1419:
1392:
1362:
1335:
1308:
1275:
1248:
1213:
1186:
1151:
1125:
1098:
1071:
1003:
961:
925:
898:
863:
836:
785:
758:
701:
677:
657:
633:
598:
563:
536:
516:
224:
193:
141:
108:
84:
locally. In the most common case the function has a
2394:Bulboacă, T.; Cho, N. E.; Kanas, S. A. R. (2012).
2201:
1924:
1855:
1783:
1454:
1432:
1405:
1378:
1348:
1321:
1294:
1261:
1234:
1199:
1172:
1133:
1111:
1084:
1036:
989:
947:
911:
884:
849:
822:
771:
717:
683:
663:
639:
619:
581:
542:
522:
237:
206:
168:
127:
1994:′ if there exist analytic coordinates near
1947:′ — at least if we use a fine enough
2367:, 1922 (4th ed., appendix by H. Röhrl, vol. 3,
2289:(1935), "Zur Theorie der Überlagerungsflächen",
2251:, 1922 (4th ed., appendix by H. Röhrl, vol. 3,
2365:Vorlesunger über allgemeine Funcktionen Theorie
2249:Vorlesunger über allgemeine Funcktionen Theorie
1119:as two simply connected regions different from
2396:"New Trends in Geometric Function Theory 2011"
2062:. In calculating the Euler characteristic of
2369:Grundlehren der mathematischen Wissenschaften
2253:Grundlehren der mathematischen Wissenschaften
823:{\displaystyle D_{1}(D_{1}\neq \mathbb {C} )}
30:. A fundamental result in the theory is the
8:
1925:{\displaystyle \chi (S')=N\cdot \chi (S).\,}
1462:can be mapped conformally onto each other."
445:The main point of Riemann surfaces is that
169:{\displaystyle U,V\subset \mathbb {C} ^{n}}
1667:types. Roughly speaking, it says that the
1055:this function. An example is given below.
725:is also holomorphic. More, one has by the
2414:
2304:
2184:
2160:
2113:
1921:
1878:
1852:
1830:
1780:
1766:
1448:
1447:
1445:
1424:
1418:
1397:
1391:
1367:
1361:
1340:
1334:
1313:
1307:
1280:
1274:
1253:
1247:
1212:
1191:
1185:
1150:
1127:
1126:
1124:
1103:
1097:
1076:
1070:
1019:
1002:
972:
960:
934:
926:
924:
903:
897:
862:
841:
835:
813:
812:
803:
790:
784:
763:
757:
706:
700:
676:
656:
632:
597:
562:
535:
515:
229:
223:
198:
192:
160:
156:
155:
140:
107:
2085:)). Now let us choose triangulations of
779:be a point in a simply-connected region
735:( this is German for plain, simple) and
276:
214:if it preserves oriented angles between
2240:
280:
128:{\displaystyle f:U\rightarrow V\qquad }
2277:MSC80 in the MSC classification system
2081:) (that is, in the inverse image of π(
2030:, but the image of any other point in
1655:is a property of solutions to certain
326:-quasiconformal if the derivative of
7:
1814:, 1, 0, 0, ... . In the case of an (
1722:of the other. It therefore connects
1710:, describes the relationship of the
1207:onto the unit disk and existence of
919:bijectively into the open unit disk
2266:http://www.ams.org/msc/msc2010.html
477:Univalent and multivalent functions
418:, first studied by and named after
38:Topics in geometric function theory
731:Alternate terms in common use are
614:
592:is a univalent function such that
576:
537:
442:or several sheets glued together.
14:
1866:that is surjective and of degree
1935:That is because each simplex of
1058:
2073: − 1 copies of
2002:) such that π takes the form π(
554:sets in the complex plane, and
124:
2196:
2177:
2150:
2144:
2129:
2118:
2066:′ we notice the loss of
2026:) has exactly one preimage in
1915:
1909:
1894:
1883:
1856:{\displaystyle \pi :S'\to S\,}
1846:
1657:partial differential equations
1229:
1223:
1167:
1161:
1065:In the above figure, consider
1037:{\displaystyle f'(z_{0})>0}
1025:
1012:
978:
965:
935:
927:
879:
873:
817:
796:
608:
602:
582:{\displaystyle f:G\to \Omega }
573:
118:
1:
2097:will have the same number of
1939:should be covered by exactly
1870:, we should have the formula
364:is a technique to extend the
1455:{\displaystyle \mathbb {C} }
1134:{\displaystyle \mathbb {C} }
620:{\displaystyle f(G)=\Omega }
1982:. The map π is said to be
1754:the Euler characteristic χ(
1636:| (necessarily) equal to 1.
1302:is a one-to-one mapping of
341:is 0, then the function is
320:continuously differentiable
2469:
2428:. AMS Chelsea Publishing.
1734:(which is its origin) and
1691:
1644:
1469:
1145:provides the existence of
990:{\displaystyle f(z_{0})=0}
651:), then the derivative of
480:
407:
382:mathematical singularities
260:
64:(bottom). It is seen that
49:
1269:onto the unit disk. Thus
1049:Riemann's mapping theorem
386:several complex variables
20:Geometric function theory
1978:, and that the map π is
948:{\displaystyle |w|<1}
2375:Krantz, Steven (2006).
2225:Riemann–Hurwitz formula
2212:(all but finitely many
2101:-dimensional faces for
1700:Riemann–Hurwitz formula
1694:Riemann-Hurwitz formula
1688:Riemann-Hurwitz formula
1501:Riemann mapping theorem
1482:Hermann Amandus Schwarz
1295:{\displaystyle g^{-1}f}
1143:Riemann mapping theorem
748:Riemann mapping theorem
543:{\displaystyle \Omega }
422:, is a one-dimensional
32:Riemann mapping theorem
2424:Ahlfors, Lars (2010).
2268:on September 16, 2014.
2203:
1961:sheets coming together
1926:
1857:
1785:
1784:{\displaystyle 2-2g\,}
1638:
1527:| < 1} be the open
1456:
1434:
1407:
1380:
1379:{\displaystyle g^{-1}}
1356:. If we can show that
1350:
1323:
1296:
1263:
1236:
1235:{\displaystyle w=g(z)}
1201:
1174:
1173:{\displaystyle w=f(z)}
1135:
1113:
1086:
1038:
991:
949:
913:
886:
885:{\displaystyle w=f(z)}
851:
824:
773:
719:
718:{\displaystyle f^{-1}}
685:
665:
641:
621:
583:
544:
524:
510:One can prove that if
451:multi-valued functions
358:
273:quasiconformal mapping
263:Quasiconformal mapping
245:with respect to their
239:
208:
170:
129:
99:More formally, a map,
69:
2204:
1957:topological invariant
1927:
1858:
1786:
1712:Euler characteristics
1510:
1490:holomorphic functions
1465:
1457:
1435:
1433:{\displaystyle D_{2}}
1408:
1406:{\displaystyle D_{1}}
1381:
1351:
1349:{\displaystyle D_{2}}
1324:
1322:{\displaystyle D_{1}}
1297:
1264:
1262:{\displaystyle D_{2}}
1237:
1202:
1200:{\displaystyle D_{1}}
1175:
1136:
1114:
1112:{\displaystyle D_{2}}
1087:
1085:{\displaystyle D_{1}}
1039:
992:
950:
914:
912:{\displaystyle D_{1}}
887:
852:
850:{\displaystyle D_{1}}
825:
774:
772:{\displaystyle z_{0}}
720:
686:
666:
642:
622:
584:
545:
525:
447:holomorphic functions
362:Analytic continuation
356:
349:Analytic continuation
240:
238:{\displaystyle u_{0}}
209:
207:{\displaystyle u_{0}}
171:
130:
59:
2351:: 367–376, 497–502,
2112:
2053:and also denoted by
1877:
1829:
1765:
1604:| for some non-zero
1444:
1417:
1390:
1360:
1333:
1306:
1273:
1246:
1211:
1184:
1149:
1123:
1096:
1069:
1001:
959:
923:
896:
861:
834:
783:
756:
699:
675:
655:
631:
596:
561:
534:
514:
489:holomorphic function
222:
191:
139:
106:
2416:10.1155/2012/976374
1671:of a function in a
459:algebraic functions
257:Quasiconformal maps
2453:Analytic functions
2371:. Springer, 1964.)
2306:10.1007/BF02420945
2255:. Springer, 1964.)
2199:
2176:
2049:ramification index
1922:
1853:
1781:
1728:algebraic topology
1452:
1430:
1403:
1376:
1346:
1319:
1292:
1259:
1232:
1197:
1170:
1131:
1109:
1082:
1034:
987:
945:
909:
882:
847:
820:
769:
743:Important theorems
715:
695:, and its inverse
681:
661:
637:
617:
579:
540:
520:
483:Univalent function
359:
235:
204:
166:
125:
70:
28:analytic functions
2363:Hurwitz-Courant,
2339:Grötzsch, Herbert
2247:Hurwitz-Courant,
2229:Hurwitz's theorem
2156:
1804:number of handles
1720:ramified covering
1653:maximum principle
1647:Maximum principle
1641:Maximum principle
1484:, is a result in
684:{\displaystyle f}
664:{\displaystyle f}
640:{\displaystyle f}
523:{\displaystyle G}
469:Extremal problems
370:analytic function
314:in the plane. If
290:Intuitively, let
2460:
2439:
2420:
2418:
2400:
2390:
2359:
2333:
2308:
2292:Acta Mathematica
2278:
2275:
2269:
2262:
2256:
2245:
2208:
2206:
2205:
2200:
2189:
2188:
2175:
2174:
2128:
1980:complex analytic
1976:Riemann surfaces
1966:Now assume that
1931:
1929:
1928:
1923:
1893:
1862:
1860:
1859:
1854:
1845:
1790:
1788:
1787:
1782:
1736:algebraic curves
1732:Riemann surfaces
1704:Bernhard Riemann
1538:centered at the
1486:complex analysis
1461:
1459:
1458:
1453:
1451:
1439:
1437:
1436:
1431:
1429:
1428:
1412:
1410:
1409:
1404:
1402:
1401:
1385:
1383:
1382:
1377:
1375:
1374:
1355:
1353:
1352:
1347:
1345:
1344:
1328:
1326:
1325:
1320:
1318:
1317:
1301:
1299:
1298:
1293:
1288:
1287:
1268:
1266:
1265:
1260:
1258:
1257:
1241:
1239:
1238:
1233:
1206:
1204:
1203:
1198:
1196:
1195:
1179:
1177:
1176:
1171:
1140:
1138:
1137:
1132:
1130:
1118:
1116:
1115:
1110:
1108:
1107:
1091:
1089:
1088:
1083:
1081:
1080:
1062:
1043:
1041:
1040:
1035:
1024:
1023:
1011:
996:
994:
993:
988:
977:
976:
954:
952:
951:
946:
938:
930:
918:
916:
915:
910:
908:
907:
891:
889:
888:
883:
856:
854:
853:
848:
846:
845:
829:
827:
826:
821:
816:
808:
807:
795:
794:
778:
776:
775:
770:
768:
767:
724:
722:
721:
716:
714:
713:
690:
688:
687:
682:
670:
668:
667:
662:
646:
644:
643:
638:
626:
624:
623:
618:
588:
586:
585:
580:
549:
547:
546:
541:
529:
527:
526:
521:
424:complex manifold
420:Bernhard Riemann
390:sheaf cohomology
275:, introduced by
269:complex analysis
267:In mathematical
244:
242:
241:
236:
234:
233:
213:
211:
210:
205:
203:
202:
185:angle-preserving
175:
173:
172:
167:
165:
164:
159:
134:
132:
131:
126:
80:which preserves
22:is the study of
2468:
2467:
2463:
2462:
2461:
2459:
2458:
2457:
2443:
2442:
2436:
2423:
2398:
2393:
2387:
2374:
2337:
2285:
2282:
2281:
2276:
2272:
2263:
2259:
2246:
2242:
2237:
2221:
2180:
2167:
2121:
2110:
2109:
2071:
2061:
1886:
1875:
1874:
1838:
1827:
1826:
1763:
1762:
1744:
1696:
1690:
1649:
1643:
1612:(0)| = 1, then
1592:Moreover, if |
1556:holomorphic map
1509:
1474:
1468:
1466:Schwarz's Lemma
1442:
1441:
1420:
1415:
1414:
1393:
1388:
1387:
1363:
1358:
1357:
1336:
1331:
1330:
1309:
1304:
1303:
1276:
1271:
1270:
1249:
1244:
1243:
1209:
1208:
1187:
1182:
1181:
1147:
1146:
1121:
1120:
1099:
1094:
1093:
1072:
1067:
1066:
1015:
1004:
999:
998:
968:
957:
956:
921:
920:
899:
894:
893:
859:
858:
837:
832:
831:
799:
786:
781:
780:
759:
754:
753:
750:
745:
702:
697:
696:
673:
672:
671:is never zero,
653:
652:
629:
628:
594:
593:
559:
558:
532:
531:
512:
511:
485:
479:
471:
416:Riemann surface
412:
410:Riemann surface
406:
404:Riemann surface
398:
374:infinite series
351:
277:Grötzsch (1928)
265:
259:
225:
220:
219:
194:
189:
188:
154:
137:
136:
104:
103:
54:
48:
40:
17:
12:
11:
5:
2466:
2464:
2456:
2455:
2445:
2444:
2441:
2440:
2435:978-0821852705
2434:
2421:
2391:
2385:
2372:
2361:
2335:
2299:(1): 157–194,
2280:
2279:
2270:
2257:
2239:
2238:
2236:
2233:
2219:
2210:
2209:
2198:
2195:
2192:
2187:
2183:
2179:
2173:
2170:
2166:
2163:
2159:
2155:
2152:
2149:
2146:
2143:
2140:
2137:
2134:
2131:
2127:
2124:
2120:
2117:
2069:
2057:
2046:is called the
2042:. The number
1933:
1932:
1920:
1917:
1914:
1911:
1908:
1905:
1902:
1899:
1896:
1892:
1889:
1885:
1882:
1864:
1863:
1851:
1848:
1844:
1841:
1837:
1834:
1792:
1791:
1779:
1776:
1773:
1770:
1743:
1740:
1718:when one is a
1702:, named after
1692:Main article:
1689:
1686:
1645:Main article:
1642:
1639:
1513:Schwarz Lemma.
1508:
1505:
1480:, named after
1470:Main article:
1467:
1464:
1450:
1427:
1423:
1400:
1396:
1373:
1370:
1366:
1343:
1339:
1316:
1312:
1291:
1286:
1283:
1279:
1256:
1252:
1231:
1228:
1225:
1222:
1219:
1216:
1194:
1190:
1169:
1166:
1163:
1160:
1157:
1154:
1129:
1106:
1102:
1079:
1075:
1033:
1030:
1027:
1022:
1018:
1014:
1010:
1007:
986:
983:
980:
975:
971:
967:
964:
944:
941:
937:
933:
929:
906:
902:
881:
878:
875:
872:
869:
866:
844:
840:
819:
815:
811:
806:
802:
798:
793:
789:
766:
762:
749:
746:
744:
741:
712:
709:
705:
680:
660:
636:
616:
613:
610:
607:
604:
601:
590:
589:
578:
575:
572:
569:
566:
539:
519:
481:Main article:
478:
475:
470:
467:
408:Main article:
405:
402:
397:
394:
384:. The case of
350:
347:
281:Ahlfors (1935)
261:Main article:
258:
255:
232:
228:
201:
197:
177:
176:
163:
158:
153:
150:
147:
144:
123:
120:
117:
114:
111:
50:Main article:
47:
46:Conformal maps
44:
39:
36:
26:properties of
15:
13:
10:
9:
6:
4:
3:
2:
2465:
2454:
2451:
2450:
2448:
2437:
2431:
2427:
2422:
2417:
2412:
2408:
2404:
2397:
2392:
2388:
2386:0-8176-4339-7
2382:
2378:
2373:
2370:
2366:
2362:
2358:
2354:
2350:
2347:(in German),
2346:
2345:
2340:
2336:
2332:
2328:
2324:
2320:
2316:
2312:
2307:
2302:
2298:
2295:(in German),
2294:
2293:
2288:
2287:Ahlfors, Lars
2284:
2283:
2274:
2271:
2267:
2261:
2258:
2254:
2250:
2244:
2241:
2234:
2232:
2230:
2226:
2222:
2215:
2193:
2190:
2185:
2181:
2171:
2168:
2164:
2161:
2157:
2153:
2147:
2141:
2138:
2135:
2132:
2125:
2122:
2115:
2108:
2107:
2106:
2104:
2100:
2096:
2092:
2088:
2084:
2080:
2076:
2072:
2065:
2060:
2056:
2052:
2050:
2045:
2041:
2038:preimages in
2037:
2033:
2029:
2025:
2021:
2017:
2013:
2009:
2005:
2001:
1997:
1993:
1989:
1985:
1981:
1977:
1973:
1969:
1964:
1962:
1958:
1954:
1950:
1949:triangulation
1946:
1942:
1938:
1918:
1912:
1906:
1903:
1900:
1897:
1890:
1887:
1880:
1873:
1872:
1871:
1869:
1849:
1842:
1839:
1835:
1832:
1825:
1824:
1823:
1821:
1817:
1813:
1809:
1808:Betti numbers
1806:), since the
1805:
1801:
1797:
1777:
1774:
1771:
1768:
1761:
1760:
1759:
1757:
1753:
1749:
1741:
1739:
1737:
1733:
1729:
1725:
1721:
1717:
1713:
1709:
1708:Adolf Hurwitz
1705:
1701:
1695:
1687:
1685:
1682:
1678:
1674:
1670:
1666:
1662:
1658:
1654:
1648:
1640:
1637:
1635:
1631:
1627:
1623:
1619:
1615:
1611:
1607:
1603:
1599:
1595:
1590:
1588:
1584:
1580:
1576:
1572:
1568:
1563:
1561:
1557:
1553:
1549:
1545:
1541:
1537:
1534:
1533:complex plane
1530:
1526:
1522:
1518:
1514:
1506:
1504:
1502:
1498:
1495:
1491:
1487:
1483:
1479:
1478:Schwarz lemma
1473:
1472:Schwarz lemma
1463:
1425:
1421:
1398:
1394:
1371:
1368:
1364:
1341:
1337:
1314:
1310:
1289:
1284:
1281:
1277:
1254:
1250:
1226:
1220:
1217:
1214:
1192:
1188:
1164:
1158:
1155:
1152:
1144:
1104:
1100:
1077:
1073:
1063:
1061:
1056:
1054:
1050:
1045:
1031:
1028:
1020:
1016:
1008:
1005:
984:
981:
973:
969:
962:
942:
939:
931:
904:
900:
876:
870:
867:
864:
842:
838:
809:
804:
800:
791:
787:
764:
760:
747:
742:
740:
738:
734:
729:
728:
710:
707:
703:
694:
678:
658:
650:
634:
611:
605:
599:
570:
567:
564:
557:
556:
555:
553:
550:are two open
517:
508:
506:
502:
498:
497:complex plane
494:
490:
484:
476:
474:
468:
466:
464:
460:
456:
452:
448:
443:
441:
437:
433:
429:
428:complex plane
425:
421:
417:
411:
403:
401:
395:
393:
391:
387:
383:
377:
375:
371:
367:
363:
355:
348:
346:
344:
340:
335:
333:
329:
325:
322:, then it is
321:
317:
313:
309:
308:homeomorphism
305:
301:
298: →
297:
293:
288:
286:
282:
279:and named by
278:
274:
270:
264:
256:
254:
252:
248:
230:
226:
217:
199:
195:
187:) at a point
186:
182:
161:
151:
148:
145:
142:
121:
115:
112:
109:
102:
101:
100:
97:
95:
94:complex plane
91:
87:
83:
79:
75:
74:conformal map
67:
63:
58:
53:
52:Conformal map
45:
43:
37:
35:
33:
29:
25:
21:
2425:
2406:
2402:
2379:. Springer.
2376:
2368:
2364:
2348:
2342:
2296:
2290:
2273:
2260:
2252:
2248:
2243:
2228:
2227:and also as
2224:
2217:
2213:
2211:
2102:
2098:
2094:
2090:
2086:
2082:
2078:
2074:
2067:
2063:
2058:
2054:
2047:
2043:
2039:
2035:
2034:has exactly
2031:
2027:
2023:
2022:such that π(
2019:
2015:
2011:
2007:
2003:
1999:
1995:
1991:
1987:
1983:
1971:
1967:
1965:
1960:
1952:
1944:
1940:
1936:
1934:
1867:
1865:
1822:of surfaces
1820:covering map
1815:
1811:
1803:
1795:
1793:
1755:
1751:
1745:
1724:ramification
1719:
1699:
1697:
1680:
1676:
1650:
1633:
1629:
1625:
1621:
1617:
1613:
1609:
1605:
1601:
1597:
1593:
1591:
1586:
1582:
1578:
1574:
1570:
1566:
1564:
1559:
1551:
1547:
1543:
1535:
1524:
1520:
1516:
1512:
1511:
1477:
1475:
1064:
1057:
1052:
1046:
751:
736:
732:
730:
591:
509:
500:
486:
472:
453:such as the
444:
415:
413:
399:
378:
361:
360:
338:
336:
331:
327:
323:
315:
306:-preserving
299:
295:
291:
289:
285:eccentricity
272:
266:
184:
180:
178:
98:
73:
71:
65:
61:
41:
19:
18:
1986:at a point
493:open subset
455:square root
368:of a given
304:orientation
247:orientation
2357:54.0378.01
2331:0012.17204
2323:61.0365.03
2235:References
1816:unramified
1748:orientable
1589:(0)| ≤ 1.
1577:| for all
1558:such that
955:such that
727:chain rule
693:invertible
649:surjective
627:(that is,
499:is called
457:and other
179:is called
2315:0001-5962
2191:−
2165:∈
2158:∑
2154:−
2142:χ
2139:⋅
2116:χ
1907:χ
1904:⋅
1881:χ
1847:→
1833:π
1772:−
1742:Statement
1665:parabolic
1659:, of the
1624:for some
1562:(0) = 0.
1529:unit disk
1523: : |
1507:Statement
1497:unit disk
1492:from the
1369:−
1282:−
1047:Although
810:≠
708:−
615:Ω
577:Ω
574:→
552:connected
538:Ω
505:injective
503:if it is
501:univalent
463:logarithm
461:, or the
343:conformal
312:open sets
251:curvature
181:conformal
152:⊂
119:→
24:geometric
2447:Category
2172:′
2126:′
2095:S′
2091:S′
2077:above π(
1984:ramified
1972:S′
1891:′
1843:′
1810:are 1, 2
1750:surface
1716:surfaces
1661:elliptic
1546: :
1542:and let
1242:mapping
1180:mapping
1009:′
892:mapping
733:schlicht
432:topology
310:between
302:′ be an
294: :
218:through
78:function
2409:: 1–2.
1798:is the
1746:For an
1714:of two
1669:maximum
1608:or if |
1565:Then, |
1531:in the
1053:exhibit
495:of the
92:in the
2432:
2383:
2355:
2329:
2321:
2313:
2010:, and
1998:and π(
1794:where
1677:strong
1673:domain
1632:with |
1600:)| = |
1573:)| ≤ |
1540:origin
1488:about
1141:. The
737:simple
491:on an
436:sphere
366:domain
216:curves
86:domain
82:angles
2399:(PDF)
2216:have
1802:(the
1800:genus
1758:) is
1726:with
1585:and |
1554:be a
1413:onto
1329:onto
440:torus
438:or a
135:with
90:range
76:is a
2430:ISBN
2407:2012
2381:ISBN
2311:ISSN
2089:and
2051:at P
2006:) =
1974:are
1970:and
1706:and
1698:the
1681:weak
1663:and
1651:The
1620:) =
1515:Let
1494:open
1476:The
1092:and
1029:>
997:and
940:<
830:and
752:Let
530:and
271:, a
183:(or
88:and
2411:doi
2353:JFM
2327:Zbl
2319:JFM
2301:doi
2018:of
1990:in
1963:).
1951:of
1943:in
1628:in
1581:in
1519:= {
691:is
647:is
337:If
318:is
2449::
2405:.
2401:.
2349:80
2325:,
2317:,
2309:,
2297:65
2231:.
1818:)
1738:.
1622:az
1610:f′
1587:f′
1550:→
1044:.
507:.
487:A
465:.
414:A
392:.
345:.
334:.
287:.
253:.
96:.
72:A
34:.
2438:.
2419:.
2413::
2389:.
2360:.
2334:.
2303::
2220:P
2218:e
2214:P
2197:)
2194:1
2186:P
2182:e
2178:(
2169:S
2162:P
2151:)
2148:S
2145:(
2136:N
2133:=
2130:)
2123:S
2119:(
2103:d
2099:d
2087:S
2083:P
2079:P
2075:P
2070:P
2068:e
2064:S
2059:P
2055:e
2044:n
2040:U
2036:n
2032:U
2028:U
2024:P
2020:P
2016:U
2012:n
2008:z
2004:z
2000:P
1996:P
1992:S
1988:P
1968:S
1953:S
1945:S
1941:N
1937:S
1919:.
1916:)
1913:S
1910:(
1901:N
1898:=
1895:)
1888:S
1884:(
1868:N
1850:S
1840:S
1836::
1812:g
1796:g
1778:g
1775:2
1769:2
1756:S
1752:S
1634:a
1630:C
1626:a
1618:z
1616:(
1614:f
1606:z
1602:z
1598:z
1596:(
1594:f
1583:D
1579:z
1575:z
1571:z
1569:(
1567:f
1560:f
1552:D
1548:D
1544:f
1536:C
1525:z
1521:z
1517:D
1449:C
1426:2
1422:D
1399:1
1395:D
1372:1
1365:g
1342:2
1338:D
1315:1
1311:D
1290:f
1285:1
1278:g
1255:2
1251:D
1230:)
1227:z
1224:(
1221:g
1218:=
1215:w
1193:1
1189:D
1168:)
1165:z
1162:(
1159:f
1156:=
1153:w
1128:C
1105:2
1101:D
1078:1
1074:D
1032:0
1026:)
1021:0
1017:z
1013:(
1006:f
985:0
982:=
979:)
974:0
970:z
966:(
963:f
943:1
936:|
932:w
928:|
905:1
901:D
880:)
877:z
874:(
871:f
868:=
865:w
843:1
839:D
818:)
814:C
805:1
801:D
797:(
792:1
788:D
765:0
761:z
711:1
704:f
679:f
659:f
635:f
612:=
609:)
606:G
603:(
600:f
571:G
568::
565:f
518:G
339:K
332:K
328:f
324:K
316:f
300:D
296:D
292:f
231:0
227:u
200:0
196:u
162:n
157:C
149:V
146:,
143:U
122:V
116:U
113::
110:f
66:f
62:f
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.