3526:
1105:
1062:
1793:
1691:
80:
50:
1576:
1342:
1096:
1053:
1660:
1543:
1426:
1309:
36:
66:
1225:
3484:
2680:
1459:
2891:
2914:
1952:
1239:
2907:
2884:
2565:
1932:
1912:
1590:
1356:
1087:
1044:
3556:
3070:
3046:
2025:
2005:
1583:
1473:
3517:
3288:
3274:
1651:
1417:
1349:
3332:
3318:
3244:
3230:
3065:
3041:
2921:
2898:
3283:
3269:
3022:
3017:
3327:
3313:
3260:
3255:
1985:
3239:
3225:
3304:
3299:
1534:
1232:
1879:
3216:
3211:
1466:
1300:
1863:
1839:
3144:
3120:
2673:
2558:
2330:
2308:
2218:
2207:
2169:
2158:
1823:
3139:
3115:
2297:
2286:
2196:
2185:
2147:
2133:
3601:
3096:
3091:
2666:
2551:
2319:
2275:
2117:
2106:
3576:
3406:
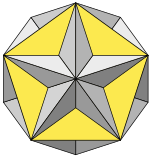
the regular convex dodecahedron, for the first time treating it as a surface rather than a solid. He noticed that by extending the edges or faces of the convex dodecahedron until they met again, he could obtain star pentagons. Further, he recognized that these star pentagons are also regular. In this
498:
A Kepler–Poinsot polyhedron covers its circumscribed sphere more than once, with the centers of faces acting as winding points in the figures which have pentagrammic faces, and the vertices in the others. Because of this, they are not necessarily topologically equivalent to the sphere as
Platonic
481:
which touch at five points around the pentagon. We could treat these triangles as 60 separate faces to obtain a new, irregular polyhedron which looks outwardly identical. Each edge would now be divided into three shorter edges (of two different kinds), and the 20 false vertices would become true
461:
In all cases, two faces can intersect along a line that is not an edge of either face, so that part of each face passes through the interior of the figure. Such lines of intersection are not part of the polyhedral structure and are sometimes called false edges. Likewise where three such lines
3407:
way he constructed the two stellated dodecahedra. Each has the central convex region of each face "hidden" within the interior, with only the triangular arms visible. Kepler's final step was to recognize that these polyhedra fit the definition of regularity, even though they were not
3422:
rediscovered Kepler's figures, by assembling star pentagons around each vertex. He also assembled convex polygons around star vertices to discover two more regular stars, the great icosahedron and great dodecahedron. Some people call these two the
3772:"These figures are so closely related the one to the dodecahedron the other to the icosahedron that the latter two figures, particularly the dodecahedron, seem somehow truncated or maimed when compared to the figures with spikes." (
413:
340:
222:
2543:
2515:
1070:
1113:
555:
does not always hold. Schläfli held that all polyhedra must have χ = 2, and he rejected the small stellated dodecahedron and great dodecahedron as proper polyhedra. This view was never widely held.
2773:
2727:
2658:
2612:
1027:
980:
933:
884:
837:
790:
3787:"A small stellated dodecahedron can be constructed by cumulation of a dodecahedron, i.e., building twelve pentagonal pyramids and attaching them to the faces of the original dodecahedron."
3346:
Most, if not all, of the Kepler-Poinsot polyhedra were known of in some form or other before Kepler. A small stellated dodecahedron appears in a marble tarsia (inlay panel) on the floor of
3152:
3128:
3078:
3054:
268:
2454:
3104:
3030:
693:
462:
intersect at a point that is not a corner of any face, these points are false vertices. The images below show spheres at the true vertices, and blue rods along the true edges.
4191:
550:
726:. Two relationships described in the article below are also easily seen in the images: That the violet edges are the same, and that the green faces lie in the same planes.
2415:
622:
595:
482:
ones, so that we have a total of 32 vertices (again of two kinds). The hidden inner pentagons are no longer part of the polyhedral surface, and can disappear. Now
224:
times the original icosahedron edge length. The small stellated dodecahedron, great dodecahedron, and great stellated dodecahedron edge lengths are respectively
3892:
3819:"Another way to construct a great stellated dodecahedron via cumulation is to make 20 triangular pyramids and attach them to the sides of an icosahedron."
3525:
3183:
2256:
The great stellated dodecahedron shares its vertices with the dodecahedron. The other three Kepler–Poinsot polyhedra share theirs with the icosahedron.
4184:
345:
272:
154:
4291:
2842:
This is just a help to visualize the shape of these solids, and not actually a claim that the edge intersections (false vertices) are vertices.
4505:
4080:
3990:
3973:
3876:
2521:
2493:
1792:
1690:
2245:
1766:
changes pentagonal faces into pentagrams. (In this sense stellation is a unique operation, and not to be confused with the more general
1104:
1061:
4177:
3608:
Regular star polyhedra first appear in
Renaissance art. A small stellated dodecahedron is depicted in a marble tarsia on the floor of
4409:
4394:
4379:
4296:
4096:
4054:
4424:
4399:
4384:
2949:
resemble the icosahedron and its dual in that they have faces and vertices on the 3-fold (yellow) and 5-fold (red) symmetry axes.
1630:
1483:
1396:
1249:
4419:
4414:
3543:
3363:
1610:
1600:
1575:
1513:
1493:
1376:
1366:
1341:
1279:
1259:
79:
49:
1620:
1503:
1386:
1269:
4374:
3981:
3852:
1625:
1508:
1391:
1274:
1615:
1605:
1498:
1488:
1381:
1371:
1264:
1254:
1095:
1052:
486:
holds: 60 − 90 + 32 = 2. However, this polyhedron is no longer the one described by the
2733:
2687:
2618:
2572:
4404:
3985:, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995,
4515:
4341:
4331:
4271:
4261:
4231:
4221:
3538:
3483:
3469:
3388:
3376:
3194:
3190:
3006:
2995:
2956:
2946:
2382:
2378:
2334:
2312:
2222:
2211:
2173:
2162:
2081:
1725:
1567:
1333:
937:
747:
466:
432:
428:
85:
55:
1659:
4346:
4336:
4286:
989:
942:
895:
846:
799:
752:
1542:
1425:
1308:
4469:
4459:
4356:
4351:
3625:
2679:
3391:. It is clear from the general arrangement of the book that he regarded only the five Platonic solids as regular.
1224:
628:, and holds both for convex polyhedra (where the correction factors are all 1), and the Kepler–Poinsot polyhedra:
35:
4474:
4464:
3682:
3584:
2068:
2890:
1951:
1458:
65:
4510:
4484:
4479:
4266:
3652:
2913:
1238:
2838:
states that the two star polyhedra can be constructed by adding pyramids to the faces of the
Platonic solids.
1931:
1911:
1589:
1355:
227:
2420:
4281:
4276:
3702:
3692:
3555:
2970:
3069:
3045:
2906:
2883:
2564:
4389:
3347:
3287:
3273:
1650:
1416:
1160:
490:{5/2, 5}, and so can not be a Kepler–Poinsot solid even though it still looks like one from outside.
634:
4068:
3961:
3934:
3633:
3516:
3384:
2963:
2850:
2024:
2004:
1582:
1472:
1348:
1086:
1043:
4153:
3609:
3492:
3331:
3317:
2826:
In his view the great stellation is related to the icosahedron as the small one is to the dodecahedron.
3243:
3229:
2959:
all faces and vertices are on 5-fold symmetry axes (so there are no yellow elements in these images).
3993:
2984:
2935:
2831:
2386:
2323:
2121:
2074:
1194:
1184:
508:
500:
3064:
3040:
2920:
2897:
4316:
3697:
3637:
3282:
3268:
2980:
2854:
2279:
2110:
2064:
984:
3021:
3016:
2786:
This implies that the pentagrams have the same size, and that the cores have the same edge length.
2395:
The pentagon faces of these cores are the invisible parts of the star polyhedra's pentagram faces.
4326:
4321:
4256:
4226:
4064:
3957:
3687:
3677:
3534:
3461:
3380:
2991:
2952:
2390:
2350:
2290:
2234:
2200:
2151:
1707:
1695:
1216:
1199:
794:
559:
478:
443:
103:
41:
3825:
3793:
3744:
Conway et al. (2008), p.405 Figure 26.1 Relationships among the three-dimensional star-polytopes
3326:
3312:
3259:
3254:
1154:
487:
3238:
3224:
4444:
4236:
4158:
4115:
4092:
4076:
4050:
3986:
3969:
3872:
3856:
3822:
3790:
3580:
3408:
3303:
3298:
3198:
3002:
2942:
2400:
2359:
2301:
2189:
2137:
2056:
1984:
1450:
890:
447:
138:. They can all be seen as three-dimensional analogues of the pentagram in one way or another.
117:
71:
2233:
If the intersections are treated as new edges and vertices, the figures obtained will not be
4454:
4449:
4042:
3864:
3774:
3759:
3672:
3561:
3372:
3215:
3210:
3201:
have the same edge length, namely the side length of a pentagram in the surrounding decagon.
1299:
2962:
The following table shows the solids in pairs of duals. In the top row they are shown with
1533:
1231:
600:
573:
4200:
3920:
3566:
3443:
3431:
3399:
3143:
3119:
2672:
2557:
2329:
2307:
2217:
2206:
2168:
2157:
1465:
1209:
841:
704:
131:
106:
4118:
3644:
17:
3623:'s interest in geometric forms often led to works based on or including regular solids;
1878:
4308:
3439:
3412:
1204:
1189:
708:
451:
1862:
1838:
4499:
4021:
4011:
3944:
3613:
3501:
3457:
gave the Kepler–Poinsot polyhedra the names by which they are generally known today.
3454:
3419:
3355:
2966:, in the bottom row with icosahedral symmetry (to which the mentioned colors refer).
2875:
1178:
715:
625:
567:
455:
135:
4147:
3660:
3620:
3138:
3114:
2459:
2296:
2285:
2195:
2184:
2146:
2132:
1822:
723:
483:
436:
120:
4137:
3861:
Shaping Space: Exploring
Polyhedra in Nature, Art, and the Geometrical Imagination
3600:
4038:, Cambridge University Press (1976) - discussion of proof of Euler characteristic
3656:
3095:
3090:
2665:
2550:
2318:
2274:
2116:
2105:
124:
711:, or more precisely, Petrie polygons with the same two dimensional projection.
4142:
3868:
3706:
3465:
3435:
3403:
2259:
2238:
2060:
1776:
maintains the type of faces, shifting and resizing them into parallel planes.
1767:
1721:
1694:
Conway's system of relations between the six polyhedra (ordered vertically by
1147:
113:
4123:
3830:
3798:
3427:. Poinsot did not know if he had discovered all the regular star polyhedra.
3173:
2835:
2781:
2467:
1799:
719:
470:
439:
424:
408:{\displaystyle \phi ^{5}={\tfrac {1}{2}}{\bigl (}11+5{\sqrt {5}}\,{\bigr )}}
128:
4169:
3575:
477:
part hidden inside the solid. The visible parts of each face comprise five
335:{\displaystyle \phi ^{2}={\tfrac {1}{2}}{\bigl (}3+{\sqrt {5}}\,{\bigr )},}
217:{\displaystyle \phi ^{4}={\tfrac {1}{2}}{\bigl (}7+3{\sqrt {5}}\,{\bigr )}}
3447:
2846:
2263:
2085:
474:
95:
2973:
from the 5-fold (red), 3-fold (yellow) and 2-fold (blue) symmetry axes.
2810:
i.e. as dodecahedron and icosahedron with pyramids added to their faces.
4163:
4132:
3778:, Book II, Proposition XXVI — p. 117 in the translation by E. J. Aiton)
3354:, Italy. It dates from the 15th century and is sometimes attributed to
3177:
2538:{\displaystyle {\frac {\text{core midradius}}{\text{hull midradius}}}}
2510:{\displaystyle {\frac {\text{hull midradius}}{\text{core midradius}}}}
3496:
3488:
3351:
3612:, Venice, Italy, dating from ca. 1430 and sometimes attributed to
3599:
3574:
3554:
3482:
2023:
2003:
1983:
1950:
1930:
1910:
3763:, Book V, Chapter III — p. 407 in the translation by E. J. Aiton)
3468:
for stellations in up to four dimensions. Within this scheme the
3733:
Star polytopes and the Schläfli function f(α,β,γ)
3394:
The small and great stellated dodecahedra, sometimes called the
2470:
is a common measure to compare the size of different polyhedra.)
4173:
4083:(Chapter 26. pp. 404: Regular star-polytopes Dimension 3)
4018:. San Carlos, CA: Wide World Publ./Tetra, p. 113, 1989.
4166:: Software used to create many of the images on this page.
3753:"augmented dodecahedron to which I have given the name of
2800:
Traditionally the two star polyhedra have been defined as
3903:
H.S.M. Coxeter, P. Du Val, H.T. Flather and J.F. Petrie;
3709:, 4-dimensional analogues of the Kepler–Poinsot polyhedra
2393:
with their edges and faces extended until they intersect.
3636:
of the great dodecahedron was used for the 1980s puzzle
4091:. California: University of California Press Berkeley.
3976:(Chapter 24, Regular Star-polytopes, pp. 404–408)
1798:
The polyhedra in this section are shown with the same
363:
290:
172:
3925:
Comptes rendus des séances de l'Académie des
Sciences
2788:(Compare the 5-fold orthographic projections below.)
2768:{\displaystyle {\frac {3-{\sqrt {5}}}{2}}=0.38196...}
2736:
2722:{\displaystyle {\frac {3+{\sqrt {5}}}{2}}=2.61803...}
2690:
2653:{\displaystyle {\frac {{\sqrt {5}}-1}{2}}=0.61803...}
2621:
2607:{\displaystyle {\frac {{\sqrt {5}}+1}{2}}=1.61803...}
2575:
2524:
2496:
2423:
2403:
992:
945:
898:
849:
802:
755:
637:
603:
576:
511:
348:
275:
230:
157:
4138:
Free paper models (nets) of Kepler–Poinsot polyhedra
2417:
times bigger than the core, and for the great it is
4433:
4365:
4305:
4245:
4207:
2090:The three others are facetings of the icosahedron.
2767:
2721:
2652:
2606:
2537:
2509:
2448:
2409:
1021:
974:
927:
878:
831:
784:
687:
616:
589:
544:
407:
334:
262:
216:
4005:Star Polytopes and the Schlafli Function f(α,β,γ)
3442:, and almost half a century after that, in 1858,
3172:The platonic hulls in these images have the same
2780:The platonic hulls in these images have the same
2397:For the small stellated dodecahedron the hull is
427:(star pentagons) as faces or vertex figures. The
3855:(2013). "Regular and semiregular polyhedra". In
2073:The three others are all the stellations of the
1022:{\displaystyle \left\{{\frac {10}{3,5}}\right\}}
3923:, Note sur la théorie des polyèdres réguliers,
3655:. The star spans 14 meters, and consists of an
3176:, so all the 5-fold projections below are in a
975:{\displaystyle \left\{{\frac {5}{2}},3\right\}}
928:{\displaystyle \left\{3,{\frac {5}{2}}\right\}}
879:{\displaystyle \left\{{\frac {6}{1,3}}\right\}}
832:{\displaystyle \left\{5,{\frac {5}{2}}\right\}}
785:{\displaystyle \left\{{\frac {5}{2}},5\right\}}
2845:If they were, the two star polyhedra would be
722:. They also show that the Petrie polygons are
4185:
415:times the original dodecahedron edge length.
400:
376:
324:
303:
209:
185:
8:
3893:File:Perspectiva Corporum Regularium 27e.jpg
3629:is based on a small stellated dodecahedron.
4154:VRML models of the Kepler–Poinsot polyhedra
3941:J. de l'École Polytechnique 9, 68–86, 1813.
2477:Hull and core of the stellated dodecahedra
4192:
4178:
4170:
4159:Stellation and facetting - a brief history
4024:, Memoire sur les polygones et polyèdres.
3863:(2nd ed.). Springer. pp. 41–52.
3162:
2860:
2473:
2092:
1778:
558:A modified form of Euler's formula, using
127:, and differ from these in having regular
3596:Regular star polyhedra in art and culture
3371:), a book of woodcuts published in 1568,
2746:
2737:
2735:
2700:
2691:
2689:
2625:
2622:
2620:
2579:
2576:
2574:
2525:
2523:
2497:
2495:
2440:
2422:
2402:
1757:great stellated dodecahedron (sgD = gsD)
1686:Relationships among the regular polyhedra
997:
991:
951:
944:
910:
897:
854:
848:
814:
801:
761:
754:
664:
642:
636:
608:
602:
581:
575:
510:
399:
398:
397:
390:
375:
374:
362:
353:
347:
323:
322:
321:
314:
302:
301:
289:
280:
274:
250:
235:
229:
208:
207:
206:
199:
184:
183:
171:
162:
156:
4133:Paper models of Kepler–Poinsot polyhedra
3947:, On Poinsot's Four New Regular Solids.
2975:
2385:stellated dodecahedron can be seen as a
2269:
1734:
1710:defines the Kepler–Poinsot polyhedra as
1689:
1130:
728:
263:{\displaystyle \phi ^{3}=2+{\sqrt {5}},}
3724:
3663:inside a great stellated dodecahedron.
2934:All Kepler–Poinsot polyhedra have full
2864:Stellated dodecahedra as augmentations
2449:{\displaystyle \varphi +1=\varphi ^{2}}
1702:
4292:nonconvex great rhombicosidodecahedron
3735:p. 121 1. The Kepler–Poinsot polyhedra
3398:, were first recognized as regular by
703:The Kepler–Poinsot polyhedra exist in
2814:Kepler calls the small stellation an
2346:
151:The great icosahedron edge length is
7:
3980:Kaleidoscopes: Selected Writings of
3383:(both shown below). There is also a
2262:of the solids sharing vertices are
2246:List of Wenninger polyhedron models
2237:, but they can still be considered
688:{\displaystyle d_{v}V-E+d_{f}F=2D.}
4103:Chapter 8: Kepler Poisot polyhedra
3907:, 3rd Edition, Tarquin, 1999. p.11
3369:Perspectives of the regular solids
714:The following images show the two
499:solids are, and in particular the
454:polygonal faces, but pentagrammic
25:
4410:great stellapentakis dodecahedron
4395:medial pentagonal hexecontahedron
4380:small stellapentakis dodecahedron
4297:great truncated icosidodecahedron
3954:, pp. 123–127 and 209, 1859.
3446:provided a more elegant proof by
3402:around 1619. He obtained them by
2834:definitions are still used. E.g.
4425:great pentagonal hexecontahedron
4400:medial disdyakis triacontahedron
4385:medial deltoidal hexecontahedron
3524:
3515:
3330:
3325:
3316:
3311:
3302:
3297:
3286:
3281:
3272:
3267:
3258:
3253:
3242:
3237:
3228:
3223:
3214:
3209:
3142:
3137:
3118:
3113:
3094:
3089:
3068:
3063:
3044:
3039:
3020:
3015:
2938:, just like their convex hulls.
2919:
2912:
2905:
2896:
2889:
2882:
2678:
2671:
2664:
2563:
2556:
2549:
2328:
2317:
2306:
2295:
2284:
2273:
2216:
2205:
2194:
2183:
2167:
2156:
2145:
2131:
2115:
2104:
1877:
1861:
1837:
1821:
1791:
1703:Conway's operational terminology
1658:
1649:
1628:
1623:
1618:
1613:
1608:
1603:
1598:
1588:
1581:
1574:
1541:
1532:
1511:
1506:
1501:
1496:
1491:
1486:
1481:
1471:
1464:
1457:
1424:
1415:
1394:
1389:
1384:
1379:
1374:
1369:
1364:
1354:
1347:
1340:
1307:
1298:
1277:
1272:
1267:
1262:
1257:
1252:
1247:
1237:
1230:
1223:
1103:
1094:
1085:
1060:
1051:
1042:
78:
64:
48:
34:
4420:great disdyakis triacontahedron
4415:great deltoidal hexecontahedron
3544:Perspectiva Corporum Regularium
3364:Perspectiva corporum regularium
2358:share vertices, skeletons form
545:{\displaystyle \chi =V-E+F=2\ }
27:Any of 4 regular star polyhedra
4375:medial rhombic triacontahedron
4049:. Cambridge University Press.
4014:, (The Kepler–Poinsot Solids)
3826:"Great Stellated Dodecahedron"
3794:"Small Stellated Dodecahedron"
3005:) and {5/2, 3} (
2994:) and {5/2, 5} (
2226:(the one with yellow vertices)
1:
4405:great rhombic triacontahedron
3939:Recherches sur les polyèdres.
1782:Conway relations illustrated
1562:great stellated dodecahedron
1328:small stellated dodecahedron
4342:great dodecahemidodecahedron
4332:small dodecahemidodecahedron
4272:truncated dodecadodecahedron
4262:truncated great dodecahedron
4232:great stellated dodecahedron
4222:small stellated dodecahedron
4164:Stella: Polyhedron Navigator
4089:Polyhedra: A Visual Approach
3931:(1858), pp. 79–82, 117.
3619:In the 20th century, artist
3539:great stellated dodecahedron
3470:small stellated dodecahedron
3434:proved the list complete by
3389:small stellated dodecahedron
3377:great stellated dodecahedron
2983:) and {5, 3} (
2335:great stellated dodecahedron
2313:small stellated dodecahedron
2082:great stellated dodecahedron
1749:stellated dodecahedron (sD)
1726:small stellated dodecahedron
1568:great stellated dodecahedron
1565:
1448:
1334:small stellated dodecahedron
1331:
1214:
938:great stellated dodecahedron
748:small stellated dodecahedron
467:small stellated dodecahedron
433:great stellated dodecahedron
86:Great stellated dodecahedron
56:Small stellated dodecahedron
4347:great icosihemidodecahedron
4337:small icosihemidodecahedron
4287:truncated great icosahedron
4026:J. de l'École Polytechnique
4003:(Paper 10) H.S.M. Coxeter,
2141:(the one with yellow faces)
707:pairs. Duals have the same
699:Duality and Petrie polygons
4532:
4470:great dodecahemidodecacron
4460:small dodecahemidodecacron
4357:small dodecahemicosahedron
4352:great dodecahemicosahedron
3997:(Paper 1) H.S.M. Coxeter,
3184:projection of the compound
2096:Stellations and facetings
4475:great icosihemidodecacron
4465:small icosihemidodecacron
3905:The Fifty-Nine Icosahedra
3869:10.1007/978-0-387-92714-5
3683:List of regular polytopes
3170:
3166:orthographic projections
3165:
2863:
2778:
2476:
2368:The stellated dodecahedra
2357:
2344:share vertices and edges
2343:
2341:share vertices and edges
2340:
2252:Shared vertices and edges
2215:
2130:
2114:
2103:
2095:
2069:The Fifty-Nine Icosahedra
2051:Stellations and facetings
1781:
100:Kepler–Poinsot polyhedron
18:Kepler-Poinsot polyhedron
4506:Kepler–Poinsot polyhedra
4485:small dodecahemicosacron
4480:great dodecahemicosacron
4267:rhombidodecadodecahedron
4201:Star-polyhedra navigator
4073:The Symmetries of Things
3883:See in particular p. 42.
3653:Oslo Airport, Gardermoen
2971:orthographic projections
2410:{\displaystyle \varphi }
1746:great dodecahedron (gD)
1136:(Conway's abbreviation)
733:horizontal edge in front
112:They may be obtained by
4282:great icosidodecahedron
4277:snub dodecadodecahedron
4031:, pp. 16–48, 1810.
3999:The Nine Regular Solids
3705:– the ten regular star
3703:Regular star 4-polytope
3693:Uniform star polyhedron
3559:Stellated dodecahedra,
3460:A hundred years later,
1754:great icosahedron (gI)
473:faces with the central
4436:uniform polyhedra with
4390:small rhombidodecacron
4119:"Kepler–Poinsot solid"
4036:Proofs and Refutations
4016:The Joy of Mathematics
3966:The Symmetry of Things
3605:
3588:
3570:
3506:
3474:stellated dodecahedron
3466:systematic terminology
2969:The table below shows
2816:augmented dodecahedron
2769:
2723:
2654:
2608:
2539:
2511:
2450:
2411:
2039:
2019:
1999:
1966:
1946:
1926:
1730:stellated dodecahedron
1699:
1114:Compound of gI and gsD
1023:
976:
929:
880:
833:
786:
738:vertical edge in front
689:
618:
591:
546:
494:Euler characteristic χ
409:
336:
264:
218:
4148:Kepler-Poinsot Solids
4143:The Uniform Polyhedra
4087:Anthony Pugh (1976).
4069:Chaim Goodman-Strauss
3962:Chaim Goodman-Strauss
3935:Augustin-Louis Cauchy
3603:
3579:Cardboard model of a
3578:
3558:
3486:
3411:, as the traditional
2964:pyritohedral symmetry
2851:pentakis dodecahedron
2770:
2724:
2655:
2609:
2540:
2512:
2451:
2412:
2027:
2007:
1987:
1954:
1934:
1914:
1718:of the convex solids.
1693:
1116:with Petrie decagrams
1071:Compound of sD and gD
1024:
977:
930:
881:
834:
787:
690:
619:
617:{\displaystyle d_{f}}
592:
590:{\displaystyle d_{v}}
547:
410:
337:
265:
219:
4438:infinite stellations
4246:Uniform truncations
3453:The following year,
2936:icosahedral symmetry
2818:(then nicknaming it
2734:
2688:
2619:
2573:
2522:
2494:
2421:
2401:
2088:of the dodecahedron.
1073:with Petrie hexagons
990:
943:
896:
847:
800:
753:
635:
601:
574:
509:
346:
273:
228:
155:
4516:Nonconvex polyhedra
4366:Duals of nonconvex
4317:tetrahemihexahedron
4150:in Visual Polyhedra
3698:Polyhedral compound
3610:St. Mark's Basilica
3585:Tübingen University
3430:Three years later,
3348:St. Mark's Basilica
2855:triakis icosahedron
1445:great dodecahedron
479:isosceles triangles
423:These figures have
4434:Duals of nonconvex
4327:octahemioctahedron
4322:cubohemioctahedron
4306:Nonconvex uniform
4257:dodecadodecahedron
4248:of Kepler-Poinsot
4227:great dodecahedron
4215:regular polyhedra)
4116:Weisstein, Eric W.
3857:Senechal, Marjorie
3823:Weisstein, Eric W.
3791:Weisstein, Eric W.
3688:Uniform polyhedron
3678:Regular polyhedron
3651:is displayed near
3606:
3589:
3571:
3535:Great dodecahedron
3507:
3381:great dodecahedron
3189:This implies that
3180:of the same size.
2953:great dodecahedron
2849:equivalent to the
2765:
2719:
2650:
2604:
2535:
2507:
2446:
2407:
2391:great dodecahedron
2351:dodecahedral graph
2291:great dodecahedron
2040:
2020:
2000:
1967:
1947:
1927:
1770:described below.)
1700:
1679:great icosahedron
1217:great dodecahedron
1019:
972:
925:
876:
829:
795:great dodecahedron
782:
685:
614:
587:
542:
444:great dodecahedron
405:
372:
332:
299:
260:
214:
181:
42:Great dodecahedron
4493:
4492:
4445:tetrahemihexacron
4368:uniform polyhedra
4237:great icosahedron
4081:978-1-56881-220-5
4067:, Heidi Burgiel,
4061:, pp. 39–41.
4043:Wenninger, Magnus
3991:978-0-471-01003-6
3974:978-1-56881-220-5
3960:, Heidi Burgiel,
3878:978-0-387-92713-8
3853:Coxeter, H. S. M.
3643:Norwegian artist
3593:
3592:
3581:great icosahedron
3505:
3425:Poinsot polyhedra
3339:
3338:
3161:
3160:
3156:
3132:
3108:
3082:
3058:
3034:
2943:great icosahedron
2927:
2926:
2793:
2792:
2757:
2751:
2711:
2705:
2642:
2630:
2596:
2584:
2533:
2532:
2529:
2505:
2504:
2501:
2471:
2365:
2364:
2360:icosahedral graph
2302:great icosahedron
2231:
2230:
2227:
2142:
2057:great icosahedron
2048:
2047:
2044:
2043:
1971:
1970:
1898:
1897:
1803:
1761:
1760:
1741:dodecahedron (D)
1722:naming convention
1683:
1682:
1451:great icosahedron
1124:
1123:
1032:
1031:
1013:
959:
918:
891:great icosahedron
870:
822:
769:
541:
465:For example, the
448:great icosahedron
437:nonconvex regular
395:
371:
319:
298:
255:
204:
180:
72:Great icosahedron
16:(Redirected from
4523:
4455:octahemioctacron
4450:hexahemioctacron
4194:
4187:
4180:
4171:
4129:
4128:
4102:
4060:
3982:H. S. M. Coxeter
3908:
3901:
3895:
3890:
3884:
3882:
3849:
3843:
3842:
3841:
3839:
3838:
3817:
3811:
3810:
3809:
3807:
3806:
3785:
3779:
3775:Harmonices Mundi
3770:
3764:
3760:Harmonices Mundi
3751:
3745:
3742:
3736:
3729:
3673:Regular polytope
3638:Alexander's Star
3604:Alexander's Star
3562:Harmonices Mundi
3528:
3519:
3499:
3479:
3478:
3396:Kepler polyhedra
3373:Wenzel Jamnitzer
3335:
3334:
3329:
3321:
3320:
3315:
3307:
3306:
3301:
3291:
3290:
3285:
3277:
3276:
3271:
3263:
3262:
3257:
3247:
3246:
3241:
3233:
3232:
3227:
3219:
3218:
3213:
3202:
3187:
3163:
3150:
3147:
3146:
3141:
3126:
3123:
3122:
3117:
3102:
3099:
3098:
3093:
3076:
3073:
3072:
3067:
3052:
3049:
3048:
3043:
3028:
3025:
3024:
3019:
2976:
2923:
2916:
2909:
2900:
2893:
2886:
2872:Star polyhedron
2861:
2858:
2843:
2827:
2811:
2774:
2772:
2771:
2766:
2758:
2753:
2752:
2747:
2738:
2728:
2726:
2725:
2720:
2712:
2707:
2706:
2701:
2692:
2682:
2675:
2668:
2659:
2657:
2656:
2651:
2643:
2638:
2631:
2626:
2623:
2613:
2611:
2610:
2605:
2597:
2592:
2585:
2580:
2577:
2567:
2560:
2553:
2544:
2542:
2541:
2536:
2534:
2530:
2527:
2526:
2516:
2514:
2513:
2508:
2506:
2502:
2499:
2498:
2485:Star polyhedron
2474:
2465:
2463:
2455:
2453:
2452:
2447:
2445:
2444:
2416:
2414:
2413:
2408:
2353:
2347:share vertices,
2332:
2321:
2310:
2299:
2288:
2277:
2270:
2267:
2225:
2220:
2209:
2198:
2187:
2171:
2160:
2149:
2140:
2135:
2119:
2108:
2093:
2038:
2032:
2018:
2012:
1998:
1992:
1980:
1979:
1965:
1959:
1945:
1939:
1925:
1919:
1907:
1906:
1889:
1881:
1872:
1865:
1848:
1841:
1832:
1825:
1813:
1812:
1796:
1795:
1779:
1738:icosahedron (I)
1735:
1662:
1653:
1633:
1632:
1631:
1627:
1626:
1622:
1621:
1617:
1616:
1612:
1611:
1607:
1606:
1602:
1601:
1592:
1585:
1578:
1545:
1536:
1516:
1515:
1514:
1510:
1509:
1505:
1504:
1500:
1499:
1495:
1494:
1490:
1489:
1485:
1484:
1475:
1468:
1461:
1428:
1419:
1399:
1398:
1397:
1393:
1392:
1388:
1387:
1383:
1382:
1378:
1377:
1373:
1372:
1368:
1367:
1358:
1351:
1344:
1311:
1302:
1282:
1281:
1280:
1276:
1275:
1271:
1270:
1266:
1265:
1261:
1260:
1256:
1255:
1251:
1250:
1241:
1234:
1227:
1131:
1107:
1098:
1089:
1064:
1055:
1046:
1034:
1033:
1028:
1026:
1025:
1020:
1018:
1014:
1012:
998:
981:
979:
978:
973:
971:
967:
960:
952:
934:
932:
931:
926:
924:
920:
919:
911:
885:
883:
882:
877:
875:
871:
869:
855:
838:
836:
835:
830:
828:
824:
823:
815:
791:
789:
788:
783:
781:
777:
770:
762:
739:
734:
729:
694:
692:
691:
686:
669:
668:
647:
646:
623:
621:
620:
615:
613:
612:
596:
594:
593:
588:
586:
585:
551:
549:
548:
543:
539:
414:
412:
411:
406:
404:
403:
396:
391:
380:
379:
373:
364:
358:
357:
341:
339:
338:
333:
328:
327:
320:
315:
307:
306:
300:
291:
285:
284:
269:
267:
266:
261:
256:
251:
240:
239:
223:
221:
220:
215:
213:
212:
205:
200:
189:
188:
182:
173:
167:
166:
82:
68:
52:
38:
21:
4531:
4530:
4526:
4525:
4524:
4522:
4521:
4520:
4511:Johannes Kepler
4496:
4495:
4494:
4489:
4437:
4435:
4429:
4367:
4361:
4307:
4301:
4249:
4247:
4241:
4214:
4210:
4209:Kepler-Poinsot
4203:
4198:
4114:
4113:
4110:
4099:
4086:
4057:
4041:
4034:Lakatos, Imre;
3917:
3912:
3911:
3902:
3898:
3891:
3887:
3879:
3851:
3850:
3846:
3836:
3834:
3821:
3820:
3818:
3814:
3804:
3802:
3789:
3788:
3786:
3782:
3771:
3767:
3752:
3748:
3743:
3739:
3730:
3726:
3721:
3716:
3669:
3649:The Kepler Star
3598:
3567:Johannes Kepler
3551:
3550:
3549:
3548:
3531:
3530:
3529:
3521:
3520:
3440:Platonic solids
3432:Augustin Cauchy
3413:Platonic solids
3400:Johannes Kepler
3387:version of the
3344:
3324:
3310:
3296:
3280:
3266:
3252:
3236:
3222:
3208:
3188:
3181:
3148:
3136:
3124:
3112:
3100:
3088:
3074:
3062:
3050:
3038:
3026:
3014:
2950:
2932:
2844:
2841:
2825:
2809:
2798:
2787:
2785:
2739:
2732:
2731:
2693:
2686:
2685:
2624:
2617:
2616:
2578:
2571:
2570:
2520:
2519:
2492:
2491:
2464:
2457:
2436:
2419:
2418:
2399:
2398:
2396:
2394:
2375:
2370:
2349:skeletons form
2348:
2333:
2322:
2311:
2300:
2289:
2278:
2257:
2254:
2221:
2210:
2199:
2188:
2172:
2161:
2150:
2136:
2120:
2109:
2089:
2072:
2053:
2034:
2028:
2014:
2008:
1994:
1988:
1961:
1955:
1941:
1935:
1921:
1915:
1894:
1893:
1892:
1891:
1890:
1884:
1882:
1874:
1873:
1868:
1866:
1853:
1852:
1851:
1850:
1849:
1844:
1842:
1834:
1833:
1828:
1826:
1797:
1719:
1705:
1688:
1676:
1663:
1654:
1645:
1637:
1629:
1624:
1619:
1614:
1609:
1604:
1599:
1597:
1596:
1570:
1559:
1546:
1537:
1528:
1520:
1512:
1507:
1502:
1497:
1492:
1487:
1482:
1480:
1479:
1453:
1442:
1429:
1420:
1411:
1403:
1395:
1390:
1385:
1380:
1375:
1370:
1365:
1363:
1362:
1336:
1325:
1312:
1303:
1294:
1286:
1278:
1273:
1268:
1263:
1258:
1253:
1248:
1246:
1245:
1219:
1183:
1180:
1174:
1166:
1159:
1158:{p, q} and
1157:
1150:
1143:
1135:
1129:
1120:
1119:
1118:
1117:
1110:
1109:
1108:
1100:
1099:
1091:
1090:
1077:
1076:
1075:
1074:
1067:
1066:
1065:
1057:
1056:
1048:
1047:
1002:
993:
988:
987:
950:
946:
941:
940:
903:
899:
894:
893:
859:
850:
845:
844:
807:
803:
798:
797:
760:
756:
751:
750:
742:Petrie polygon
737:
732:
701:
660:
638:
633:
632:
624:) was given by
604:
599:
598:
577:
572:
571:
507:
506:
496:
488:Schläfli symbol
484:Euler's formula
421:
349:
344:
343:
276:
271:
270:
231:
226:
225:
158:
153:
152:
149:
144:
142:Characteristics
102:is any of four
92:
91:
90:
89:
88:
83:
75:
74:
69:
60:
59:
58:
53:
45:
44:
39:
28:
23:
22:
15:
12:
11:
5:
4529:
4527:
4519:
4518:
4513:
4508:
4498:
4497:
4491:
4490:
4488:
4487:
4482:
4477:
4472:
4467:
4462:
4457:
4452:
4447:
4441:
4439:
4431:
4430:
4428:
4427:
4422:
4417:
4412:
4407:
4402:
4397:
4392:
4387:
4382:
4377:
4371:
4369:
4363:
4362:
4360:
4359:
4354:
4349:
4344:
4339:
4334:
4329:
4324:
4319:
4313:
4311:
4303:
4302:
4300:
4299:
4294:
4289:
4284:
4279:
4274:
4269:
4264:
4259:
4253:
4251:
4243:
4242:
4240:
4239:
4234:
4229:
4224:
4218:
4216:
4205:
4204:
4199:
4197:
4196:
4189:
4182:
4174:
4168:
4167:
4161:
4156:
4151:
4145:
4140:
4135:
4130:
4109:
4108:External links
4106:
4105:
4104:
4097:
4084:
4065:John H. Conway
4062:
4055:
4039:
4032:
4019:
4009:
4008:
4007:
4001:
3977:
3958:John H. Conway
3955:
3942:
3932:
3916:
3913:
3910:
3909:
3896:
3885:
3877:
3844:
3812:
3780:
3765:
3746:
3737:
3723:
3722:
3720:
3717:
3715:
3712:
3711:
3710:
3700:
3695:
3690:
3685:
3680:
3675:
3668:
3665:
3597:
3594:
3591:
3590:
3572:
3552:
3533:
3532:
3523:
3522:
3514:
3513:
3512:
3511:
3510:
3508:
3343:
3340:
3337:
3336:
3322:
3308:
3293:
3292:
3278:
3264:
3249:
3248:
3234:
3220:
3205:
3204:
3168:
3167:
3159:
3158:
3134:
3110:
3085:
3084:
3060:
3036:
3011:
3010:
2999:
2988:
2931:
2928:
2925:
2924:
2917:
2910:
2902:
2901:
2894:
2887:
2879:
2878:
2873:
2870:
2866:
2865:
2797:
2794:
2791:
2790:
2776:
2775:
2764:
2761:
2756:
2750:
2745:
2742:
2729:
2718:
2715:
2710:
2704:
2699:
2696:
2683:
2676:
2669:
2661:
2660:
2649:
2646:
2641:
2637:
2634:
2629:
2614:
2603:
2600:
2595:
2591:
2588:
2583:
2568:
2561:
2554:
2546:
2545:
2531:hull midradius
2528:core midradius
2517:
2503:core midradius
2500:hull midradius
2489:
2486:
2483:
2479:
2478:
2456:times bigger.
2443:
2439:
2435:
2432:
2429:
2426:
2406:
2374:
2371:
2369:
2366:
2363:
2362:
2355:
2354:
2345:
2342:
2338:
2337:
2326:
2315:
2304:
2293:
2282:
2253:
2250:
2229:
2228:
2214:
2203:
2192:
2181:
2177:
2176:
2165:
2154:
2143:
2129:
2125:
2124:
2113:
2102:
2098:
2097:
2059:is one of the
2052:
2049:
2046:
2045:
2042:
2041:
2021:
2001:
1977:
1973:
1972:
1969:
1968:
1948:
1928:
1904:
1900:
1899:
1896:
1895:
1883:
1876:
1875:
1867:
1860:
1859:
1858:
1857:
1856:
1854:
1843:
1836:
1835:
1827:
1820:
1819:
1818:
1817:
1816:
1810:
1806:
1805:
1788:
1784:
1783:
1759:
1758:
1755:
1751:
1750:
1747:
1743:
1742:
1739:
1704:
1701:
1687:
1684:
1681:
1680:
1677:
1674:
1671:
1668:
1665:
1656:
1647:
1642:
1639:
1634:
1593:
1586:
1579:
1572:
1564:
1563:
1560:
1557:
1554:
1551:
1548:
1539:
1530:
1525:
1522:
1517:
1476:
1469:
1462:
1455:
1447:
1446:
1443:
1440:
1437:
1434:
1431:
1422:
1413:
1408:
1405:
1400:
1359:
1352:
1345:
1338:
1330:
1329:
1326:
1323:
1320:
1317:
1314:
1305:
1296:
1291:
1288:
1283:
1242:
1235:
1228:
1221:
1213:
1212:
1207:
1202:
1197:
1192:
1190:Petrie polygon
1187:
1176:
1171:
1168:
1163:
1161:Coxeter-Dynkin
1152:
1145:
1140:
1137:
1128:
1125:
1122:
1121:
1112:
1111:
1102:
1101:
1093:
1092:
1084:
1083:
1082:
1081:
1080:
1078:
1069:
1068:
1059:
1058:
1050:
1049:
1041:
1040:
1039:
1038:
1037:
1030:
1029:
1017:
1011:
1008:
1005:
1001:
996:
982:
970:
966:
963:
958:
955:
949:
935:
923:
917:
914:
909:
906:
902:
887:
886:
874:
868:
865:
862:
858:
853:
839:
827:
821:
818:
813:
810:
806:
792:
780:
776:
773:
768:
765:
759:
744:
743:
740:
735:
718:with the same
716:dual compounds
709:Petrie polygon
700:
697:
696:
695:
684:
681:
678:
675:
672:
667:
663:
659:
656:
653:
650:
645:
641:
611:
607:
584:
580:
568:vertex figures
553:
552:
538:
535:
532:
529:
526:
523:
520:
517:
514:
501:Euler relation
495:
492:
456:vertex figures
420:
417:
402:
394:
389:
386:
383:
378:
370:
367:
361:
356:
352:
331:
326:
318:
313:
310:
305:
297:
294:
288:
283:
279:
259:
254:
249:
246:
243:
238:
234:
211:
203:
198:
195:
192:
187:
179:
176:
170:
165:
161:
148:
145:
143:
140:
136:vertex figures
107:star polyhedra
84:
77:
76:
70:
63:
62:
61:
54:
47:
46:
40:
33:
32:
31:
30:
29:
26:
24:
14:
13:
10:
9:
6:
4:
3:
2:
4528:
4517:
4514:
4512:
4509:
4507:
4504:
4503:
4501:
4486:
4483:
4481:
4478:
4476:
4473:
4471:
4468:
4466:
4463:
4461:
4458:
4456:
4453:
4451:
4448:
4446:
4443:
4442:
4440:
4432:
4426:
4423:
4421:
4418:
4416:
4413:
4411:
4408:
4406:
4403:
4401:
4398:
4396:
4393:
4391:
4388:
4386:
4383:
4381:
4378:
4376:
4373:
4372:
4370:
4364:
4358:
4355:
4353:
4350:
4348:
4345:
4343:
4340:
4338:
4335:
4333:
4330:
4328:
4325:
4323:
4320:
4318:
4315:
4314:
4312:
4310:
4309:hemipolyhedra
4304:
4298:
4295:
4293:
4290:
4288:
4285:
4283:
4280:
4278:
4275:
4273:
4270:
4268:
4265:
4263:
4260:
4258:
4255:
4254:
4252:
4244:
4238:
4235:
4233:
4230:
4228:
4225:
4223:
4220:
4219:
4217:
4212:
4206:
4202:
4195:
4190:
4188:
4183:
4181:
4176:
4175:
4172:
4165:
4162:
4160:
4157:
4155:
4152:
4149:
4146:
4144:
4141:
4139:
4136:
4134:
4131:
4126:
4125:
4120:
4117:
4112:
4111:
4107:
4100:
4098:0-520-03056-7
4094:
4090:
4085:
4082:
4078:
4074:
4070:
4066:
4063:
4058:
4056:0-521-54325-8
4052:
4048:
4044:
4040:
4037:
4033:
4030:
4027:
4023:
4022:Louis Poinsot
4020:
4017:
4013:
4012:Theoni Pappas
4010:
4006:
4002:
4000:
3996:
3995:
3994:
3992:
3988:
3984:
3983:
3978:
3975:
3971:
3967:
3963:
3959:
3956:
3953:
3950:
3946:
3945:Arthur Cayley
3943:
3940:
3936:
3933:
3930:
3926:
3922:
3919:
3918:
3914:
3906:
3900:
3897:
3894:
3889:
3886:
3880:
3874:
3870:
3866:
3862:
3858:
3854:
3848:
3845:
3833:
3832:
3827:
3824:
3816:
3813:
3801:
3800:
3795:
3792:
3784:
3781:
3777:
3776:
3769:
3766:
3762:
3761:
3756:
3750:
3747:
3741:
3738:
3734:
3728:
3725:
3718:
3713:
3708:
3704:
3701:
3699:
3696:
3694:
3691:
3689:
3686:
3684:
3681:
3679:
3676:
3674:
3671:
3670:
3666:
3664:
3662:
3658:
3654:
3650:
3647:'s sculpture
3646:
3641:
3639:
3635:
3630:
3628:
3627:
3622:
3617:
3615:
3614:Paulo Uccello
3611:
3602:
3595:
3587:(around 1860)
3586:
3582:
3577:
3573:
3568:
3564:
3563:
3557:
3553:
3546:
3545:
3540:
3536:
3527:
3518:
3509:
3503:
3502:Paolo Uccello
3500:(possibly by
3498:
3494:
3490:
3485:
3481:
3480:
3477:
3475:
3471:
3467:
3463:
3458:
3456:
3455:Arthur Cayley
3451:
3449:
3445:
3441:
3437:
3433:
3428:
3426:
3421:
3420:Louis Poinsot
3416:
3414:
3410:
3405:
3401:
3397:
3392:
3390:
3386:
3382:
3378:
3374:
3370:
3366:
3365:
3359:
3357:
3356:Paolo Uccello
3353:
3349:
3341:
3333:
3328:
3323:
3319:
3314:
3309:
3305:
3300:
3295:
3294:
3289:
3284:
3279:
3275:
3270:
3265:
3261:
3256:
3251:
3250:
3245:
3240:
3235:
3231:
3226:
3221:
3217:
3212:
3207:
3206:
3203:
3200:
3196:
3192:
3185:
3179:
3175:
3169:
3164:
3157:
3154:
3145:
3140:
3135:
3133:
3130:
3121:
3116:
3111:
3109:
3106:
3097:
3092:
3087:
3086:
3083:
3080:
3071:
3066:
3061:
3059:
3056:
3047:
3042:
3037:
3035:
3032:
3023:
3018:
3013:
3012:
3008:
3004:
3000:
2997:
2993:
2989:
2986:
2982:
2978:
2977:
2974:
2972:
2967:
2965:
2960:
2958:
2954:
2948:
2944:
2939:
2937:
2929:
2922:
2918:
2915:
2911:
2908:
2904:
2903:
2899:
2895:
2892:
2888:
2885:
2881:
2880:
2877:
2876:Catalan solid
2874:
2871:
2868:
2867:
2862:
2859:
2856:
2852:
2848:
2847:topologically
2839:
2837:
2833:
2828:
2823:
2821:
2817:
2812:
2807:
2803:
2802:augmentations
2796:Augmentations
2795:
2789:
2783:
2777:
2762:
2759:
2754:
2748:
2743:
2740:
2730:
2716:
2713:
2708:
2702:
2697:
2694:
2684:
2681:
2677:
2674:
2670:
2667:
2663:
2662:
2647:
2644:
2639:
2635:
2632:
2627:
2615:
2601:
2598:
2593:
2589:
2586:
2581:
2569:
2566:
2562:
2559:
2555:
2552:
2548:
2547:
2518:
2490:
2487:
2484:
2481:
2480:
2475:
2472:
2469:
2461:
2441:
2437:
2433:
2430:
2427:
2424:
2404:
2392:
2388:
2384:
2380:
2373:Hull and core
2372:
2367:
2361:
2356:
2352:
2339:
2336:
2331:
2327:
2325:
2320:
2316:
2314:
2309:
2305:
2303:
2298:
2294:
2292:
2287:
2283:
2281:
2276:
2272:
2271:
2268:
2265:
2264:topologically
2261:
2251:
2249:
2247:
2242:
2240:
2236:
2224:
2219:
2213:
2208:
2204:
2202:
2197:
2193:
2191:
2186:
2182:
2179:
2178:
2175:
2170:
2166:
2164:
2159:
2155:
2153:
2148:
2144:
2139:
2134:
2127:
2126:
2123:
2118:
2112:
2107:
2100:
2099:
2094:
2091:
2087:
2083:
2078:
2076:
2070:
2066:
2062:
2058:
2050:
2037:
2031:
2026:
2022:
2017:
2011:
2006:
2002:
1997:
1991:
1986:
1982:
1981:
1978:
1975:
1974:
1964:
1958:
1953:
1949:
1944:
1938:
1933:
1929:
1924:
1918:
1913:
1909:
1908:
1905:
1902:
1901:
1888:
1880:
1871:
1864:
1855:
1847:
1840:
1831:
1824:
1815:
1814:
1811:
1808:
1807:
1804:
1801:
1794:
1789:
1786:
1785:
1780:
1777:
1775:
1771:
1769:
1765:
1756:
1753:
1752:
1748:
1745:
1744:
1740:
1737:
1736:
1733:
1731:
1727:
1723:
1717:
1713:
1709:
1697:
1692:
1685:
1678:
1672:
1669:
1666:
1661:
1657:
1652:
1648:
1643:
1640:
1635:
1595:{5/2, 3}
1594:
1591:
1587:
1584:
1580:
1577:
1573:
1569:
1566:
1561:
1555:
1552:
1549:
1544:
1540:
1535:
1531:
1526:
1523:
1518:
1478:{3, 5/2}
1477:
1474:
1470:
1467:
1463:
1460:
1456:
1452:
1449:
1444:
1438:
1435:
1432:
1427:
1423:
1418:
1414:
1409:
1406:
1401:
1361:{5/2, 5}
1360:
1357:
1353:
1350:
1346:
1343:
1339:
1335:
1332:
1327:
1321:
1318:
1315:
1310:
1306:
1301:
1297:
1292:
1289:
1284:
1244:{5, 5/2}
1243:
1240:
1236:
1233:
1229:
1226:
1222:
1218:
1215:
1211:
1208:
1206:
1203:
1201:
1198:
1196:
1193:
1191:
1188:
1186:
1182:
1177:
1172:
1169:
1164:
1162:
1156:
1153:
1149:
1146:
1141:
1138:
1133:
1132:
1126:
1115:
1106:
1097:
1088:
1079:
1072:
1063:
1054:
1045:
1036:
1035:
1015:
1009:
1006:
1003:
999:
994:
986:
983:
968:
964:
961:
956:
953:
947:
939:
936:
921:
915:
912:
907:
904:
900:
892:
889:
888:
872:
866:
863:
860:
856:
851:
843:
840:
825:
819:
816:
811:
808:
804:
796:
793:
778:
774:
771:
766:
763:
757:
749:
746:
745:
741:
736:
731:
730:
727:
725:
721:
717:
712:
710:
706:
698:
682:
679:
676:
673:
670:
665:
661:
657:
654:
651:
648:
643:
639:
631:
630:
629:
627:
626:Arthur Cayley
609:
605:
597:) and faces (
582:
578:
569:
565:
561:
556:
536:
533:
530:
527:
524:
521:
518:
515:
512:
505:
504:
503:
502:
493:
491:
489:
485:
480:
476:
472:
468:
463:
459:
457:
453:
449:
445:
441:
438:
434:
430:
426:
419:Non-convexity
418:
416:
392:
387:
384:
381:
368:
365:
359:
354:
350:
329:
316:
311:
308:
295:
292:
286:
281:
277:
257:
252:
247:
244:
241:
236:
232:
201:
196:
193:
190:
177:
174:
168:
163:
159:
146:
141:
139:
137:
133:
130:
126:
122:
119:
115:
110:
108:
105:
101:
97:
87:
81:
73:
67:
57:
51:
43:
37:
19:
4208:
4122:
4088:
4072:
4046:
4035:
4028:
4025:
4015:
4004:
3998:
3979:
3965:
3951:
3948:
3938:
3928:
3924:
3915:Bibliography
3904:
3899:
3888:
3860:
3847:
3835:. Retrieved
3829:
3815:
3803:. Retrieved
3797:
3783:
3773:
3768:
3758:
3754:
3749:
3740:
3732:
3727:
3661:dodecahedron
3648:
3645:Vebjørn Sand
3642:
3631:
3624:
3621:M. C. Escher
3618:
3607:
3560:
3542:
3473:
3472:is just the
3464:developed a
3459:
3452:
3429:
3424:
3417:
3395:
3393:
3375:depicts the
3368:
3362:
3360:
3345:
3171:
3149:
3125:
3101:
3075:
3051:
3027:
2968:
2961:
2940:
2933:
2840:
2829:
2824:
2819:
2815:
2813:
2805:
2801:
2799:
2779:
2460:Golden ratio
2376:
2324:dodecahedron
2255:
2243:
2232:
2128:Stellations
2122:dodecahedron
2079:
2075:dodecahedron
2054:
2035:
2029:
2015:
2009:
1995:
1989:
1962:
1956:
1942:
1936:
1922:
1916:
1886:
1869:
1845:
1829:
1790:
1773:
1772:
1763:
1762:
1729:
1728:is just the
1715:
1711:
1706:
1571:(sgD = gsD)
713:
702:
563:
557:
554:
497:
464:
460:
422:
150:
129:pentagrammic
121:dodecahedron
116:the regular
111:
99:
93:
4213:(nonconvex
4047:Dual Models
3921:J. Bertrand
3707:4-polytopes
3657:icosahedron
3626:Gravitation
3462:John Conway
2806:cumulations
2280:icosahedron
2266:equivalent.
2239:stellations
2111:icosahedron
2065:icosahedron
2061:stellations
1903:greatening
1809:stellation
1716:stellations
1712:greatenings
1708:John Conway
720:edge radius
442:faces. The
125:icosahedron
4500:Categories
3949:Phil. Mag.
3837:2018-09-21
3805:2018-09-21
3714:References
3634:dissection
3436:stellating
3404:stellating
3153:animations
3129:animations
3105:animations
3079:animations
3055:animations
3031:animations
3001:{3, 5/2} (
2990:{5, 5/2} (
2763:0.38196...
2717:2.61803...
2648:0.61803...
2602:1.61803...
2244:(See also
2180:Facetings
1774:Greatening
1768:stellation
1764:Stellation
1148:Stellation
475:pentagonal
425:pentagrams
114:stellating
4250:polyhedra
4211:polyhedra
4124:MathWorld
3831:MathWorld
3799:MathWorld
3731:Coxeter,
3493:St Mark's
3418:In 1809,
3385:truncated
3182:(Compare
3174:midradius
2836:MathWorld
2782:midradius
2744:−
2633:−
2468:midradius
2438:φ
2425:φ
2405:φ
2260:skeletons
1800:midradius
1185:(config.)
1142:Spherical
652:−
566:) of the
522:−
513:χ
471:pentagram
440:pentagram
351:ϕ
278:ϕ
233:ϕ
160:ϕ
4045:(1983).
3667:See also
3448:faceting
3444:Bertrand
2979:{3, 5} (
2957:its dual
2947:its dual
2930:Symmetry
2853:and the
2820:hedgehog
2086:faceting
1976:duality
1787:diagram
1205:Symmetry
1173:Vertices
1155:Schläfli
1151:diagram
1139:Picture
985:decagram
96:geometry
3859:(ed.).
3755:Echinus
3361:In his
3342:History
3178:decagon
2951:In the
2387:regular
2235:regular
2101:Convex
2067:. (See
2063:of the
1720:In his
1696:density
1664:{10/3}
1547:{10/3}
1200:Density
1144:tiling
1127:Summary
842:hexagon
560:density
469:has 12
104:regular
4095:
4079:
4075:2008,
4053:
3989:
3972:
3968:2008,
3875:
3659:and a
3569:(1619)
3547:(1568)
3497:Venice
3489:mosaic
3487:Floor
3450:them.
3415:were.
3409:convex
3379:and a
3352:Venice
2830:These
2389:and a
1885:sgD =
1655:(5/2)
1638:{5/2}
1538:(3)/2
1421:(5/2)
1404:{5/2}
1304:(5)/2
1181:figure
1179:Vertex
1170:Edges
540:
452:convex
118:convex
3719:Notes
3583:from
2869:Core
2832:naïve
2488:Core
2482:Hull
2466:(The
2458:(See
2383:great
2379:small
2084:is a
1529:{5/2}
1454:(gI)
1337:(sD)
1295:{5/2}
1220:(gD)
1165:Faces
450:have
435:have
429:small
147:Sizes
132:faces
4093:ISBN
4077:ISBN
4051:ISBN
3987:ISBN
3970:ISBN
3873:ISBN
3537:and
3438:the
3197:and
2955:and
2945:and
2941:The
2804:(or
2381:and
2377:The
2258:The
2080:The
2055:The
2033:and
2013:and
1993:and
1960:and
1940:and
1920:and
1724:the
1714:and
1521:{3}
1430:{6}
1313:{6}
1287:{5}
1210:Dual
1175:{q}
1167:{p}
1134:Name
724:skew
705:dual
446:and
431:and
342:and
123:and
98:, a
3865:doi
3757:" (
3565:by
3541:in
3491:in
3195:gsD
3007:gsD
2822:).
2808:),
2223:gsD
2174:gsD
2036:gsD
1963:gsD
1887:gsD
1646:{3}
1412:{5}
134:or
94:In
4502::
4121:.
4071:,
3964:,
3952:17
3937:,
3929:46
3927:,
3871:.
3828:.
3796:.
3640:.
3632:A
3616:.
3495:,
3476:.
3358:.
3350:,
3199:gI
3193:,
3191:sD
3186:.)
3009:)
3003:gI
2998:)
2996:sD
2992:gD
2987:)
2248:)
2241:.
2212:sD
2201:gD
2190:gI
2163:sD
2152:gD
2138:gI
2077:.
2030:gI
2016:sD
2010:gD
1957:sD
1943:gI
1923:gD
1870:gD
1846:sD
1732:.
1644:20
1641:30
1636:12
1527:12
1524:30
1519:20
1433:−6
1410:12
1407:30
1402:12
1316:−6
1293:12
1290:30
1285:12
1000:10
458:.
382:11
109:.
4193:e
4186:t
4179:v
4127:.
4101:.
4059:.
4029:9
3881:.
3867::
3840:.
3808:.
3504:)
3367:(
3155:)
3151:(
3131:)
3127:(
3107:)
3103:(
3081:)
3077:(
3057:)
3053:(
3033:)
3029:(
2985:D
2981:I
2857:.
2784:.
2760:=
2755:2
2749:5
2741:3
2714:=
2709:2
2703:5
2698:+
2695:3
2645:=
2640:2
2636:1
2628:5
2599:=
2594:2
2590:1
2587:+
2582:5
2462:)
2442:2
2434:=
2431:1
2428:+
2071:)
1996:I
1990:D
1937:I
1917:D
1830:D
1802:.
1698:)
1675:h
1673:I
1670:7
1667:2
1558:h
1556:I
1553:7
1550:2
1441:h
1439:I
1436:3
1324:h
1322:I
1319:3
1195:χ
1016:}
1010:5
1007:,
1004:3
995:{
969:}
965:3
962:,
957:2
954:5
948:{
922:}
916:2
913:5
908:,
905:3
901:{
873:}
867:3
864:,
861:1
857:6
852:{
826:}
820:2
817:5
812:,
809:5
805:{
779:}
775:5
772:,
767:2
764:5
758:{
683:.
680:D
677:2
674:=
671:F
666:f
662:d
658:+
655:E
649:V
644:v
640:d
610:f
606:d
583:v
579:d
570:(
564:D
562:(
537:2
534:=
531:F
528:+
525:E
519:V
516:=
401:)
393:5
388:5
385:+
377:(
369:2
366:1
360:=
355:5
330:,
325:)
317:5
312:+
309:3
304:(
296:2
293:1
287:=
282:2
258:,
253:5
248:+
245:2
242:=
237:3
210:)
202:5
197:3
194:+
191:7
186:(
178:2
175:1
169:=
164:4
20:)
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.