1878:
1234:
1873:{\displaystyle {\begin{aligned}\sum _{k=1}^{n}k^{3}&=1+8+27+64+\cdots +n^{3}\\&=\underbrace {1} _{1^{3}}+\underbrace {3+5} _{2^{3}}+\underbrace {7+9+11} _{3^{3}}+\underbrace {13+15+17+19} _{4^{3}}+\cdots +\underbrace {\left(n^{2}-n+1\right)+\cdots +\left(n^{2}+n-1\right)} _{n^{3}}\\&=\underbrace {\underbrace {\underbrace {\underbrace {1} _{1^{2}}+3} _{2^{2}}+5} _{3^{2}}+\cdots +\left(n^{2}+n-1\right)} _{\left({\frac {n^{2}+n}{2}}\right)^{2}}\\&=(1+2+\cdots +n)^{2}\\&=\left(\sum _{k=1}^{n}k\right)^{2}.\end{aligned}}}
533:
27:
4927:
1905:
1027:
798:
of these two triangles, so its size is the square of a triangular number on the right hand side of the
Nichomachus identity. The probabilities themselves are respectively the left and right sides of the Nichomachus identity, normalized to make probabilities by dividing both sides
332:, pointed out that if one writes a list of the odd numbers, the first is the cube of 1, the sum of the next two is the cube of 2, the sum of the next three is the cube of 3, and so on. He does not go further than this, but from this it follows that the sum of the first
823:
184:
289:
2106:
1139:
1239:
2441:
626:
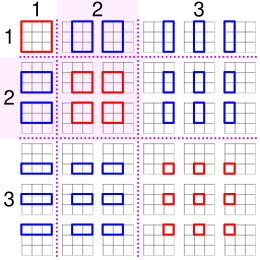
grid (or a square made up of three smaller squares on a side) can form 36 different rectangles. The number of squares in a square grid is similarly counted by the square pyramidal numbers.
1229:
1897:
times a triangular number, from which it follows that the sum of all the rows is the square of a triangular number. Alternatively, one can decompose the table into a sequence of nested
1901:, each consisting of the products in which the larger of the two terms is some fixed value. The sum within each gmonon is a cube, so the sum of the whole table is a sum of cubes.
1022:{\displaystyle n^{3}=\underbrace {\left(n^{2}-n+1\right)+\left(n^{2}-n+1+2\right)+\left(n^{2}-n+1+4\right)+\cdots +\left(n^{2}+n-1\right)} _{n{\text{ consecutive odd numbers}}}.}
3029:
34:. The nth coloured region shows n squares of dimension n by n (the rectangle is 1 evenly divided square), hence the area of the nth region is n times n x n.
2664:
2573:
67:
2000:
820:) gives a particularly simple derivation, by expanding each cube in the sum into a set of consecutive odd numbers. He begins by giving the identity
3022:
1046:
2513:
2349:
199:
1974:
study polynomial analogues of the square triangular number formula, in which series of polynomials add to the square of another polynomial.
2375:
3829:
3015:
2633:
2533:
2266:
3824:
1960:, of which the sum of cubes is the simplest and most elegant example. However, in no other case is one power sum a square of another.
3839:
3819:
1162:
30:
A square whose side length is a triangular number can be partitioned into squares and half-squares whose areas add to cubes. From
4532:
4112:
2039:
3834:
2657:
4618:
4961:
3934:
4966:
4284:
3603:
3396:
2842:
2392:
4319:
4289:
3964:
3954:
4460:
3874:
3608:
3588:
2847:
2827:
2160:
2115:
4150:
4314:
4956:
4409:
4032:
3789:
3598:
3580:
3474:
3464:
3454:
2837:
2819:
2718:
2708:
2698:
794:
form isosceles right triangles, and the set counted by the right hand side of the equation of probabilities is the
4294:
4537:
4082:
3489:
3484:
3479:
3469:
3446:
2733:
2728:
2723:
2713:
2690:
2650:
1924:
uses the rectangle-counting interpretation of these numbers to form a geometric proof of the identity (see also
3522:
2212:
20:
3779:
2309:
Kanim, Katherine (2004), "Proofs without words: The sum of cubes—An extension of
Archimedes' sum of squares",
4648:
4613:
4399:
4309:
4183:
4158:
4067:
4057:
3669:
3651:
3571:
2908:
2885:
2810:
609:
observes, these numbers also count the number of rectangles with horizontal and vertical sides formed in an
599:
545:
1956:, namely that odd power sums (sums of odd powers) are a polynomial in triangular numbers. These are called
4951:
4908:
4178:
4052:
3683:
3459:
3239:
3166:
2922:
2703:
2480:
1957:
1953:
295:
2381:
4163:
4017:
3944:
3099:
2977:
4872:
4512:
1966:
studies more general conditions under which the sum of a consecutive sequence of cubes forms a square.
4805:
4699:
4663:
4404:
4127:
4107:
3924:
3593:
3381:
3353:
2832:
2582:
2445:
2311:
1928:); he observes that it may also be proved easily (but uninformatively) by induction, and states that
1886:
2298:
4527:
4391:
4386:
4354:
4117:
4092:
4087:
4062:
3992:
3988:
3919:
3809:
3641:
3437:
3406:
2875:
2681:
514:
4926:
4930:
4684:
4679:
4593:
4567:
4465:
4444:
4216:
4097:
4047:
3969:
3939:
3879:
3646:
3626:
3557:
3270:
2903:
2880:
2796:
2564:
2524:
2462:
2328:
2245:
2229:
2207:
2177:
2144:
2132:
2035:
2031:
1917:
1030:
813:
3814:
2257:
750:
is largest is a sum of cubes, the left hand side of the
Nichomachus identity. The sets of pairs
460:
claims that "every student of number theory surely must have marveled at this miraculous fact".
4824:
4769:
4623:
4598:
4572:
4349:
4027:
4022:
3949:
3929:
3914:
3636:
3618:
3537:
3527:
3512:
3290:
3275:
2870:
2857:
2776:
2766:
2756:
2613:
2509:
2345:
795:
595:
532:
58:
2630:
4860:
4653:
4239:
4211:
4201:
4193:
4077:
4042:
4037:
4004:
3698:
3661:
3552:
3547:
3542:
3532:
3504:
3391:
3343:
3338:
3295:
3234:
2937:
2895:
2791:
2786:
2781:
2771:
2748:
2590:
2542:
2454:
2359:
Pengelley, David (2002), "The bridge between continuous and discrete via original sources",
2320:
2275:
2221:
2169:
2124:
2556:
2493:
2289:
2241:
4836:
4725:
4658:
4584:
4507:
4481:
4299:
4012:
3869:
3804:
3774:
3764:
3759:
3425:
3333:
3280:
3124:
3064:
2673:
2637:
2552:
2489:
2285:
2237:
2152:
1898:
591:
2617:
2586:
4841:
4709:
4694:
4558:
4522:
4497:
4373:
4344:
4329:
4206:
4102:
4072:
3799:
3754:
3631:
3229:
3224:
3219:
3191:
3176:
3089:
3074:
3052:
3039:
2865:
2189:
2148:
619:
46:
2475:
26:
4945:
4764:
4748:
4689:
4643:
4339:
4324:
4234:
3959:
3517:
3386:
3348:
3305:
3186:
3171:
3161:
3119:
3109:
3084:
2987:
2761:
2501:
2249:
50:
39:
456:
Many early mathematicians have studied and provided proofs of
Nicomachus's theorem.
189:
The same equation may be written more compactly using the mathematical notation for
4800:
4789:
4704:
4542:
4517:
4434:
4334:
4304:
4279:
4263:
4168:
4135:
3884:
3858:
3769:
3708:
3285:
3181:
3114:
3094:
3069:
2992:
2947:
2193:
579:
1908:
Visual demonstration that the square of a triangular number equals a sum of cubes.
179:{\displaystyle 1^{3}+2^{3}+3^{3}+\cdots +n^{3}=\left(1+2+3+\cdots +n\right)^{2}.}
4759:
4634:
4439:
3903:
3794:
3749:
3744:
3494:
3401:
3300:
3129:
3104:
3079:
2982:
2738:
1990:
629:
The identity also admits a natural probabilistic interpretation as follows. Let
575:
4896:
4877:
4173:
3784:
2360:
571:
567:
563:
503:
465:
303:
3007:
647:
be four integer numbers independently and uniformly chosen at random between
4502:
4429:
4421:
4226:
4140:
3258:
2962:
2622:
499:
481:
473:
190:
2595:
1904:
4603:
498:
mentions several additional early mathematical works on this formula, by
2368:, National Center for Mathematics Education, Univ. of Gothenburg, Sweden
2181:
4608:
4267:
2466:
2332:
2233:
2136:
284:{\displaystyle \sum _{k=1}^{n}k^{3}=\left(\sum _{k=1}^{n}k\right)^{2}.}
2173:
491:
469:
2568:
2458:
2324:
2225:
2128:
2642:
2547:
2280:
1903:
1159:. Applying this property, along with another well-known identity:
531:
477:
25:
4894:
4858:
4822:
4786:
4746:
4371:
4260:
3986:
3901:
3856:
3733:
3423:
3370:
3322:
3256:
3208:
3146:
3050:
3011:
2646:
659:
is the largest of the four numbers equals the probability that
2569:"On the formation of powers from arithmetical progressions"
1994:
528:
Numeric values; geometric and probabilistic interpretation
594:, a four-dimensional hyperpyramidal generalization of the
464:
finds references to the identity not only in the works of
2101:{\displaystyle \textstyle \sum k^{3}={n+1 \choose 2}^{2}}
1885:
obtains another proof by summing the numbers in a square
2476:"On the sum of consecutive cubes being a perfect square"
1134:{\displaystyle n^{3}=\sum _{k=T_{n-1}+1}^{T_{n}}(2k-1),}
1952:
A similar result to
Nicomachus's theorem holds for all
1147:
start off just after those forming all previous values
19:
For triangular numbers that are themselves square, see
2396:
2043:
2395:
2389:
Stein, Robert G. (1971), "A combinatorial proof that
2042:
1237:
1165:
1049:
826:
724:
so (adding the size of this cube over all choices of
202:
70:
4718:
4672:
4632:
4583:
4557:
4490:
4474:
4453:
4420:
4385:
4225:
4192:
4149:
4126:
4003:
3691:
3682:
3660:
3617:
3579:
3570:
3503:
3445:
3436:
2970:
2960:
2930:
2921:
2894:
2856:
2818:
2809:
2747:
2689:
2680:
524:, India); he reproduces Nilakantha's visual proof.
2436:{\displaystyle \textstyle \sum k^{3}=(\sum k)^{2}}
2435:
2100:
1925:
1872:
1223:
1133:
1021:
283:
178:
2297:Gulley, Ned (March 4, 2010), Shure, Loren (ed.),
2210:(1957), "Sums of powers of the natural numbers",
361:odd numbers, that is, the odd numbers from 1 to
1937:
2256:Garrett, Kristina C.; Hummel, Kristen (2004),
559:The sequence of squared triangular numbers is
3023:
2658:
2362:Study the Masters: The Abel-Fauvel Conference
2085:
2064:
1967:
585:441, 784, 1296, 2025, 3025, 4356, 6084, 8281,
8:
2195:Calculus before Newton and Leibniz, Part III
1932:provides "an interesting old Arabic proof".
1912:In the more recent mathematical literature,
1224:{\displaystyle n^{2}=\sum _{k=1}^{n}(2k-1),}
472:in the 1st century CE, but also in those of
372:. The average of these numbers is obviously
328:Nicomachus, at the end of Chapter 20 of his
4891:
4855:
4819:
4783:
4743:
4417:
4382:
4368:
4257:
4000:
3983:
3898:
3853:
3730:
3688:
3576:
3442:
3433:
3420:
3367:
3324:Possessing a specific set of other numbers
3319:
3253:
3205:
3143:
3047:
3030:
3016:
3008:
2967:
2927:
2815:
2686:
2665:
2651:
2643:
2574:Proceedings of the Royal Society of London
817:
2594:
2546:
2426:
2404:
2394:
2279:
2091:
2084:
2063:
2061:
2051:
2041:
2001:On-Line Encyclopedia of Integer Sequences
1857:
1843:
1832:
1806:
1760:
1738:
1731:
1725:
1696:
1670:
1665:
1645:
1640:
1620:
1615:
1605:
1601:
1597:
1593:
1574:
1569:
1540:
1499:
1487:
1469:
1464:
1434:
1422:
1417:
1393:
1381:
1376:
1358:
1346:
1341:
1331:
1314:
1267:
1257:
1246:
1238:
1236:
1194:
1183:
1170:
1164:
1102:
1097:
1078:
1067:
1054:
1048:
1009:
1005:
976:
929:
888:
853:
841:
831:
825:
461:
272:
258:
247:
228:
218:
207:
201:
167:
120:
101:
88:
75:
69:
1963:
1929:
495:
457:
2014:
1982:
1971:
1913:
2631:A visual proof of Nicomachus's theorem
2153:"Summing cubes by counting rectangles"
1941:
1889:in two different ways. The sum of the
31:
2258:"A combinatorial proof of the sum of
1933:
1921:
606:
7:
2377:Geometric Exercises in Paper Folding
480:in the 5th century, and in those of
2534:Electronic Journal of Combinatorics
2267:Electronic Journal of Combinatorics
2036:"Two quick combinatorial proofs of
1940:provide two additional proofs, and
1882:
1231:produces the following derivation:
552:(red), in a 3 × 3 square
2068:
336:cubes equals the sum of the first
14:
4925:
4533:Perfect digit-to-digit invariant
2506:The Calculus, a Genetic Approach
1936:provides a purely visual proof,
1926:Benjamin, Quinn & Wurtz 2006
728:) the number of combinations of
622:. For instance, the points of a
2508:, University of Chicago Press,
590:These numbers can be viewed as
2529:-analogue of the sum of cubes"
2423:
2413:
2344:, Cambridge University Press,
1944:gives seven geometric proofs.
1803:
1778:
1215:
1200:
1141:and thus the summands forming
1125:
1110:
685:. For any particular value of
1:
3372:Expressible via specific sums
2843:Centered dodecahedral numbers
1938:Benjamin & Orrison (2002)
1011: consecutive odd numbers
655:. Then, the probability that
554:(4 × 4 vertex) grid
518:
507:
484:
314:
307:
16:Square of a triangular number
2848:Centered icosahedral numbers
2828:Centered tetrahedral numbers
1029:That identity is related to
4461:Multiplicative digital root
2838:Centered octahedral numbers
2719:Centered heptagonal numbers
2709:Centered pentagonal numbers
2699:Centered triangular numbers
2161:College Mathematics Journal
2116:College Mathematics Journal
1968:Garrett & Hummel (2004)
4983:
2943:Squared triangular numbers
2734:Centered decagonal numbers
2729:Centered nonagonal numbers
2724:Centered octagonal numbers
2714:Centered hexagonal numbers
1991:Sloane, N. J. A.
330:Introduction to Arithmetic
18:
4921:
4904:
4890:
4868:
4854:
4832:
4818:
4796:
4782:
4755:
4742:
4538:Perfect digital invariant
4381:
4367:
4275:
4256:
4113:Superior highly composite
3999:
3982:
3910:
3897:
3865:
3852:
3740:
3729:
3432:
3419:
3377:
3366:
3329:
3318:
3266:
3252:
3215:
3204:
3157:
3142:
3060:
3046:
2340:Nelsen, Roger B. (1993),
2151:; Wurtz, Calyssa (2006),
2034:; Orrison, M. E. (2002),
422:of them, so their sum is
4151:Euler's totient function
3935:Euler–Jacobi pseudoprime
3210:Other polynomial numbers
2909:Square pyramidal numbers
2886:Stella octangula numbers
2523:Warnaar, S. Ole (2004),
2474:Stroeker, R. J. (1995),
2374:Row, T. Sundara (1893),
2213:The Mathematical Gazette
671:is at least as large as
663:is at least as large as
600:square pyramidal numbers
544:) rectangles, including
21:square triangular number
3965:Somer–Lucas pseudoprime
3955:Lucas–Carmichael number
3790:Lazy caterer's sequence
2704:Centered square numbers
2274:(1), Research Paper 9,
1995:"Sequence A000537"
1916:provides a proof using
502:(10th century Arabia),
42:, the sum of the first
3840:Wedderburn–Etherington
3240:Lucky numbers of Euler
2618:"Nicomachus's theorem"
2596:10.1098/rspl.1854.0036
2481:Compositio Mathematica
2437:
2102:
1909:
1874:
1848:
1262:
1225:
1199:
1135:
1109:
1043:in the following way:
1023:
814:Charles Wheatstone
689:, the combinations of
556:
285:
263:
223:
180:
35:
4128:Prime omega functions
3945:Frobenius pseudoprime
3735:Combinatorial numbers
3604:Centered dodecahedral
3397:Primary pseudoperfect
2833:Centered cube numbers
2438:
2103:
1958:Faulhaber polynomials
1907:
1875:
1828:
1242:
1226:
1179:
1136:
1063:
1024:
535:
286:
243:
203:
181:
29:
4962:Algebraic identities
4587:-composition related
4387:Arithmetic functions
3989:Arithmetic functions
3925:Elliptic pseudoprime
3609:Centered icosahedral
3589:Centered tetrahedral
2876:Dodecahedral numbers
2446:Mathematics Magazine
2393:
2342:Proofs without Words
2312:Mathematics Magazine
2300:Nicomachus's Theorem
2040:
1887:multiplication table
1235:
1163:
1047:
824:
705:largest form a cube
304:Nicomachus of Gerasa
300:Nicomachus's theorem
298:is sometimes called
200:
68:
4967:Proof without words
4513:Kaprekar's constant
4033:Colossally abundant
3920:Catalan pseudoprime
3820:Schröder–Hipparchus
3599:Centered octahedral
3475:Centered heptagonal
3465:Centered pentagonal
3455:Centered triangular
3055:and related numbers
2993:8-hypercube numbers
2988:7-hypercube numbers
2983:6-hypercube numbers
2978:5-hypercube numbers
2948:Tesseractic numbers
2904:Tetrahedral numbers
2881:Icosahedral numbers
2797:Dodecagonal numbers
2587:1854RSPS....7..145W
2380:, Madras: Addison,
2145:Benjamin, Arthur T.
2032:Benjamin, Arthur T.
515:Nilakantha Somayaji
4931:Mathematics portal
4873:Aronson's sequence
4619:Smarandache–Wellin
4376:-dependent numbers
4083:Primitive abundant
3970:Strong pseudoprime
3960:Perrin pseudoprime
3940:Fermat pseudoprime
3880:Wolstenholme prime
3704:Squared triangular
3490:Centered decagonal
3485:Centered nonagonal
3480:Centered octagonal
3470:Centered hexagonal
2871:Octahedral numbers
2777:Heptagonal numbers
2767:Pentagonal numbers
2757:Triangular numbers
2636:2019-10-19 at the
2614:Weisstein, Eric W.
2433:
2432:
2208:Edmonds, Sheila M.
2149:Quinn, Jennifer J.
2098:
2097:
1918:summation by parts
1910:
1870:
1868:
1767:
1723:
1677:
1663:
1652:
1638:
1627:
1613:
1581:
1567:
1476:
1462:
1429:
1415:
1388:
1374:
1353:
1339:
1221:
1131:
1031:triangular numbers
1019:
1015:
1003:
596:triangular numbers
557:
281:
176:
36:
4957:Integer sequences
4939:
4938:
4917:
4916:
4886:
4885:
4850:
4849:
4814:
4813:
4778:
4777:
4738:
4737:
4734:
4733:
4553:
4552:
4363:
4362:
4252:
4251:
4248:
4247:
4194:Aliquot sequences
4005:Divisor functions
3978:
3977:
3950:Lucas pseudoprime
3930:Euler pseudoprime
3915:Carmichael number
3893:
3892:
3848:
3847:
3725:
3724:
3721:
3720:
3717:
3716:
3678:
3677:
3566:
3565:
3523:Square triangular
3415:
3414:
3362:
3361:
3314:
3313:
3248:
3247:
3200:
3199:
3138:
3137:
3005:
3004:
3001:
3000:
2956:
2955:
2938:Pentatope numbers
2917:
2916:
2805:
2804:
2792:Decagonal numbers
2787:Nonagonal numbers
2782:Octagonal numbers
2772:Hexagonal numbers
2515:978-0-226-80667-9
2351:978-0-88385-700-7
2083:
2004:, OEIS Foundation
1754:
1606:
1604:
1602:
1600:
1598:
1596:
1594:
1592:
1488:
1486:
1435:
1433:
1394:
1392:
1359:
1357:
1332:
1330:
1012:
842:
840:
796:Cartesian product
59:triangular number
4974:
4929:
4892:
4861:Natural language
4856:
4820:
4788:Generated via a
4784:
4744:
4649:Digit-reassembly
4614:Self-descriptive
4418:
4383:
4369:
4320:Lucas–Carmichael
4310:Harmonic divisor
4258:
4184:Sparsely totient
4159:Highly cototient
4068:Multiply perfect
4058:Highly composite
4001:
3984:
3899:
3854:
3835:Telephone number
3731:
3689:
3670:Square pyramidal
3652:Stella octangula
3577:
3443:
3434:
3426:Figurate numbers
3421:
3368:
3320:
3254:
3206:
3144:
3048:
3032:
3025:
3018:
3009:
2968:
2928:
2816:
2687:
2674:Figurate numbers
2667:
2660:
2653:
2644:
2627:
2626:
2599:
2598:
2559:
2550:
2528:
2518:
2496:
2488:(1–2): 295–307,
2469:
2442:
2440:
2439:
2434:
2431:
2430:
2409:
2408:
2384:
2369:
2367:
2354:
2335:
2304:
2303:, Matlab Central
2292:
2283:
2261:
2252:
2220:(337): 187–188,
2202:
2200:
2184:
2174:10.2307/27646391
2157:
2139:
2112:
2107:
2105:
2104:
2099:
2096:
2095:
2090:
2089:
2088:
2079:
2067:
2056:
2055:
2018:
2012:
2006:
2005:
1987:
1896:
1892:
1879:
1877:
1876:
1871:
1869:
1862:
1861:
1856:
1852:
1847:
1842:
1815:
1811:
1810:
1771:
1766:
1765:
1764:
1759:
1755:
1750:
1743:
1742:
1732:
1724:
1719:
1718:
1714:
1701:
1700:
1676:
1675:
1674:
1664:
1659:
1651:
1650:
1649:
1639:
1634:
1626:
1625:
1624:
1614:
1585:
1580:
1579:
1578:
1568:
1563:
1562:
1558:
1545:
1544:
1521:
1517:
1504:
1503:
1475:
1474:
1473:
1463:
1458:
1428:
1427:
1426:
1416:
1411:
1387:
1386:
1385:
1375:
1370:
1352:
1351:
1350:
1340:
1323:
1319:
1318:
1272:
1271:
1261:
1256:
1230:
1228:
1227:
1222:
1198:
1193:
1175:
1174:
1158:
1150:
1146:
1140:
1138:
1137:
1132:
1108:
1107:
1106:
1096:
1089:
1088:
1059:
1058:
1042:
1028:
1026:
1025:
1020:
1014:
1013:
1010:
1004:
999:
998:
994:
981:
980:
957:
953:
934:
933:
916:
912:
893:
892:
875:
871:
858:
857:
836:
835:
804:
793:
783:
771:
761:
749:
745:
727:
723:
704:
700:
696:
692:
688:
684:
674:
670:
666:
662:
658:
654:
650:
646:
625:
618:
592:figurate numbers
586:
583:
555:
549:
543:
539:
523:
520:
512:
509:
489:
486:
462:Pengelley (2002)
452:
448:
446:
445:
442:
439:
421:
420:
418:
417:
414:
411:
397:, and there are
396:
395:
393:
392:
389:
386:
371:
360:
359:
357:
356:
353:
350:
335:
319:
316:
312:
309:
290:
288:
287:
282:
277:
276:
271:
267:
262:
257:
233:
232:
222:
217:
185:
183:
182:
177:
172:
171:
166:
162:
125:
124:
106:
105:
93:
92:
80:
79:
56:
45:
4982:
4981:
4977:
4976:
4975:
4973:
4972:
4971:
4942:
4941:
4940:
4935:
4913:
4909:Strobogrammatic
4900:
4882:
4864:
4846:
4828:
4810:
4792:
4774:
4751:
4730:
4714:
4673:Divisor-related
4668:
4628:
4579:
4549:
4486:
4470:
4449:
4416:
4389:
4377:
4359:
4271:
4270:related numbers
4244:
4221:
4188:
4179:Perfect totient
4145:
4122:
4053:Highly abundant
3995:
3974:
3906:
3889:
3861:
3844:
3830:Stirling second
3736:
3713:
3674:
3656:
3613:
3562:
3499:
3460:Centered square
3428:
3411:
3373:
3358:
3325:
3310:
3262:
3261:defined numbers
3244:
3211:
3196:
3167:Double Mersenne
3153:
3134:
3056:
3042:
3040:natural numbers
3036:
3006:
2997:
2952:
2913:
2890:
2852:
2801:
2743:
2676:
2671:
2638:Wayback Machine
2612:
2611:
2608:
2603:
2563:
2526:
2522:
2516:
2500:
2473:
2459:10.2307/2688231
2422:
2400:
2391:
2390:
2388:
2373:
2365:
2358:
2352:
2339:
2325:10.2307/3219288
2308:
2296:
2259:
2255:
2226:10.2307/3609189
2206:
2198:
2190:Bressoud, David
2188:
2155:
2143:
2129:10.2307/1559017
2110:
2069:
2062:
2060:
2047:
2038:
2037:
2030:
2026:
2021:
2013:
2009:
1989:
1988:
1984:
1980:
1964:Stroeker (1995)
1950:
1948:Generalizations
1930:Toeplitz (1963)
1894:
1890:
1867:
1866:
1827:
1823:
1822:
1813:
1812:
1802:
1769:
1768:
1734:
1733:
1727:
1726:
1692:
1691:
1687:
1666:
1641:
1616:
1603:
1599:
1595:
1583:
1582:
1570:
1536:
1535:
1531:
1495:
1494:
1490:
1489:
1465:
1436:
1418:
1395:
1377:
1360:
1342:
1321:
1320:
1310:
1273:
1263:
1233:
1232:
1166:
1161:
1160:
1152:
1148:
1142:
1098:
1074:
1050:
1045:
1044:
1041:
1033:
972:
971:
967:
925:
924:
920:
884:
883:
879:
849:
848:
844:
843:
827:
822:
821:
811:
800:
785:
773:
763:
751:
747:
729:
725:
706:
702:
698:
694:
690:
686:
676:
672:
668:
664:
660:
656:
652:
648:
630:
623:
610:
588:
584:
562:
553:
547:
541:
537:
530:
521:
513:, France), and
510:
496:Bressoud (2004)
487:
468:in what is now
458:Stroeker (1995)
451:
443:
440:
430:
429:
427:
426:
423:
415:
412:
402:
401:
399:
398:
390:
387:
377:
376:
374:
373:
362:
354:
351:
341:
340:
338:
337:
333:
326:
317:
310:
242:
238:
237:
224:
198:
197:
134:
130:
129:
116:
97:
84:
71:
66:
65:
54:
43:
24:
17:
12:
11:
5:
4980:
4978:
4970:
4969:
4964:
4959:
4954:
4944:
4943:
4937:
4936:
4934:
4933:
4922:
4919:
4918:
4915:
4914:
4912:
4911:
4905:
4902:
4901:
4895:
4888:
4887:
4884:
4883:
4881:
4880:
4875:
4869:
4866:
4865:
4859:
4852:
4851:
4848:
4847:
4845:
4844:
4842:Sorting number
4839:
4837:Pancake number
4833:
4830:
4829:
4823:
4816:
4815:
4812:
4811:
4809:
4808:
4803:
4797:
4794:
4793:
4787:
4780:
4779:
4776:
4775:
4773:
4772:
4767:
4762:
4756:
4753:
4752:
4749:Binary numbers
4747:
4740:
4739:
4736:
4735:
4732:
4731:
4729:
4728:
4722:
4720:
4716:
4715:
4713:
4712:
4707:
4702:
4697:
4692:
4687:
4682:
4676:
4674:
4670:
4669:
4667:
4666:
4661:
4656:
4651:
4646:
4640:
4638:
4630:
4629:
4627:
4626:
4621:
4616:
4611:
4606:
4601:
4596:
4590:
4588:
4581:
4580:
4578:
4577:
4576:
4575:
4564:
4562:
4559:P-adic numbers
4555:
4554:
4551:
4550:
4548:
4547:
4546:
4545:
4535:
4530:
4525:
4520:
4515:
4510:
4505:
4500:
4494:
4492:
4488:
4487:
4485:
4484:
4478:
4476:
4475:Coding-related
4472:
4471:
4469:
4468:
4463:
4457:
4455:
4451:
4450:
4448:
4447:
4442:
4437:
4432:
4426:
4424:
4415:
4414:
4413:
4412:
4410:Multiplicative
4407:
4396:
4394:
4379:
4378:
4374:Numeral system
4372:
4365:
4364:
4361:
4360:
4358:
4357:
4352:
4347:
4342:
4337:
4332:
4327:
4322:
4317:
4312:
4307:
4302:
4297:
4292:
4287:
4282:
4276:
4273:
4272:
4261:
4254:
4253:
4250:
4249:
4246:
4245:
4243:
4242:
4237:
4231:
4229:
4223:
4222:
4220:
4219:
4214:
4209:
4204:
4198:
4196:
4190:
4189:
4187:
4186:
4181:
4176:
4171:
4166:
4164:Highly totient
4161:
4155:
4153:
4147:
4146:
4144:
4143:
4138:
4132:
4130:
4124:
4123:
4121:
4120:
4115:
4110:
4105:
4100:
4095:
4090:
4085:
4080:
4075:
4070:
4065:
4060:
4055:
4050:
4045:
4040:
4035:
4030:
4025:
4020:
4018:Almost perfect
4015:
4009:
4007:
3997:
3996:
3987:
3980:
3979:
3976:
3975:
3973:
3972:
3967:
3962:
3957:
3952:
3947:
3942:
3937:
3932:
3927:
3922:
3917:
3911:
3908:
3907:
3902:
3895:
3894:
3891:
3890:
3888:
3887:
3882:
3877:
3872:
3866:
3863:
3862:
3857:
3850:
3849:
3846:
3845:
3843:
3842:
3837:
3832:
3827:
3825:Stirling first
3822:
3817:
3812:
3807:
3802:
3797:
3792:
3787:
3782:
3777:
3772:
3767:
3762:
3757:
3752:
3747:
3741:
3738:
3737:
3734:
3727:
3726:
3723:
3722:
3719:
3718:
3715:
3714:
3712:
3711:
3706:
3701:
3695:
3693:
3686:
3680:
3679:
3676:
3675:
3673:
3672:
3666:
3664:
3658:
3657:
3655:
3654:
3649:
3644:
3639:
3634:
3629:
3623:
3621:
3615:
3614:
3612:
3611:
3606:
3601:
3596:
3591:
3585:
3583:
3574:
3568:
3567:
3564:
3563:
3561:
3560:
3555:
3550:
3545:
3540:
3535:
3530:
3525:
3520:
3515:
3509:
3507:
3501:
3500:
3498:
3497:
3492:
3487:
3482:
3477:
3472:
3467:
3462:
3457:
3451:
3449:
3440:
3430:
3429:
3424:
3417:
3416:
3413:
3412:
3410:
3409:
3404:
3399:
3394:
3389:
3384:
3378:
3375:
3374:
3371:
3364:
3363:
3360:
3359:
3357:
3356:
3351:
3346:
3341:
3336:
3330:
3327:
3326:
3323:
3316:
3315:
3312:
3311:
3309:
3308:
3303:
3298:
3293:
3288:
3283:
3278:
3273:
3267:
3264:
3263:
3257:
3250:
3249:
3246:
3245:
3243:
3242:
3237:
3232:
3227:
3222:
3216:
3213:
3212:
3209:
3202:
3201:
3198:
3197:
3195:
3194:
3189:
3184:
3179:
3174:
3169:
3164:
3158:
3155:
3154:
3147:
3140:
3139:
3136:
3135:
3133:
3132:
3127:
3122:
3117:
3112:
3107:
3102:
3097:
3092:
3087:
3082:
3077:
3072:
3067:
3061:
3058:
3057:
3051:
3044:
3043:
3037:
3035:
3034:
3027:
3020:
3012:
3003:
3002:
2999:
2998:
2996:
2995:
2990:
2985:
2980:
2974:
2972:
2965:
2958:
2957:
2954:
2953:
2951:
2950:
2945:
2940:
2934:
2932:
2925:
2919:
2918:
2915:
2914:
2912:
2911:
2906:
2900:
2898:
2892:
2891:
2889:
2888:
2883:
2878:
2873:
2868:
2862:
2860:
2854:
2853:
2851:
2850:
2845:
2840:
2835:
2830:
2824:
2822:
2813:
2807:
2806:
2803:
2802:
2800:
2799:
2794:
2789:
2784:
2779:
2774:
2769:
2764:
2762:Square numbers
2759:
2753:
2751:
2745:
2744:
2742:
2741:
2736:
2731:
2726:
2721:
2716:
2711:
2706:
2701:
2695:
2693:
2684:
2678:
2677:
2672:
2670:
2669:
2662:
2655:
2647:
2641:
2640:
2628:
2607:
2606:External links
2604:
2602:
2601:
2565:Wheatstone, C.
2561:
2541:(1), Note 13,
2520:
2514:
2502:Toeplitz, Otto
2498:
2471:
2453:(3): 161–162,
2429:
2425:
2421:
2418:
2415:
2412:
2407:
2403:
2399:
2386:
2371:
2356:
2350:
2337:
2319:(4): 298–299,
2306:
2294:
2253:
2204:
2186:
2168:(5): 387–389,
2141:
2123:(5): 406–408,
2094:
2087:
2082:
2078:
2075:
2072:
2066:
2059:
2054:
2050:
2046:
2027:
2025:
2022:
2020:
2019:
2015:Edmonds (1957)
2007:
1981:
1979:
1976:
1972:Warnaar (2004)
1949:
1946:
1914:Edmonds (1957)
1865:
1860:
1855:
1851:
1846:
1841:
1838:
1835:
1831:
1826:
1821:
1818:
1816:
1814:
1809:
1805:
1801:
1798:
1795:
1792:
1789:
1786:
1783:
1780:
1777:
1774:
1772:
1770:
1763:
1758:
1753:
1749:
1746:
1741:
1737:
1730:
1722:
1717:
1713:
1710:
1707:
1704:
1699:
1695:
1690:
1686:
1683:
1680:
1673:
1669:
1662:
1658:
1655:
1648:
1644:
1637:
1633:
1630:
1623:
1619:
1612:
1609:
1591:
1588:
1586:
1584:
1577:
1573:
1566:
1561:
1557:
1554:
1551:
1548:
1543:
1539:
1534:
1530:
1527:
1524:
1520:
1516:
1513:
1510:
1507:
1502:
1498:
1493:
1485:
1482:
1479:
1472:
1468:
1461:
1457:
1454:
1451:
1448:
1445:
1442:
1439:
1432:
1425:
1421:
1414:
1410:
1407:
1404:
1401:
1398:
1391:
1384:
1380:
1373:
1369:
1366:
1363:
1356:
1349:
1345:
1338:
1335:
1329:
1326:
1324:
1322:
1317:
1313:
1309:
1306:
1303:
1300:
1297:
1294:
1291:
1288:
1285:
1282:
1279:
1276:
1274:
1270:
1266:
1260:
1255:
1252:
1249:
1245:
1241:
1240:
1220:
1217:
1214:
1211:
1208:
1205:
1202:
1197:
1192:
1189:
1186:
1182:
1178:
1173:
1169:
1130:
1127:
1124:
1121:
1118:
1115:
1112:
1105:
1101:
1095:
1092:
1087:
1084:
1081:
1077:
1073:
1070:
1066:
1062:
1057:
1053:
1037:
1018:
1008:
1002:
997:
993:
990:
987:
984:
979:
975:
970:
966:
963:
960:
956:
952:
949:
946:
943:
940:
937:
932:
928:
923:
919:
915:
911:
908:
905:
902:
899:
896:
891:
887:
882:
878:
874:
870:
867:
864:
861:
856:
852:
847:
839:
834:
830:
810:
807:
561:
529:
526:
449:
424:
325:
322:
292:
291:
280:
275:
270:
266:
261:
256:
253:
250:
246:
241:
236:
231:
227:
221:
216:
213:
210:
206:
187:
186:
175:
170:
165:
161:
158:
155:
152:
149:
146:
143:
140:
137:
133:
128:
123:
119:
115:
112:
109:
104:
100:
96:
91:
87:
83:
78:
74:
15:
13:
10:
9:
6:
4:
3:
2:
4979:
4968:
4965:
4963:
4960:
4958:
4955:
4953:
4952:Number theory
4950:
4949:
4947:
4932:
4928:
4924:
4923:
4920:
4910:
4907:
4906:
4903:
4898:
4893:
4889:
4879:
4876:
4874:
4871:
4870:
4867:
4862:
4857:
4853:
4843:
4840:
4838:
4835:
4834:
4831:
4826:
4821:
4817:
4807:
4804:
4802:
4799:
4798:
4795:
4791:
4785:
4781:
4771:
4768:
4766:
4763:
4761:
4758:
4757:
4754:
4750:
4745:
4741:
4727:
4724:
4723:
4721:
4717:
4711:
4708:
4706:
4703:
4701:
4700:Polydivisible
4698:
4696:
4693:
4691:
4688:
4686:
4683:
4681:
4678:
4677:
4675:
4671:
4665:
4662:
4660:
4657:
4655:
4652:
4650:
4647:
4645:
4642:
4641:
4639:
4636:
4631:
4625:
4622:
4620:
4617:
4615:
4612:
4610:
4607:
4605:
4602:
4600:
4597:
4595:
4592:
4591:
4589:
4586:
4582:
4574:
4571:
4570:
4569:
4566:
4565:
4563:
4560:
4556:
4544:
4541:
4540:
4539:
4536:
4534:
4531:
4529:
4526:
4524:
4521:
4519:
4516:
4514:
4511:
4509:
4506:
4504:
4501:
4499:
4496:
4495:
4493:
4489:
4483:
4480:
4479:
4477:
4473:
4467:
4464:
4462:
4459:
4458:
4456:
4454:Digit product
4452:
4446:
4443:
4441:
4438:
4436:
4433:
4431:
4428:
4427:
4425:
4423:
4419:
4411:
4408:
4406:
4403:
4402:
4401:
4398:
4397:
4395:
4393:
4388:
4384:
4380:
4375:
4370:
4366:
4356:
4353:
4351:
4348:
4346:
4343:
4341:
4338:
4336:
4333:
4331:
4328:
4326:
4323:
4321:
4318:
4316:
4313:
4311:
4308:
4306:
4303:
4301:
4298:
4296:
4293:
4291:
4290:Erdős–Nicolas
4288:
4286:
4283:
4281:
4278:
4277:
4274:
4269:
4265:
4259:
4255:
4241:
4238:
4236:
4233:
4232:
4230:
4228:
4224:
4218:
4215:
4213:
4210:
4208:
4205:
4203:
4200:
4199:
4197:
4195:
4191:
4185:
4182:
4180:
4177:
4175:
4172:
4170:
4167:
4165:
4162:
4160:
4157:
4156:
4154:
4152:
4148:
4142:
4139:
4137:
4134:
4133:
4131:
4129:
4125:
4119:
4116:
4114:
4111:
4109:
4108:Superabundant
4106:
4104:
4101:
4099:
4096:
4094:
4091:
4089:
4086:
4084:
4081:
4079:
4076:
4074:
4071:
4069:
4066:
4064:
4061:
4059:
4056:
4054:
4051:
4049:
4046:
4044:
4041:
4039:
4036:
4034:
4031:
4029:
4026:
4024:
4021:
4019:
4016:
4014:
4011:
4010:
4008:
4006:
4002:
3998:
3994:
3990:
3985:
3981:
3971:
3968:
3966:
3963:
3961:
3958:
3956:
3953:
3951:
3948:
3946:
3943:
3941:
3938:
3936:
3933:
3931:
3928:
3926:
3923:
3921:
3918:
3916:
3913:
3912:
3909:
3905:
3900:
3896:
3886:
3883:
3881:
3878:
3876:
3873:
3871:
3868:
3867:
3864:
3860:
3855:
3851:
3841:
3838:
3836:
3833:
3831:
3828:
3826:
3823:
3821:
3818:
3816:
3813:
3811:
3808:
3806:
3803:
3801:
3798:
3796:
3793:
3791:
3788:
3786:
3783:
3781:
3778:
3776:
3773:
3771:
3768:
3766:
3763:
3761:
3758:
3756:
3753:
3751:
3748:
3746:
3743:
3742:
3739:
3732:
3728:
3710:
3707:
3705:
3702:
3700:
3697:
3696:
3694:
3690:
3687:
3685:
3684:4-dimensional
3681:
3671:
3668:
3667:
3665:
3663:
3659:
3653:
3650:
3648:
3645:
3643:
3640:
3638:
3635:
3633:
3630:
3628:
3625:
3624:
3622:
3620:
3616:
3610:
3607:
3605:
3602:
3600:
3597:
3595:
3594:Centered cube
3592:
3590:
3587:
3586:
3584:
3582:
3578:
3575:
3573:
3572:3-dimensional
3569:
3559:
3556:
3554:
3551:
3549:
3546:
3544:
3541:
3539:
3536:
3534:
3531:
3529:
3526:
3524:
3521:
3519:
3516:
3514:
3511:
3510:
3508:
3506:
3502:
3496:
3493:
3491:
3488:
3486:
3483:
3481:
3478:
3476:
3473:
3471:
3468:
3466:
3463:
3461:
3458:
3456:
3453:
3452:
3450:
3448:
3444:
3441:
3439:
3438:2-dimensional
3435:
3431:
3427:
3422:
3418:
3408:
3405:
3403:
3400:
3398:
3395:
3393:
3390:
3388:
3385:
3383:
3382:Nonhypotenuse
3380:
3379:
3376:
3369:
3365:
3355:
3352:
3350:
3347:
3345:
3342:
3340:
3337:
3335:
3332:
3331:
3328:
3321:
3317:
3307:
3304:
3302:
3299:
3297:
3294:
3292:
3289:
3287:
3284:
3282:
3279:
3277:
3274:
3272:
3269:
3268:
3265:
3260:
3255:
3251:
3241:
3238:
3236:
3233:
3231:
3228:
3226:
3223:
3221:
3218:
3217:
3214:
3207:
3203:
3193:
3190:
3188:
3185:
3183:
3180:
3178:
3175:
3173:
3170:
3168:
3165:
3163:
3160:
3159:
3156:
3151:
3145:
3141:
3131:
3128:
3126:
3123:
3121:
3120:Perfect power
3118:
3116:
3113:
3111:
3110:Seventh power
3108:
3106:
3103:
3101:
3098:
3096:
3093:
3091:
3088:
3086:
3083:
3081:
3078:
3076:
3073:
3071:
3068:
3066:
3063:
3062:
3059:
3054:
3049:
3045:
3041:
3033:
3028:
3026:
3021:
3019:
3014:
3013:
3010:
2994:
2991:
2989:
2986:
2984:
2981:
2979:
2976:
2975:
2973:
2969:
2966:
2964:
2959:
2949:
2946:
2944:
2941:
2939:
2936:
2935:
2933:
2929:
2926:
2924:
2923:4-dimensional
2920:
2910:
2907:
2905:
2902:
2901:
2899:
2897:
2893:
2887:
2884:
2882:
2879:
2877:
2874:
2872:
2869:
2867:
2864:
2863:
2861:
2859:
2855:
2849:
2846:
2844:
2841:
2839:
2836:
2834:
2831:
2829:
2826:
2825:
2823:
2821:
2817:
2814:
2812:
2811:3-dimensional
2808:
2798:
2795:
2793:
2790:
2788:
2785:
2783:
2780:
2778:
2775:
2773:
2770:
2768:
2765:
2763:
2760:
2758:
2755:
2754:
2752:
2750:
2746:
2740:
2737:
2735:
2732:
2730:
2727:
2725:
2722:
2720:
2717:
2715:
2712:
2710:
2707:
2705:
2702:
2700:
2697:
2696:
2694:
2692:
2688:
2685:
2683:
2682:2-dimensional
2679:
2675:
2668:
2663:
2661:
2656:
2654:
2649:
2648:
2645:
2639:
2635:
2632:
2629:
2625:
2624:
2619:
2615:
2610:
2609:
2605:
2597:
2592:
2588:
2584:
2580:
2576:
2575:
2570:
2566:
2562:
2558:
2554:
2549:
2548:10.37236/1854
2544:
2540:
2536:
2535:
2530:
2521:
2517:
2511:
2507:
2503:
2499:
2495:
2491:
2487:
2483:
2482:
2477:
2472:
2468:
2464:
2460:
2456:
2452:
2448:
2447:
2427:
2419:
2416:
2410:
2405:
2401:
2397:
2387:
2383:
2379:
2378:
2372:
2364:
2363:
2357:
2353:
2347:
2343:
2338:
2334:
2330:
2326:
2322:
2318:
2314:
2313:
2307:
2302:
2301:
2295:
2291:
2287:
2282:
2281:10.37236/1762
2277:
2273:
2269:
2268:
2263:
2254:
2251:
2247:
2243:
2239:
2235:
2231:
2227:
2223:
2219:
2215:
2214:
2209:
2205:
2197:
2196:
2191:
2187:
2183:
2179:
2175:
2171:
2167:
2163:
2162:
2154:
2150:
2146:
2142:
2138:
2134:
2130:
2126:
2122:
2118:
2117:
2109:
2092:
2080:
2076:
2073:
2070:
2057:
2052:
2048:
2044:
2033:
2029:
2028:
2023:
2016:
2011:
2008:
2003:
2002:
1996:
1992:
1986:
1983:
1977:
1975:
1973:
1969:
1965:
1961:
1959:
1955:
1947:
1945:
1943:
1942:Nelsen (1993)
1939:
1935:
1931:
1927:
1923:
1919:
1915:
1906:
1902:
1900:
1888:
1884:
1880:
1863:
1858:
1853:
1849:
1844:
1839:
1836:
1833:
1829:
1824:
1819:
1817:
1807:
1799:
1796:
1793:
1790:
1787:
1784:
1781:
1775:
1773:
1761:
1756:
1751:
1747:
1744:
1739:
1735:
1728:
1720:
1715:
1711:
1708:
1705:
1702:
1697:
1693:
1688:
1684:
1681:
1678:
1671:
1667:
1660:
1656:
1653:
1646:
1642:
1635:
1631:
1628:
1621:
1617:
1610:
1607:
1589:
1587:
1575:
1571:
1564:
1559:
1555:
1552:
1549:
1546:
1541:
1537:
1532:
1528:
1525:
1522:
1518:
1514:
1511:
1508:
1505:
1500:
1496:
1491:
1483:
1480:
1477:
1470:
1466:
1459:
1455:
1452:
1449:
1446:
1443:
1440:
1437:
1430:
1423:
1419:
1412:
1408:
1405:
1402:
1399:
1396:
1389:
1382:
1378:
1371:
1367:
1364:
1361:
1354:
1347:
1343:
1336:
1333:
1327:
1325:
1315:
1311:
1307:
1304:
1301:
1298:
1295:
1292:
1289:
1286:
1283:
1280:
1277:
1275:
1268:
1264:
1258:
1253:
1250:
1247:
1243:
1218:
1212:
1209:
1206:
1203:
1195:
1190:
1187:
1184:
1180:
1176:
1171:
1167:
1156:
1145:
1128:
1122:
1119:
1116:
1113:
1103:
1099:
1093:
1090:
1085:
1082:
1079:
1075:
1071:
1068:
1064:
1060:
1055:
1051:
1040:
1036:
1032:
1016:
1006:
1000:
995:
991:
988:
985:
982:
977:
973:
968:
964:
961:
958:
954:
950:
947:
944:
941:
938:
935:
930:
926:
921:
917:
913:
909:
906:
903:
900:
897:
894:
889:
885:
880:
876:
872:
868:
865:
862:
859:
854:
850:
845:
837:
832:
828:
819:
815:
808:
806:
803:
797:
792:
788:
781:
777:
772:and of pairs
770:
766:
759:
755:
744:
740:
736:
732:
722:
718:
714:
710:
683:
679:
645:
641:
637:
633:
627:
621:
617:
613:
608:
603:
601:
597:
593:
581:
577:
573:
569:
565:
560:
551:
538:= (1 + 2 + 3)
534:
527:
525:
516:
505:
501:
497:
493:
483:
479:
475:
471:
467:
463:
459:
454:
437:
433:
409:
405:
384:
380:
369:
365:
348:
344:
331:
323:
321:
318: 120 CE
305:
301:
297:
278:
273:
268:
264:
259:
254:
251:
248:
244:
239:
234:
229:
225:
219:
214:
211:
208:
204:
196:
195:
194:
192:
173:
168:
163:
159:
156:
153:
150:
147:
144:
141:
138:
135:
131:
126:
121:
117:
113:
110:
107:
102:
98:
94:
89:
85:
81:
76:
72:
64:
63:
62:
60:
52:
48:
41:
40:number theory
33:
32:Gulley (2010)
28:
22:
4664:Transposable
4528:Narcissistic
4435:Digital root
4355:Super-Poulet
4315:Jordan–Pólya
4264:prime factor
4169:Noncototient
4136:Almost prime
4118:Superperfect
4093:Refactorable
4088:Quasiperfect
4063:Hyperperfect
3904:Pseudoprimes
3875:Wall–Sun–Sun
3810:Ordered Bell
3780:Fuss–Catalan
3703:
3692:non-centered
3642:Dodecahedral
3619:non-centered
3505:non-centered
3407:Wolstenholme
3152:× 2 ± 1
3149:
3148:Of the form
3115:Eighth power
3095:Fourth power
2971:non-centered
2942:
2931:non-centered
2866:Cube numbers
2858:non-centered
2749:non-centered
2739:Star numbers
2621:
2578:
2572:
2538:
2532:
2505:
2485:
2479:
2450:
2444:
2376:
2361:
2341:
2316:
2310:
2299:
2271:
2265:
2217:
2211:
2201:, AP Central
2194:
2165:
2159:
2120:
2114:
2010:
1998:
1985:
1962:
1951:
1934:Kanim (2004)
1922:Stein (1971)
1911:
1881:
1154:
1143:
1038:
1034:
812:
801:
790:
786:
779:
775:
768:
764:
757:
753:
742:
738:
734:
730:
720:
716:
712:
708:
681:
677:
643:
639:
635:
631:
628:
615:
611:
607:Stein (1971)
604:
589:
558:
455:
435:
431:
407:
403:
382:
378:
367:
363:
346:
342:
329:
327:
299:
293:
188:
37:
4685:Extravagant
4680:Equidigital
4635:permutation
4594:Palindromic
4568:Automorphic
4466:Sum-product
4445:Sum-product
4400:Persistence
4295:Erdős–Woods
4217:Untouchable
4098:Semiperfect
4048:Hemiperfect
3709:Tesseractic
3647:Icosahedral
3627:Tetrahedral
3558:Dodecagonal
3259:Recursively
3130:Prime power
3105:Sixth power
3100:Fifth power
3080:Power of 10
3038:Classes of
2963:dimensional
2581:: 145–151,
675:. That is,
548:= 1 + 2 + 3
522: 1500
511: 1300
488: 1000
61:. That is,
4946:Categories
4897:Graphemics
4770:Pernicious
4624:Undulating
4599:Pandigital
4573:Trimorphic
4174:Nontotient
4023:Arithmetic
3637:Octahedral
3538:Heptagonal
3528:Pentagonal
3513:Triangular
3354:Sierpiński
3276:Jacobsthal
3075:Power of 3
3070:Power of 2
2024:References
1954:power sums
1893:th row is
1883:Row (1893)
746:for which
701:that make
504:Gersonides
466:Nicomachus
4654:Parasitic
4503:Factorion
4430:Digit sum
4422:Digit sum
4240:Fortunate
4227:Primorial
4141:Semiprime
4078:Practical
4043:Descartes
4038:Deficient
4028:Betrothed
3870:Wieferich
3699:Pentatope
3662:pyramidal
3553:Decagonal
3548:Nonagonal
3543:Octagonal
3533:Hexagonal
3392:Practical
3339:Congruent
3271:Fibonacci
3235:Loeschian
2896:pyramidal
2623:MathWorld
2417:∑
2398:∑
2382:pp. 47–48
2250:126165678
2045:∑
1830:∑
1794:⋯
1721:⏟
1709:−
1682:⋯
1661:⏟
1636:⏟
1611:⏟
1565:⏟
1553:−
1526:⋯
1506:−
1481:⋯
1460:⏟
1413:⏟
1372:⏟
1337:⏟
1305:⋯
1244:∑
1210:−
1181:∑
1120:−
1083:−
1065:∑
1001:⏟
989:−
962:⋯
936:−
895:−
860:−
707:1 ≤
667:and that
550:) squares
542:1 + 2 + 3
500:Al-Qabisi
482:Al-Karaji
474:Aryabhata
311: 60
245:∑
205:∑
191:summation
154:⋯
111:⋯
4726:Friedman
4659:Primeval
4604:Repdigit
4561:-related
4508:Kaprekar
4482:Meertens
4405:Additive
4392:dynamics
4300:Friendly
4212:Sociable
4202:Amicable
4013:Abundant
3993:dynamics
3815:Schröder
3805:Narayana
3775:Eulerian
3765:Delannoy
3760:Dedekind
3581:centered
3447:centered
3334:Amenable
3291:Narayana
3281:Leonardo
3177:Mersenne
3125:Powerful
3065:Achilles
2820:centered
2691:centered
2634:Archived
2567:(1854),
2525:"On the
2504:(1963),
2192:(2004),
2182:27646391
799:by
536:All 36 (
370:+ 1) − 1
302:, after
296:identity
4899:related
4863:related
4827:related
4825:Sorting
4710:Vampire
4695:Harshad
4637:related
4609:Repunit
4523:Lychrel
4498:Dudeney
4350:Størmer
4345:Sphenic
4330:Regular
4268:divisor
4207:Perfect
4103:Sublime
4073:Perfect
3800:Motzkin
3755:Catalan
3296:Padovan
3230:Leyland
3225:Idoneal
3220:Hilbert
3192:Woodall
2961:Higher
2583:Bibcode
2557:2114194
2494:1355130
2467:2688231
2333:3219288
2290:2034423
2262:-cubes"
2242:0096615
2234:3609189
2137:1559017
1993:(ed.),
1899:gnomons
816: (
447:
428:
419:
400:
394:
375:
358:
339:
324:History
53:of the
49:is the
4765:Odious
4690:Frugal
4644:Cyclic
4633:Digit-
4340:Smooth
4325:Pronic
4285:Cyclic
4262:Other
4235:Euclid
3885:Wilson
3859:Primes
3518:Square
3387:Polite
3349:Riesel
3344:Knödel
3306:Perrin
3187:Thabit
3172:Fermat
3162:Cullen
3085:Square
3053:Powers
2555:
2512:
2492:
2465:
2348:
2331:
2288:
2248:
2240:
2232:
2180:
2135:
1151:up to
809:Proofs
697:, and
582:, 225,
492:Persia
470:Jordan
51:square
4806:Prime
4801:Lucky
4790:sieve
4719:Other
4705:Smith
4585:Digit
4543:Happy
4518:Keith
4491:Other
4335:Rough
4305:Giuga
3770:Euler
3632:Cubic
3286:Lucas
3182:Proth
2463:JSTOR
2366:(PDF)
2329:JSTOR
2246:S2CID
2230:JSTOR
2199:(PDF)
2178:JSTOR
2156:(PDF)
2133:JSTOR
2111:(PDF)
1978:Notes
784:with
762:with
624:4 × 4
587:... .
478:India
294:This
47:cubes
4760:Evil
4440:Self
4390:and
4280:Blum
3991:and
3795:Lobb
3750:Cake
3745:Bell
3495:Star
3402:Ulam
3301:Pell
3090:Cube
2510:ISBN
2346:ISBN
1999:The
1970:and
1157:− 1)
818:1854
651:and
620:grid
598:and
546:14 (
438:+ 1)
410:+ 1)
385:+ 1)
349:+ 1)
4878:Ban
4266:or
3785:Lah
2591:doi
2543:doi
2455:doi
2443:",
2321:doi
2276:doi
2222:doi
2170:doi
2125:doi
605:As
580:100
490:in
476:in
320:).
57:th
38:In
4948::
2620:,
2616:,
2589:,
2577:,
2571:,
2553:MR
2551:,
2539:11
2537:,
2531:,
2490:MR
2486:97
2484:,
2478:,
2461:,
2451:44
2449:,
2327:,
2317:77
2315:,
2286:MR
2284:,
2272:11
2270:,
2264:,
2244:,
2238:MR
2236:,
2228:,
2218:41
2216:,
2176:,
2166:37
2164:,
2158:,
2147:;
2131:,
2121:33
2119:,
2113:,
1997:,
1920:.
1456:19
1450:17
1444:15
1438:13
1409:11
1299:64
1293:27
805:.
789:≤
778:,
767:≤
756:,
741:,
737:,
733:,
719:≤
715:,
711:,
693:,
680:=
642:,
638:,
634:,
614:×
602:.
578:,
576:36
574:,
570:,
566:,
540:=
519:c.
508:c.
494:.
485:c.
453:.
315:c.
313:–
308:c.
193::
3150:a
3031:e
3024:t
3017:v
2666:e
2659:t
2652:v
2600:.
2593::
2585::
2579:7
2560:.
2545::
2527:q
2519:.
2497:.
2470:.
2457::
2428:2
2424:)
2420:k
2414:(
2411:=
2406:3
2402:k
2385:.
2370:.
2355:.
2336:.
2323::
2305:.
2293:.
2278::
2260:q
2224::
2203:.
2185:.
2172::
2140:.
2127::
2108:"
2093:2
2086:)
2081:2
2077:1
2074:+
2071:n
2065:(
2058:=
2053:3
2049:k
2017:.
1895:i
1891:i
1864:.
1859:2
1854:)
1850:k
1845:n
1840:1
1837:=
1834:k
1825:(
1820:=
1808:2
1804:)
1800:n
1797:+
1791:+
1788:2
1785:+
1782:1
1779:(
1776:=
1762:2
1757:)
1752:2
1748:n
1745:+
1740:2
1736:n
1729:(
1716:)
1712:1
1706:n
1703:+
1698:2
1694:n
1689:(
1685:+
1679:+
1672:2
1668:3
1657:5
1654:+
1647:2
1643:2
1632:3
1629:+
1622:2
1618:1
1608:1
1590:=
1576:3
1572:n
1560:)
1556:1
1550:n
1547:+
1542:2
1538:n
1533:(
1529:+
1523:+
1519:)
1515:1
1512:+
1509:n
1501:2
1497:n
1492:(
1484:+
1478:+
1471:3
1467:4
1453:+
1447:+
1441:+
1431:+
1424:3
1420:3
1406:+
1403:9
1400:+
1397:7
1390:+
1383:3
1379:2
1368:5
1365:+
1362:3
1355:+
1348:3
1344:1
1334:1
1328:=
1316:3
1312:n
1308:+
1302:+
1296:+
1290:+
1287:8
1284:+
1281:1
1278:=
1269:3
1265:k
1259:n
1254:1
1251:=
1248:k
1219:,
1216:)
1213:1
1207:k
1204:2
1201:(
1196:n
1191:1
1188:=
1185:k
1177:=
1172:2
1168:n
1155:n
1153:(
1149:1
1144:n
1129:,
1126:)
1123:1
1117:k
1114:2
1111:(
1104:n
1100:T
1094:1
1091:+
1086:1
1080:n
1076:T
1072:=
1069:k
1061:=
1056:3
1052:n
1039:n
1035:T
1017:.
1007:n
996:)
992:1
986:n
983:+
978:2
974:n
969:(
965:+
959:+
955:)
951:4
948:+
945:1
942:+
939:n
931:2
927:n
922:(
918:+
914:)
910:2
907:+
904:1
901:+
898:n
890:2
886:n
881:(
877:+
873:)
869:1
866:+
863:n
855:2
851:n
846:(
838:=
833:3
829:n
802:n
791:W
787:Z
782:)
780:W
776:Z
774:(
769:Y
765:X
760:)
758:Y
754:X
752:(
748:W
743:W
739:Z
735:Y
731:X
726:W
721:n
717:Z
713:Y
709:X
703:W
699:Z
695:Y
691:X
687:W
682:P
678:P
673:Z
669:W
665:X
661:Y
657:W
653:n
649:1
644:W
640:Z
636:Y
632:X
616:n
612:n
572:9
568:1
564:0
517:(
506:(
450:)
444:2
441:/
436:n
434:(
432:n
425:(
416:2
413:/
408:n
406:(
404:n
391:2
388:/
383:n
381:(
379:n
368:n
366:(
364:n
355:2
352:/
347:n
345:(
343:n
334:n
306:(
279:.
274:2
269:)
265:k
260:n
255:1
252:=
249:k
240:(
235:=
230:3
226:k
220:n
215:1
212:=
209:k
174:.
169:2
164:)
160:n
157:+
151:+
148:3
145:+
142:2
139:+
136:1
132:(
127:=
122:3
118:n
114:+
108:+
103:3
99:3
95:+
90:3
86:2
82:+
77:3
73:1
55:n
44:n
23:.
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.