1299:
1290:
1603:
75:
1612:
84:
66:
1590:
2395:
2736:
2386:
2699:
2560:
2518:
2662:
2653:
2329:
2320:
2593:
1268:
2437:
2725:
808:
2366:
34:
2377:
1970:
1641:
349:
2551:
2309:
1419:
673:
2509:
1095:
475:
580:
43:
2428:
2222:
1945:
1923:
648:
628:
326:
319:
298:
293:
2540:
2498:
2208:
1869:
1394:
1372:
568:
312:
305:
2355:
2298:
2215:
1318:
25:
2688:
1259:
2582:
2201:
1910:
1888:
615:
595:
1359:
1337:
1899:
1348:
606:
2143:
2417:
2185:
2171:
2157:
2767:
1934:
1383:
637:
269:
258:
225:
220:
247:
236:
1021:
1820:. The pentagons are reduced in size and new faces, flattened hexagons, are added in place of all the original edges. For a certain depth of chamfering, all (final) edges of the cD have the same length; then, the hexagons are
937:
2253:
The chamfer operation applied in series creates progressively larger polyhedra with new faces, hexagonal, replacing the edges of the current one. The chamfer operator transforms GP(m,n) to GP(2m,2n).
140:. (Equivalently: it separates the faces by reducing them, and adds a new face between each two adjacent faces; but it only moves the vertices inward.) For a polyhedron, this operation adds a new
1222:
1174:
2778:
For polychora, new cells are created around the original edges. The cells are prisms, containing two copies of the original face, with pyramids augmented onto the prism sides.
575:
looks similar; but its hexagons correspond to the 4 faces, not to the 6 edges, of the yellow tetrahedron, i.e. to the 4 vertices, not to the 6 edges, of the red tetrahedron.
1048:
1876:
looks similar, but its hexagons correspond to the 20 faces, not to the 30 edges, of the icosahedron, i.e. to the 20 vertices, not to the 30 edges, of the dodecahedron.
1325:
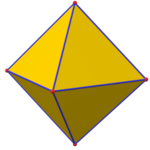
looks similar; but its hexagons correspond to the 8 faces, not to the 12 edges, of the octahedron, i.e. to the 8 vertices, not to the 12 edges, of the cube.
1298:
1289:
942:
2994:
1602:
74:
2917:
1611:
83:
877:
3055:
2039:
1488:
759:
2829:
1589:
65:
2692:
2394:
3091:
2958:
2931:
136:
apart (outward), and adds a new face between each two adjacent faces; but contrary to expansion, it maintains the original
1129:
of a rhombic dodecahedron and a cube of edge length 1 when the eight order-3 vertices of the rhombic dodecahedron are at
3019:
534:
For a certain depth of chamfering/truncation, all (final) edges of the cT have the same length; then, the hexagons are
3081:
2864:
2586:
841:: the squares are reduced in size and new faces, hexagons, are added in place of all the original edges. The cC is a
2735:
3029:
2787:
1981:
1927:
1847:
1765:
1652:
1430:
684:
360:
152:
2385:
2698:
2559:
2819:, p. 575, or p. 597 on Wikisource, CRYSTALLOGRAPHY, 1. CUBIC SYSTEM, TETRAHEDRAL CLASS, FIGS. 30 & 31.
2517:
2797:
2792:
2229:
2102:
1903:
1564:
For a certain depth of truncation, all (final) edges of the cO have the same length; then, the hexagons are
1179:
579:
2592:
2880:
2661:
2652:
2436:
2370:
2328:
2319:
1832:
1793:
1376:
1077:
864:
788:
523:
3020:
Vertex- and edge-truncation of the
Platonic and Archimedean solids leading to vertex-transitive polyhedra
1132:
3039:
2979:
2618:
2611:
2544:
2463:
2098:
1873:
1811:
1559:
1547:
1267:
1235:
846:
772:
572:
516:
197:
version where all edges have the same length, and in a canonical version where all edges touch the same
2724:
807:
2859:
2365:
2502:
2459:
2044:
2027:
1995:
1817:
1748:
1731:
1551:
1476:
1444:
1352:
1322:
1066:
747:
440:
423:
391:
129:
2376:
1969:
1640:
348:
3025:
2614:
2550:
2308:
2106:
1854:
1821:
1665:
1565:
1493:
1418:
1108:
1058:
856:
764:
694:
672:
553:
535:
512:
370:
194:
33:
2508:
1094:
1026:
3086:
2257:
1098:
Chamfered cube (3 zones are shown by 3 colors for their hexagons — each square is in 2 zones —.)
2984:
2901:
1944:
1922:
647:
627:
325:
318:
297:
292:
2956:; Grishukhin, V. (1998), "Fullerenes and coordination polyhedra versus half-cube embeddings",
2913:
2889:
2161:
2094:
1938:
1868:
1803:
1543:
1393:
1371:
842:
715:
567:
504:
474:
311:
304:
137:
42:
2967:
2905:
2893:
2729:
2427:
2421:
2237:
2221:
2175:
1317:
812:
641:
479:
3009:
2833:
2687:
2539:
2497:
2207:
1307:
Historical crystallographic models of axis shallower and deeper truncations of pyritohedron
2354:
2297:
2214:
2110:
2055:
1807:
1760:
1657:
1569:
1504:
860:
783:
539:
452:
145:
133:
24:
1909:
1887:
1258:
614:
594:
3060:
3046:
2927:
2885:
2313:
1839:, because only the (12) order-5 vertices of the rhombic triacontahedron are truncated.
1743:
1554:. These truncated vertices become congruent equilateral triangles, and the original 12
1358:
1336:
1084:
871:
435:
202:
190:
97:
2971:
2581:
2200:
1898:
1016:{\displaystyle \pi -{\frac {1}{2}}\cos ^{-1}(-{\frac {1}{3}})\approx 125.26^{\circ },}
3075:
2989:
2894:
2147:
1387:
1125:
93:
2359:
1347:
1239:
605:
262:
201:. (They look noticeably different only for solids containing triangles.) The shown
3066:
2953:
2909:
583:
Historical drawings of truncated tetrahedron and slightly chamfered tetrahedron.
273:
229:
2766:
2142:
1933:
2946:
2466:, GP(1,1), creates a Goldberg sequence: GP(1,1), GP(2,2), GP(4,4), GP(8,8)...
2416:
2184:
2170:
2156:
2068:
1775:
1517:
1088:
816:
798:
465:
251:
125:
3014:
2998:. Vol. 07 (11th ed.). Cambridge University Press. pp. 569–591.
1593:
Historical drawings of rhombic dodecahedron and slightly chamfered octahedron
1238:
and rectangular faces, can be constructed by chamfering the axial edges of a
2189:
1682:
1620:
Historical models of triakis cuboctahedron and slightly chamfered octahedron
1382:
636:
198:
268:
257:
224:
219:
530:: replacing 4 of its 8 vertices with congruent equilateral-triangle faces.
246:
235:
2086:
1699:
1535:
1227:
819:
are shown by 3 colors for their hexagons — each square is in 2 zones —.)
113:
2758:
Like the expansion operation, chamfer can be applied to any dimension.
2000:
1704:
1555:
1449:
867:
720:
396:
141:
106:
2983:
2621:, GP(3,0), creates a Goldberg sequence: GP(3,0), GP(6,0), GP(12,0)...
1243:
1083:
Because all the faces of the cC have an even number of sides and are
1959:
1867:
1630:
1588:
1408:
1316:
1093:
932:{\displaystyle \cos ^{-1}(-{\frac {1}{3}})\approx 109.47^{\circ }}
662:
578:
566:
338:
2940:
Fullerenes and coordination polyhedra versus half-cube embeddings
3035:
2302:
838:
527:
240:
52:
2943:
851:
For a certain depth of chamfering, all (final) edges of the
849:(and regular) squares, and 12 congruent flattened hexagons.
2761:
For polygons, it triples the number of vertices. Example:
2260:
sequence: GP(1,0), GP(2,0), GP(4,0), GP(8,0), GP(16,0)...
16:
Geometric operation which truncates the edges of polyhedra
1806:
with 80 vertices, 120 edges, and 42 faces: 12 congruent
1176:
and its six order-4 vertices are at the permutations of
2127:
The dual of the cI is the triakis icosidodecahedron.
1182:
1135:
1029:
945:
880:
159:
is represented by the letter "c". A polyhedron with
2938:Antoine Deza, Michel Deza, Viatcheslav Grishukhin,
105:For the concept in machining and architecture, see
1216:
1168:
1042:
1015:
931:
1586:The dual of the cO is the triakis cuboctahedron.
189:In the chapters below, the chamfers of the five
2772:(See also the previous version of this figure.)
1065:, because only the (6) order-4 vertices of the
51:Unchamfered, slightly chamfered, and chamfered
2932:"Mathematical Impressions: Goldberg Polyhedra"
1865:, containing pentagonal and hexagonal faces.
855:have the same length; then, the hexagons are
564:, containing triangular and hexagonal faces.
193:are described in detail. Each is shown in an
8:
2105:. The hexagonal faces of the cI can be made
845:with 32 vertices, 48 edges, and 18 faces: 6
163:edges will have a chamfered form containing
124:is a topological operator that modifies one
2136:Chamfered regular and quasiregular tilings
1962:
1633:
1411:
665:
549:is the alternate-triakis tetratetrahedron.
341:
1879:
1328:
586:
2256:A regular polyhedron, GP(1,0), creates a
1835:. The cD can more accurately be called a
1189:
1181:
1134:
1120:, containing square and hexagonal faces.
1061:. The cC can more accurately be called a
1034:
1028:
1004:
984:
966:
952:
944:
923:
903:
885:
879:
488:for a certain chamfering/truncating depth
3047:3.2.7. Systematic numbering for (C80-Ih)
2878:Clinton’s Equal Central Angle Conjecture
2765:
2623:
2468:
2262:
2134:
1967:
1638:
1416:
805:
670:
472:
346:
207:
2816:
2809:
1831:, although that name rather suggests a
1057:, although that name rather suggests a
1023:while a regular hexagon would have all
2948:(p. 72 Fig. 26. Chamfered tetrahedron)
2860:"A class of multi-symmetric polyhedra"
2113:, with a certain depth of truncation.
1837:pentatruncated rhombic triacontahedron
1217:{\displaystyle (\pm {\sqrt {3}},0,0).}
1881:Icosahedral chamfers and their duals
1827:The cD is also inaccurately called a
1053:The cC is also inaccurately called a
588:Tetrahedral chamfers and their duals
7:
2122:tritruncated rhombic triacontahedron
1816:It is constructed as a chamfer of a
1330:Octahedral chamfers and their duals
1276:Pyritohedron and its axis truncation
205:are dual to the canonical versions.
2900:(2nd ed.). Springer. pp.
1169:{\displaystyle (\pm 1,\pm 1,\pm 1)}
1063:tetratruncated rhombic dodecahedron
652:alternate-triakis tetratetrahedron
632:alternate-triakis tetratetrahedron
457:Alternate-triakis tetratetrahedron
2754:Chamfered polytopes and honeycombs
14:
2888:(2012). "Goldberg Polyhedra". In
1829:truncated rhombic triacontahedron
1581:tritruncated rhombic dodecahedron
837:is constructed as a chamfer of a
2734:
2723:
2697:
2686:
2660:
2651:
2591:
2580:
2558:
2549:
2538:
2516:
2507:
2496:
2435:
2426:
2415:
2393:
2384:
2375:
2364:
2353:
2327:
2318:
2307:
2296:
2220:
2213:
2206:
2199:
2183:
2169:
2155:
2141:
1968:
1943:
1932:
1921:
1908:
1897:
1886:
1639:
1610:
1601:
1417:
1392:
1381:
1370:
1357:
1346:
1335:
1297:
1288:
1266:
1257:
806:
671:
646:
635:
626:
613:
604:
593:
473:
347:
324:
317:
310:
303:
296:
291:
267:
256:
245:
234:
223:
218:
82:
73:
64:
41:
32:
23:
2101:the 20 order-3 vertices of the
2064:
2054:
2038:
2026:
2018:
2006:
1990:
1980:
1771:
1759:
1742:
1730:
1722:
1710:
1694:
1681:
1664:
1651:
1513:
1503:
1487:
1475:
1467:
1455:
1439:
1429:
794:
782:
758:
746:
738:
726:
710:
693:
683:
461:
451:
434:
422:
414:
402:
386:
369:
359:
144:face in place of each original
128:into another. It is similar to
2249:Relation to Goldberg polyhedra
2078:for a certain truncating depth
1785:for a certain chamfering depth
1550:the 8 order-3 vertices of the
1527:for a certain truncating depth
1208:
1183:
1163:
1136:
1055:truncated rhombic dodecahedron
994:
978:
913:
897:
826:for a certain chamfering depth
1:
2972:10.1016/S0012-365X(98)00065-X
610:dual of the tetratetrahedron
515:: replacing its 6 edges with
3067:How to make a chamfered cube
2713:
2676:
2641:
2570:
2528:
2486:
2405:
2343:
2286:
2227:
2197:
2139:
1043:{\displaystyle 120^{\circ }}
278:
209:
2910:10.1007/978-0-387-92714-5_9
2865:Tohoku Mathematical Journal
3108:
3030:Conway polyhedron notation
2858:Goldberg, Michael (1937).
2788:Conway polyhedron notation
2060:Triakis icosidodecahedron
1999:30 congruent equilateral*
1949:triakis icosidodecahedron
1928:pentakis icosidodecahedron
1848:pentakis icosidodecahedron
1791:
1766:Pentakis icosidodecahedron
1703:30 congruent equilateral*
1448:12 congruent equilateral*
719:12 congruent equilateral*
153:Conway polyhedron notation
104:
3026:VRML polyhedral generator
2131:Chamfered regular tilings
2075:
1782:
1524:
939:and 4 internal angles of
823:
485:
395:6 congruent equilateral*
185:Chamfered Platonic solids
501:alternate truncated cube
2995:Encyclopædia Britannica
2985:"Crystallography"
2798:Cantellation (geometry)
2793:Near-miss Johnson solid
2103:rhombic triacontahedron
1904:rhombic triacontahedron
1634:Chamfered dodecahedron
2980:Spencer, Leonard James
2934:. Simons Science News.
2773:
1963:Chamfered icosahedron
1892:chamfered dodecahedron
1877:
1844:chamfered dodecahedron
1833:rhombicosidodecahedron
1800:chamfered dodecahedron
1794:Chamfered dodecahedron
1627:Chamfered dodecahedron
1594:
1509:Triakis cuboctahedron
1398:triakis cuboctahedron
1377:tetrakis cuboctahedron
1326:
1218:
1170:
1099:
1078:tetrakis cuboctahedron
1044:
1017:
933:
789:Tetrakis cuboctahedron
584:
576:
524:alternately truncating
342:Chamfered tetrahedron
96:of slightly chamfered
3092:Mathematical notation
3010:Chamfered Tetrahedron
2769:
2619:pentakis dodecahedron
2464:truncated icosahedron
2120:can also be called a
2118:chamfered icosahedron
2091:chamfered icosahedron
1996:equilateral triangles
1956:Chamfered icosahedron
1914:chamfered icosahedron
1874:truncated icosahedron
1871:
1698:12 congruent regular
1592:
1579:can also be called a
1445:equilateral triangles
1412:Chamfered octahedron
1320:
1236:pyritohedral symmetry
1219:
1171:
1097:
1045:
1018:
934:
865:alternately truncated
863:. They are congruent
619:chamfered tetrahedron
599:chamfered tetrahedron
582:
573:truncated tetrahedron
570:
547:chamfered tetrahedron
497:chamfered tetrahedron
392:equilateral triangles
335:Chamfered tetrahedron
181:new hexagonal faces.
2959:Discrete Mathematics
2460:truncated octahedron
2028:Vertex configuration
1818:regular dodecahedron
1732:Vertex configuration
1577:chamfered octahedron
1552:rhombic dodecahedron
1540:chamfered octahedron
1477:Vertex configuration
1405:Chamfered octahedron
1363:chamfered octahedron
1353:rhombic dodecahedron
1323:truncated octahedron
1180:
1133:
1067:rhombic dodecahedron
1027:
943:
878:
748:Vertex configuration
424:Vertex configuration
2876:Joseph D. Clinton,
2615:tetrakis hexahedron
2137:
1882:
1855:Goldberg polyhedron
1824:, but not regular.
1814:flattened hexagons.
1666:Goldberg polyhedron
1562:flattened hexagons.
1331:
1109:Goldberg polyhedron
1085:centrally symmetric
1059:rhombicuboctahedron
695:Goldberg polyhedron
589:
554:Goldberg polyhedron
519:flattened hexagons;
513:regular tetrahedron
371:Goldberg polyhedron
3082:Goldberg polyhedra
2890:Senechal, Marjorie
2774:
2770:A chamfered square
2258:Goldberg polyhedra
2135:
1975:(equilateral form)
1880:
1878:
1646:(equilateral form)
1595:
1424:(equilateral form)
1329:
1327:
1230:equivalent to the
1214:
1166:
1100:
1040:
1013:
929:
678:(equilateral form)
587:
585:
577:
354:(equilateral form)
2930:(June 18, 2013).
2919:978-0-387-92713-8
2751:
2750:
2608:
2607:
2456:
2455:
2246:
2245:
2162:Triangular tiling
2095:convex polyhedron
2083:
2082:
2014:hexagon-hexagon)
2012:triangle-hexagon,
1953:
1952:
1939:icosidodecahedron
1916:(canonical form)
1894:(canonical form)
1810:pentagons and 30
1804:convex polyhedron
1790:
1789:
1718:hexagon-hexagon)
1716:pentagon-hexagon,
1544:convex polyhedron
1532:
1531:
1463:hexagon-hexagon)
1461:triangle-hexagon,
1402:
1401:
1365:(canonical form)
1343:(canonical form)
1315:
1314:
1242:. This occurs in
1194:
1050:internal angles.
992:
960:
911:
843:convex polyhedron
831:
830:
734:hexagon-hexagon)
656:
655:
621:(canonical form)
601:(canonical form)
505:convex polyhedron
493:
492:
410:hexagon-hexagon)
408:triangle-hexagon,
332:
331:
3099:
3015:Chamfered Solids
2999:
2987:
2974:
2935:
2923:
2899:
2873:
2845:
2844:
2842:
2841:
2832:. Archived from
2826:
2820:
2814:
2738:
2727:
2701:
2690:
2664:
2655:
2624:
2595:
2584:
2562:
2553:
2542:
2520:
2511:
2500:
2469:
2439:
2430:
2419:
2397:
2388:
2379:
2368:
2357:
2331:
2322:
2311:
2300:
2263:
2224:
2217:
2210:
2203:
2187:
2176:Hexagonal tiling
2173:
2159:
2145:
2138:
2079:
1976:
1972:
1960:
1947:
1936:
1925:
1912:
1901:
1890:
1883:
1842:The dual of the
1786:
1660:= t5daD = dk5aD
1647:
1643:
1631:
1614:
1605:
1528:
1425:
1421:
1409:
1396:
1385:
1374:
1361:
1350:
1339:
1332:
1301:
1292:
1270:
1261:
1249:
1248:
1223:
1221:
1220:
1215:
1195:
1190:
1175:
1173:
1172:
1167:
1128:
1111:
1072:The dual of the
1049:
1047:
1046:
1041:
1039:
1038:
1022:
1020:
1019:
1014:
1009:
1008:
993:
985:
974:
973:
961:
953:
938:
936:
935:
930:
928:
927:
912:
904:
893:
892:
827:
810:
679:
675:
663:
650:
642:tetratetrahedron
639:
630:
617:
608:
597:
590:
545:The dual of the
511:by chamfering a
489:
477:
355:
351:
339:
328:
321:
314:
307:
300:
295:
271:
260:
249:
238:
227:
222:
208:
180:
176:
169:
162:
86:
77:
68:
45:
36:
27:
3107:
3106:
3102:
3101:
3100:
3098:
3097:
3096:
3072:
3071:
3006:
2978:
2951:
2926:
2920:
2884:
2857:
2854:
2849:
2848:
2839:
2837:
2828:
2827:
2823:
2815:
2811:
2806:
2784:
2771:
2756:
2743:
2739:
2728:
2719:
2718:
2706:
2702:
2691:
2682:
2681:
2669:
2665:
2656:
2647:
2646:
2600:
2596:
2585:
2576:
2575:
2563:
2554:
2543:
2534:
2533:
2521:
2512:
2501:
2492:
2491:
2448:
2444:
2440:
2431:
2420:
2411:
2410:
2398:
2389:
2380:
2369:
2358:
2349:
2348:
2336:
2332:
2323:
2312:
2301:
2292:
2291:
2251:
2193:
2188:
2179:
2174:
2165:
2160:
2151:
2146:
2133:
2097:constructed by
2077:
2071:, equilateral*
2056:Dual polyhedron
2048:
2034:(12) 6.6.6.6.6
2033:
2013:
2011:
1998:
1982:Conway notation
1974:
1973:
1958:
1948:
1937:
1926:
1915:
1913:
1902:
1893:
1891:
1864:
1861:(2,0) or {5+,3}
1860:
1815:
1796:
1784:
1778:, equilateral*
1761:Dual polyhedron
1754:
1737:
1717:
1715:
1702:
1690:
1677:
1673:
1653:Conway notation
1645:
1644:
1629:
1624:
1623:
1622:
1621:
1617:
1616:
1615:
1607:
1606:
1563:
1546:constructed by
1526:
1520:, equilateral*
1505:Dual polyhedron
1497:
1482:
1462:
1460:
1447:
1431:Conway notation
1423:
1422:
1407:
1397:
1386:
1375:
1364:
1362:
1351:
1342:
1340:
1311:
1310:
1309:
1308:
1304:
1303:
1302:
1294:
1293:
1280:
1279:
1278:
1277:
1273:
1272:
1271:
1263:
1262:
1178:
1177:
1131:
1130:
1123:
1119:
1116:(2,0) or {4+,3}
1115:
1102:
1069:are truncated.
1030:
1025:
1024:
1000:
962:
941:
940:
919:
881:
876:
875:
872:internal angles
850:
825:
811:
801:, equilateral*
784:Dual polyhedron
776:
771:
768:
753:
733:
732:square-hexagon,
731:
718:
706:
702:
685:Conway notation
677:
676:
666:Chamfered cube
661:
651:
640:
631:
620:
618:
609:
600:
598:
563:
560:(2,0) or {3+,3}
559:
487:
478:
468:, equilateral*
453:Dual polyhedron
446:
429:
409:
407:
394:
382:
378:
361:Conway notation
353:
352:
337:
287:
285:
283:
281:
272:
261:
250:
239:
228:
214:
212:
191:Platonic solids
187:
178:
177:new edges, and
171:
164:
160:
132:: it moves the
122:edge-truncation
110:
103:
102:
101:
100:
98:Platonic solids
89:
88:
87:
79:
78:
70:
69:
58:
57:
56:
55:
48:
47:
46:
38:
37:
29:
28:
17:
12:
11:
5:
3105:
3103:
3095:
3094:
3089:
3084:
3074:
3073:
3070:
3069:
3064:
3063:
3062:
3058:
3057:(Number 7 -Ih)
3052:Fullerene C80
3050:
3044:
3043:
3042:
3040:Chamfered cube
3023:
3017:
3012:
3005:
3004:External links
3002:
3001:
3000:
2990:Chisholm, Hugh
2976:
2949:
2936:
2924:
2918:
2882:
2874:
2853:
2850:
2847:
2846:
2821:
2808:
2807:
2805:
2802:
2801:
2800:
2795:
2790:
2783:
2780:
2776:
2775:
2755:
2752:
2749:
2748:
2745:
2741:
2732:
2721:
2716:
2712:
2711:
2708:
2704:
2695:
2684:
2679:
2675:
2674:
2671:
2667:
2658:
2649:
2644:
2640:
2639:
2636:
2633:
2630:
2627:
2606:
2605:
2602:
2598:
2589:
2578:
2573:
2569:
2568:
2565:
2556:
2547:
2536:
2531:
2527:
2526:
2523:
2514:
2505:
2494:
2489:
2485:
2484:
2481:
2478:
2475:
2472:
2454:
2453:
2450:
2446:
2442:
2433:
2424:
2413:
2408:
2404:
2403:
2400:
2391:
2382:
2373:
2362:
2351:
2346:
2342:
2341:
2338:
2334:
2325:
2316:
2305:
2294:
2289:
2285:
2284:
2281:
2278:
2275:
2272:
2269:
2266:
2250:
2247:
2244:
2243:
2240:
2235:
2232:
2226:
2225:
2218:
2211:
2204:
2196:
2195:
2181:
2167:
2153:
2132:
2129:
2081:
2080:
2073:
2072:
2066:
2062:
2061:
2058:
2052:
2051:
2046:
2042:
2036:
2035:
2030:
2024:
2023:
2020:
2016:
2015:
2008:
2004:
2003:
1992:
1988:
1987:
1984:
1978:
1977:
1965:
1964:
1957:
1954:
1951:
1950:
1941:
1930:
1918:
1917:
1906:
1895:
1862:
1858:
1853:The cD is the
1792:Main article:
1788:
1787:
1780:
1779:
1773:
1769:
1768:
1763:
1757:
1756:
1752:
1746:
1744:Symmetry group
1740:
1739:
1734:
1728:
1727:
1724:
1720:
1719:
1712:
1708:
1707:
1696:
1692:
1691:
1688:
1685:
1679:
1678:
1675:
1674:(2,0) = {5+,3}
1671:
1668:
1662:
1661:
1655:
1649:
1648:
1636:
1635:
1628:
1625:
1619:
1618:
1609:
1608:
1600:
1599:
1598:
1597:
1596:
1530:
1529:
1522:
1521:
1515:
1511:
1510:
1507:
1501:
1500:
1495:
1491:
1485:
1484:
1479:
1473:
1472:
1469:
1465:
1464:
1457:
1453:
1452:
1441:
1437:
1436:
1433:
1427:
1426:
1414:
1413:
1406:
1403:
1400:
1399:
1390:
1379:
1367:
1366:
1355:
1344:
1341:chamfered cube
1313:
1312:
1306:
1305:
1296:
1295:
1287:
1286:
1285:
1284:
1283:
1281:
1275:
1274:
1265:
1264:
1256:
1255:
1254:
1253:
1252:
1232:chamfered cube
1213:
1210:
1207:
1204:
1201:
1198:
1193:
1188:
1185:
1165:
1162:
1159:
1156:
1153:
1150:
1147:
1144:
1141:
1138:
1124:The cC is the
1117:
1113:
1105:chamfered cube
1074:chamfered cube
1037:
1033:
1012:
1007:
1003:
999:
996:
991:
988:
983:
980:
977:
972:
969:
965:
959:
956:
951:
948:
926:
922:
918:
915:
910:
907:
902:
899:
896:
891:
888:
884:
853:chamfered cube
835:chamfered cube
829:
828:
821:
820:
803:
802:
796:
792:
791:
786:
780:
779:
774:
766:
762:
756:
755:
750:
744:
743:
740:
736:
735:
728:
724:
723:
712:
708:
707:
704:
703:(2,0) = {4+,3}
700:
697:
691:
690:
687:
681:
680:
668:
667:
660:
659:Chamfered cube
657:
654:
653:
644:
633:
623:
622:
611:
602:
561:
557:
552:The cT is the
532:
531:
520:
491:
490:
483:
482:
470:
469:
463:
459:
458:
455:
449:
448:
444:
438:
436:Symmetry group
432:
431:
426:
420:
419:
416:
412:
411:
404:
400:
399:
388:
384:
383:
380:
379:(2,0) = {3+,3}
376:
373:
367:
366:
363:
357:
356:
344:
343:
336:
333:
330:
329:
322:
315:
308:
301:
289:
277:
276:
265:
254:
243:
232:
216:
203:dual polyhedra
186:
183:
170:new vertices,
94:crystal models
91:
90:
81:
80:
72:
71:
63:
62:
61:
60:
59:
50:
49:
40:
39:
31:
30:
22:
21:
20:
19:
18:
15:
13:
10:
9:
6:
4:
3:
2:
3104:
3093:
3090:
3088:
3085:
3083:
3080:
3079:
3077:
3068:
3065:
3061:
3059:
3056:
3054:
3053:
3051:
3048:
3045:
3041:
3037:
3034:
3033:
3031:
3027:
3024:
3021:
3018:
3016:
3013:
3011:
3008:
3007:
3003:
2997:
2996:
2991:
2986:
2981:
2977:
2973:
2969:
2965:
2961:
2960:
2955:
2950:
2947:
2945:
2941:
2937:
2933:
2929:
2925:
2921:
2915:
2911:
2907:
2903:
2898:
2897:
2896:Shaping Space
2891:
2887:
2883:
2881:
2879:
2875:
2871:
2867:
2866:
2861:
2856:
2855:
2851:
2836:on 2014-08-12
2835:
2831:
2830:"C80 Isomers"
2825:
2822:
2818:
2813:
2810:
2803:
2799:
2796:
2794:
2791:
2789:
2786:
2785:
2781:
2779:
2768:
2764:
2763:
2762:
2759:
2753:
2746:
2742:
2737:
2733:
2731:
2726:
2722:
2714:
2709:
2705:
2700:
2696:
2694:
2689:
2685:
2677:
2672:
2668:
2663:
2659:
2654:
2650:
2642:
2637:
2634:
2631:
2628:
2626:
2625:
2622:
2620:
2616:
2613:
2603:
2599:
2594:
2590:
2588:
2583:
2579:
2571:
2566:
2561:
2557:
2552:
2548:
2546:
2541:
2537:
2529:
2524:
2519:
2515:
2510:
2506:
2504:
2499:
2495:
2487:
2482:
2479:
2476:
2473:
2471:
2470:
2467:
2465:
2461:
2451:
2447:
2443:
2438:
2434:
2429:
2425:
2423:
2418:
2414:
2406:
2401:
2396:
2392:
2387:
2383:
2378:
2374:
2372:
2367:
2363:
2361:
2356:
2352:
2344:
2339:
2335:
2330:
2326:
2321:
2317:
2315:
2310:
2306:
2304:
2299:
2295:
2287:
2282:
2279:
2276:
2273:
2270:
2267:
2265:
2264:
2261:
2259:
2254:
2248:
2241:
2239:
2236:
2233:
2231:
2228:
2223:
2219:
2216:
2212:
2209:
2205:
2202:
2198:
2191:
2186:
2182:
2177:
2172:
2168:
2163:
2158:
2154:
2149:
2148:Square tiling
2144:
2140:
2130:
2128:
2125:
2123:
2119:
2114:
2112:
2108:
2104:
2100:
2096:
2092:
2088:
2074:
2070:
2067:
2063:
2059:
2057:
2053:
2049:
2043:
2041:
2037:
2031:
2029:
2025:
2022:72 (2 types)
2021:
2017:
2010:120 (2 types:
2009:
2005:
2002:
1997:
1994:20 congruent
1993:
1989:
1985:
1983:
1979:
1971:
1966:
1961:
1955:
1946:
1942:
1940:
1935:
1931:
1929:
1924:
1920:
1919:
1911:
1907:
1905:
1900:
1896:
1889:
1885:
1884:
1875:
1870:
1866:
1856:
1851:
1849:
1845:
1840:
1838:
1834:
1830:
1825:
1823:
1819:
1813:
1809:
1805:
1801:
1795:
1781:
1777:
1774:
1770:
1767:
1764:
1762:
1758:
1750:
1747:
1745:
1741:
1735:
1733:
1729:
1726:80 (2 types)
1725:
1721:
1714:120 (2 types:
1713:
1709:
1706:
1701:
1697:
1693:
1686:
1684:
1680:
1669:
1667:
1663:
1659:
1656:
1654:
1650:
1642:
1637:
1632:
1626:
1613:
1604:
1591:
1587:
1584:
1582:
1578:
1573:
1571:
1567:
1561:
1558:faces become
1557:
1553:
1549:
1545:
1541:
1537:
1523:
1519:
1516:
1512:
1508:
1506:
1502:
1498:
1492:
1490:
1486:
1480:
1478:
1474:
1471:30 (2 types)
1470:
1466:
1458:
1454:
1451:
1446:
1442:
1438:
1434:
1432:
1428:
1420:
1415:
1410:
1404:
1395:
1391:
1389:
1388:cuboctahedron
1384:
1380:
1378:
1373:
1369:
1368:
1360:
1356:
1354:
1349:
1345:
1338:
1334:
1333:
1324:
1319:
1300:
1291:
1282:
1269:
1260:
1251:
1250:
1247:
1245:
1241:
1237:
1233:
1229:
1224:
1211:
1205:
1202:
1199:
1196:
1191:
1186:
1160:
1157:
1154:
1151:
1148:
1145:
1142:
1139:
1127:
1126:Minkowski sum
1121:
1110:
1106:
1096:
1092:
1090:
1086:
1081:
1079:
1075:
1070:
1068:
1064:
1060:
1056:
1051:
1035:
1031:
1010:
1005:
1001:
997:
989:
986:
981:
975:
970:
967:
963:
957:
954:
949:
946:
924:
920:
916:
908:
905:
900:
894:
889:
886:
882:
873:
869:
866:
862:
858:
854:
848:
844:
840:
836:
822:
818:
814:
809:
804:
800:
797:
793:
790:
787:
785:
781:
777:
769:
763:
761:
757:
751:
749:
745:
742:32 (2 types)
741:
737:
729:
725:
722:
717:
713:
709:
698:
696:
692:
688:
686:
682:
674:
669:
664:
658:
649:
645:
643:
638:
634:
629:
625:
624:
616:
612:
607:
603:
596:
592:
591:
581:
574:
569:
565:
555:
550:
548:
543:
541:
537:
529:
525:
521:
518:
514:
510:
509:
508:
507:constructed:
506:
502:
498:
484:
481:
476:
471:
467:
464:
460:
456:
454:
450:
442:
439:
437:
433:
427:
425:
421:
418:16 (2 types)
417:
413:
405:
401:
398:
393:
389:
385:
374:
372:
368:
364:
362:
358:
350:
345:
340:
334:
327:
323:
320:
316:
313:
309:
306:
302:
299:
294:
290:
279:
275:
270:
266:
264:
259:
255:
253:
248:
244:
242:
237:
233:
231:
226:
221:
217:
210:
206:
204:
200:
196:
192:
184:
182:
175:
168:
158:
154:
149:
147:
143:
139:
135:
131:
127:
123:
119:
115:
108:
99:
95:
85:
76:
67:
54:
44:
35:
26:
3022:Livio Zefiro
2993:
2966:(1): 41–80,
2963:
2957:
2939:
2928:Hart, George
2895:
2886:Hart, George
2877:
2869:
2863:
2838:. Retrieved
2834:the original
2824:
2817:Spencer 1911
2812:
2777:
2760:
2757:
2609:
2457:
2255:
2252:
2126:
2121:
2117:
2115:
2090:
2084:
1852:
1843:
1841:
1836:
1828:
1826:
1799:
1797:
1585:
1580:
1576:
1574:
1539:
1533:
1483:(6) 6.6.6.6
1459:48 (2 types:
1443:8 congruent
1240:pyritohedron
1231:
1225:
1122:
1107:is also the
1104:
1101:
1082:
1073:
1071:
1062:
1054:
1052:
852:
834:
832:
730:48 (2 types:
714:6 congruent
551:
546:
544:
533:
526:a (regular)
500:
496:
494:
406:24 (2 types:
390:4 congruent
286:(equilateral
188:
173:
166:
156:
150:
121:
117:
111:
2107:equilateral
2050:, , (*532)
1986:cI = t3daI
1822:equilateral
1749:Icosahedral
1738:(20) 6.6.6
1566:equilateral
1499:, , (*432)
1435:cO = t3daO
1234:, but with
1228:topological
857:equilateral
689:cC = t4daC
536:equilateral
441:Tetrahedral
195:equilateral
92:Historical
3076:Categories
2952:Deza, A.;
2872:: 104–108.
2840:2014-08-09
2804:References
2109:, but not
2099:truncating
2065:Properties
2032:(24) 3.6.6
1772:Properties
1736:(60) 5.6.6
1568:, but not
1548:truncating
1514:Properties
1481:(24) 3.6.6
1246:crystals.
1089:zonohedron
1087:, it is a
859:, but not
795:Properties
778:, , (3*2)
770:, , (*432)
754:(8) 6.6.6
752:(24) 4.6.6
538:, but not
462:Properties
430:(4) 6.6.6
428:(12) 3.6.6
157:chamfering
126:polyhedron
118:chamfering
3087:Polyhedra
3049:fullerene
2635:GP(12,0)
2612:truncated
2280:GP(16,0)
2190:Rhombille
1812:congruent
1700:pentagons
1683:Fullerene
1560:congruent
1187:±
1158:±
1149:±
1140:±
1036:∘
1006:∘
998:≈
982:−
976:
968:−
950:−
947:π
925:∘
917:≈
901:−
895:
887:−
870:, have 2
847:congruent
517:congruent
280:Chamfered
199:midsphere
142:hexagonal
130:expansion
2982:(1911).
2954:Deza, M.
2782:See also
2632:GP(6,0)
2629:GP(3,0)
2480:GP(4,4)
2477:GP(2,2)
2474:GP(1,1)
2277:GP(8,0)
2274:GP(4,0)
2271:GP(2,0)
2268:GP(1,0)
2194:dr{6,3}
2087:geometry
2040:Symmetry
2019:Vertices
2001:hexagons
1723:Vertices
1705:hexagons
1536:geometry
1489:Symmetry
1468:Vertices
1450:hexagons
760:Symmetry
739:Vertices
721:hexagons
415:Vertices
397:hexagons
282:Platonic
213:Platonic
138:vertices
114:geometry
2992:(ed.).
2942:, 1998
2892:(ed.).
2852:Sources
2720:{6+,3}
2683:{5+,3}
2648:{4+,3}
2577:{6+,3}
2535:{5+,3}
2493:{4+,3}
2412:{6+,3}
2350:{5+,3}
2293:{4+,3}
2111:regular
1846:is the
1808:regular
1570:regular
1556:rhombic
1076:is the
861:regular
716:squares
540:regular
107:chamfer
3038:model
2916:
2904:–138.
2744:cctkH
2707:cctkD
2670:cctkC
2449:ccccH
2399:ccccD
2337:ccccC
2180:{6,3}
2166:{3,6}
2152:{4,4}
2089:, the
2069:convex
1776:convex
1538:, the
1518:convex
1244:pyrite
1002:125.26
921:109.47
868:rhombi
799:convex
522:or by
466:convex
288:form)
215:solid
2988:. In
2740:ctkH
2703:ctkD
2666:ctkC
2601:cctΔ
2564:cctI
2522:cctO
2445:cccH
2390:cccD
2333:cccC
2242:cdaH
2192:, daH
2093:is a
2007:Edges
1991:Faces
1802:is a
1711:Edges
1695:Faces
1542:is a
1456:Edges
1440:Faces
817:zones
727:Edges
711:Faces
503:is a
403:Edges
387:Faces
284:solid
274:{3,5}
263:{5,3}
252:{3,4}
241:{4,3}
230:{3,3}
134:faces
3036:VRML
2914:ISBN
2747:...
2710:...
2673:...
2657:tkC
2638:...
2604:...
2597:ctΔ
2567:...
2555:ctI
2525:...
2513:ctO
2483:...
2458:The
2452:...
2441:ccH
2402:...
2381:ccD
2340:...
2324:ccC
2283:...
2116:The
1872:The
1798:The
1575:The
1321:The
1103:The
839:cube
833:The
571:The
528:cube
495:The
211:Seed
146:edge
53:cube
2968:doi
2964:192
2944:PDF
2906:doi
2902:125
2730:tkH
2693:tkD
2617:or
2462:or
2432:cH
2234:cΔ
2178:, H
2164:, Δ
2150:, Q
2085:In
1863:2,0
1676:2,0
1534:In
1118:2,0
1032:120
964:cos
883:cos
874:of
815:(3
813:Net
705:2,0
562:2,0
558:III
499:or
480:Net
381:2,0
377:III
365:cT
151:In
120:or
112:In
3078::
3032:)
2962:,
2912:.
2870:43
2868:.
2862:.
2717:VI
2715:GP
2678:GP
2645:IV
2643:GP
2610:A
2587:tΔ
2574:VI
2572:GP
2545:tI
2530:GP
2503:tO
2490:IV
2488:GP
2409:VI
2407:GP
2371:cD
2345:GP
2314:cC
2290:IV
2288:GP
2238:cH
2230:cQ
2124:.
1857:GP
1850:.
1755:)
1751:(I
1689:80
1670:GP
1658:cD
1583:.
1572:.
1226:A
1114:IV
1112:GP
1091::
1080:.
701:IV
699:GP
556:GP
542:.
447:)
443:(T
375:GP
155:,
148:.
116:,
3028:(
2975:.
2970::
2922:.
2908::
2843:.
2680:V
2532:V
2422:H
2360:D
2347:V
2303:C
2076:*
2047:h
2045:I
1859:V
1783:*
1753:h
1687:C
1672:V
1525:*
1496:h
1494:O
1212:.
1209:)
1206:0
1203:,
1200:0
1197:,
1192:3
1184:(
1164:)
1161:1
1155:,
1152:1
1146:,
1143:1
1137:(
1011:,
995:)
990:3
987:1
979:(
971:1
958:2
955:1
914:)
909:3
906:1
898:(
890:1
824:*
775:h
773:T
767:h
765:O
486:*
445:d
179:e
174:e
172:3
167:e
165:2
161:e
109:.
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.