1240:
1004:
406:
33:
606:
588:
1050:; a sublattice is a subset that is closed under the meet and join operations of the original lattice. Note that this is not the same as being a subset that is a lattice under the original order (but possibly with different join and meet operations). Further characterizations derive from the representation theory in the next section.
2057:
1870:
The numbers above count the number of elements in free distributive lattices in which the lattice operations are joins and meets of finite sets of elements, including the empty set. If empty joins and empty meets are disallowed, the resulting free distributive lattices have two fewer elements; their
415:
Distributive lattices are ubiquitous but also rather specific structures. As already mentioned the main example for distributive lattices are lattices of sets, where join and meet are given by the usual set-theoretic operations. Further examples include:
886:
397:, i.e. a function that is compatible with the two lattice operations. Because such a morphism of lattices preserves the lattice structure, it will consequently also preserve the distributivity (and thus be a morphism of distributive lattices).
381:) are always true. A lattice is distributive if one of the converse inequalities holds, too. More information on the relationship of this condition to other distributivity conditions of order theory can be found in the article
1127:
stated below. The important insight from this characterization is that the identities (equations) that hold in all distributive lattices are exactly the ones that hold in all lattices of sets in the above sense.
1200:
1410:
1223:
As a consequence of Stone's and
Priestley's theorems, one easily sees that any distributive lattice is really isomorphic to a lattice of sets. However, the proofs of both statements require the
1166:
1111:
The introduction already hinted at the most important characterization for distributive lattices: a lattice is distributive if and only if it is isomorphic to a lattice of sets (closed under
1831:
1527:
766:
1206:
1185:
931:
1123:
in this context.) That set union and intersection are indeed distributive in the above sense is an elementary fact. The other direction is less trivial, in that it requires the
1203:. In this formulation, a distributive lattice is used to construct a topological space with an additional partial order on its points, yielding a (completely order-separated)
969:
1303:
can be constructed much more easily than a general free lattice. The first observation is that, using the laws of distributivity, every term formed by the binary operations
1243:
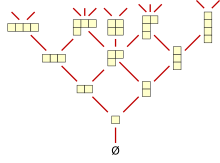
Free distributive lattices on zero, one, two, and three generators. The elements labeled "0" and "1" are the empty join and meet, and the element labeled "majority" is (
1683:
1626:
1341:
1749:
1714:
1653:
1596:
1557:
1440:
1321:
998:
2885:
1882:
1863:
546:
closed under coordinatewise minimum and coordinatewise maximum operations), with these two operations as the join and meet operations of the lattice.
2868:
1901:
247:
2398:
2234:
503:
forms a distributive lattice, again with the greatest common divisor as meet and the least common multiple as join. This is a
Boolean algebra
50:
1563:. However, it is still possible that two such terms denote the same element of the distributive lattice. This occurs when there are indices
2715:
148:
over each other. The prototypical examples of such structures are collections of sets for which the lattice operations can be given by set
2120:
1131:
2110:
Balbes and
Dwinger (1975), p. 63 citing Birkhoff, G. "Subdirect unions in universal algebra", Bull. Amer. Math. Soc. SO (1944), 764-768.
554:
believed that all lattices are distributive, that is, distributivity follows from the rest of the lattice axioms. However, independence
1766:. The join of two finite irredundant sets is obtained from their union by removing all redundant sets. Likewise the meet of two sets
2851:
2710:
2191:
1942:
116:
559:
97:
2705:
69:
1154:) between the class of all finite posets and the class of all finite distributive lattices. This bijection can be extended to a
2341:
1895:
1062:
1875:
0, 1, 4, 18, 166, 7579, 7828352, 2414682040996, 56130437228687557907786, 286386577668298411128469151667598498812364 (sequence
1856:
2, 3, 6, 20, 168, 7581, 7828354, 2414682040998, 56130437228687557907788, 286386577668298411128469151667598498812366 (sequence
2423:
54:
1353:
421:
76:
2742:
2662:
382:
2336:
2527:
2456:
1072:
Finally distributivity entails several other pleasant properties. For example, an element of a distributive lattice is
2430:
2418:
2381:
2356:
2331:
2285:
2254:
1224:
1066:
1058:
440:
83:
2361:
2351:
1777:
1464:
2727:
2227:
488:
as join. This lattice also has a least element, namely 1, which therefore serves as the identity element for joins.
2700:
2366:
1155:
1116:
153:
65:
2632:
2259:
451:
1162:
of finite posets. Generalizing this result to infinite lattices, however, requires adding further structure.
2918:
2880:
2863:
1036:, the "pentagon lattice". A lattice is distributive if and only if none of its sublattices is isomorphic to
481:
156:. Indeed, these lattices of sets describe the scenery completely: every distributive lattice is—up to
43:
250:
non-empty finite joins. It is a basic fact of lattice theory that the above condition is equivalent to its
2792:
2408:
1999:
1458:, one can ignore duplicates and order, and represent a join of meets like the one above as a set of sets:
1124:
251:
898:
2770:
2605:
2596:
2465:
2300:
2264:
2220:
1170:
1143:
539:
485:
2346:
881:{\displaystyle (x\wedge y)\vee (y\wedge z)\vee (z\wedge x)=(x\vee y)\wedge (y\vee z)\wedge (z\vee x).}
2858:
2817:
2807:
2797:
2542:
2495:
2475:
2460:
936:
463:
2785:
2696:
2642:
2601:
2591:
2480:
2413:
2376:
511:
433:
429:
1959:
Felsner, Stefan; Knauer, Kolja (2011), "Distributive lattices, polyhedra, and generalized flows",
1003:
90:
2824:
2677:
2586:
2576:
2517:
2435:
2037:
1834:
1159:
1112:
555:
149:
2897:
2737:
2371:
1716:
without changing the interpretation of the whole term. Consequently, a set of finite subsets of
524:
409:
2834:
2812:
2672:
2657:
2637:
2440:
2187:
1938:
1181:
1089:
1054:
551:
532:
459:
393:
A morphism of distributive lattices is just a lattice homomorphism as given in the article on
177:
157:
1065:
member of the class of distributive lattices is the two-element chain. As a corollary, every
2647:
2500:
2149:
2133:
2069:
2029:
1968:
1934:
1658:
1601:
1326:
1239:
1196:
571:
2163:
1982:
1751:
are mutually incomparable (with respect to the subset ordering); that is, when it forms an
1727:
1692:
1631:
1574:
1535:
1418:
1306:
17:
2829:
2612:
2490:
2485:
2470:
2295:
2280:
2159:
2098:
1978:
1845:
1228:
1212:
1100:
1085:
1081:
1077:
1073:
974:
543:
447:
394:
181:
138:
2386:
563:
168:
As in the case of arbitrary lattices, one can choose to consider a distributive lattice
2747:
2732:
2722:
2581:
2559:
2537:
1906:
1752:
744:
504:
477:
145:
1080:, though the latter is in general a weaker property. By duality, the same is true for
2912:
2846:
2802:
2780:
2652:
2522:
2510:
2315:
1451:
1189:
1174:
623:
567:
528:
184:. In the present situation, the algebraic description appears to be more convenient.
142:
2154:
1927:
1053:
An alternative way of stating the same fact is that every distributive lattice is a
2667:
2549:
2532:
2450:
2290:
2243:
2181:
1120:
1093:
173:
2137:
1173:, who first proved it). It characterizes distributive lattices as the lattices of
2003:
1933:. Colloquium Publications (3rd ed.). American Mathematical Society. p.
1833:
The verification that this structure is a distributive lattice with the required
2873:
2566:
2445:
2310:
1455:
1447:
1296:
1151:
1146:
of its join-prime (equivalently: join-irreducible) elements. This establishes a
1007:
Distributive lattice which contains N5 (solid lines, left) and M3 (right) as sub
518:
470:
405:
130:
32:
2841:
2775:
2616:
1973:
1217:
246:
Viewing lattices as partially ordered sets, this says that the meet operation
180:. Both views and their mutual correspondence are discussed in the article on
2892:
2765:
2571:
1147:
1139:
739:
Various equivalent formulations to the above definition exist. For example,
2204:
sequence A006982 (Number of unlabeled distributive lattices with
436:
is a distributive lattice, i.e. "and" distributes over "or" and vice versa.
1762:
is defined on the set of all finite irredundant sets of finite subsets of
462:. Also note that Heyting algebras can be viewed as Lindenbaum algebras of
2687:
2554:
2305:
1177:
455:
2121:
Birkhoff's representation theorem#The partial order of join-irreducibles
1898:— a lattice in which infinite joins distribute over infinite meets
1343:
on a set of generators can be transformed into the following equivalent
626:
of the two prototypical non-distributive lattices. The diamond lattice
2073:
2041:
1184:. This result can be viewed both as a generalization of Stone's famous
496:
2033:
605:
587:
480:
form a (conditionally complete) distributive lattice by taking the
1002:
425:
404:
2200:
2216:
2212:
26:
2203:
2078:
Korselt's non-distributive lattice example is a variant of
1994:
1992:
1877:
1858:
1758:
Now the free distributive lattice over a set of generators
473:
is a distributive lattice with max as join and min as meet.
1840:
The number of elements in free distributive lattices with
1216:). The original lattice is recovered as the collection of
1158:
between homomorphisms of finite distributive lattices and
1167:
Stone's representation theorem for distributive lattices
450:
is a distributive lattice. Especially this includes all
466:, which makes them a special case of the first example.
1405:{\displaystyle M_{1}\lor M_{2}\lor \cdots \lor M_{n},}
1195:
A further important representation was established by
2020:
Charles S. Peirce (1880). "On the
Algebra of Logic".
1848:. These numbers grow rapidly, and are known only for
1780:
1730:
1695:
1661:
1634:
1604:
1577:
1538:
1467:
1421:
1356:
1329:
1309:
1165:
Another early representation theorem is now known as
1138:
distributive lattice is isomorphic to the lattice of
977:
939:
901:
769:
305:
In every lattice, if one defines the order relation
2758:
2686:
2625:
2395:
2324:
2273:
195:if the following additional identity holds for all
57:. Unsourced material may be challenged and removed.
1926:
1825:
1743:
1708:
1677:
1647:
1620:
1590:
1551:
1521:
1434:
1404:
1335:
1315:
1188:and as a specialization of the general setting of
992:
963:
925:
880:
2180:Burris, Stanley N.; Sankappanavar, H.P. (1981).
2101:, and three distinct points on it, respectively.
1201:representation theorem for distributive lattices
1099:Furthermore, every distributive lattice is also
1119:). (The latter structure is sometimes called a
550:Early in the development of the lattice theory
2138:"A ternary operation in distributive lattices"
1826:{\displaystyle \{N\cup M\mid N\in S,M\in T\}.}
1522:{\displaystyle \{N_{1},N_{2},\ldots ,N_{n}\},}
1299:distributive lattice over a set of generators
2228:
2142:Bulletin of the American Mathematical Society
8:
1817:
1781:
1513:
1468:
1134:for distributive lattices states that every
1088:elements. If a lattice is distributive, its
1186:representation theorem for Boolean algebras
2886:Positive cone of a partially ordered group
2235:
2221:
2213:
2002:; Fisch, M. H.; Kloesel, C. J. W. (1989),
2153:
1972:
1779:
1735:
1729:
1700:
1694:
1666:
1660:
1639:
1633:
1609:
1603:
1582:
1576:
1543:
1537:
1507:
1488:
1475:
1466:
1446:. Moreover, since both meet and join are
1426:
1420:
1393:
1374:
1361:
1355:
1328:
1308:
976:
938:
900:
768:
117:Learn how and when to remove this message
2869:Positive cone of an ordered vector space
2005:Writings of Charles S. Peirce: 1879–1884
1902:Duality theory for distributive lattices
1238:
160:—given as such a lattice of sets.
1917:
1871:numbers of elements form the sequence
747:the following holds for all elements
7:
1685:and hence one can safely remove the
55:adding citations to reliable sources
926:{\displaystyle x\wedge z=y\wedge z}
527:given by the inclusion ordering of
2396:Properties & Types (
2097:corresponding to the empty set, a
25:
2852:Positive cone of an ordered field
2058:"Bemerkung zur Algebra der Logik"
1961:European Journal of Combinatorics
1132:Birkhoff's representation theorem
2706:Ordered topological vector space
1442:are finite meets of elements of
604:
586:
31:
2155:10.1090/S0002-9904-1947-08864-9
2022:American Journal of Mathematics
1896:Completely distributive lattice
964:{\displaystyle x\vee z=y\vee z}
892:is distributive if and only if
42:needs additional citations for
1774:is the irredundant version of
872:
860:
854:
842:
836:
824:
818:
806:
800:
788:
782:
770:
1:
2663:Series-parallel partial order
2183:A Course in Universal Algebra
1724:whenever all of its elements
1029:, the "diamond lattice", and
676:, while the pentagon lattice
383:Distributivity (order theory)
2342:Cantor's isomorphism theorem
683:is non-distributive because
633:is non-distributive because
519:lattice-ordered vector space
2382:Szpilrajn extension theorem
2357:Hausdorff maximal principle
2332:Boolean prime ideal theorem
1844:generators is given by the
1225:Boolean prime ideal theorem
1069:has this property as well.
141:in which the operations of
18:Distributive lattice/Proofs
2935:
2728:Topological vector lattice
2008:, Indiana University Press
1925:Birkhoff, Garrett (1967).
1655:will be below the meet of
1235:Free distributive lattices
1220:lower sets of this space.
535:is a distributive lattice.
521:is a distributive lattice.
495:, the set of all positive
443:is a distributive lattice.
2250:
1974:10.1016/j.ejc.2010.07.011
1852: ≤ 9; they are
1628:In this case the meet of
578:Characteristic properties
491:Given a positive integer
172:either as a structure of
2337:Cantor–Bernstein theorem
1753:antichain of finite sets
2881:Partially ordered group
2701:Specialization preorder
1125:representation theorems
1063:subdirectly irreducible
482:greatest common divisor
2367:Kruskal's tree theorem
2362:Knaster–Tarski theorem
2352:Dushnik–Miller theorem
2136:; Kiss, S. A. (1947),
1827:
1745:
1710:
1679:
1678:{\displaystyle N_{j},}
1649:
1622:
1621:{\displaystyle N_{k}.}
1592:
1559:are finite subsets of
1553:
1523:
1436:
1406:
1337:
1336:{\displaystyle \land }
1317:
1292:
1015:
994:
965:
927:
882:
412:
325:, then the inequality
66:"Distributive lattice"
2062:Mathematische Annalen
1828:
1746:
1744:{\displaystyle N_{i}}
1711:
1709:{\displaystyle N_{k}}
1680:
1650:
1648:{\displaystyle N_{k}}
1623:
1593:
1591:{\displaystyle N_{j}}
1554:
1552:{\displaystyle N_{i}}
1524:
1437:
1435:{\displaystyle M_{i}}
1407:
1338:
1318:
1316:{\displaystyle \lor }
1242:
1227:, a weak form of the
1171:Marshall Harvey Stone
1156:duality of categories
1107:Representation theory
1076:if and only if it is
1006:
995:
966:
928:
883:
540:distributive polytope
486:least common multiple
408:
2859:Ordered vector space
1778:
1728:
1693:
1659:
1632:
1602:
1575:
1536:
1465:
1419:
1354:
1327:
1307:
993:{\displaystyle x=y.}
975:
937:
899:
767:
464:intuitionistic logic
135:distributive lattice
51:improve this article
2697:Alexandrov topology
2643:Lexicographic order
2602:Well-quasi-ordering
2186:. Springer-Verlag.
2056:A. Korselt (1894).
1061:, or that the only
471:totally ordered set
2678:Transitive closure
2638:Converse/Transpose
2347:Dilworth's theorem
2074:10.1007/bf01446978
2000:Peirce, Charles S.
1835:universal property
1823:
1741:
1706:
1675:
1645:
1618:
1588:
1549:
1519:
1432:
1402:
1333:
1313:
1293:
1182:topological spaces
1160:monotone functions
1016:
990:
961:
923:
878:
533:integer partitions
460:topological spaces
422:Lindenbaum algebra
413:
2906:
2905:
2864:Partially ordered
2673:Symmetric closure
2658:Reflexive closure
2401:
2134:Birkhoff, Garrett
2085:, with 0, 1, and
1169:(the name honors
1090:covering relation
1059:two-element chain
1057:of copies of the
1055:subdirect product
611:pentagon lattice
552:Charles S. Peirce
313:as usual to mean
285:) for all
178:universal algebra
127:
126:
119:
101:
16:(Redirected from
2926:
2648:Linear extension
2397:
2377:Mirsky's theorem
2237:
2230:
2223:
2214:
2202:
2197:
2168:
2166:
2157:
2130:
2124:
2117:
2111:
2108:
2102:
2077:
2053:
2047:
2045:
2017:
2011:
2009:
1996:
1987:
1985:
1976:
1956:
1950:
1948:
1932:
1922:
1880:
1861:
1846:Dedekind numbers
1832:
1830:
1829:
1824:
1750:
1748:
1747:
1742:
1740:
1739:
1715:
1713:
1712:
1707:
1705:
1704:
1684:
1682:
1681:
1676:
1671:
1670:
1654:
1652:
1651:
1646:
1644:
1643:
1627:
1625:
1624:
1619:
1614:
1613:
1597:
1595:
1594:
1589:
1587:
1586:
1558:
1556:
1555:
1550:
1548:
1547:
1528:
1526:
1525:
1520:
1512:
1511:
1493:
1492:
1480:
1479:
1441:
1439:
1438:
1433:
1431:
1430:
1411:
1409:
1408:
1403:
1398:
1397:
1379:
1378:
1366:
1365:
1342:
1340:
1339:
1334:
1322:
1320:
1319:
1314:
1197:Hilary Priestley
1180:sets of certain
1086:join-irreducible
1078:meet-irreducible
1020:non-distributive
1011:, but not as sub
999:
997:
996:
991:
970:
968:
967:
962:
932:
930:
929:
924:
887:
885:
884:
879:
743:is distributive
733:
697:
675:
647:
608:
593:diamond lattice
590:
538:The points of a
484:as meet and the
122:
115:
111:
108:
102:
100:
59:
35:
27:
21:
2934:
2933:
2929:
2928:
2927:
2925:
2924:
2923:
2909:
2908:
2907:
2902:
2898:Young's lattice
2754:
2682:
2621:
2471:Heyting algebra
2419:Boolean algebra
2391:
2372:Laver's theorem
2320:
2286:Boolean algebra
2281:Binary relation
2269:
2246:
2241:
2194:
2179:
2176:
2174:Further reading
2171:
2132:
2131:
2127:
2118:
2114:
2109:
2105:
2084:
2055:
2054:
2050:
2034:10.2307/2369442
2019:
2018:
2014:
1998:
1997:
1990:
1958:
1957:
1953:
1945:
1924:
1923:
1919:
1915:
1892:
1876:
1857:
1776:
1775:
1731:
1726:
1725:
1720:will be called
1696:
1691:
1690:
1662:
1657:
1656:
1635:
1630:
1629:
1605:
1600:
1599:
1598:is a subset of
1578:
1573:
1572:
1539:
1534:
1533:
1503:
1484:
1471:
1463:
1462:
1422:
1417:
1416:
1389:
1370:
1357:
1352:
1351:
1325:
1324:
1305:
1304:
1237:
1229:axiom of choice
1213:Priestley space
1109:
1067:Boolean lattice
1049:
1042:
1035:
1028:
973:
972:
935:
934:
897:
896:
765:
764:
737:
736:
735:
734:
715:
684:
682:
657:
634:
632:
620:
619:
618:
617:
609:
601:
600:
599:
591:
580:
544:convex polytope
525:Young's lattice
478:natural numbers
448:Heyting algebra
441:Boolean algebra
410:Young's lattice
403:
391:
353:) and its dual
166:
123:
112:
106:
103:
60:
58:
48:
36:
23:
22:
15:
12:
11:
5:
2932:
2930:
2922:
2921:
2919:Lattice theory
2911:
2910:
2904:
2903:
2901:
2900:
2895:
2890:
2889:
2888:
2878:
2877:
2876:
2871:
2866:
2856:
2855:
2854:
2844:
2839:
2838:
2837:
2832:
2825:Order morphism
2822:
2821:
2820:
2810:
2805:
2800:
2795:
2790:
2789:
2788:
2778:
2773:
2768:
2762:
2760:
2756:
2755:
2753:
2752:
2751:
2750:
2745:
2743:Locally convex
2740:
2735:
2725:
2723:Order topology
2720:
2719:
2718:
2716:Order topology
2713:
2703:
2693:
2691:
2684:
2683:
2681:
2680:
2675:
2670:
2665:
2660:
2655:
2650:
2645:
2640:
2635:
2629:
2627:
2623:
2622:
2620:
2619:
2609:
2599:
2594:
2589:
2584:
2579:
2574:
2569:
2564:
2563:
2562:
2552:
2547:
2546:
2545:
2540:
2535:
2530:
2528:Chain-complete
2520:
2515:
2514:
2513:
2508:
2503:
2498:
2493:
2483:
2478:
2473:
2468:
2463:
2453:
2448:
2443:
2438:
2433:
2428:
2427:
2426:
2416:
2411:
2405:
2403:
2393:
2392:
2390:
2389:
2384:
2379:
2374:
2369:
2364:
2359:
2354:
2349:
2344:
2339:
2334:
2328:
2326:
2322:
2321:
2319:
2318:
2313:
2308:
2303:
2298:
2293:
2288:
2283:
2277:
2275:
2271:
2270:
2268:
2267:
2262:
2257:
2251:
2248:
2247:
2242:
2240:
2239:
2232:
2225:
2217:
2211:
2210:
2198:
2192:
2175:
2172:
2170:
2169:
2148:(1): 749–752,
2125:
2112:
2103:
2082:
2048:
2046:, p. 33 bottom
2012:
1988:
1951:
1943:
1929:Lattice Theory
1916:
1914:
1911:
1910:
1909:
1907:Spectral space
1904:
1899:
1891:
1888:
1887:
1886:
1868:
1867:
1822:
1819:
1816:
1813:
1810:
1807:
1804:
1801:
1798:
1795:
1792:
1789:
1786:
1783:
1738:
1734:
1703:
1699:
1674:
1669:
1665:
1642:
1638:
1617:
1612:
1608:
1585:
1581:
1546:
1542:
1530:
1529:
1518:
1515:
1510:
1506:
1502:
1499:
1496:
1491:
1487:
1483:
1478:
1474:
1470:
1429:
1425:
1413:
1412:
1401:
1396:
1392:
1388:
1385:
1382:
1377:
1373:
1369:
1364:
1360:
1332:
1312:
1236:
1233:
1108:
1105:
1047:
1040:
1033:
1026:
1001:
1000:
989:
986:
983:
980:
960:
957:
954:
951:
948:
945:
942:
922:
919:
916:
913:
910:
907:
904:
877:
874:
871:
868:
865:
862:
859:
856:
853:
850:
847:
844:
841:
838:
835:
832:
829:
826:
823:
820:
817:
814:
811:
808:
805:
802:
799:
796:
793:
790:
787:
784:
781:
778:
775:
772:
745:if and only if
680:
656:≠ 0 = 0 ∨ 0 =
630:
624:Hasse diagrams
622:
621:
615:
610:
603:
602:
597:
592:
585:
584:
583:
582:
581:
579:
576:
558:were given by
548:
547:
536:
529:Young diagrams
522:
515:
505:if and only if
489:
474:
467:
454:and hence all
444:
437:
402:
399:
390:
387:
303:
302:
244:
243:
165:
162:
125:
124:
39:
37:
30:
24:
14:
13:
10:
9:
6:
4:
3:
2:
2931:
2920:
2917:
2916:
2914:
2899:
2896:
2894:
2891:
2887:
2884:
2883:
2882:
2879:
2875:
2872:
2870:
2867:
2865:
2862:
2861:
2860:
2857:
2853:
2850:
2849:
2848:
2847:Ordered field
2845:
2843:
2840:
2836:
2833:
2831:
2828:
2827:
2826:
2823:
2819:
2816:
2815:
2814:
2811:
2809:
2806:
2804:
2803:Hasse diagram
2801:
2799:
2796:
2794:
2791:
2787:
2784:
2783:
2782:
2781:Comparability
2779:
2777:
2774:
2772:
2769:
2767:
2764:
2763:
2761:
2757:
2749:
2746:
2744:
2741:
2739:
2736:
2734:
2731:
2730:
2729:
2726:
2724:
2721:
2717:
2714:
2712:
2709:
2708:
2707:
2704:
2702:
2698:
2695:
2694:
2692:
2689:
2685:
2679:
2676:
2674:
2671:
2669:
2666:
2664:
2661:
2659:
2656:
2654:
2653:Product order
2651:
2649:
2646:
2644:
2641:
2639:
2636:
2634:
2631:
2630:
2628:
2626:Constructions
2624:
2618:
2614:
2610:
2607:
2603:
2600:
2598:
2595:
2593:
2590:
2588:
2585:
2583:
2580:
2578:
2575:
2573:
2570:
2568:
2565:
2561:
2558:
2557:
2556:
2553:
2551:
2548:
2544:
2541:
2539:
2536:
2534:
2531:
2529:
2526:
2525:
2524:
2523:Partial order
2521:
2519:
2516:
2512:
2511:Join and meet
2509:
2507:
2504:
2502:
2499:
2497:
2494:
2492:
2489:
2488:
2487:
2484:
2482:
2479:
2477:
2474:
2472:
2469:
2467:
2464:
2462:
2458:
2454:
2452:
2449:
2447:
2444:
2442:
2439:
2437:
2434:
2432:
2429:
2425:
2422:
2421:
2420:
2417:
2415:
2412:
2410:
2409:Antisymmetric
2407:
2406:
2404:
2400:
2394:
2388:
2385:
2383:
2380:
2378:
2375:
2373:
2370:
2368:
2365:
2363:
2360:
2358:
2355:
2353:
2350:
2348:
2345:
2343:
2340:
2338:
2335:
2333:
2330:
2329:
2327:
2323:
2317:
2316:Weak ordering
2314:
2312:
2309:
2307:
2304:
2302:
2301:Partial order
2299:
2297:
2294:
2292:
2289:
2287:
2284:
2282:
2279:
2278:
2276:
2272:
2266:
2263:
2261:
2258:
2256:
2253:
2252:
2249:
2245:
2238:
2233:
2231:
2226:
2224:
2219:
2218:
2215:
2209:
2207:
2199:
2195:
2193:3-540-90578-2
2189:
2185:
2184:
2178:
2177:
2173:
2165:
2161:
2156:
2151:
2147:
2143:
2139:
2135:
2129:
2126:
2122:
2116:
2113:
2107:
2104:
2100:
2096:
2092:
2088:
2081:
2075:
2071:
2067:
2063:
2059:
2052:
2049:
2043:
2039:
2035:
2031:
2027:
2023:
2016:
2013:
2007:
2006:
2001:
1995:
1993:
1989:
1984:
1980:
1975:
1970:
1966:
1962:
1955:
1952:
1949:§6, Theorem 9
1946:
1944:0-8218-1025-1
1940:
1936:
1931:
1930:
1921:
1918:
1912:
1908:
1905:
1903:
1900:
1897:
1894:
1893:
1889:
1884:
1879:
1874:
1873:
1872:
1865:
1860:
1855:
1854:
1853:
1851:
1847:
1843:
1838:
1836:
1820:
1814:
1811:
1808:
1805:
1802:
1799:
1796:
1793:
1790:
1787:
1784:
1773:
1769:
1765:
1761:
1756:
1754:
1736:
1732:
1723:
1719:
1701:
1697:
1688:
1672:
1667:
1663:
1640:
1636:
1615:
1610:
1606:
1583:
1579:
1570:
1566:
1562:
1544:
1540:
1516:
1508:
1504:
1500:
1497:
1494:
1489:
1485:
1481:
1476:
1472:
1461:
1460:
1459:
1457:
1453:
1449:
1445:
1427:
1423:
1399:
1394:
1390:
1386:
1383:
1380:
1375:
1371:
1367:
1362:
1358:
1350:
1349:
1348:
1346:
1330:
1310:
1302:
1298:
1290:
1286:
1282:
1278:
1274:
1270:
1266:
1262:
1258:
1254:
1250:
1246:
1241:
1234:
1232:
1230:
1226:
1221:
1219:
1215:
1214:
1209:
1208:
1202:
1198:
1193:
1191:
1190:Stone duality
1187:
1183:
1179:
1176:
1172:
1168:
1163:
1161:
1157:
1153:
1149:
1145:
1141:
1137:
1133:
1129:
1126:
1122:
1118:
1114:
1106:
1104:
1102:
1097:
1095:
1091:
1087:
1083:
1079:
1075:
1070:
1068:
1064:
1060:
1056:
1051:
1046:
1039:
1032:
1025:
1022:lattices are
1021:
1018:The simplest
1014:
1010:
1005:
987:
984:
981:
978:
971:always imply
958:
955:
952:
949:
946:
943:
940:
920:
917:
914:
911:
908:
905:
902:
895:
894:
893:
891:
875:
869:
866:
863:
857:
851:
848:
845:
839:
833:
830:
827:
821:
815:
812:
809:
803:
797:
794:
791:
785:
779:
776:
773:
762:
758:
754:
750:
746:
742:
731:
727:
723:
719:
713:
709:
705:
701:
695:
691:
687:
679:
673:
669:
665:
661:
655:
651:
645:
641:
637:
629:
625:
614:
607:
596:
589:
577:
575:
573:
569:
565:
561:
557:
553:
545:
541:
537:
534:
531:representing
530:
526:
523:
520:
516:
513:
509:
506:
502:
498:
494:
490:
487:
483:
479:
475:
472:
468:
465:
461:
457:
453:
449:
445:
442:
438:
435:
431:
428:that support
427:
423:
419:
418:
417:
411:
407:
400:
398:
396:
388:
386:
384:
380:
376:
372:
368:
364:
360:
356:
352:
348:
344:
340:
336:
332:
328:
324:
320:
316:
312:
308:
300:
296:
292:
288:
284:
280:
276:
272:
268:
264:
260:
257:
256:
255:
253:
249:
241:
237:
233:
229:
225:
221:
217:
214:
213:
212:
210:
206:
202:
198:
194:
190:
185:
183:
179:
175:
171:
163:
161:
159:
155:
151:
147:
144:
143:join and meet
140:
136:
132:
121:
118:
110:
99:
96:
92:
89:
85:
82:
78:
75:
71:
68: –
67:
63:
62:Find sources:
56:
52:
46:
45:
40:This article
38:
34:
29:
28:
19:
2690:& Orders
2668:Star product
2597:Well-founded
2550:Prefix order
2506:Distributive
2505:
2496:Complemented
2466:Foundational
2431:Completeness
2387:Zorn's lemma
2291:Cyclic order
2274:Key concepts
2244:Order theory
2205:
2182:
2145:
2141:
2128:
2115:
2106:
2094:
2090:
2086:
2079:
2065:
2061:
2051:
2025:
2021:
2015:
2004:
1967:(1): 45–59,
1964:
1960:
1954:
1928:
1920:
1869:
1849:
1841:
1839:
1837:is routine.
1771:
1767:
1763:
1759:
1757:
1721:
1717:
1686:
1568:
1564:
1560:
1531:
1443:
1414:
1344:
1300:
1294:
1288:
1284:
1280:
1276:
1272:
1268:
1264:
1260:
1256:
1252:
1248:
1244:
1222:
1211:
1204:
1194:
1164:
1135:
1130:
1121:ring of sets
1117:intersection
1110:
1098:
1094:median graph
1071:
1052:
1044:
1037:
1030:
1023:
1019:
1017:
1012:
1008:
889:
760:
756:
752:
748:
740:
738:
729:
725:
721:
717:
711:
707:
703:
699:
693:
689:
685:
677:
671:
667:
663:
659:
653:
649:
643:
639:
635:
627:
612:
594:
549:
507:
500:
492:
458:lattices of
414:
392:
378:
374:
370:
366:
362:
358:
354:
350:
346:
342:
338:
334:
330:
326:
322:
318:
314:
310:
306:
304:
298:
294:
290:
286:
282:
278:
274:
270:
266:
262:
258:
245:
239:
235:
231:
227:
223:
219:
215:
208:
204:
200:
196:
193:distributive
192:
188:
186:
174:order theory
169:
167:
154:intersection
134:
128:
113:
104:
94:
87:
80:
73:
61:
49:Please help
44:verification
41:
2874:Riesz space
2835:Isomorphism
2711:Normal cone
2633:Composition
2567:Semilattice
2476:Homogeneous
2461:Equivalence
2311:Total order
2068:: 156–157.
2010:, p. xlvii.
1722:irredundant
1452:commutative
1448:associative
1345:normal form
1207:Stone space
1152:isomorphism
888:Similarly,
512:square-free
434:disjunction
430:conjunction
187:A lattice (
158:isomorphism
131:mathematics
2842:Order type
2776:Cofinality
2617:Well-order
2592:Transitive
2481:Idempotent
2414:Asymmetric
1913:References
1571:such that
1532:where the
1456:idempotent
1140:lower sets
1082:join-prime
1074:meet-prime
164:Definition
146:distribute
77:newspapers
2893:Upper set
2830:Embedding
2766:Antichain
2587:Tolerance
2577:Symmetric
2572:Semiorder
2518:Reflexive
2436:Connected
2208:elements)
2028:: 15–57.
1812:∈
1800:∈
1794:∣
1788:∪
1687:redundant
1498:…
1387:∨
1384:⋯
1381:∨
1368:∨
1331:∧
1311:∨
1148:bijection
1113:set union
956:∨
944:∨
918:∧
906:∧
867:∨
858:∧
849:∨
840:∧
831:∨
813:∧
804:∨
795:∧
786:∨
777:∧
562:, Voigt,
389:Morphisms
248:preserves
191:,∨,∧) is
2913:Category
2688:Topology
2555:Preorder
2538:Eulerian
2501:Complete
2451:Directed
2441:Covering
2306:Preorder
2265:Category
2260:Glossary
1890:See also
1205:ordered
1092:forms a
1043:or
572:Dedekind
560:Schröder
497:divisors
456:open set
424:of most
401:Examples
395:lattices
182:lattices
107:May 2011
2793:Duality
2771:Cofinal
2759:Related
2738:Fréchet
2615:)
2491:Bounded
2486:Lattice
2459:)
2457:Partial
2325:Results
2296:Lattice
2164:0021540
2042:2369442
1983:2727459
1881:in the
1878:A007153
1862:in the
1859:A000372
1199:in her
1175:compact
1150:(up to
1142:of the
1101:modular
1013:lattice
568:Korselt
452:locales
139:lattice
91:scholar
2818:Subnet
2798:Filter
2748:Normed
2733:Banach
2699:&
2606:Better
2543:Strict
2533:Graded
2424:topics
2255:Topics
2190:
2162:
2040:
1981:
1941:
1415:where
1218:clopen
1136:finite
710:= 0 ∨
702:∧ 1 =
652:∧ 1 =
570:, and
564:Lüroth
556:proofs
469:Every
446:Every
439:Every
426:logics
293:, and
203:, and
176:or of
93:
86:
79:
72:
64:
2808:Ideal
2786:Graph
2582:Total
2560:Total
2446:Dense
2038:JSTOR
1283:) ∧ (
1275:) ∧ (
1267:) = (
1259:) ∨ (
1251:) ∨ (
1144:poset
724:) ∨ (
666:) ∨ (
373:) ∧ (
365:) ≤ (
345:) ∨ (
337:) ≥ (
277:) ∧ (
269:) = (
234:) ∨ (
226:) = (
150:union
137:is a
133:, a
98:JSTOR
84:books
2399:list
2201:OEIS
2188:ISBN
2119:See
2099:line
1939:ISBN
1883:OEIS
1864:OEIS
1770:and
1689:set
1567:and
1454:and
1323:and
1297:free
1295:The
1210:(or
1178:open
1115:and
1084:and
933:and
476:The
432:and
420:The
252:dual
152:and
70:news
2813:Net
2613:Pre
2150:doi
2070:doi
2030:doi
1969:doi
1009:set
759:in
688:∧ (
638:∧ (
542:(a
510:is
499:of
357:∨ (
329:∧ (
297:in
261:∨ (
218:∧ (
207:in
129:In
53:by
2915::
2160:MR
2158:,
2146:53
2144:,
2140:,
2093:,
2089:,
2066:44
2064:.
2060:.
2036:.
2024:.
1991:^
1979:MR
1977:,
1965:32
1963:,
1937:.
1935:11
1885:).
1866:).
1755:.
1450:,
1347::
1291:).
1287:∨
1279:∨
1271:∨
1263:∧
1255:∧
1247:∧
1231:.
1192:.
1103:.
1096:.
763::
755:,
751:,
728:∧
720:∧
714:=
706:≠
698:=
692:∨
670:∧
662:∧
648:=
642:∨
574:.
566:,
517:A
385:.
377:∨
369:∨
361:∧
349:∧
341:∧
333:∨
289:,
281:∨
273:∨
265:∧
254::
242:).
238:∧
230:∧
222:∨
211::
199:,
2611:(
2608:)
2604:(
2455:(
2402:)
2236:e
2229:t
2222:v
2206:n
2196:.
2167:.
2152::
2123:.
2095:z
2091:y
2087:x
2083:3
2080:M
2076:.
2072::
2044:.
2032::
2026:3
1986:.
1971::
1947:.
1850:n
1842:n
1821:.
1818:}
1815:T
1809:M
1806:,
1803:S
1797:N
1791:M
1785:N
1782:{
1772:T
1768:S
1764:G
1760:G
1737:i
1733:N
1718:G
1702:k
1698:N
1673:,
1668:j
1664:N
1641:k
1637:N
1616:.
1611:k
1607:N
1584:j
1580:N
1569:k
1565:j
1561:G
1545:i
1541:N
1517:,
1514:}
1509:n
1505:N
1501:,
1495:,
1490:2
1486:N
1482:,
1477:1
1473:N
1469:{
1444:G
1428:i
1424:M
1400:,
1395:n
1391:M
1376:2
1372:M
1363:1
1359:M
1301:G
1289:z
1285:y
1281:z
1277:x
1273:y
1269:x
1265:z
1261:y
1257:z
1253:x
1249:y
1245:x
1048:5
1045:N
1041:3
1038:M
1034:5
1031:N
1027:3
1024:M
988:.
985:y
982:=
979:x
959:z
953:y
950:=
947:z
941:x
921:z
915:y
912:=
909:z
903:x
890:L
876:.
873:)
870:x
864:z
861:(
855:)
852:z
846:y
843:(
837:)
834:y
828:x
825:(
822:=
819:)
816:x
810:z
807:(
801:)
798:z
792:y
789:(
783:)
780:y
774:x
771:(
761:L
757:z
753:y
749:x
741:L
732:)
730:z
726:x
722:y
718:x
716:(
712:z
708:z
704:x
700:x
696:)
694:z
690:y
686:x
681:5
678:N
674:)
672:z
668:x
664:y
660:x
658:(
654:x
650:x
646:)
644:z
640:y
636:x
631:3
628:M
616:5
613:N
598:3
595:M
514:.
508:n
501:n
493:n
379:z
375:x
371:y
367:x
363:z
359:y
355:x
351:z
347:x
343:y
339:x
335:z
331:y
327:x
323:p
321:=
319:q
317:∧
315:p
311:q
309:≤
307:p
301:.
299:L
295:z
291:y
287:x
283:z
279:x
275:y
271:x
267:z
263:y
259:x
240:z
236:x
232:y
228:x
224:z
220:y
216:x
209:L
205:z
201:y
197:x
189:L
170:L
120:)
114:(
109:)
105:(
95:·
88:·
81:·
74:·
47:.
20:)
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.