5496:
5279:
5517:
5485:
644:, under appropriate conditions followed from the version of compactness that was formulated in terms of the existence of finite subcovers. It was this notion of compactness that became the dominant one, because it was not only a stronger property, but it could be formulated in a more general setting with a minimum of additional technical machinery, as it relied only on the structure of the open sets in a space.
5554:
5527:
5507:
486:: the sequence was placed into an interval that was then divided into two equal parts, and a part containing infinitely many terms of the sequence was selected. The process could then be repeated by dividing the resulting smaller interval into smaller and smaller parts – until it closes down on the desired limit point. The full significance of
38:
3173:
to of mapping each infinity to its corresponding unit and every real number to its sign multiplied by the unique number in the positive part of interval that results in its absolute value when divided by one minus itself, and since homeomorphisms preserve covers, the Heine-Borel property can be
814:
are compact since for any infinite number of points sampled from a disk, some subset of those points must get arbitrarily close either to a point within the disc, or to a point on the boundary. However, an open disk is not compact, because a sequence of points can tend to the boundary – without
2598:
holds for such spaces: a continuous real-valued function on a nonempty compact space is bounded above and attains its supremum. (Slightly more generally, this is true for an upper semicontinuous function.) As a sort of converse to the above statements, the pre-image of a compact space under a
521:
sequence of functions from a suitable family of functions. The uniform limit of this sequence then played precisely the same role as
Bolzano's "limit point". Towards the beginning of the twentieth century, results similar to that of Arzelà and Ascoli began to accumulate in the area of
3699:
if, for any positive epsilon, there exists a compact subset containing all but at most epsilon of the mass of each of the measures. Helly's theorem then asserts that a collection of probability measures is relatively compact for the vague topology if and only if it is
355:, those same sets of points would not accumulate to any point of it, so the open unit interval is not compact. Although subsets (subspaces) of Euclidean space can be compact, the entire space itself is not compact, since it is not bounded. For example, considering
898:
of each point of the space – and to extend it to information that holds globally throughout the space. An example of this phenomenon is
Dirichlet's theorem, to which it was originally applied by Heine, that a continuous function on a compact interval is
581:. In the course of the proof, he made use of a lemma that from any countable cover of the interval by smaller open intervals, it was possible to select a finite number of these that also covered it. The significance of this lemma was recognized by
815:
getting arbitrarily close to any point in the interior. Likewise, spheres are compact, but a sphere missing a point is not since a sequence of points can still tend to the missing point, thereby not getting arbitrarily close to any point
1525:
is a metric space, the conditions in the next subsection also apply to all of its subsets. Of all of the equivalent conditions, it is in practice easiest to verify that a subset is closed and bounded, for example, for a closed
4959:
2131:
2935:
4670:
3562:
1241:
1177:
1061:
997:
248:
states that a subset of
Euclidean space is compact in this sequential sense if and only if it is closed and bounded. Thus, if one chooses an infinite number of points in the closed
478:) had been aware that any bounded sequence of points (in the line or plane, for instance) has a subsequence that must eventually get arbitrarily close to some other point, called a
3052:
419:. In general topological spaces, however, these notions of compactness are not necessarily equivalent. The most useful notion — and the standard definition of the unqualified term
1275:
3376:
A subset of the Banach space of real-valued continuous functions on a compact
Hausdorff space is relatively compact if and only if it is equicontinuous and pointwise bounded (
2776:
382:
1462:
4115:
4061:
4034:
4002:
3970:
3679:
3628:
3606:
3340:
3245:
3152:
3106:
3081:
2837:
2741:
1838:– these three conditions are equivalent for metric spaces. The converse is not true; e.g., a countable discrete space satisfies these three conditions, but is not compact.
156:
127:
5062:
3656:
3284:
612:
about a set (such as the continuity of a function) to global information about the set (such as the uniform continuity of a function). This sentiment was expressed by
202:
179:
407:
exemplify applications of this notion of compactness to classical analysis. Following its initial introduction, various equivalent notions of compactness, including
4648:
Rein analytischer Beweis des
Lehrsatzes, dass zwischen je zwey Werthen, die ein entgegengesetzes Resultat gewähren, wenigstens eine reele Wurzel der Gleichung liege
501:
rather than just numbers or geometrical points. The idea of regarding functions as themselves points of a generalized space dates back to the investigations of
5557:
787:
get arbitrarily close to 0, while the even-numbered ones get arbitrarily close to 1. The given example sequence shows the importance of including the
656:
is compact; a finite subcover can be obtained by selecting, for each point, an open set containing it. A nontrivial example of a compact space is the (closed)
4831:
4646:
4603:
Arkhangel'skii, A.V.; Fedorchuk, V.V. (1990). "The basic concepts and constructions of general topology". In
Arkhangel'skii, A.V.; Pontrjagin, L.S. (eds.).
2214:, this is equivalent to every maximal ideal being the kernel of an evaluation homomorphism. There are pseudocompact spaces that are not compact, though.
470:
In the 19th century, several disparate mathematical properties were understood that would later be seen as consequences of compactness. On the one hand,
4655:
Purely analytic proof of the theorem that between any two values which give results of opposite sign, there lies at least one real root of the equation
5588:
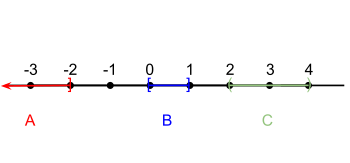
104:(0,1) would not be compact because it excludes the limiting values of 0 and 1, whereas the closed interval would be compact. Similarly, the space of
2784:. Using the one-point compactification, one can also easily construct compact spaces which are not Hausdorff, by starting with a non-Hausdorff space.
819:
the space. Lines and planes are not compact, since one can take a set of equally-spaced points in any given direction without approaching any point.
447:– that is, in a neighborhood of each point – into corresponding statements that hold throughout the space, and many theorems are of this character.
3061:
but this cover does not have a finite subcover. Here, the sets are open in the subspace topology even though they are not open as subsets of
2718:
5092:
5010:
4948:
4897:
4768:
4720:
4612:
1483:
Bourbaki defines a compact space (quasi-compact space) as a topological space where each filter has a cluster point (i.e., 8. in the above).
795:
must be in the space itself — an open (or half-open) interval of the real numbers is not compact. It is also crucial that the interval be
538:
coming from solutions of integral equations, Schmidt had shown that a property analogous to the Arzelà–Ascoli theorem held in the sense of
4413:" but is used because "collection of open subsets" is less awkward than "set of open subsets". Similarly, "subcollection" means "subset".
2086:
252:, some of those points will get arbitrarily close to some real number in that space. For instance, some of the numbers in the sequence
214:
be compact, since it contains both infinities. There are many ways to make this heuristic notion precise. These ways usually agree in a
431:" the space in the sense that each point of the space lies in some set contained in the family. This more subtle notion, introduced by
5578:
5191:
3108:
of all real numbers is not compact as there is a cover of open intervals that does not have a finite subcover. For example, intervals
1198:
1134:
5545:
5540:
5119:
565:
However, a different notion of compactness altogether had also slowly emerged at the end of the 19th century from the study of the
840:
487:
245:
5535:
5029:
3807:
Hausdorff spaces, form the abstract framework in which these spectra are studied. Such spaces are also useful in the study of
3630:
arises in this manner, as the spectrum of some bounded linear operator. For instance, a diagonal operator on the
Hilbert space
1335:
3195:
is compact. This is not true for infinite dimensions; in fact, a normed vector space is finite-dimensional if and only if its
2884:
2624:
2591:
2378:
1018:
954:
5437:
4885:
4634:
2288:
3377:
510:
400:
3881:
3796:
1360:
28:
5082:
4629:
3912:
3461:
1422:
895:
562:
to refer to this general phenomenon (he used the term already in his 1904 paper which led to the famous 1906 thesis).
4592:
Koninklijke
Nederlandse Akademie van Wetenschappen te Amsterdam, Proceedings of the Section of Mathematical Sciences
5445:
3866:
3804:
2616:
1790:
590:
551:
408:
903:; here, continuity is a local property of the function, and uniform continuity the corresponding global property.
5583:
4191:
3851:
3750:
3158:
2420:
2191:
479:
2391:
is not
Hausdorff then the intersection of two compact subsets may fail to be compact (see footnote for example).
605:, as the result is now known, is another special property possessed by closed and bounded sets of real numbers.
5516:
5244:
4271:
2688:
2471:
2211:
1407:
886:
below). That this form of compactness holds for closed and bounded subsets of
Euclidean space is known as the
653:
2871:
2384:
The intersection of any non-empty collection of compact subsets of a Hausdorff space is compact (and closed);
1515:
887:
602:
497:
In the 1880s, it became clear that results similar to the Bolzano–Weierstrass theorem could be formulated for
42:
351:
accumulate to 0 (while others accumulate to 1). Since neither 0 nor 1 are members of the open unit interval
5530:
3576:
3572:
2811:
2322:
1857:
1588:
404:
3202:
On the other hand, the closed unit ball of the dual of a normed space is compact for the weak-* topology. (
5465:
5460:
5386:
5263:
5251:
5224:
5184:
5078:
3896:
2957:
2850:
on an uncountable set, no infinite set is compact. Like the previous example, the space as a whole is not
2401:
1827:
1527:
852:
844:
641:
443:. In spaces that are compact in this sense, it is often possible to patch together information that holds
101:
1248:
5307:
5234:
4311:
3901:
3784:(except in trivial cases). In algebraic geometry, such topological spaces are examples of quasi-compact
3358:
2595:
2249:
2241:
205:
5495:
5163:
2752:
1860:
of the space is an open cover which admits no finite subcover. It is complete but not totally bounded.
1334:
has a sub-base such that every cover of the space, by members of the sub-base, has a finite subcover (
358:
5455:
5407:
5381:
5229:
4877:
3785:
3718:
3203:
3165:
compact; note that the cover described above would never reach the points at infinity and thus would
2847:
2840:
2788:
2465:
is not Hausdorff then the closure of a compact set may fail to be compact (see footnote for example).
2416:
1655:
1382:
900:
667:. If one chooses an infinite number of distinct points in the unit interval, then there must be some
578:
535:
230:
1435:
490:, and its method of proof, would not emerge until almost 50 years later when it was rediscovered by
94:. The idea is that a compact space has no "punctures" or "missing endpoints", i.e., it includes all
5506:
5302:
4889:
4750:
4730:
4624:
3856:
3685:
3456:
3402:
3192:
2800:
2796:
2792:
2199:
1647:
856:
792:
788:
574:
518:
514:
483:
412:
219:
95:
4098:
4044:
4017:
3985:
3953:
3662:
3611:
3589:
3303:
3228:
3135:
3089:
3064:
2820:
2814:
of the space is an open cover which admits no finite subcover. Finite discrete spaces are compact.
2724:
139:
110:
5500:
5450:
5371:
5361:
5239:
5219:
5138:
5054:
4856:
3886:
3861:
3769:
2371:
1066:
827:
Various definitions of compactness may apply, depending on the level of generality. A subset of
811:
668:
621:
5470:
4822:
392:
3634:
3256:
3191:
is compact. Again from the Heine–Borel theorem, the closed unit ball of any finite-dimensional
2717:
Hausdorff space can be turned into a compact space by adding a single point to it, by means of
890:. Compactness, when defined in this manner, often allows one to take information that is known
5593:
5488:
5354:
5312:
5177:
5115:
5088:
5074:
5006:
4944:
4903:
4893:
4764:
4716:
4608:
4410:
3891:
3876:
3871:
3758:
3710:
3370:
2855:
2707:
1835:
1677:
1375:
1367:
1349:
1345:
1114:
912:
867:
633:
632:, formulated Heine–Borel compactness in a way that could be applied to the modern notion of a
617:
523:
459:
237:
223:
184:
161:
130:
1877:(i.e. a totally ordered set equipped with the order topology), the following are equivalent:
5268:
5214:
5044:
4998:
4978:
4848:
4840:
4794:
4778:
4708:
4689:
4679:
4583:
3777:
3773:
3754:
3714:
3362:
3196:
2946:
2700:
2675:
2412:
2397:
2313:
2156:
1933:
1555:
1070:
875:
625:
566:
547:
539:
506:
491:
432:
428:
75:
5102:
5020:
4990:
4742:
4702:
395:
in 1906 to generalize the Bolzano–Weierstrass theorem from spaces of geometrical points to
5327:
5322:
5098:
5016:
4986:
4911:
4738:
4693:
4642:
3917:
3906:
3833:
3815:
3808:
3792:
3781:
3631:
2851:
2714:
2696:
2635:
is an open dense subspace of a compact Hausdorff space having at most one point more than
2628:
2437:
2405:
2252:
allows for the following alternative characterization of compactness: a topological space
2245:
1853:
1831:
1626:
1592:
1522:
1499:
1082:
828:
671:
among these points in that interval. For instance, the odd-numbered terms of the sequence
471:
105:
91:
2478:. However, every non-Hausdorff TVS contains compact (and thus complete) subsets that are
851:
that converges to a point in the set. Various equivalent notions of compactness, such as
3213:
is compact. In fact, every compact metric space is a continuous image of the Cantor set.
1921:
An ordered space satisfying (any one of) these conditions is called a complete lattice.
5417:
5349:
4661:
3819:
3725:
3689:
3584:
3178:
2807:
2663:
2475:
1465:
891:
807:, of which no sub-sequence ultimately gets arbitrarily close to any given real number.
609:
594:
582:
531:
498:
444:
396:
5572:
5427:
5337:
4937:
4860:
4806:
4758:
4587:
3762:
3170:
2862:
2544:
2533:
2267:
2173:
2169:
1629:
1596:
657:
640:
showed that the earlier version of compactness due to Fréchet, now called (relative)
629:
543:
527:
502:
436:
249:
5520:
3695:
A collection of probability measures on the Borel sets of Euclidean space is called
569:, which was seen as fundamental for the rigorous formulation of analysis. In 1870,
558:, had distilled the essence of the Bolzano–Weierstrass property and coined the term
5412:
5332:
5278:
4932:
4754:
3837:
3826:
3739:
3732:
3580:
2540:
860:
570:
416:
215:
5087:(Dover Publications reprint of 1978 ed.). Berlin, New York: Springer-Verlag.
2354:
contains infinitesimals, which are infinitely close to 0, which is not a point of
1844:
is closed and bounded (as a subset of any metric space whose restricted metric is
5155:
4386:
4266:
5510:
5422:
3800:
3249:
from the real number line to the closed unit interval, and define a topology on
2659:
2648:
1511:
866:
In contrast, the different notions of compactness are not equivalent in general
848:
836:
796:
664:
241:
134:
129:
is not compact, because it has infinitely many "punctures" corresponding to the
87:
71:
5366:
5297:
5256:
5159:
4852:
4712:
4041:
with the topology generated by the following basic open sets: every subset of
3746:
3210:
2879:
2600:
1699:
1507:
1469:
1317:
931:
923:
832:
440:
83:
454:
is sometimes used as a synonym for compact space, but also often refers to a
17:
5391:
4907:
2692:
1849:
4982:
4915:
2699:(only finitely many open sets) is compact; this includes in particular the
1650:(also called weakly countably compact); that is, every infinite subset of
5376:
5344:
5293:
5200:
3185:
2217:
In general, for non-pseudocompact spaces there are always maximal ideals
1614:
1325:
934:
879:
513:, was a generalization of the Bolzano–Weierstrass theorem to families of
424:
2532:
A continuous bijection from a compact space into a Hausdorff space is a
517:, the precise conclusion of which was that it was possible to extract a
5137:
Sundström, Manya Raman (2010). "A pedagogical history of compactness".
5058:
4844:
4684:
4665:
608:
This property was significant because it allowed for the passage from
4607:. Encyclopedia of the Mathematical Sciences. Vol. 17. Springer.
1492:
5143:
5049:
4826:
4811:
Atti della R. Accad. Dei Lincei Memorie della Cl. Sci. Fis. Mat. Nat
2810:
with an infinite number of points is compact. The collection of all
59:
is not compact because it is not closed (but bounded). The interval
589:), and it was generalized to arbitrary collections of intervals by
158:
is not compact either, because it excludes the two limiting values
37:
2126:{\displaystyle \operatorname {ev} _{p}\colon C(X)\to \mathbb {R} }
41:
Per the compactness criteria for Euclidean space as stated in the
36:
4961:
Leçons sur l'intégration et la recherche des fonctions primitives
1924:
In addition, the following are equivalent for all ordered spaces
550:
as an offshoot of the general notion of a compact space. It was
3788:, "quasi" referring to the non-Hausdorff nature of the topology.
3357:. There is only one such topology; it is called the topology of
1856:
is closed and bounded but not compact, as the collection of all
870:, and the most useful notion of compactness – originally called
5173:
4927:. Graduate Texts in Mathematics. Vol. 27. Springer-Verlag.
4797:(1882–1883). "Un'osservazione intorno alle serie di funzioni".
1475:
Every open cover linearly ordered by subset inclusion contains
2954:
is not compact: the sets of rational numbers in the intervals
4969:
Mack, John (1967). "Directed covers and paracompact spaces".
4809:(1883–1884). "Le curve limiti di una varietà data di curve".
3780:(that is, the set of all prime ideals) is compact, but never
1848:). The converse may fail for a non-Euclidean space; e.g. the
1113:
is said to be compact if it is compact as a subspace (in the
423:— is phrased in terms of the existence of finite families of
1997:
Every decreasing nested sequence of nonempty closed subsets
1705:
Every decreasing nested sequence of nonempty closed subsets
5169:
4554:
4542:
4530:
4482:
4735:
The history of the calculus and its conceptual development
4095:
are both compact subsets but their intersection, which is
1309:
is a topological space then the following are equivalent:
4799:
Rend. Dell' Accad. R. Delle Sci. dell'Istituto di Bologna
4590:(1929). "Mémoire sur les espaces topologiques compacts".
4125:
are compact open subsets, neither one of which is closed.
2930:{\textstyle \left({\frac {1}{n}},1-{\frac {1}{n}}\right)}
2400:
of any collection of compact spaces is compact. (This is
3169:
cover the extended real line. In fact, the set has the
2022:
Every increasing nested sequence of proper open subsets
1737:
Every increasing nested sequence of proper open subsets
3829:
is compact, again a consequence of Tychonoff's theorem.
3749:, and the quotient space is compact. This was used in
2944:
does not have a finite subcover. Similarly, the set of
439:
in 1929, exhibits compact spaces as generalizations of
229:
One such generalization is that a topological space is
82:
is a property that seeks to generalize the notion of a
52:
is not compact because it is not bounded. The interval
3369:
is a compact topological space; this follows from the
2960:
2887:
2619:
of a compact space having at most one point more than
388:
has no subsequence that converges to any real number.
4101:
4047:
4020:
3988:
3956:
3665:
3637:
3614:
3592:
3464:
3306:
3259:
3231:
3138:
3092:
3067:
2823:
2755:
2727:
2089:
1438:
1251:
1201:
1137:
1021:
957:
577:
defined on a closed and bounded interval was in fact
361:
187:
164:
142:
113:
4230:
is not compact since the collection of open subsets
2554:
is compact and Hausdorff, then no finer topology on
5436:
5400:
5286:
5207:
4671:
Annales Scientifiques de l'École Normale Supérieure
1245:Compactness is a topological property. That is, if
4936:
4109:
4055:
4028:
3996:
3964:
3673:
3650:
3622:
3600:
3556:
3334:
3278:
3239:
3146:
3100:
3075:
3046:
2929:
2831:
2770:
2735:
2493:are disjoint compact subsets of a Hausdorff space
2426:A finite set endowed with any topology is compact.
2125:
1456:
1269:
1235:
1171:
1055:
991:
616:, who also exploited it in the development of the
376:
196:
173:
150:
121:
27:"Compactness" redirects here. For other uses, see
5037:Transactions of the American Mathematical Society
4939:Mathematical thought from ancient to modern times
4666:"Sur quelques points de la théorie des fonctions"
637:
542:– or convergence in what would later be dubbed a
240:of points sampled from the space has an infinite
66:is compact because it is both closed and bounded.
5164:Creative Commons Attribution/Share-Alike License
3688:on a compact Hausdorff space is compact for the
3557:{\displaystyle d(f,g)=\sup _{x\in }|f(x)-g(x)|.}
3487:
2695:, is compact. More generally, any space with a
1906:has an infimum (i.e. a greatest lower bound) in
1236:{\displaystyle K\subseteq \bigcup _{S\in F}S\ .}
1172:{\displaystyle K\subseteq \bigcup _{S\in C}S\ ,}
509:. The culmination of their investigations, the
4783:Mem. Accad. Sci. Ist. Bologna Cl. Sci. Fis. Mat
2350:is not compact because its hyperreal extension
1621:has a convergent subsequence whose limit is in
1069:, typically influenced by the French school of
384:(the real number line), the sequence of points
244:that converges to some point of the space. The
4506:
4186:be the set of non-negative integers. We endow
2367:A closed subset of a compact space is compact.
1944:is compact. (The converse in general fails if
1698:is an image of a continuous function from the
1089:. A compact set is sometimes referred to as a
5185:
4339:
1895:has a supremum (i.e. a least upper bound) in
1121:is compact if for every arbitrary collection
1077:for the general notion, and reserve the term
8:
4832:Rendiconti del Circolo Matematico di Palermo
4566:
4370:
4366:"Generalisation d'un theorem de Weierstrass"
4364:
3564:Then by the Arzelà–Ascoli theorem the space
3329:
3307:
3273:
3260:
2678:(i.e. all subsets have suprema and infima).
2651:has a greatest element and a least element.
1625:(this is also equivalent to compactness for
1595:(this is also equivalent to compactness for
1374:has a convergent subnet (see the article on
4827:"Sur quelques points du calcul fonctionnel"
4449:, § 10.2. Theorem 1, Corollary 1.
3735:to the Cantor set, they form a compact set.
2415:, a subset is compact if and only if it is
455:
5553:
5526:
5192:
5178:
5170:
2450:is not Hausdorff then a compact subset of
1281:equipped with the subspace topology, then
803:, one could choose the sequence of points
5142:
5048:
4943:(3rd ed.). Oxford University Press.
4868:Gillman, Leonard; Jerison, Meyer (1976).
4683:
4103:
4102:
4100:
4049:
4048:
4046:
4022:
4021:
4019:
3990:
3989:
3987:
3958:
3957:
3955:
3667:
3666:
3664:
3642:
3636:
3616:
3615:
3613:
3594:
3593:
3591:
3546:
3514:
3490:
3463:
3314:
3305:
3267:
3258:
3233:
3232:
3230:
3140:
3139:
3137:
3094:
3093:
3091:
3069:
3068:
3066:
3023:
3010:
3000:
2985:
2972:
2959:
2912:
2893:
2886:
2825:
2824:
2822:
2762:
2758:
2757:
2754:
2729:
2728:
2726:
2594:image of a compact space is compact, the
2119:
2118:
2094:
2088:
2069:the ring of real continuous functions on
1812:is contained in some member of the cover.
1786:also satisfies the following properties:
1680:; that is, every countable open cover of
1554:, the following are equivalent (assuming
1437:
1250:
1212:
1200:
1148:
1136:
1032:
1020:
968:
956:
831:in particular is called compact if it is
546:. This ultimately led to the notion of a
368:
364:
363:
360:
186:
163:
144:
143:
141:
115:
114:
112:
5154:This article incorporates material from
4518:
4470:
4446:
3795:is compact, a fact which is part of the
3659:may have any compact nonempty subset of
2210:has residue field the real numbers. For
2053:Characterization by continuous functions
1613:is sequentially compact; that is, every
613:
598:
5028:Scarborough, C.T.; Stone, A.H. (1966).
4763:(2nd ed.). John Wiley & Sons.
4494:
4258:
4063:is open; the only open sets containing
3930:
3745:is a discrete additive subgroup of its
2325:) has the property that every point of
1056:{\displaystyle X=\bigcup _{S\in F}S\ .}
992:{\displaystyle X=\bigcup _{S\in C}S\ ,}
555:
475:
391:Compactness was formally introduced by
4434:
4173:is a compact set but it is not closed.
2719:Alexandroff one-point compactification
2625:Alexandroff one-point compactification
2558:is compact and no coarser topology on
2497:, then there exist disjoint open sets
2256:is compact if and only if every point
2202:if and only if every maximal ideal in
1986:Every monotone decreasing sequence in
1975:Every monotone increasing sequence in
4422:
4351:
4335:
3047:{\textstyle \left{\text{ and }}\left}
2341:. For example, an open real interval
2331:is infinitely close to some point of
2190:is the field of real numbers, by the
1081:for topological spaces that are both
1065:Some branches of mathematics such as
586:
7:
4458:
4306:
4304:
4075:; and the only open sets containing
3717:are compact, while groups such as a
3608:. Conversely, any compact subset of
3583:is a nonempty compact subset of the
2749:; the one-point compactification of
2721:. The one-point compactification of
2381:image of a compact space is compact.
1964:has a subsequence that converges in
1917:has a maximum and a minimum element.
1355:Any collection of closed subsets of
620:. Ultimately, the Russian school of
5030:"Products of nearly compact spaces"
4781:(1895). "Sulle funzioni di linee".
4704:Topologie générale. Chapitres 1 à 4
4555:Arkhangel'skii & Fedorchuk 1990
4543:Arkhangel'skii & Fedorchuk 1990
4531:Arkhangel'skii & Fedorchuk 1990
4483:Arkhangel'skii & Fedorchuk 1990
2627:. By the same construction, every
1270:{\displaystyle K\subset Z\subset Y}
941:is compact if for every collection
2870:is compact. This follows from the
2454:may fail to be a closed subset of
2321:(constructed, for example, by the
791:points of the interval, since the
191:
168:
25:
4117:, is not compact. Note that both
2647:A nonempty compact subset of the
482:. Bolzano's proof relied on the
100:of points. For example, the open
5589:Properties of topological spaces
5552:
5525:
5515:
5505:
5494:
5484:
5483:
5277:
5068:from the original on 2017-08-16.
4737:. New York: Dover Publications.
4473:, § 9.1. Definition 1.
4246:does not have a finite subcover.
3161:carrying the analogous topology
3154:but there is no finite subcover.
3054:cover all the rationals in for
2843:, no uncountable set is compact.
2771:{\displaystyle \mathbb {R} ^{2}}
1913:Every nonempty closed subset of
1506:is compact if and only if it is
1414:converges to at least one point.
377:{\displaystyle \mathbb {R} ^{1}}
4971:Canadian Journal of Mathematics
4316:www-groups.mcs.st-andrews.ac.uk
4297:. Warsaw, PL: PWN. p. 266.
1990:converges to a unique limit in
1979:converges to a unique limit in
638:Alexandrov & Urysohn (1929)
5162:, which is licensed under the
5005:. Princeton University Press.
4892:Science & Business Media.
4623:Arkhangel'skii, A.V. (2001) ,
4318:. MT 4522 course lectures
3547:
3543:
3537:
3528:
3522:
3515:
3509:
3497:
3480:
3468:
3326:
3320:
2778:is homeomorphic to the sphere
2743:is homeomorphic to the circle
2565:If a subset of a metric space
2115:
2112:
2106:
1457:{\displaystyle X\times Y\to Y}
1448:
859:, can be developed in general
1:
4886:Graduate Texts in Mathematics
4870:Rings of continuous functions
3822:is a compact Hausdorff space.
2539:A compact Hausdorff space is
2404:, which is equivalent to the
2155:is a ring homomorphism. The
618:integral now bearing his name
4882:Modern Analysis and Topology
4110:{\displaystyle \mathbb {N} }
4056:{\displaystyle \mathbb {N} }
4029:{\displaystyle \mathbb {N} }
3997:{\displaystyle \mathbb {N} }
3965:{\displaystyle \mathbb {N} }
3882:Noetherian topological space
3797:Stone representation theorem
3674:{\displaystyle \mathbb {C} }
3623:{\displaystyle \mathbb {C} }
3601:{\displaystyle \mathbb {C} }
3335:{\displaystyle \{f_{n}(x)\}}
3240:{\displaystyle \mathbb {R} }
3147:{\displaystyle \mathbb {R} }
3126:takes all integer values in
3101:{\displaystyle \mathbb {R} }
3076:{\displaystyle \mathbb {R} }
2832:{\displaystyle \mathbb {R} }
2736:{\displaystyle \mathbb {R} }
2586:Functions and compact spaces
2431:Properties of compact spaces
2229:such that the residue field
2019:has a nonempty intersection.
1734:has a nonempty intersection.
1428:For every topological space
1389:has a convergent refinement.
1361:finite intersection property
1336:Alexander's sub-base theorem
415:, were developed in general
151:{\displaystyle \mathbb {R} }
122:{\displaystyle \mathbb {Q} }
29:Compactness (disambiguation)
5084:Counterexamples in Topology
4630:Encyclopedia of Mathematics
4293:Engelking, Ryszard (1977).
4282:– via britannica.com.
4218:is compact, the closure of
3913:Relatively compact subspace
2799:is compact. In particular,
2474:(TVS), a compact subset is
2458:(see footnote for example).
2374:of compact sets is compact.
2061:be a topological space and
1423:complete accumulation point
841:Bolzano–Weierstrass theorem
246:Bolzano–Weierstrass theorem
5610:
5446:Banach fixed-point theorem
5156:Examples of compact spaces
4701:Bourbaki, Nicolas (2007).
4507:Gillman & Jerison 1976
4409:Here, "collection" means "
4204:to be open if and only if
3867:Exhaustion by compact sets
3455:the metric induced by the
2670:is compact if and only if
1952:is not also metrizable.):
1804:such that every subset of
1793:: For every open cover of
1363:has nonempty intersection.
810:In two dimensions, closed
534:. For a certain class of
34:Type of mathematical space
26:
5579:Compactness (mathematics)
5479:
5275:
5110:Willard, Stephen (1970).
4713:10.1007/978-3-540-33982-3
4365:
4340:Boyer & Merzbach 1991
4192:particular point topology
3852:Compactly generated space
3692:, by the Alaoglu theorem.
3651:{\displaystyle \ell ^{2}}
3279:{\displaystyle \{f_{n}\}}
3159:extended real number line
2260:of the natural extension
2212:completely regular spaces
2192:first isomorphism theorem
1417:Every infinite subset of
624:, under the direction of
4958:Lebesgue, Henri (1904).
4760:A History of Mathematics
4567:Steen & Seebach 1995
4312:"Sequential compactness"
4272:Encyclopaedia Britannica
3818:of a commutative unital
2689:finite topological space
2611:Every topological space
2472:topological vector space
1797:, there exists a number
1316:is compact; i.e., every
799:, since in the interval
197:{\displaystyle -\infty }
174:{\displaystyle +\infty }
4425:, pp. xxvi–xxviii.
3577:bounded linear operator
3157:On the other hand, the
2950:in the closed interval
2706:Any space carrying the
2323:ultrapower construction
1791:Lebesgue's number lemma
1774:A compact metric space
1109:of a topological space
857:limit point compactness
839:. This implies, by the
413:limit point compactness
405:Peano existence theorem
5501:Mathematics portal
5401:Metrics and properties
5387:Second-countable space
5114:. Dover publications.
5079:Seebach, J. Arthur Jr.
4983:10.4153/CJM-1967-059-0
4371:
4111:
4057:
4030:
3998:
3966:
3897:Quasi-compact morphism
3675:
3652:
3624:
3602:
3558:
3336:
3280:
3241:
3148:
3102:
3077:
3048:
2931:
2833:
2772:
2737:
2643:Ordered compact spaces
2577:is compact then it is
2436:A compact subset of a
2194:. A topological space
2127:
1684:has a finite subcover.
1458:
1271:
1237:
1173:
1101:Compactness of subsets
1057:
993:
853:sequential compactness
642:sequential compactness
466:Historical development
409:sequential compactness
378:
198:
175:
152:
123:
67:
5003:Non-standard analysis
4923:Kelley, John (1955).
4521:, Theorem 4.1.13
4194:by defining a subset
4112:
4058:
4031:
3999:
3967:
3676:
3653:
3625:
3603:
3559:
3378:Arzelà–Ascoli theorem
3359:pointwise convergence
3353:for all real numbers
3337:
3281:
3242:
3149:
3103:
3078:
3049:
2932:
2834:
2773:
2738:
2662:set endowed with the
2596:extreme value theorem
2362:Sufficient conditions
2250:non-standard analysis
2128:
2083:, the evaluation map
1869:For an ordered space
1542:For any metric space
1459:
1272:
1238:
1174:
1058:
994:
907:Open cover definition
884:Open cover definition
526:, as investigated by
511:Arzelà–Ascoli theorem
401:Arzelà–Ascoli theorem
379:
199:
176:
153:
124:
40:
5456:Invariance of domain
5408:Euler characteristic
5382:Bundle (mathematics)
4751:Boyer, Carl Benjamin
4707:. Berlin: Springer.
4651:. Wilhelm Engelmann.
4485:, Theorem 5.3.7
4372:Analyse Mathematique
4338:, pp. 952–953;
4099:
4045:
4018:
3986:
3954:
3805:totally disconnected
3719:general linear group
3686:probability measures
3663:
3635:
3612:
3590:
3462:
3425:| ≤ |
3304:
3257:
3229:
3136:
3090:
3065:
2958:
2885:
2878:is not compact: the
2874:. The open interval
2848:cocountable topology
2841:lower limit topology
2821:
2789:right order topology
2753:
2725:
2417:sequentially compact
2304:Hyperreal definition
2287:is contained in the
2087:
1936:) are true whenever
1436:
1403:has a cluster point.
1396:has a cluster point.
1249:
1199:
1135:
1019:
955:
901:uniformly continuous
843:, that any infinite
579:uniformly continuous
519:uniformly convergent
515:continuous functions
359:
185:
162:
140:
111:
5466:Tychonoff's theorem
5461:Poincaré conjecture
5215:General (point-set)
4964:. Gauthier-Villars.
4385:Weisstein, Eric W.
3857:Compactness theorem
3684:The space of Borel
3403:Lipschitz condition
3253:so that a sequence
3193:normed vector space
2872:Heine–Borel theorem
2797:totally ordered set
2793:left order topology
2402:Tychonoff's theorem
2314:hyperreal extension
2248:. The framework of
1648:limit point compact
1516:Heine–Borel theorem
1125:of open subsets of
945:of open subsets of
888:Heine–Borel theorem
874:– is defined using
847:from the set has a
603:Heine–Borel theorem
575:continuous function
499:spaces of functions
484:method of bisection
397:spaces of functions
133:, and the space of
43:Heine–Borel theorem
5451:De Rham cohomology
5372:Polyhedral complex
5362:Simplicial complex
5075:Steen, Lynn Arthur
4872:. Springer-Verlag.
4853:10338.dmlcz/100655
4845:10.1007/BF03018603
4685:10.24033/asens.406
4605:General Topology I
4497:Theorem 30.7.
4363:Frechet, M. 1904.
4153:with the topology
4107:
4053:
4026:
3994:
3962:
3887:Orthocompact space
3862:Eberlein compactum
3791:The spectrum of a
3711:Topological groups
3705:Algebraic examples
3671:
3648:
3620:
3598:
3554:
3513:
3342:converges towards
3332:
3290:converges towards
3276:
3237:
3144:
3098:
3073:
3044:
2927:
2829:
2768:
2733:
2312:is compact if its
2123:
1956:Every sequence in
1852:equipped with the
1454:
1267:
1233:
1223:
1169:
1159:
1067:algebraic geometry
1053:
1043:
989:
979:
868:topological spaces
669:accumulation point
622:point-set topology
524:integral equations
386:0, 1, 2, 3, ...
374:
224:topological spaces
194:
171:
148:
131:irrational numbers
119:
68:
5566:
5565:
5355:fundamental group
5094:978-0-486-68735-3
5012:978-0-691-04490-3
4999:Robinson, Abraham
4950:978-0-19-506136-9
4899:978-0-387-97986-1
4770:978-0-471-54397-8
4722:978-3-540-33982-3
4614:978-0-387-18178-3
4584:Alexandrov, Pavel
4557:, Corollary 5.2.1
4391:Wolfram MathWorld
3892:Paracompact space
3877:Metacompact space
3759:harmonic analysis
3486:
3387:of all functions
3383:Consider the set
3371:Tychonoff theorem
3220:of all functions
3216:Consider the set
3204:Alaoglu's theorem
3031:
3018:
3003:
2993:
2980:
2920:
2901:
2708:cofinite topology
2607:Compactifications
2283:(more precisely,
1808:of diameter <
1678:countably compact
1654:has at least one
1432:, the projection
1350:countably compact
1229:
1208:
1165:
1144:
1115:subspace topology
1049:
1028:
985:
964:
913:topological space
634:topological space
610:local information
536:Green's functions
488:Bolzano's theorem
460:topological space
238:infinite sequence
218:, but may not be
16:(Redirected from
5601:
5584:General topology
5556:
5555:
5529:
5528:
5519:
5509:
5499:
5498:
5487:
5486:
5281:
5194:
5187:
5180:
5171:
5148:
5146:
5125:
5112:General Topology
5106:
5069:
5067:
5052:
5034:
5024:
4994:
4965:
4954:
4942:
4928:
4925:General topology
4919:
4880:(23 June 1995).
4878:Howes, Norman R.
4873:
4864:
4823:Fréchet, Maurice
4818:
4802:
4790:
4774:
4746:
4726:
4697:
4687:
4652:
4643:Bolzano, Bernard
4637:
4618:
4599:
4570:
4564:
4558:
4552:
4546:
4540:
4534:
4528:
4522:
4516:
4510:
4504:
4498:
4492:
4486:
4480:
4474:
4468:
4462:
4456:
4450:
4444:
4438:
4432:
4426:
4420:
4414:
4407:
4401:
4400:
4398:
4397:
4382:
4376:
4374:
4368:
4367:
4361:
4355:
4354:, Chapter 46, §2
4349:
4343:
4333:
4327:
4326:
4324:
4323:
4308:
4299:
4298:
4295:General Topology
4290:
4284:
4283:
4281:
4280:
4263:
4247:
4245:
4229:
4225:
4221:
4217:
4210:
4203:
4189:
4185:
4180:
4174:
4172:
4164:
4152:
4148:
4132:
4126:
4124:
4120:
4116:
4114:
4113:
4108:
4106:
4094:
4090:
4086:
4082:
4078:
4074:
4070:
4066:
4062:
4060:
4059:
4054:
4052:
4040:
4036:
4035:
4033:
4032:
4027:
4025:
4004:
4003:
4001:
4000:
3995:
3993:
3972:
3971:
3969:
3968:
3963:
3961:
3935:
3809:profinite groups
3778:Zariski topology
3774:commutative ring
3728:
3715:orthogonal group
3680:
3678:
3677:
3672:
3670:
3657:
3655:
3654:
3649:
3647:
3646:
3629:
3627:
3626:
3621:
3619:
3607:
3605:
3604:
3599:
3597:
3567:
3563:
3561:
3560:
3555:
3550:
3518:
3512:
3457:uniform distance
3454:
3450:
3449:
3436:
3434:
3424:
3400:
3399:
3395:
3386:
3368:
3363:product topology
3356:
3352:
3341:
3339:
3338:
3333:
3319:
3318:
3299:
3289:
3285:
3283:
3282:
3277:
3272:
3271:
3252:
3248:
3246:
3244:
3243:
3238:
3236:
3219:
3197:closed unit ball
3188:
3183:
3153:
3151:
3150:
3145:
3143:
3131:
3125:
3121:
3120:
3118:
3114:
3107:
3105:
3104:
3099:
3097:
3082:
3080:
3079:
3074:
3072:
3060:
3058:
3053:
3051:
3050:
3045:
3043:
3039:
3032:
3024:
3019:
3011:
3004:
3001:
2999:
2995:
2994:
2986:
2981:
2973:
2953:
2947:rational numbers
2943:
2941:
2936:
2934:
2933:
2928:
2926:
2922:
2921:
2913:
2902:
2894:
2877:
2869:
2868:
2838:
2836:
2835:
2830:
2828:
2801:Sierpiński space
2783:
2777:
2775:
2774:
2769:
2767:
2766:
2761:
2748:
2742:
2740:
2739:
2734:
2732:
2701:trivial topology
2691:, including the
2676:complete lattice
2673:
2669:
2657:
2638:
2634:
2631:Hausdorff space
2622:
2614:
2580:
2576:
2561:
2557:
2553:
2528:
2518:
2508:
2504:
2500:
2496:
2492:
2488:
2464:
2457:
2453:
2449:
2442:
2421:countable choice
2413:metrizable space
2390:
2357:
2353:
2349:
2348:
2340:
2330:
2320:
2311:
2299:
2286:
2282:
2278:
2268:infinitely close
2265:
2259:
2255:
2239:
2228:
2220:
2209:
2197:
2189:
2188:
2167:
2154:
2132:
2130:
2129:
2124:
2122:
2099:
2098:
2082:
2072:
2068:
2060:
2047:
2043:
2032:
2025:
2018:
2007:
2000:
1993:
1989:
1982:
1978:
1971:
1963:
1951:
1943:
1934:countable choice
1932:, and (assuming
1931:
1916:
1909:
1905:
1902:Every subset of
1898:
1894:
1891:Every subset of
1887:
1876:
1847:
1843:
1828:second-countable
1825:
1811:
1807:
1803:
1796:
1785:
1769:
1765:
1753:
1733:
1721:
1697:
1683:
1675:
1661:
1653:
1645:
1624:
1620:
1612:
1586:
1572:
1556:countable choice
1553:
1533:
1505:
1497:
1478:
1463:
1461:
1460:
1455:
1431:
1420:
1413:
1402:
1399:Every filter on
1395:
1388:
1373:
1358:
1343:
1333:
1323:
1315:
1308:
1301:Characterization
1296:
1292:
1288:
1284:
1280:
1276:
1274:
1273:
1268:
1242:
1240:
1239:
1234:
1227:
1222:
1192:
1188:
1178:
1176:
1175:
1170:
1163:
1158:
1128:
1124:
1120:
1112:
1108:
1062:
1060:
1059:
1054:
1047:
1042:
1012:
1008:
998:
996:
995:
990:
983:
978:
948:
944:
940:
929:
917:
806:
802:
786:
784:
782:
781:
778:
775:
768:
766:
765:
762:
759:
752:
750:
749:
746:
743:
736:
734:
733:
730:
727:
720:
718:
717:
714:
711:
704:
702:
701:
698:
695:
688:
686:
685:
682:
679:
662:
626:Pavel Alexandrov
548:compact operator
540:mean convergence
492:Karl Weierstrass
456:compact subspace
433:Pavel Alexandrov
387:
383:
381:
380:
375:
373:
372:
367:
354:
350:
348:
346:
345:
342:
339:
332:
330:
329:
326:
323:
316:
314:
313:
310:
307:
300:
298:
297:
294:
291:
284:
282:
281:
278:
275:
268:
266:
265:
262:
259:
209:real number line
203:
201:
200:
195:
180:
178:
177:
172:
157:
155:
154:
149:
147:
128:
126:
125:
120:
118:
106:rational numbers
76:general topology
65:
58:
51:
21:
5609:
5608:
5604:
5603:
5602:
5600:
5599:
5598:
5569:
5568:
5567:
5562:
5493:
5475:
5471:Urysohn's lemma
5432:
5396:
5282:
5273:
5245:low-dimensional
5203:
5198:
5151:
5136:
5133:
5128:
5122:
5109:
5095:
5073:
5065:
5050:10.2307/1994440
5032:
5027:
5013:
4997:
4968:
4957:
4951:
4931:
4922:
4900:
4890:Springer-Verlag
4876:
4867:
4821:
4805:
4793:
4777:
4771:
4755:Merzbach, Uta C
4749:
4729:
4723:
4700:
4660:
4641:
4625:"Compact space"
4622:
4615:
4602:
4582:
4578:
4573:
4565:
4561:
4553:
4549:
4545:, Theorem 5.2.2
4541:
4537:
4533:, Theorem 5.2.3
4529:
4525:
4517:
4513:
4505:
4501:
4493:
4489:
4481:
4477:
4469:
4465:
4457:
4453:
4445:
4441:
4433:
4429:
4421:
4417:
4408:
4404:
4395:
4393:
4387:"Compact Space"
4384:
4383:
4379:
4362:
4358:
4350:
4346:
4334:
4330:
4321:
4319:
4310:
4309:
4302:
4292:
4291:
4287:
4278:
4276:
4265:
4264:
4260:
4256:
4251:
4250:
4231:
4227:
4223:
4219:
4212:
4205:
4195:
4187:
4183:
4181:
4177:
4166:
4154:
4150:
4135:
4133:
4129:
4122:
4118:
4097:
4096:
4092:
4088:
4084:
4080:
4076:
4072:
4068:
4064:
4043:
4042:
4038:
4016:
4015:
4006:
3984:
3983:
3974:
3952:
3951:
3938:
3936:
3932:
3927:
3922:
3918:Totally bounded
3907:totally bounded
3847:
3834:profinite group
3816:structure space
3793:Boolean algebra
3726:
3707:
3661:
3660:
3638:
3633:
3632:
3610:
3609:
3588:
3587:
3585:complex numbers
3565:
3460:
3459:
3452:
3451:. Consider on
3447:
3438:
3426:
3407:
3405:
3401:satisfying the
3397:
3393:
3388:
3384:
3366:
3354:
3343:
3310:
3302:
3301:
3300:if and only if
3291:
3287:
3263:
3255:
3254:
3250:
3227:
3226:
3221:
3217:
3186:
3181:
3134:
3133:
3127:
3123:
3116:
3112:
3110:
3109:
3088:
3087:
3063:
3062:
3056:
3055:
3009:
3005:
3002: and
2965:
2961:
2956:
2955:
2951:
2939:
2938:
2892:
2888:
2883:
2882:
2875:
2866:
2865:
2852:locally compact
2819:
2818:
2795:on any bounded
2779:
2756:
2751:
2750:
2744:
2723:
2722:
2715:locally compact
2697:finite topology
2684:
2671:
2667:
2655:
2645:
2636:
2632:
2629:locally compact
2620:
2612:
2609:
2588:
2578:
2566:
2559:
2555:
2551:
2520:
2510:
2506:
2502:
2498:
2494:
2490:
2486:
2462:
2455:
2451:
2447:
2440:
2438:Hausdorff space
2433:
2406:axiom of choice
2388:
2364:
2355:
2351:
2343:
2342:
2332:
2326:
2316:
2309:
2306:
2298:
2292:
2284:
2280:
2277:
2271:
2261:
2257:
2253:
2246:hyperreal field
2242:non-Archimedean
2230:
2222:
2218:
2203:
2195:
2187:
2177:
2176:
2166:
2160:
2140:
2134:
2090:
2085:
2084:
2074:
2070:
2062:
2058:
2055:
2045:
2044:fails to cover
2037:
2035:
2030:
2028:
2023:
2012:
2010:
2005:
2003:
1998:
1991:
1987:
1980:
1976:
1965:
1957:
1945:
1937:
1925:
1914:
1907:
1903:
1896:
1892:
1881:
1870:
1867:
1854:discrete metric
1845:
1841:
1815:
1809:
1805:
1798:
1794:
1775:
1767:
1766:fails to cover
1755:
1751:
1744:
1738:
1723:
1719:
1712:
1706:
1687:
1681:
1665:
1659:
1651:
1635:
1627:first-countable
1622:
1618:
1602:
1593:totally bounded
1576:
1562:
1543:
1540:
1531:
1523:Euclidean space
1503:
1500:Euclidean space
1495:
1489:
1487:Euclidean space
1476:
1434:
1433:
1429:
1418:
1411:
1400:
1393:
1386:
1371:
1356:
1341:
1331:
1321:
1313:
1306:
1303:
1294:
1290:
1289:if and only if
1286:
1282:
1278:
1247:
1246:
1197:
1196:
1190:
1186:
1133:
1132:
1126:
1122:
1118:
1110:
1106:
1103:
1073:, use the term
1017:
1016:
1010:
1006:
953:
952:
946:
942:
938:
927:
915:
909:
829:Euclidean space
825:
805:0, 1, 2, 3, ...
804:
800:
779:
776:
773:
772:
770:
763:
760:
757:
756:
754:
747:
744:
741:
740:
738:
731:
728:
725:
724:
722:
715:
712:
709:
708:
706:
699:
696:
693:
692:
690:
683:
680:
677:
676:
674:
672:
660:
650:
614:Lebesgue (1904)
552:Maurice Fréchet
472:Bernard Bolzano
468:
393:Maurice Fréchet
385:
362:
357:
356:
352:
343:
340:
337:
336:
334:
327:
324:
321:
320:
318:
311:
308:
305:
304:
302:
295:
292:
289:
288:
286:
279:
276:
273:
272:
270:
263:
260:
257:
256:
254:
253:
204:. However, the
183:
182:
160:
159:
138:
137:
109:
108:
97:limiting values
92:Euclidean space
74:, specifically
60:
53:
46:
45:, the interval
35:
32:
23:
22:
15:
12:
11:
5:
5607:
5605:
5597:
5596:
5591:
5586:
5581:
5571:
5570:
5564:
5563:
5561:
5560:
5550:
5549:
5548:
5543:
5538:
5523:
5513:
5503:
5491:
5480:
5477:
5476:
5474:
5473:
5468:
5463:
5458:
5453:
5448:
5442:
5440:
5434:
5433:
5431:
5430:
5425:
5420:
5418:Winding number
5415:
5410:
5404:
5402:
5398:
5397:
5395:
5394:
5389:
5384:
5379:
5374:
5369:
5364:
5359:
5358:
5357:
5352:
5350:homotopy group
5342:
5341:
5340:
5335:
5330:
5325:
5320:
5310:
5305:
5300:
5290:
5288:
5284:
5283:
5276:
5274:
5272:
5271:
5266:
5261:
5260:
5259:
5249:
5248:
5247:
5237:
5232:
5227:
5222:
5217:
5211:
5209:
5205:
5204:
5199:
5197:
5196:
5189:
5182:
5174:
5150:
5149:
5132:
5131:External links
5129:
5127:
5126:
5120:
5107:
5093:
5071:
5043:(1): 131–147.
5025:
5011:
4995:
4966:
4955:
4949:
4929:
4920:
4898:
4874:
4865:
4819:
4803:
4795:Arzelà, Cesare
4791:
4779:Arzelà, Cesare
4775:
4769:
4747:
4731:Boyer, Carl B.
4727:
4721:
4698:
4658:
4639:
4620:
4613:
4600:
4588:Urysohn, Pavel
4579:
4577:
4574:
4572:
4571:
4559:
4547:
4535:
4523:
4511:
4499:
4487:
4475:
4463:
4451:
4439:
4427:
4415:
4402:
4377:
4356:
4344:
4328:
4300:
4285:
4257:
4255:
4252:
4249:
4248:
4175:
4127:
4105:
4051:
4024:
3992:
3960:
3929:
3928:
3926:
3923:
3921:
3920:
3915:
3910:
3904:- also called
3902:Precompact set
3899:
3894:
3889:
3884:
3879:
3874:
3872:Lindelöf space
3869:
3864:
3859:
3854:
3848:
3846:
3843:
3842:
3841:
3830:
3823:
3820:Banach algebra
3812:
3789:
3766:
3761:to be used in
3736:
3729:-adic integers
3722:
3706:
3703:
3702:
3701:
3693:
3690:vague topology
3682:
3669:
3645:
3641:
3618:
3596:
3569:
3553:
3549:
3545:
3542:
3539:
3536:
3533:
3530:
3527:
3524:
3521:
3517:
3511:
3508:
3505:
3502:
3499:
3496:
3493:
3489:
3485:
3482:
3479:
3476:
3473:
3470:
3467:
3415:) −
3398:[0, 1]
3394:[0, 1]
3381:
3374:
3331:
3328:
3325:
3322:
3317:
3313:
3309:
3275:
3270:
3266:
3262:
3235:
3214:
3207:
3200:
3179:natural number
3175:
3155:
3142:
3096:
3084:
3071:
3042:
3038:
3035:
3030:
3027:
3022:
3017:
3014:
3008:
2998:
2992:
2989:
2984:
2979:
2976:
2971:
2968:
2964:
2925:
2919:
2916:
2911:
2908:
2905:
2900:
2897:
2891:
2867:[0, 1]
2859:
2844:
2827:
2815:
2808:discrete space
2804:
2785:
2765:
2760:
2731:
2711:
2704:
2683:
2680:
2664:order topology
2660:simply ordered
2644:
2641:
2617:dense subspace
2608:
2605:
2587:
2584:
2583:
2582:
2563:
2548:
2537:
2530:
2483:
2468:
2467:
2466:
2459:
2432:
2429:
2428:
2427:
2424:
2409:
2394:
2393:
2392:
2382:
2375:
2368:
2363:
2360:
2305:
2302:
2296:
2275:
2183:
2162:
2136:
2121:
2117:
2114:
2111:
2108:
2105:
2102:
2097:
2093:
2054:
2051:
2050:
2049:
2033:
2026:
2020:
2008:
2001:
1995:
1984:
1973:
1919:
1918:
1911:
1900:
1889:
1866:
1865:Ordered spaces
1863:
1862:
1861:
1839:
1813:
1772:
1771:
1749:
1742:
1735:
1717:
1710:
1703:
1685:
1663:
1633:
1630:uniform spaces
1600:
1597:uniform spaces
1574:
1539:
1536:
1514:; this is the
1488:
1485:
1481:
1480:
1473:
1466:closed mapping
1453:
1450:
1447:
1444:
1441:
1426:
1415:
1404:
1397:
1390:
1379:
1364:
1353:
1339:
1329:
1302:
1299:
1293:is compact in
1285:is compact in
1277:, with subset
1266:
1263:
1260:
1257:
1254:
1232:
1226:
1221:
1218:
1215:
1211:
1207:
1204:
1185:subcollection
1168:
1162:
1157:
1154:
1151:
1147:
1143:
1140:
1102:
1099:
1052:
1046:
1041:
1038:
1035:
1031:
1027:
1024:
1005:subcollection
988:
982:
977:
974:
971:
967:
963:
960:
908:
905:
878:consisting of
824:
821:
649:
648:Basic examples
646:
595:Henri Lebesgue
573:showed that a
532:Erhard Schmidt
467:
464:
371:
366:
193:
190:
170:
167:
146:
117:
33:
24:
14:
13:
10:
9:
6:
4:
3:
2:
5606:
5595:
5592:
5590:
5587:
5585:
5582:
5580:
5577:
5576:
5574:
5559:
5551:
5547:
5544:
5542:
5539:
5537:
5534:
5533:
5532:
5524:
5522:
5518:
5514:
5512:
5508:
5504:
5502:
5497:
5492:
5490:
5482:
5481:
5478:
5472:
5469:
5467:
5464:
5462:
5459:
5457:
5454:
5452:
5449:
5447:
5444:
5443:
5441:
5439:
5435:
5429:
5428:Orientability
5426:
5424:
5421:
5419:
5416:
5414:
5411:
5409:
5406:
5405:
5403:
5399:
5393:
5390:
5388:
5385:
5383:
5380:
5378:
5375:
5373:
5370:
5368:
5365:
5363:
5360:
5356:
5353:
5351:
5348:
5347:
5346:
5343:
5339:
5336:
5334:
5331:
5329:
5326:
5324:
5321:
5319:
5316:
5315:
5314:
5311:
5309:
5306:
5304:
5301:
5299:
5295:
5292:
5291:
5289:
5285:
5280:
5270:
5267:
5265:
5264:Set-theoretic
5262:
5258:
5255:
5254:
5253:
5250:
5246:
5243:
5242:
5241:
5238:
5236:
5233:
5231:
5228:
5226:
5225:Combinatorial
5223:
5221:
5218:
5216:
5213:
5212:
5210:
5206:
5202:
5195:
5190:
5188:
5183:
5181:
5176:
5175:
5172:
5168:
5167:
5165:
5161:
5157:
5145:
5140:
5135:
5134:
5130:
5123:
5121:0-486-43479-6
5117:
5113:
5108:
5104:
5100:
5096:
5090:
5086:
5085:
5080:
5076:
5072:
5064:
5060:
5056:
5051:
5046:
5042:
5038:
5031:
5026:
5022:
5018:
5014:
5008:
5004:
5000:
4996:
4992:
4988:
4984:
4980:
4976:
4972:
4967:
4963:
4962:
4956:
4952:
4946:
4941:
4940:
4934:
4933:Kline, Morris
4930:
4926:
4921:
4917:
4913:
4909:
4905:
4901:
4895:
4891:
4887:
4883:
4879:
4875:
4871:
4866:
4862:
4858:
4854:
4850:
4846:
4842:
4838:
4834:
4833:
4828:
4824:
4820:
4817:(3): 521–586.
4816:
4812:
4808:
4804:
4800:
4796:
4792:
4788:
4784:
4780:
4776:
4772:
4766:
4762:
4761:
4756:
4752:
4748:
4744:
4740:
4736:
4732:
4728:
4724:
4718:
4714:
4710:
4706:
4705:
4699:
4695:
4691:
4686:
4681:
4677:
4673:
4672:
4667:
4663:
4659:
4656:
4650:
4649:
4644:
4640:
4636:
4632:
4631:
4626:
4621:
4616:
4610:
4606:
4601:
4597:
4593:
4589:
4585:
4581:
4580:
4575:
4568:
4563:
4560:
4556:
4551:
4548:
4544:
4539:
4536:
4532:
4527:
4524:
4520:
4519:Robinson 1996
4515:
4512:
4508:
4503:
4500:
4496:
4491:
4488:
4484:
4479:
4476:
4472:
4471:Bourbaki 2007
4467:
4464:
4460:
4455:
4452:
4448:
4447:Bourbaki 2007
4443:
4440:
4437:, p. 163
4436:
4431:
4428:
4424:
4419:
4416:
4412:
4406:
4403:
4392:
4388:
4381:
4378:
4373:
4360:
4357:
4353:
4348:
4345:
4342:, p. 561
4341:
4337:
4332:
4329:
4317:
4313:
4307:
4305:
4301:
4296:
4289:
4286:
4275:. mathematics
4274:
4273:
4268:
4267:"Compactness"
4262:
4259:
4253:
4243:
4239:
4235:
4215:
4209:
4202:
4198:
4193:
4179:
4176:
4170:
4162:
4158:
4146:
4142:
4138:
4131:
4128:
4013:
4009:
3981:
3977:
3949:
3945:
3941:
3934:
3931:
3924:
3919:
3916:
3914:
3911:
3909:
3908:
3903:
3900:
3898:
3895:
3893:
3890:
3888:
3885:
3883:
3880:
3878:
3875:
3873:
3870:
3868:
3865:
3863:
3860:
3858:
3855:
3853:
3850:
3849:
3844:
3840:) is compact.
3839:
3835:
3831:
3828:
3824:
3821:
3817:
3813:
3810:
3806:
3802:
3798:
3794:
3790:
3787:
3783:
3779:
3775:
3771:
3767:
3764:
3763:number theory
3760:
3756:
3752:
3748:
3744:
3741:
3737:
3734:
3730:
3723:
3720:
3716:
3712:
3709:
3708:
3704:
3698:
3694:
3691:
3687:
3683:
3658:
3643:
3639:
3586:
3582:
3578:
3574:
3570:
3551:
3540:
3534:
3531:
3525:
3519:
3506:
3503:
3500:
3494:
3491:
3483:
3477:
3474:
3471:
3465:
3458:
3448:[0,1]
3446: ∈
3445:
3441:
3433:
3430: −
3429:
3422:
3418:
3414:
3410:
3404:
3391:
3382:
3379:
3375:
3372:
3364:
3360:
3350:
3346:
3323:
3315:
3311:
3298:
3294:
3268:
3264:
3224:
3215:
3212:
3208:
3205:
3201:
3198:
3194:
3190:
3180:
3176:
3172:
3171:homeomorphism
3168:
3164:
3160:
3156:
3130:
3085:
3040:
3036:
3033:
3028:
3025:
3020:
3015:
3012:
3006:
2996:
2990:
2987:
2982:
2977:
2974:
2969:
2966:
2962:
2952:[0,1]
2949:
2948:
2923:
2917:
2914:
2909:
2906:
2903:
2898:
2895:
2889:
2881:
2873:
2864:
2863:unit interval
2860:
2857:
2854:but is still
2853:
2849:
2845:
2842:
2839:carrying the
2816:
2813:
2809:
2805:
2802:
2798:
2794:
2790:
2786:
2782:
2763:
2747:
2720:
2716:
2712:
2709:
2705:
2702:
2698:
2694:
2690:
2686:
2685:
2681:
2679:
2677:
2665:
2661:
2652:
2650:
2642:
2640:
2630:
2626:
2618:
2606:
2604:
2602:
2597:
2593:
2585:
2574:
2570:
2564:
2562:is Hausdorff.
2549:
2546:
2542:
2538:
2535:
2534:homeomorphism
2531:
2527:
2523:
2517:
2513:
2484:
2481:
2477:
2473:
2469:
2460:
2445:
2444:
2439:
2435:
2434:
2430:
2425:
2422:
2418:
2414:
2410:
2407:
2403:
2399:
2395:
2386:
2385:
2383:
2380:
2376:
2373:
2369:
2366:
2365:
2361:
2359:
2346:
2339:
2335:
2329:
2324:
2319:
2315:
2303:
2301:
2295:
2290:
2274:
2269:
2264:
2251:
2247:
2243:
2238:
2234:
2226:
2215:
2213:
2207:
2201:
2200:pseudocompact
2193:
2186:
2181:
2175:
2174:residue field
2171:
2170:maximal ideal
2165:
2158:
2152:
2148:
2144:
2139:
2109:
2103:
2100:
2095:
2091:
2081:
2077:
2066:
2052:
2041:
2021:
2016:
1996:
1985:
1974:
1969:
1961:
1955:
1954:
1953:
1949:
1941:
1935:
1929:
1922:
1912:
1901:
1890:
1885:
1880:
1879:
1878:
1874:
1864:
1859:
1855:
1851:
1840:
1837:
1833:
1829:
1823:
1819:
1814:
1801:
1792:
1789:
1788:
1787:
1783:
1779:
1763:
1759:
1748:
1741:
1736:
1731:
1727:
1716:
1709:
1704:
1701:
1695:
1691:
1686:
1679:
1673:
1669:
1664:
1657:
1649:
1643:
1639:
1634:
1631:
1628:
1616:
1610:
1606:
1601:
1598:
1594:
1590:
1584:
1580:
1575:
1570:
1566:
1561:
1560:
1559:
1557:
1551:
1547:
1538:Metric spaces
1537:
1535:
1529:
1524:
1519:
1517:
1513:
1509:
1501:
1494:
1486:
1484:
1474:
1471:
1467:
1451:
1445:
1442:
1439:
1427:
1424:
1416:
1409:
1405:
1398:
1392:Every net on
1391:
1384:
1380:
1378:for a proof).
1377:
1369:
1365:
1362:
1354:
1351:
1347:
1340:
1337:
1330:
1327:
1324:has a finite
1319:
1312:
1311:
1310:
1300:
1298:
1264:
1261:
1258:
1255:
1252:
1243:
1230:
1224:
1219:
1216:
1213:
1209:
1205:
1202:
1194:
1184:
1179:
1166:
1160:
1155:
1152:
1149:
1145:
1141:
1138:
1130:
1116:
1100:
1098:
1096:
1092:
1088:
1087:quasi-compact
1084:
1080:
1076:
1075:quasi-compact
1072:
1068:
1063:
1050:
1044:
1039:
1036:
1033:
1029:
1025:
1022:
1014:
1004:
999:
986:
980:
975:
972:
969:
965:
961:
958:
950:
936:
933:
925:
921:
914:
906:
904:
902:
897:
896:neighbourhood
893:
889:
885:
881:
877:
873:
872:bicompactness
869:
864:
862:
861:metric spaces
858:
854:
850:
846:
842:
838:
834:
830:
822:
820:
818:
813:
808:
798:
794:
790:
670:
666:
661:[0,1]
659:
658:unit interval
655:
647:
645:
643:
639:
635:
631:
630:Pavel Urysohn
627:
623:
619:
615:
611:
606:
604:
600:
596:
592:
591:Pierre Cousin
588:
584:
580:
576:
572:
568:
563:
561:
557:
553:
549:
545:
544:Hilbert space
541:
537:
533:
529:
528:David Hilbert
525:
520:
516:
512:
508:
507:Cesare Arzelà
504:
503:Giulio Ascoli
500:
495:
493:
489:
485:
481:
477:
473:
465:
463:
461:
457:
453:
448:
446:
442:
438:
437:Pavel Urysohn
434:
430:
426:
422:
418:
417:metric spaces
414:
410:
406:
402:
398:
394:
389:
369:
251:
250:unit interval
247:
243:
239:
235:
233:
227:
225:
221:
217:
213:
210:
208:
188:
165:
136:
132:
107:
103:
99:
98:
93:
89:
85:
81:
77:
73:
63:
56:
49:
44:
39:
30:
19:
18:Quasi-compact
5558:Publications
5423:Chern number
5413:Betti number
5317:
5296: /
5287:Key concepts
5235:Differential
5153:
5152:
5111:
5083:
5040:
5036:
5002:
4974:
4970:
4960:
4938:
4924:
4888:. New York:
4881:
4869:
4836:
4830:
4814:
4810:
4798:
4786:
4782:
4759:
4734:
4703:
4675:
4669:
4662:Borel, Émile
4654:
4647:
4628:
4604:
4595:
4591:
4576:Bibliography
4569:, p. 67
4562:
4550:
4538:
4526:
4514:
4502:
4495:Willard 1970
4490:
4478:
4466:
4454:
4442:
4430:
4418:
4405:
4394:. Retrieved
4390:
4380:
4359:
4347:
4331:
4320:. Retrieved
4315:
4294:
4288:
4277:. Retrieved
4270:
4261:
4241:
4237:
4233:
4216: := {0}
4213:
4207:
4200:
4196:
4178:
4168:
4160:
4156:
4144:
4140:
4136:
4130:
4011:
4007:
3979:
3975:
3947:
3943:
3939:
3933:
3905:
3838:Galois group
3827:Hilbert cube
3801:Stone spaces
3742:
3740:global field
3733:homeomorphic
3696:
3681:as spectrum.
3581:Banach space
3443:
3439:
3431:
3427:
3420:
3416:
3412:
3408:
3389:
3348:
3344:
3296:
3292:
3222:
3166:
3162:
3128:
3059:= 4, 5, ...
2945:
2942:= 3, 4, ...
2780:
2745:
2653:
2649:real numbers
2646:
2610:
2603:is compact.
2589:
2572:
2568:
2525:
2521:
2515:
2511:
2479:
2344:
2337:
2333:
2327:
2317:
2307:
2293:
2272:
2262:
2236:
2232:
2224:
2216:
2205:
2184:
2179:
2172:, since the
2163:
2150:
2146:
2142:
2137:
2079:
2075:
2073:. For each
2064:
2056:
2039:
2014:
1967:
1959:
1947:
1939:
1927:
1923:
1920:
1883:
1872:
1868:
1821:
1817:
1799:
1781:
1777:
1773:
1761:
1757:
1746:
1739:
1729:
1725:
1714:
1707:
1693:
1689:
1671:
1667:
1641:
1637:
1608:
1604:
1582:
1578:
1568:
1564:
1549:
1545:
1541:
1520:
1490:
1482:
1304:
1244:
1195:
1182:
1180:
1131:
1117:). That is,
1104:
1094:
1090:
1086:
1078:
1074:
1064:
1015:
1002:
1000:
951:
919:
911:Formally, a
910:
883:
871:
865:
826:
816:
809:
793:limit points
665:real numbers
654:finite space
651:
607:
571:Eduard Heine
564:
559:
496:
469:
451:
449:
420:
390:
232:sequentially
231:
228:
216:metric space
211:
206:
135:real numbers
96:
79:
69:
61:
54:
47:
5521:Wikiversity
5438:Key results
5144:1006.4131v1
4977:: 649–654.
4839:(1): 1–72.
4789:(5): 55–74.
4435:Kelley 1955
3713:such as an
3568:is compact.
3199:is compact.
2861:The closed
2803:is compact.
2710:is compact.
2615:is an open
2550:If a space
2443:is closed.
2270:to a point
1888:is compact.
1656:limit point
1573:is compact.
1408:ultrafilter
1181:there is a
1001:there is a
937:. That is,
849:subsequence
823:Definitions
593:(1895) and
583:Émile Borel
560:compactness
480:limit point
452:compact set
441:finite sets
421:compactness
242:subsequence
80:compactness
72:mathematics
5573:Categories
5367:CW complex
5308:Continuity
5298:Closed set
5257:cohomology
5160:PlanetMath
4807:Ascoli, G.
4801:: 142–159.
4694:26.0429.03
4423:Howes 1995
4396:2019-11-25
4352:Kline 1990
4336:Kline 1990
4322:2019-11-25
4279:2019-11-25
4254:References
4222:is all of
4149:and endow
4014:} ∪
3982:} ∪
3950:} ∪
3803:, compact
3747:adele ring
3724:Since the
3211:Cantor set
3177:For every
2880:open cover
2812:singletons
2601:proper map
2592:continuous
2509:such that
2419:(assuming
2379:continuous
1858:singletons
1700:Cantor set
1530:or closed
1470:proper map
1318:open cover
1193:such that
1129:such that
1013:such that
949:such that
924:open cover
918:is called
220:equivalent
90:subset of
50:= (−∞, −2]
5546:geometric
5541:algebraic
5392:Cobordism
5328:Hausdorff
5323:connected
5240:Geometric
5230:Continuum
5220:Algebraic
5081:(1995) .
4935:(1990) .
4861:123251660
4635:EMS Press
4459:Mack 1967
4236:} :
4190:with the
3782:Hausdorff
3776:with the
3757:to allow
3751:John Tate
3640:ℓ
3532:−
3495:∈
3174:inferred.
3016:π
2983:−
2978:π
2910:−
2693:empty set
2623:, by the
2581:-bounded.
2370:A finite
2133:given by
2116:→
2101::
2036:⊆ ... in
2011:⊇ ... in
1850:real line
1832:separable
1449:→
1443:×
1359:with the
1262:⊂
1256:⊂
1217:∈
1210:⋃
1206:⊆
1153:∈
1146:⋃
1142:⊆
1105:A subset
1093:, plural
1091:compactum
1083:Hausdorff
1037:∈
1030:⋃
973:∈
966:⋃
922:if every
880:open sets
801:[0,∞)
567:continuum
450:The term
425:open sets
236:if every
222:in other
192:∞
189:−
169:∞
5594:Topology
5511:Wikibook
5489:Category
5377:Manifold
5345:Homotopy
5303:Interior
5294:Open set
5252:Homology
5201:Topology
5063:Archived
5001:(1996).
4916:1272666M
4908:31969970
4825:(1906).
4757:(1991).
4733:(1959).
4678:: 9–55.
4664:(1895).
4645:(1817).
4037:. Endow
3845:See also
3770:spectrum
3721:are not.
3573:spectrum
3437:for all
3396: →
3392: :
3225: :
3132:, cover
3122:, where
3086:The set
2856:Lindelöf
2682:Examples
2666:. Then
2590:Since a
2476:complete
2347:= (0, 1)
2308:A space
2182:)/ker ev
1836:Lindelöf
1615:sequence
1589:complete
1528:interval
1491:For any
1346:Lindelöf
1326:subcover
1095:compacta
1071:Bourbaki
935:subcover
845:sequence
789:boundary
554:who, in
403:and the
207:extended
102:interval
57:= (2, 4)
5536:general
5338:uniform
5318:compact
5269:Digital
5103:0507446
5059:1994440
5021:0205854
4991:0211382
4743:0124178
4211:. Then
4165:. Then
4087:. Then
3786:schemes
3772:of any
3575:of any
3442:,
3365:. Then
3361:or the
3189:-sphere
2846:In the
2545:regular
2482:closed.
2470:In any
2398:product
2042:, <)
2017:, <)
1970:, <)
1962:, <)
1950:, <)
1942:, <)
1930:, <)
1886:, <)
1875:, <)
1534:-ball.
1512:bounded
1079:compact
920:compact
894:– in a
892:locally
837:bounded
797:bounded
783:
771:
767:
755:
751:
739:
735:
723:
719:
707:
703:
691:
687:
675:
601:). The
445:locally
347:
335:
331:
319:
315:
303:
299:
287:
283:
271:
267:
255:
234:compact
88:bounded
5531:Topics
5333:metric
5208:Fields
5118:
5101:
5091:
5057:
5019:
5009:
4989:
4947:
4914:
4906:
4896:
4859:
4767:
4741:
4719:
4692:
4611:
4509:, §5.6
4226:, but
4159:, ∅, {
4005:, and
3836:(e.g.
3755:thesis
3700:tight.
3435:|
3406:|
3184:, the
2876:(0, 1)
2541:normal
2352:*(0,1)
2240:is a (
2157:kernel
1802:> 0
1508:closed
1493:subset
1421:has a
1406:Every
1383:filter
1381:Every
1366:Every
1228:
1183:finite
1164:
1048:
1003:finite
984:
932:finite
930:has a
876:covers
833:closed
817:within
427:that "
399:. The
353:(0, 1)
84:closed
5313:Space
5139:arXiv
5066:(PDF)
5055:JSTOR
5033:(PDF)
4857:S2CID
4674:. 3.
4232:{{0,
3925:Notes
3697:tight
3579:on a
3115:− 1,
2674:is a
2658:be a
2411:In a
2372:union
2289:monad
2168:is a
1752:⊆ ...
1720:⊇ ...
1521:As a
1468:(see
1464:is a
882:(see
812:disks
785:, ...
458:of a
429:cover
349:, ...
212:would
5116:ISBN
5089:ISBN
5007:ISBN
4945:ISBN
4904:OCLC
4894:ISBN
4765:ISBN
4717:ISBN
4609:ISBN
4206:0 ∈
4182:Let
4134:Let
4121:and
4091:and
4083:and
4079:are
4071:and
4067:are
3937:Let
3825:The
3814:The
3768:The
3738:Any
3731:are
3571:The
3209:The
3119:+ 1)
2937:for
2787:The
2713:Any
2687:Any
2654:Let
2543:and
2519:and
2501:and
2489:and
2396:The
2145:) =
2057:Let
1834:and
1591:and
1510:and
1376:nets
1348:and
1085:and
855:and
835:and
652:Any
628:and
599:1904
587:1895
556:1906
530:and
505:and
476:1817
435:and
411:and
181:and
86:and
5158:on
5045:doi
5041:124
4979:doi
4849:hdl
4841:doi
4709:doi
4690:JFM
4680:doi
4411:set
4139:= {
4010:= {
3978:= {
3942:= {
3753:'s
3488:sup
3286:in
3167:not
2817:In
2806:No
2791:or
2505:in
2485:If
2480:not
2461:If
2446:If
2387:If
2300:).
2291:of
2279:of
2266:is
2221:in
2198:is
2159:of
1826:is
1754:in
1722:in
1676:is
1658:in
1646:is
1617:in
1587:is
1558:):
1498:of
1410:on
1385:on
1370:on
1368:net
1344:is
1320:of
1305:If
926:of
673:1,
663:of
70:In
5575::
5099:MR
5097:.
5077:;
5061:.
5053:.
5039:.
5035:.
5017:MR
5015:.
4987:MR
4985:.
4975:19
4973:.
4912:OL
4910:.
4902:.
4884:.
4855:.
4847:.
4837:22
4835:.
4829:.
4815:18
4813:.
4785:.
4753:;
4739:MR
4715:.
4688:.
4676:12
4668:.
4657:).
4633:,
4627:,
4596:14
4594:.
4586:;
4389:.
4369:.
4314:.
4303:^
4269:.
4240:∈
4199:⊆
4163:}}
4143:,
3973:,
3946:,
3832:A
3799:.
3380:).
3295:∈
3247:→
3163:is
2639:.
2571:,
2524:⊆
2514:⊆
2408:.)
2377:A
2358:.
2338:*X
2336:⊂
2328:*X
2318:*X
2263:*X
2244:)
2235:)/
2231:C(
2223:C(
2204:C(
2178:C(
2161:ev
2135:ev
2092:ev
2078:∈
2063:C(
2029:⊆
2004:⊇
1830:,
1820:,
1780:,
1760:,
1745:⊆
1728:,
1713:⊇
1692:,
1670:,
1640:,
1632:).
1607:,
1599:).
1581:,
1567:,
1548:,
1518:.
1502:,
1472:).
1338:).
1297:.
1189:⊆
1097:.
1009:⊆
863:.
769:,
753:,
737:,
721:,
705:,
689:,
636:.
494:.
462:.
333:,
317:,
301:,
285:,
269:,
226:.
78:,
64:=
5193:e
5186:t
5179:v
5166:.
5147:.
5141::
5124:.
5105:.
5070:.
5047::
5023:.
4993:.
4981::
4953:.
4918:.
4863:.
4851::
4843::
4787:5
4773:.
4745:.
4725:.
4711::
4696:.
4682::
4653:(
4638:.
4619:.
4617:.
4598:.
4461:.
4399:.
4375:.
4325:.
4244:}
4242:X
4238:x
4234:x
4228:X
4224:X
4220:S
4214:S
4208:U
4201:X
4197:U
4188:X
4184:X
4171:}
4169:a
4167:{
4161:a
4157:X
4155:{
4151:X
4147:}
4145:b
4141:a
4137:X
4123:V
4119:U
4104:N
4093:V
4089:U
4085:V
4081:X
4077:b
4073:U
4069:X
4065:a
4050:N
4039:X
4023:N
4012:b
4008:V
3991:N
3980:a
3976:U
3959:N
3948:b
3944:a
3940:X
3811:.
3765:.
3743:K
3727:p
3668:C
3644:2
3617:C
3595:C
3566:K
3552:.
3548:|
3544:)
3541:x
3538:(
3535:g
3529:)
3526:x
3523:(
3520:f
3516:|
3510:]
3507:1
3504:,
3501:0
3498:[
3492:x
3484:=
3481:)
3478:g
3475:,
3472:f
3469:(
3466:d
3453:K
3444:y
3440:x
3432:y
3428:x
3423:)
3421:y
3419:(
3417:f
3413:x
3411:(
3409:f
3390:f
3385:K
3373:.
3367:K
3355:x
3351:)
3349:x
3347:(
3345:f
3330:}
3327:)
3324:x
3321:(
3316:n
3312:f
3308:{
3297:K
3293:f
3288:K
3274:}
3269:n
3265:f
3261:{
3251:K
3234:R
3223:f
3218:K
3206:)
3187:n
3182:n
3141:R
3129:Z
3124:n
3117:n
3113:n
3111:(
3095:R
3083:.
3070:R
3057:n
3041:]
3037:1
3034:,
3029:n
3026:1
3021:+
3013:1
3007:[
2997:]
2991:n
2988:1
2975:1
2970:,
2967:0
2963:[
2940:n
2924:)
2918:n
2915:1
2907:1
2904:,
2899:n
2896:1
2890:(
2858:.
2826:R
2781:S
2764:2
2759:R
2746:S
2730:R
2703:.
2672:X
2668:X
2656:X
2637:X
2633:X
2621:X
2613:X
2579:d
2575:)
2573:d
2569:X
2567:(
2560:X
2556:X
2552:X
2547:.
2536:.
2529:.
2526:V
2522:B
2516:U
2512:A
2507:X
2503:V
2499:U
2495:X
2491:B
2487:A
2463:X
2456:X
2452:X
2448:X
2441:X
2423:)
2389:X
2356:X
2345:X
2334:X
2310:X
2297:0
2294:x
2285:x
2281:X
2276:0
2273:x
2258:x
2254:X
2237:m
2233:X
2227:)
2225:X
2219:m
2208:)
2206:X
2196:X
2185:p
2180:X
2164:p
2153:)
2151:p
2149:(
2147:f
2143:f
2141:(
2138:p
2120:R
2113:)
2110:X
2107:(
2104:C
2096:p
2080:X
2076:p
2071:X
2067:)
2065:X
2059:X
2048:.
2046:X
2040:X
2038:(
2034:2
2031:S
2027:1
2024:S
2015:X
2013:(
2009:2
2006:S
2002:1
1999:S
1994:.
1992:X
1988:X
1983:.
1981:X
1977:X
1972:.
1968:X
1966:(
1960:X
1958:(
1948:X
1946:(
1940:X
1938:(
1928:X
1926:(
1915:X
1910:.
1908:X
1904:X
1899:.
1897:X
1893:X
1884:X
1882:(
1873:X
1871:(
1846:d
1842:X
1824:)
1822:d
1818:X
1816:(
1810:δ
1806:X
1800:δ
1795:X
1784:)
1782:d
1778:X
1776:(
1770:.
1768:X
1764:)
1762:d
1758:X
1756:(
1750:2
1747:S
1743:1
1740:S
1732:)
1730:d
1726:X
1724:(
1718:2
1715:S
1711:1
1708:S
1702:.
1696:)
1694:d
1690:X
1688:(
1682:X
1674:)
1672:d
1668:X
1666:(
1662:.
1660:X
1652:X
1644:)
1642:d
1638:X
1636:(
1623:X
1619:X
1611:)
1609:d
1605:X
1603:(
1585:)
1583:d
1579:X
1577:(
1571:)
1569:d
1565:X
1563:(
1552:)
1550:d
1546:X
1544:(
1532:n
1504:A
1496:A
1479:.
1477:X
1452:Y
1446:Y
1440:X
1430:Y
1425:.
1419:X
1412:X
1401:X
1394:X
1387:X
1372:X
1357:X
1352:.
1342:X
1332:X
1328:.
1322:X
1314:X
1307:X
1295:Y
1291:K
1287:Z
1283:K
1279:Z
1265:Y
1259:Z
1253:K
1231:.
1225:S
1220:F
1214:S
1203:K
1191:C
1187:F
1167:,
1161:S
1156:C
1150:S
1139:K
1127:X
1123:C
1119:K
1111:X
1107:K
1051:.
1045:S
1040:F
1034:S
1026:=
1023:X
1011:C
1007:F
987:,
981:S
976:C
970:S
962:=
959:X
947:X
943:C
939:X
928:X
916:X
780:8
777:/
774:7
764:7
761:/
758:1
748:6
745:/
742:5
732:5
729:/
726:1
716:4
713:/
710:3
700:3
697:/
694:1
684:2
681:/
678:1
597:(
585:(
474:(
370:1
365:R
344:7
341:/
338:6
328:4
325:/
322:1
312:6
309:/
306:5
296:3
293:/
290:1
280:5
277:/
274:4
264:2
261:/
258:1
166:+
145:R
116:Q
62:B
55:C
48:A
31:.
20:)
Text is available under the Creative Commons Attribution-ShareAlike License. Additional terms may apply.